-
Posts
520 -
Joined
-
Last visited
-
Days Won
1
Content Type
Profiles
Forums
Events
Everything posted by Trurl
-

Mathematical Formula to Determine Object Size at Various Distances
Trurl replied to chr2019's topic in Mathematics
I never studied optics in physics, but can you guys tell me if a lense would go linear? It sounds like chr2019 is working on a video game. I have a vr headset and distances don't focus in distance to reading writing on the viewpoint. This is why a standard viewpoint using trig and an angle would work for a rifle simulator and eye chart but what do you do if you want to emulate a person's viewpoint? i realize you could always have a linear viewpoint calculated for different perspectives. But I am not skilled enough as a programmer to do this. It seems too memory and computational anyway. There are already Unity and other tools libraries. Isn't this a major problem in optics. My eye doctor said if I could invent glasses that focus near and far I'd be a millionaire. -
We can argue back and forth in all good fun. Your believe in some sort of Creator. I guess you just had an experience, maybe science that made you think otherwise. There are Christian scientist. And a lot of scientist believe in creation designed. Depeding on your beliefs, they determine how you interpret the Bible. You can apply pure science, but that won't always lead to answers. But to me the answers are found by study and research. Reading the Words in red, I see things that apply to family and relationships with other people. I my opinion if I follow the given examples I will live a more meaningful life. I say a guy interviewed on YouTube that had nothing to do with religion. He had a 160 iq and was living modestly on a farm. The reporters ask him why he wasn't a millionaire and he replied that he lived the way that made him happy. I don't think we were born evil. But obviously we are imperfect. This thread is just my opinion. But I don't see the Bible's purpose to convert everyone to Christianity. The goal is to present the Word and let the reader decide what it means to them. Obviosly not everything in the Bible makes sense to me. But neither does every theory in science. But just because I do not understand something make it invalid.
-
The original post does refer to Pastor Doug's description of slave. This is simply meaning only the Jewish people should participate. Even the slave can eat. Simple enough. This is not a new question. Anyone who reads the Bible will probably ask this. IMHO anything can be ask but there is no easy way to settle the debate. We don't have a way to know why things are the way they are other than history, the Bible, and science. To me it would be like blaming the leader of a country for everything that is wrong in the country. It would also mean we have no choice in our actions which is a whole other debate. But I think what is looked over how important the present is. It isn't like this stuff is just in the past, we are living it.
- 21 replies
-
-2
-
No Pastor Doug is correct. He is referring to Hebrew slaves. It is my explanation that is wrong. Christains don't deny bad things happen in the Bible. We are not blindly following the Bible. Nor with good Pastors we are not being lied to. The Old Testament is difficult to understand. The Old Testiment was Hebrew law. But the question is unanswerable. And no Christian can answer it. But one unanswered question does discredit the Bible. In my own opinion, the question is not why God allows slavery, but why does Man allow slavery. Why is God or how is God responsible?
- 21 replies
-
-2
-
As was explained to me by Pastor Doug, slavery in the Bible is not like slavery that led to civil rights in the U.S. A person could become a slave to pay a debt or support themselves financially. They wouldn't be treated like the slaves of the United State's South. But slavery relates to service. It is important to serve. That would be the meaning in the New Testament. But I admit is does sound confusing in certain verses. I cannot interpret them for you. There would be a reason for the wording, but you would have study it verse by verse. But don't think because it says slavery it means the way we know it. The Bible is difficult to understand without a knowledgeable person to help put it into context. Often you have to know the history.
-
Solve for x. I don't have the math background to solve for x. But if you put in N and p, you will see this simplified equation. I plugged it into Wolfram Alpha, but solving for x is still a challenge. x^2 + (2 x^5)/N^2 alternate form (x^2 * (N^2 + 2 * x^3) / N^2 Plug in the known value N. Still impossible to solve. But if you plug in both x and N it proves true. Do you believe me now?
-
Don’t let me distract you on your original idea. I know how important it is to pursue your vision. I like how your matrices did not have to rely on the previous matrices. I don’t understand the patterns you are using or what your method is. Maybe you could explain in a book format. What interests me now is if you can take my equation and find a pattern between Prime numbers. N^2 = ((((p^2 * N^4 + 2 * N^2 * p^5) + p^8 / N^4) – ((1 – p^2 / (2 * N)))) * ((N^2 / p^2))) I have other less complex equations that will prove p is Prime knowing q and N. The equation is cumbersome, but it will show if 2 numbers are Prime knowing all values. If you are interested in perhaps using any of these equations, I will email you a full set of equations. I don’t mean to distract you from your work, I am just informing you of a pattern you might not otherwise test. My email address is snyder97_bob@hotmail.com Email me if you are interested in working together. And if you don’t want to team up, I will still send you a copy of my work, because the matrices are your work and I know how it is hard to change one’s ideas when you have a lot of effort put into a project. If you take these equations and prove something it only validates my work. And if you win the Fields Metal in math, I want part of the prize. But I encourage you to write and Amazon Kindle book of how to program these matrices once they are perfected. I want to ask you what is your goal in solving Prime numbers? I will share mine after you share yours. But I am just wondering why we work on such an impossible problem. We probably share a like view. Maybe, Maybe not. Also why do you use Excel instead of other more powerful programs? I admit the Excel computation is impressive. But you did mention it runs out of memory then crashes. Anyway, carry on with your idea and don’t let my equations distract you, but if you ever want to test patterns in semi-Primes as they apply to Primality testing, I am willing to share my work.
-
I enjoy the idea of applying physics to Prime numbers. There should be a wave that shows a pattern in Prime numbers. I once posted the idea of having a logarithmic spiral to show a pattern in Prime numbers. I couldn’t get it to work but relating geometry to patterns does things computation can’t. I think the entire problem of finding a pattern in Prime numbers is starting at zero. That is how we count but finding a series is near impossible. Have you ever thought of starting at a starting point other than zero? It may be impossible not to. But I do like your computation and charts. I also like your idea of relating them to physics. I will close with this idea. What if you stop looking at a pattern in Prime numbers and look for patterns in the way they interact with other numbers. For example, I have been trying to solve semi-Primes. If you could prove a number is a semi-Prime, its factors are Prime numbers. So if you take one known Prime number and multiply it by another number if you could prove the resulting number is a semi-Prime, the unknown number is Prime. So what I am saying is that if your charts tested for Primality based on one known Prime and a test value forming a semi-Prime, you would have a pattern. I know this is no easy task. But looking at Prime numbers for awhile now I don’t think a pattern will be formed without somehow placing Prime numbers into a known function, and then find a pattern in that function. Which I think is what you are trying to do with physics, harmonics, and time. I am just suggesting using semi-Primes to see what you can come up with. Also you’ve got to teach me how to create these matrices. And how you are getting those graphics of the patterns. That is just awesome. But I would like you to find patterns where semi-Primes occur in similar charts. You could start with any Prime number. I think it would be less computational. Just an idea. May work, may not.
-

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
Did the previous explanation explain this impossible triangle? I might be able to solve the triangle knowing only N because of the geometric constructions of the angles surrounding N. I don’t know if they still teach using tools like a straight edge and compass. But along with the constructions, I have equations that given p and q in terms of N. I know it isn’t very believable. I’m not claiming this will give a correct solution. But I do think relating my previous posts equations to a geometric figure will help to simplify it. I can see it, but I don’t think others are interested. That is ok. But if I am going to prove my equations useful, I need them to work and be simplified. I like your description of the one-way-function. That’s how I’d define it. I believe that one-way-functions exist for us. My concern is RSA and cryptography. For example, if my triangle worked, we’d have to rethink one-way-functions and RSA cryptography. With the Prime factorization problem, many have tried, so we all believe it is impossible to solve with patterns. I would like to see it solved by similar triangles or vectors. I will get laughed at in the process, but I realize the impossibility of the problem. It may not be humanly possible to solve patterns of semi-Primes, but I thought I came up with a good model. I know my equations are too complex to solve for p, but the equation does show a pattern in semi-Primes. I just need to get others to see the potential of the problem. So if what I am explaining does not make sense or is not explained enough let me know. If something is plain wrong or breaks rules let me know. I am seriously trying as hard as I can to break the Prime factorization problem. No I’m not aware of the ambiguity of the Law of Sines. It has been 20 years since I had a trig class. The rules and Laws are “imbedded” in my mind. By that I mean that I know trig, I just don’t remember how I learnt it. I’m usually good after reviewing a Law or identity. I thought the ambiguity was the tangent of angles above 180; a difference in the direction of the vector. -

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
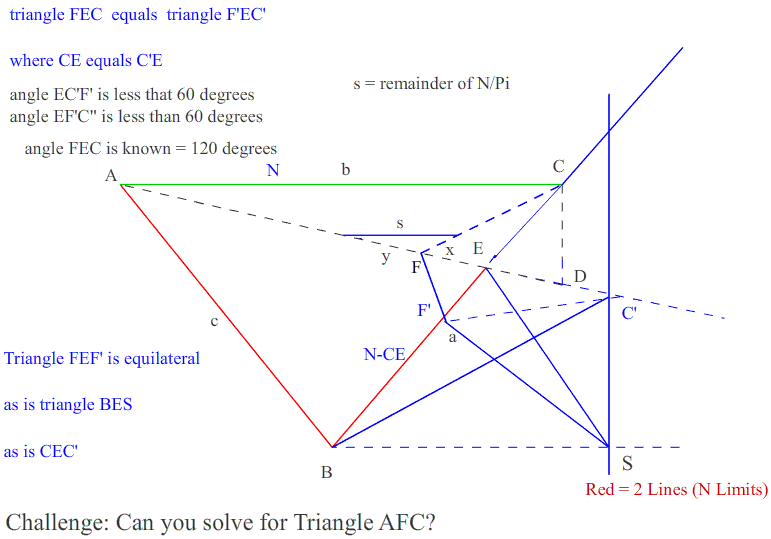
Ok, so I know nobody likes my hypothesis. But don’t let it discourage you, I think there is a relevant problem here in this triangle. The only thing I am trying to do is relate a one-way-function, where N is the product of two Prime numbers p and q. The triangle shows my thought of coming up with equations. I posted earlier about finding p knowing only N. Imatfaal agreed there was a pattern but deemed it useless. I argue that only the polynomial was too complex to solve for p, it still showed a relationship between N and p, giving the distance a test p was from N. It would be good for a computer loop. Like the equations or not, they show patterns. Patterns never before used. So, if I say I have a pattern and that pattern is ugly, what should such a pattern look like? Simply put, the job of this triangle is to simplify my Prime patterns. Does it do that? I don’t know. But I designed it to do so. In my previous post of “Prime Products One More Time”, look on page 3, posted Nov. 6, 2017. CE is equal to p. N mod p = 0. We don’t know CE; only N. We get a new length N – CE. N – CE has an unknown length FE. FE is the remainder when subtracting q from N-CE. As an added challenge, we do not know the value of FE or q. CE is sliding along AC’. It increases from the perpendicular CD to CE until it is displaced along a length FE from E. AF equals q. Now to the part you are not going to like. Using vector edition: AC = N [Absolute value [ AC – AE – (AC-CE) – CE]] = FE I know this is mostly likely wrong, but it helped me imagine 2 vectors of p and q added together at an unknown angle with a side opposite that angle equal to N. Also, I don’t think CE = CF in all cases. I would solve for FC then solve CE. __________________________________________________________________- So why did I give the new hypothesis? I believe that if you take any triangle that has N as its largest side, the sum of those sides are factors fall on an angle that forms N. Yes, I know the term factors is not correct, because these are decimal values. That is until p and q fall along those segments of the triangle. Yes, I know this isn’t vector addition. I am not adding p and q at different angles to get N. I am only using a triangle with longest length N and stating a similar triangle with lengths p and q exist on that similar triangle. So, these are two different approaches; one vector addition; one similar triangles. This is what my triangle is supposed to solve. Does it do it? The odds are against it. After all, it is a one -way-function. But know that I just didn’t throw this thing together with willy-nilly lines. I know in the development the logic in the formation can not be explained why I chose it. I mean, why did I develop a triangle in this way to solve an impossible function? Well, I know the teacher says: “show your work.” But I cannot show my reasoning; only if something works or doesn’t work. -

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
Hypothesis, Given a triangle with known N, the similar triangle formed at any given triangle with the largest side equal to N, will have a similar triangle with sides equal to p and q. This triangle will have the largest side similar to N. This is probably absolutely wrong, but I base it on the fact that the 2 sides multiplied together equal N. I know I am probably breaking a rule of sine and cosine by addition of trigonometry, but my logic is this: If p and q are the products of N then a triangle the contains N as the largest side, the triangle will have a similar triangle that has sides of the products. After all, a similar triangle is just proportions and since p and q are proportional to the original triangle, multiplication does solve the similar triangle. These similar triangles solve the one-way function of N = p *q. The reason it isn’t easy to visualize is because to find the answer because we did not have the equation to find the relationship between p and q. It is ok if I am wrong. This is after all an impossible one-way-function triangle. But if you read through it, you may understand what I was attempting to do. I will respond to each individual post later. I just wanted to clarify my idea, if possible. Yes, I know there are infinitely many triangles. I’m counting on it so that my sides equal to p and q exist. Again, they always exist. I am just using properties of triangles to simplify an equation that is used to find p and q knowing only N. -

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
I know no one believes in my problem. But I need someone to follow along with the solution (or disprove my ideas). It is something a problem solver must work through themselves. I know FC does not equal CE. And if we can’t solve for FE or find FC. I will not break the rules of geometry by using my side-angle solution. But I propose a math exercise to go through. Instead of believing my solution, prove the exercise wrong. (Probably not that hard to do, but I think it is worth trying.) FE = CD / cos[angle ECD + angle FCE] = CE / cos[FCE] This may be solvable with FE the unknown, but we need to solve for CD and CE to for this to work. Angle FCE is also unknown. N/sin[120] = CE/sin[60] To me I drew this triangle to represent the one-way function. I believe it is solvable. This is why I have reposed it. Proving this triangle solvable means that semi-Primes are no longer able to be used in cryptography. It sounds simple enough, but this is no ordinary triangle. I know when I start talking about Prime number solutions or one-way-functions or solving impossible triangles, it draws a red flag. If it were so easy someone would have solved it already. But if you do not believe we can reach a solution, you are probably correct. It may seem like I do not know math because I am always looking for work-arounds. I know the problem is unsolved and I know the probability of finding a solution equals my probability of solving this triangle. But I believe my approach is different. Solved or unsolved semi-Primes, it is still worth the mathematical exercise. -

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
I could make this long, but I will get to the point. I realize most don’t like my Prime equations. I design these triangles as a graphic representation of my problem. Do you agree that triangle yxs is similar to triangle AFC? Do you believe that triangle AFC has no importance other than the fact that it has PNP (also called N) equals the product of 2 unknow Prime numbers? Do you believe that since N is known and that AFC can be solved for and that triangle yxs angles are the same because of the definition of similar triangles? Most importantly, do you believe that y and x are equal to in proportion to N? (This is the most important step, I am still working on.) If y is the larger Prime factor and x is the smallest, we will use similar triangles y/x = AF/FC. All angles and sides of AFC are known. The proportions of y/x can be written with the following equations: N = 85 y = (N^2/x) + x^2) / N x = x N = y * x, but we only know N y/x = AF/FC AF/FC is known as solved by the triangle and is a real number proportion. So (N^2/x) + x^2) / N * (1/x) = proportion AF/FC Calculate it out I am still working on this. I got x = Sqrt(N/ proportion AF/FC) Again I am still verifying everything is mathematically proper. But I post here to get feedback. -

Is there a higher mathematical method to solve similar triangles.
Trurl replied to Trurl's topic in Mathematics
Ok, here is my question simplified: Given: two similar triangles ABC and DEF, Are segment AC divided by segment AB proportionate to segment DF divided by segment DE? If in triangle ABC, all lengths are known, and all angles are known; And in triangle DEF all angles are known; Using the similarity of AC/AB = DF/DE DF and DE are known, but AC and AB are known only by equations, can the equation between AC and AB be simplified by relation to the known DF/DE? That is my question simplified. I did not include example because the problem relies on AC/AB = DF/DE. Other questions I have just realized that the Law of Sines relates to similar triangles. But what I was not taught in school is: d = Sin (a) / A = Sin (b) / B = Sin (c) / C We were not taught that d equals the proportion and is the circumscribed circle of the triangle. But this can be discussed later. I just need to know if what I described above is possible. To me it makes sense and is simple. But I can’t remember ever studying similar triangles this way and I don’t know if a text book would list it under its laws. But I think it isn’t there just like we didn’t learn about “d” because the application is limited. I could be completely wrong. But a side divided by the other in one triangle should equal a side divided by another in a second, similar triangle. -
Ok, you are going to laugh at this one. But I am seriously asking this question. I need to think outside the box if I want to break a one-way-function. In this case outside the triangle. My question is: Why can’t I combine the Law of Sines and the property of similar triangles to solve 2 different similar triangles of different sizes? And secondly why can’t I say the proportions in one triangle are not proportional to the same proportion in a similar triangle? I mean if all sides and angles are known in one and the other similar triangle all the angles are known, there has to be a method to solve the segments of the unknown, second similar triangle. Is there a method I do not know of? For example, you know the lengths and angles of triangle one; and you know the angles of a smaller, similar triangle angle two. I know, if you new one side of angle 2 you could solve with similar triangles no problem. If it were that easy the problem wouldn’t be a one-way-function. I just wanted to get advice on the possibility and if anyone sees this as a worthy problem. To be specific I do not know the lengths of the segments on angle 2, but I do know the equations that make up the lengths. Does anyone believe it would be possible to solve angle 2 with only equations as the known for angle 2? I have put much thought into this. Because being able to break a one-way-function is the result. I know that there are infinite similar angles. And yes, I know trigonometry. This has just been bugging me. Why can’t I do this? Or it must be possible? But are there any higher geometry or higher math’s that solve such a problem? I’m sure someone has encountered this problem before.
-
Ok, I have a thought experiment; a visual model. I used to run daily. I would run down the trail at an 8-minute mile. Somehow a fly that has a maximum speed of 5 mph lands on my neck. I swat the fly and it leaves my neck. Accordingly, I increase my speed to a 6-minute mile. The same fly somehow lands on me again. Every time it leaves my body from my swat in comes right back. The thing to ponder is that the fly moves slower than me yet can intercept me and after intercepting me leaves my body only to intercept me again. What are some physical properties behind this? I would like to get a variety of answers. But to show what I was thinking: suppose drones were set up to intercept a jet aircraft or missile. They don’t have the speed to match the missile or time to acquire exact coordinates. Could a drone intercept a missile as the fly has intercepted me (multiple times)? And would being able to intercept multiple times solve the problem of finding exact coordinates of the missile?
-
I think I have an understanding of what you are doing with factoring. You just want it in that form. I would like to see an updated proof, since this thread has so many explanations. This is the first time I saw this conjecture, so I hope some experts on this forum take interest. The problem is worth working through. Right or wrong it is a fresh approach. Just because the conjecture is unsolved does not mean the idea isn’t valid.
-
I think the approach is brilliant and maybe simple enough to work. But I am still not convinced. You take 2 to a power and multiply by Prime factors. I agree that you can build any number this way, but I don’t believe multiplying by 2 here is the same as dividing an even number by 2 in the conjecture. The order of operations. And I cannot test this algorithm in a computer since we cannot factor the numbers in this series. Again I’m probably wrong but this is not a linear series. My understanding is that you are building a pattern linearly and the conjecture is not a one-to-one function. This is just my understanding but if you can prove your method does this I will buy multiple copies when you get it published.
-
Ok, I’m listening. Where does this go from here? This is how I understand you: Add 1 to a Prime number and it can be written as a power of 2. Since all Composite numbers factors are Prime numbers, those Prime factors added to 1 reduce to a power of 2. This will reduce to 1 and prove the conjecture. Your job is to prove this is possible. My question is how do you factor the Primes and apply the conjecture without changing the value of original number. By this I mean you factor the number into two Prime numbers and add 2 (+1 each Prime) to coverage to 1 and prove the conjecture. But at the same time you did not apply 3x +1 to the original value breaking the series. I am probably wrong again. I just don’t follow the modifications to the conjecture series. I’m am just making clear if I am following what you are doing. But if you explain this, I’m onboard. I am still looking at the original proof to make sure my question made sense. I am not completely sure of all the functions affecting x. But if the above question is confusing I will simply ask this: Are you breaking the rules of the conjecture by apply functions?
-
Ok here is what I don’t yet understand. Try a series for 85. In your example you would multiply by 3 and add 1. This would find Prime factors for the new number and not 85. I believe that would make it hard to factor Primes or semi-Primes. But then again I don’t have a full understanding of the problem. You are the expert on this problem. This is your problem. I am just making you defend and explain it more clearly. Also I took interest in you explaining your idea. I don’t know if you are right or wrong. A mathematician must decide for himself if a problem is worth pursing. But if it doesn’t work on first explanation don’t give up. If x is even divide by 2 If x is odd multiple 3 add 1 gives you even so divide by 2 still even divide by 2 till equals 1 The conjecture shows a relationship in factors but does not show those factors. Let me know what you think. I am problem wrong in the understanding of this problem. But that is ok. It just leads to more discussion.
-
I am also an amateur mathematician. I agree your last example equals. But with all these posts the thread is out of control. I have read 3pages but all these changes confuse the reader. I know it is for your benefit to improve your proof, but I can’t understand it. I know it is bad practice to work with only limited examples, but could you show from the beginning an example? Here is an excerpt from the book Primes and Programing “The fundamental theorem 1.1 has a simple look about it, and indeed it is nice to know that every number greater than 1 is prime..or factors into primes. But actually finding the factorization…(or primality) can be very hard.” I request a through example because I don’t know what is the given and what we are solving. I think an example will show what you are describing and what variables we know. Can you write in 2 sentences what is the goal of the Proof. (A short abstract) Again I am also an amateur mathematician but for your proof to work everyone must understand what you are attempting to show. I like to work with examples when trying to understand series.
-
Are you saying that to add 1 to a number divisible by 2 will make it odd? And if you can determine if this odd number is a semi-Prime the Prime factors must be less than 2 to the power of the semi-Prime? My question is what way did you use the conjecture to solve the problem of factoring? This will increase in difficulty with larger numbers. Lemme 2, lines 48 & 49 lost me.
-
As you know I have a complex equation describing semi-Primes. The fact is I cannot at this time solve the polynomial. But instead, it is more of a guessing attack to discover Prime factors knowing only N. What I have discovered is more of a series. It only works with Prime factors. More importantly, the series works with the same Prime factor multiplied by other Prime factors. So: · The series is a guess attack, comparing N to computed N. · The series only works for semi-Prime factors that are Prime numbers. · The series works for a given Prime number and infinite other, different, Prime number factors, multiplied for different results of different N’s. (If the equation did not work for multiple factors, multiplied by the same factor, the equation would be useless.) · The equation that produces the series can be used to test if a number is truly Prime. Other significant facts of the equations: · The equation is too complex and factors with imaginary numbers. · The equation is simple algebra. · Even though the equation cannot be solved with a perfect mathematical answer, the series is beneficial to computer algorithms. · The equations may have relationships to logarithmic spirals. I have been working with Prime numbers and Logarithmic spirals since 2006. But have recently tried to switch my efforts to other projects. I haven’t posted to SFN for 5 months, in this thread. But instead of trying to defeat RSA and use my math series to factor p and q knowing only N; there is a twist. If you know p (also what I usually refer to as x), you can test to see if it is a Prime number by multiplying by Prime number test values. If the equations hold true, then a test for Primality exists. https://www.3dbuzz.com/forum/threads/200441-New-One-Way-Function I don’t know if anyone believes me when I say this series is significant. But I posted the link to me “old” work from the time before I found the more useful equations. Much of that work is just plain wrong. But it will show you how I ended up with the equations I promote now. So, if you think there is any meaning to my equations, please post me a message in this thread to let me know. I will respond with more information.
-

How to get ,The Area of Trapezium from Triangle.
Trurl replied to mathspassion's topic in Mathematics
Congrats on the minor discovery. Any discovery however little, is significant. But the next thing I would do is see where the discovery applies. It may seem like a simple polygon, but it is also a geometric construction. (What I am calling is using drawing and geometry to form shapes with measurable properties. Like dividing a line in half with 2 arcs of a compass.) I know it seems like the use for drawing this way is minor. However, what if this drawing method was applied to computer graphics. Imagine drawing a polygon mesh in 3DS Max and have that mesh be measurable by your polygons. It is difficult to come up with a major application, because this isn’t my design, but if you expand it to polygons of more sides with the inside of those polygons measured; you’d have a brick to build a polygon block and measure it at the same time. Now that isn’t a little discovery. -
I hope this is the right place to post this, but where is imatfaal? I always enjoyed her comments. I wanted to get her opinion. I don't know her. She could be anyone, as this is an Internet forum. But I was wondering why she hasn't returned.