caracal

Hi, thanks for the link i'll read it The equation $$ E = hf $$ would Still be valid for photon that has velocity c. I am not saying that it would be any different for photon. And the de Broglie frequency if it is $$ f = \frac{m\gamma v^2}{h} $$ it would become also close to $$ f \rightarrow \frac{m\gamma c^2}{h} = \frac{E_{tot}}{h}$$ when the velocity of particle approaches light velocity. Photoelectric effect only confirms that light is consisting of photons (or either absorbed as energy packets in metal) whose energy is E = hf. The maximum kinetic energy of electrons leaving the metal is $$E_{kin_max} = hf - W $$ Where W is a work needed for electrons to get out from the metal. I looked this wikipedia article, i need to read it better. But looks like Crystal scattering experiment model also needs only equation for de Broglie wavelength for electron, not frequency and equation for constructive/destructive interference. It is similar experiment than double slit experiment. If the electrons phase velocity and Group velocity are both v, then the equation for de Broglie frequency would be $$ f \lambda = v $$ $$ \rightarrow f = v/\lambda = \frac{m \gamma v^2}{h} = \frac{m \gamma c^2}{h}(\gamma - 1/\gamma) $$ (This is exactly a term what bakhoum was thinking. i also was thinking could de Broglie frequency be beating, that is a difference of two frequency components. ) But as commonly what is accepted for de Broglie frequency being $$ f = \frac{m \gamma c^2}{h} = E_{tot}/h $$ Then this equation between wavelength, frequency and velocity does not hold. The phase velocity is theoretically thought to be c^2/v while group velocity is v. But is this only a theoretical argument?

Hi, Can you give an example of such experiment where de Broglie frequency is measured in practice? Is it confirmed experimentally in some experiment or calculation? De Broglie wavelength is confirmed for sure, i know that. Double slit experiment for example only needs formula of de Broglie wavelength in order to calculate the interference pattern behind the slit. It does not need any information on de Broglie frequency. Double slit experiment model only needs this formula $$ \lambda = \frac{h}{p} $$ And then you just need the equation for constructive or destructive interference.

Hi all! (I wrote this article in my own language first, then i used google translator to translate it into English. Then i read whole text and checked and corrected some words) This thinking is really based on that i try to make phase velocity to be v with assuming new de Broglie frequency and then i check whether de Broglie wave can be wobble/beating of two components since $ m\gamma v^2/h = mc^2(\gamma - 1/\gamma)/h $. And then i try to calculate phase and group velocities of such beating wave. So, to begin with, I could say why I think something like this? I have just enough knowledge of the subject to be able to do some kind of mathematical analysis. This subject belongs to signal analysis and in deeper analysis there are some concepts of quantum mechanics that are unknown to me, such as wave packets and the Dirac equation. Therefore, my review is not very far-reaching. The reason for my reflection is: There may be an error in the first theory of quantum mechanics, de Broglie theory. Due to the error, the phase velocity of the de Broglie matter wave becomes $ v_{phase} = c^2/v $ which is above the speed of light. The same error may also produce a velocity c for the particle in Dirac's theory, which is the first relativistic theory of quantum mechanics. (Usually this has been tried to be explained by the Zitterbewegung phenomenon.) Ezzat Bakhoum has already noticed in his 2002 article ("Fundamental Disagreement of Wave Mechanics with Relativity", Physics essays 2002) that if the de Broglie frequency is set to $ f = \frac{m \gamma v^2}{h} $ , the phase velocity of the matter wave becomes v instead of c^2/v . The de Broglie wavelength, on the other hand, is still considered to be $ \lambda = \frac{h}{p} $ . Bakhoum also makes other observations that I am not very familiar with. Unfortunately, in this article Bakhoum draws the wrong conclusion on the matter. He claims that the mass-energy equivalence would be of the form E = mv^2 instead of E = mc^2 (in these two formulas m is now the so-called "relativistic mass"). This is a wrong conclusion. I made another thread about "is the total energy of a particle really E=mc^2 but I finally concluded that this is indeed the case. In the following, I will use the notation m for the rest mass of a particle, so the total energy of the particle is written as $$ E_{tot} = m \gamma c^2 $$ , where $ \gamma $ is the Lorentz gamma function: $$ \gamma = \frac{1}{\sqrt{1-(v/c)^2}} = \sqrt{1+(\frac{p}{mc})^2} $$ Well, why wasn't this de Broglie frequency error noticed ages ago? -------- I don't know. I haven't found any information on what the de Broglie frequency is used for in practice. Instead, the de Broglie wavelength is used successfully in many quantum mechanics calculations and has been experimentally confirmed and very accurately. Maybe this correction doesn't work for some reason in the end of the day. It is also known that the total energy of a particle is $E_{tot} = m\gamma c^2 $ very accurately. But is it known that there is a connection between the total energy and the de Broglie frequency $ E_{tot} = hf $? If the de Broglie frequency is not needed in any significant calculation, could it still be different from $f =\frac{m\gamma c^2}{h}$ ? That's a question I can't answer myself. That's why I'm doing these calculations. First ------- There is the following interesting mathematical identity: $$ m\gamma v^2/h = mc^2(\gamma - 1/\gamma)/h $$ This is a surprising coincidence. This formula resembles the formula for the oscillation frequency. It gave me the idea to think about whether a matter wave could be an oscillation instead of a wave motion with a single frequency. When the speed of the particle is small, the de Broglie length of the matter wave $ \lambda = \frac{h}{p} $ can be very large. When the speed approaches the speed of light, the length of the matter wave can again approach zero. It might make sense to explain this by saying that the matter wave is an oscillation between two components. De Broglie is said to have pondered the problem with the de Broglie frequency, is it affected by time dilation and slows down or does it behave like total energy? He chose the latter option. But perhaps it does both, and splits into two components? The mathematical analysis of a "wobble/beating quantum" or "wobble/beating wave packet" is too difficult for me, so in the following I will only consider the wobble between two frequency components. How did Bakhoum see the de Broglie frequency? ----- Bakhoum probably thought that the new de-Broglie frequency $ f = m\gamma v^2 $ was created by just one matter wave and that the de Broglie wavelength was $ \lambda = \frac{h}{m\gamma v} $. Then the phase velocity of the wave becomes $v_{phase} = \lambda f = v $. The group velocity for a single wave is simply $ v_{group} = v $. (er...Or is it?) Analysis of Two-Wave Oscillation ------- Let's start by setting $$ f_2 = mc^2 \gamma $$ $$ f_1 = mc^2 1/\gamma $$ where $ \gamma $ is the Lorentz gamma function $$ \gamma = \frac{1}{\sqrt{1-(v/c)^2}} = \sqrt{1+(\frac{p}{mc})^2} $$ and consider the following function: $$ F(t) = cos(2\pi f_1 t) + cos(2\pi f_2 t) $$ Using trigonometry, this function can be written as $$ F(t) = cos[(2\pi)\frac{f_2+f_1}{2}t]cos[(2\pi)\frac{f_2-f_1}{2}t] $$ And let's write this in the form $$ F(t) = cos[(2\pi)f_{carrier}t]cos[(2\pi) f_{envelope}t] $$ Now the question is, if the function describes a quantum mechanical "wobble" - a matter wave and its quantum, then what is the energy associated with this quantum? "In a technical sense" it could be possible that there is an energy term $ E_{QM} = m \gamma v^2 $. Which would describe "quantum mechanical energy". But is this real energy? Another option that comes to mind is that the energy of a wobble quantum is calculated in some other way than the energy of a light quantum of the corresponding frequency. So are there two different energies? Total energy and "quantum mechanical" energy that participates in the matter wave phenomenon? I leave this question open. It is quite easy to see, just by looking at the arguments of the above formulas, that the total energy of a particle can be expressed in terms of envelope and carrier frequencies as follows: $$ E_{tot} = (f_{carrier}+f_{envelope})h $$ not $$ E_{tot} = hf $$ The following relation would exist between the oscillation frequency and the total energy: $$ E_{tot} = m\gamma c^2 = \frac{1}{2}(hf + \sqrt{(hf)^2 + 4mc^2} $$ $$ (hf)^2 = 2( \sqrt{m^2c^4 + E_{tot}^2} - mc^2) $$ These relations are valid if the de Broglie frequency is $$ f = \frac{mc^2}{h}(\gamma - 1/\gamma) $$ instead of $$ f = \frac{mc^2}{h}\gamma = \frac{E_{tot}}{h}$$ The quantum energy of a free particle is probably some kind of integral of some kind of wave packet. If the wave packet is indeed an oscillation wave, common sense tells us that its total energy cannot be E = hf , where f is the oscillation frequency, but more than this. An oscillation wave has more movement. The "total energy of a particle with an beating quantum" corresponds to the sum of the energies of two photons with frequencies $f_{carrier} = \frac{mc^2}{2h}(\gamma + 1/\gamma) $ and $f_{envelope} = \frac{mc^2}{2h}(\gamma - 1/\gamma)$. What happens at low speeds? --- Let's now examine these frequencies $ f_{carrier} $ and $f_{envelope} $ , when the particle's speed is low. We can make a Maclaurian series expansion of each of them, and extract the first two terms from them $$ f_{carrier} \rightarrow \frac{mc^2}{h} + 0 $$ $$ f_{envelope} \rightarrow 0 + \frac{mv^2}{2h} $$ The formulas approach the formulas for rest energy and kinetic energy divided by h. In this context, one can think that the carrier frequency can almost be omitted because it is very high. In this case, the envelope frequency is left. I am not sure if this corresponds to the frequency of one of the solutions of the Schrödinger equation. The above formula seems to produce the same energy as a single light quantum, or photon, whose frequency happens to be $$ f = \frac{m\gammac^2}{h} $$ if the particle's speed approaches the speed of light, f1 approaches zero, so the wobble disappears and only the component f2 remains. In this case, the formula E = hf is still valid. Some thoughts ----- So it seems that the formula E = hf does not hold when the matter wave is wobbling/beating. The de Broglie frequency instead of being $f_{broglie} = \frac{E_{tot}}{h}$ would be $$ f_{broglie} = f_2 - f_1 = mc^2(\gamma - 1\gamma) $$ Note that the wobbling frequency $f = f_2-f_1 $ is actually twice as large as the Envelope frequency. The wobbling frequency f is what is observed, for example, in music when two notes are wobbling close together. Now at this point I will present the identity from the beginning again: $$ mc^2(\gamma - 1/gamma) = m\gamma v^2 $$ which holds for all speeds 0 < v < c. The de Broglie frequency can therefore also be written in the form $$ f_{broglie} = mc^2(\gamma - 1/\gamma)/h = m\gamma v^2 /h = pv/h $$ So de Broglie may have postulated the wrong de Broglie frequency in his theory. The de Broglie wavelength postulate is correct instead and the wavelength remains the same, which is known to be $$ \lambda = \frac{h}{p} = \frac{h}{m\gamma v} $$ It seems that the de Broglie wavelength postulate is independent of the de Broglie frequency postulate. It does not tell whether there is wobble in the matter wave. Phase and Group Velocities of the beating Wave ----- Now we could calculate the phase and group velocities of the new de Broglie wave. The oscillation frequency formula can be written in the following form: $$ F(x,t) = 2cos[kx-wt]cos[\Delta k x - \Delta wt] $$ and the following formulas apply to the phase and group velocities of these two waves: $$ v_{phase} = \frac{w}{k} $$ $$ v_{group} = \frac{\Delta w}{\Delta k} $$ The angular frequency and change in angular frequency are then: $$ \omega = \pi (\gamma + 1/\gamma)(\frac{mc^2}{h}) $$ $$ \Delta \omega = \pi (\gamma - 1/\gamma)(\frac{mc^2}{h}) $$ In order to obtain the phase velocity of the oscillation wave as v, $\Delta k$ must be $$ \Delta k = \frac{1}{2}\frac{2\pi m}{h}\gamma v $$ ,then the wavelength is $$ \Delta \lambda = 2\pi/\Delta k =2 (\frac{h}{m \gamma v}) $$ At this point, it must be remembered that the observed oscillation frequency is twice the actual frequency, with the same logic the observed oscillation wavelength is half of this. (or is it?) The wavenumber of the carrier wave depends on the phase velocity. The following relation exists between the phase velocity and the wavenumber of the carrier wave: $$ v_{phase} = \frac{w}{k} = \frac{1}{k}(\frac{2\pi mc^2}{h})(\gamma + 1/\gamma) $$ is the carrier wave velocity, or phase velocity, v , or is it c or some velocity in between? But it remains open, how do the actual frequencies, wavenumber and the above-mentioned velocities behave in a wave packet? Application to the Schrödinger equation - some thoughts ------ How does such a beating wave of matter fit into the Schrödinger equation? The equation in question is a postulate, or initial assumption, of non-relativistic quantum mechanics. Thinking in this way with common sense, the complex wave function that comes as a solution to the equation probably remains the same otherwise, but it adds a very high-frequency carrier component, whose frequency is $$ f = \frac{mc^2}{h} $$ . The carrier frequency is precisely this expression when the speeds are very small compared to the speed of light. What the wave number of the carrier wave would be remains open in this reflection. Such a "second-order" carrier wave would perhaps produce its own interference pattern in a double-slit experiment, but this is very difficult to distinguish in practice, because the carrier frequency is very high. This frequency is of the same order as the frequency of the Zitterbewegung phenomenon. This phenomenon was needed to explain why the speed of the Dirac particle is the speed of light. According to the phenomenon, the particle moves from its position at a very fast frequency so that its average speed becomes v. The end.

It may not be just anything, but i would need more information. I don't know which total energy would be better. Bakhoum just treats the definite integral differently to get his total energy. Here is reference: https://arxiv.org/pdf/physics/0206061.pdf (page 6). But this is just mathematical trick. This does not prove that his suggestion is the right total energy. Work integral [math] E_{k} = \int_{0}^{s} F dx = \int_{0}^{p}v dp [/math] , is mathematically speaking always a difference between two things : [math] E_{k} = E(s) - E(0) [/math]. You can add same function to E(s) and E(0) and still get same [math] E_{k} [/math]. In other words, you don't know how much energy particle has at rest and how does the total energy vary between 0 and s. Bakhoum suggested that [math] E(s) = m \gamma v^2 = m c^2 (\gamma - 1/\gamma) [/math] and [math] E(0) = m c^2(1-1/\gamma) [/math]. I think Nuclear reactions support [math] E_{tot} = m \gamma c^2 [/math], but Bakhoum states that [math] E_{tot} = m \gamma v^2 [/math] reconcile some equations in relativistic quantum mechanics better. Bakhoum studied Dirac equation and Hydrogen atom, how different total energy change them. But Bakhoum also claims that nuclear fission would have different energy spectrum, and beta decay happens differently. I tend to think that he might be wrong there. I think the energy spectrum and beta decay could be explained well without this new total energy. I suggest two different kind of things to explain energies in nuclear reactions: 1. there are actually two different kind of total energies - energy that participates to relativistic QM and energy that participates to mass to energy conversions or 2. All nuclear reactions and decays just happen to involve high velocities + powerful interaction of the mass that is converting (to particles, kinetic energy or photons) , when [math] m \gamma v^2 [/math] will aprroach [math] m \gamma c^2 [/math]. For example in beta decay that seem to happen in rest - I listed these possibilities that could make option 2. possible - Heisenberg uncertainty principle, quark kinetic energies, potential energies and quark field interactions could make the reaction involve something that is accelerating from low velocities to high velocities - therefore mass to energy conversion look like to be form [math] \Delta E = \Delta mc^2 + \Delta E_{kin} + \Delta E_{binding} + etc [/math]. Actually equation [math] \Delta E = \delta mc^2 [/math] in nuclear reactions could be still right. That would mean that Mass is still the measure of the energy content of matter. But particles just have this different total energy [math] E = (\gamma - 1/\gamma ) mc^2 [/math] That would mean that the energy budget of protons mass or nucleus mass could be slightly different. There is that 'could' because my thinking is not working now. I don't know where de Broglie frequency is needed, if is it needed anywhere. If it is not needed, then different de Broglie frequency does not change any practical calculations. Looks like total energy of particle is used in relativistic quantum mechanics such as quantum field theory. I didn't manage to find information where it is needed exactly. But i understood that it is an assumption that is inserted to the theory. (It is assumed that total energy would be [math] m \gamma c^2 [/math].)

Reconciling this new total energy to quantum mechanical theories is what Bakhoum has done in his writings. I don't go to check them now. But what it seemed to be is that It just clears out some things in the theories of quantum mechanics. The simplest is that there is not anymore super luminal phase velocity. ... Yes i can see what kind of experiment that is. A sample of radioactive material contain an atom nuclei that undergo beta decays and that happens in rest. Energy,momentum,electric charge and spin are conserved. Proton has less mass than neutron and therefore it is energetically possible for neutron to decay to proton, electron and antineutrino. Down quark emits W- boson and changes to up quark. W- boson decays after some time into electron and antineutrino. In fact it does not matter whether the parent is in rest or in constant velocity - the situation would be same, only the sample would be rest in other inertial reference frame. That is because of Relativity principle. What i mean by this suggestion is high relative velocity between interacting particles + powerful interaction. But what happens at the moment when down quark changes to up quark and emit W- boson? That is a moment that takes about [math]N * 10^{-25}s [/math] or less since the lifetime of W boson is already [math] T = 3 * 10^{25}s [/math] And also what happens at the moment when W- boson decays into electron and antineutrino? I don't know. Particles just seem to appear or change to some other particles. There are three reasons why there might be high velocities present: - Quarks already have high kinetic energies - Potential energies - Heisenberg uncertainty principle Two last ones can temporarily give more kinetic energy to particles. This kinetic energy does not show up in the outcome of the reaction.

Looks like he appears in arxiv. I think he is not a bad writer. But he may not have 'proofreaders' or 'moderators' so there may be mistakes. The idea is very solid - just one change and one equation. [math] E_{tot} = m \gamma v^2 = mc^2(\gamma - 1/\gamma) [/math] and that's it. I repeat here a little.: The derivation of equation for kinetic energy [math] E_{kin} = mc^2(\gamma - 1) [/math] is done by looking how much work has to be done for particle in order it to gain velocity v. But after that it is interpreted this equation such that [math] E_{kin} = E_{tot} - E_{0} = m \gamma c^2 - mc^2 [/math] But actually what you have is [math] E_{kin} = (m \gamma c^2 + X ) - (mc^2 + X ) [/math] where this X can be anything - any function of v + any constant. Looks like there is room for alternative interpretation. That would be that [math] E_{tot} = m \gamma v^2 [/math] . This new energy term [math] E_{new} = mc^2(1-1/\gamma) [/math] appears in the equations of quantum mechanics. For example de Broglie frequency [math] f =\frac{m \gamma v^2}{h} [/math] which is now different. Most of Bakhoums writings deals with how quantum mechanics and i think QED changes when there is different total energy. But it seems that nothing practical comes out of it - equations just changes and he claims that they become better. I am not sure how it can be converted to other form of energies, but my own suggestion is that it can in particle decays and nuclear reactions but only when velocity of interacting particles approaches c. Lets go directly to the reactions of beta decay or annihilation. From vertexes like this, without knowing the details of the reaction, i can't say whether particles have high velocities at the moment of reaction itself or not - in the places where the vertices meet or separate. It is probably quantum tunneling what makes these reactions possible. But what happen during the quantum tunneling and during the reaction itself? Can particles have high velocities during quantum tunneling? (pictures) The mean Z and W bosons lifetime is [math] 3 * 10^{-25}s [/math] so i guess beta decay event when W-boson is ejected from proton is even less than this. The reaction itself where particles separate may therefore be very rapid and takes time about that much : [math] 10^{-25}s [/math] ? In order to estimate the event time of reaction: Radius of proton is [math] r = 0.87*10^{-15}m [/math]. If some particle is moving at light speed, it goes across proton in time T : [math] T = 2R/c = \frac{1.74*10^{-15}m}{2.998*10^{8} m/s} = 5.804*10^{-24} s [/math] In such very short time scales the Heisenberg uncertainty principle may also play bigger role: Particles may have for short period of time high kinetic energies such that [math] \Delta E \Delta T = \frac{h}{2 \pi} [/math] If [math] \Delta T = 10^{-25}s [/math] , then [math] \Delta E = \frac{6.582 * 10^{-16}eVs}{10^{-25}s} = 6.582 * 10^{9} eV = 6582 MeV [/math] Maybe particles could have high velocities during very short moment of tunneling and after that lose the velocity, possibly both due to Heisenberg uncertainty principle? In that case they could have also high velocities at the moment of reaction.

I answer here to all of the things you pointed out. There is actually one possibility more: Maybe there are two different kind of energies: Total energy [math] E_{tot} = m \gamma c^2 [/math] that is converted to other forms of energy in nuclear reactions and radioactive decays and "energy that is participating quantum mechanical behavior" [math] E_{qm} = m \gamma v^2 [/math] . It would be this later one that should be inserted to quantum mechanical theories instead of the total energy. Yes, rest energy was derived by Einstein but it is coming from interpretation of the formula of kinetic energy. You can add same term to both of the energies "rest energy" and "total energy" and have still same kinetic energy. citation: https://arxiv.org/abs/physics/0206061 "Fundamental disagreement of wave mechanics with relativity" page 4 about. This is the first of his papers. There he argues that some equations become nicer if you add different total energy. But by thinking that possible rule that "nuclear reactions happen only when some of the particles have high velocities", There are three reasons that comes to mind that could accelerate particle 1. Interactions: EM interaction, strong interaction and weak interaction 2. Quantum tunneling: It may be possible that particles have high velocities during quantum tunneling. 3. Heisenberg's uncertainty principle, the energy time form of it. [math] \Delta E \Delta t = h/2\pi [/math] It allows particle to have temporarily high amount of kinetic energy but that energy is taken back also very soon. Just before electron-positron annihilation the both particles are being accelerated towards each other. I don't know is this enough. E.Bakhoum also writes about this in the article "Dialogue on the principle of mass-energy equivalence"

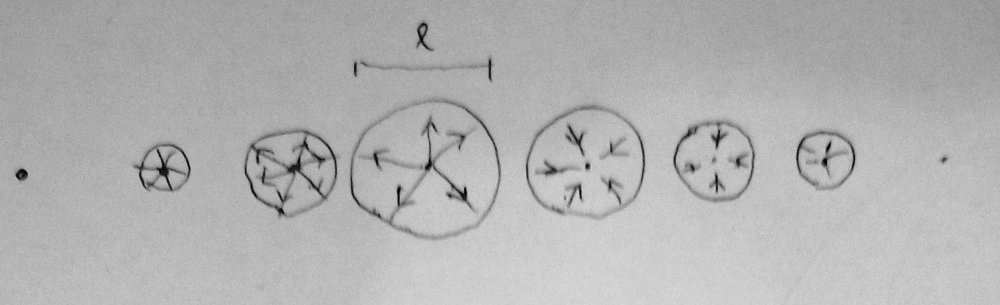
Hi all. Life is short, and i have another interesting idea regarding special relativity. I want to write this thread independently from the other thread i have written earlier that is about pulsating dots. Don't bring that up here in this thread. There may not be any connection between these ideas. the reason is that whatever kind of dots there may be, they may be extremely small. I have found interesting articles that are written by E.Bakhoum starting from the year 2001 where he suggest different total energy for the particle. I want to bring it up here as i think it is an interesting idea. I don't know if it its true. I don't agree with everything and i have also own thoughts about the issue, so this thread is not just repeating what he has written. But i think it is better to introduce his texts here as a reference. https://arxiv.org/search/physics?searchtype=author&query=Bakhoum%2C+E+G Unfortunately, it looks like that most of the predictions from this new total energy are theoretical and does not have practical meaning. --- The usual comprehension of the subject of energies in special relativity is that the total energy, kinetic energy and momentum are (i don't know if latex works here) [math] E_{tot}= m \gamma c^2 [/math] [math] E_{kin} = m c^2 (\gamma-1) [/math] [math] p = m \gamma v [/math] [math] \gamma = \frac{1}{\sqrt{1-(v/c)^2}} [/math] ,where [math] \gamma [/math] is Lorentz factor and m is mass of the particle in rest. In this notation m is always 'mass in the rest' m=m0 . How this kinetic energy is derived? It is derived from the situation where particle starts to accelerate from rest into velocity v and determining how much work is required to do this. I don't go there. Where does this total energy is derived from? It is actually interpretation from the kinetic energy formula - which is coming from definite integral of work put to a particle that accelerates from the rest to velocity v. It looks like there is some kind of 'rest energy' when particle is at rest. But this definite integral is a difference of two things. You can add same thing to both of the components and still have same difference. It turns out that there is one thing that could be added to both of the components. First note that there is following important mathematical identity: [math] \gamma v^{2} = c^{2}( \gamma - 1/\gamma) [/math] E. Bakhoum suggest that there is such component in both of the terms that is [math] -mc^2(1/\gamma) [/math] . He suggest that the total energy would be different but kinetic energy and momentum would be same. [math] E_{tot} = m \gamma v^2 = m c^2 (\gamma - 1/\gamma )= pv [/math] [math] E_{kin} = m c^2(\gamma -1) [/math] [math] p = m \gamma v [/math] what this new total energy would mean? Particle does not have rest energy. Mass is converted to energy with ratio E=mc^2 only when particles collide in near light velocity. There is no 'relativistic mass' that is energy divided by c^2 , since the another energy component in the total energy besides kinetic energy - is not same but depends on the velocity: [math] E_{2} = mc^2(1-1/\gamma) [/math] instead of [math] E_{2} = mc^2 [/math] Now i ask question that comes clearly to my mind: How about radioactive decays? They are proven to follow formula [math] \Delta E = \Delta m c^2 [/math] ? I think the answer is that particles that participate on radioactive decay are accelerated before their interaction to near light velocity and decay does not happen otherwise. These accelerations may be 'hidden' under the phenomenon of quantum tunneling that is the most prominent quantum mechanical phenomenon in nuclear reactions and decays. Therefore i don't completely agree with E. Bakhoum. I think E. Bakhoum makes mistake when he assumes that particle decays and nuclear decays and reactions can also happen when interacting particles have non-relativistic velocities at the moment of the reaction. He thinks that that there are different kind of energy distributions. I think any reaction or decay can happen only when interacting particles approach the light velocity. If nuclear reactions and radioactive decays happen only when the participating particles are accelerated to high velocity, both total energies give same predictions for nuclear reactions and decays. With this rule added - i think the idea might work. You may not be aware that the total energy is inserted to theory of quantum mechanics from outside of the theory. If you insert this new total energy there, many things changes. For example: 1. phase velocity = group velocity = v 2. de Broglie frequency [math] f = \frac{E_{tot}}{h} = \frac{m \gamma v^{2}}{h} [/math] 3. Energy-momentum relation [math] E^{2} = (\frac{pv^{2}}{c})^{2} + (mv^{2})^{2} [/math] instead of [math] E^{2} = (pc)^{2} + (mc^{2})^{2} [/math] (which may be now unpractical equation) 4. Classical radius of electron [math] r_{e} = \frac{1}{4 \pi \varepsilon_0} \frac{e^{2}}{m_e \gamma v^{2}} [/math] The radius depend on velocity and is infinite at rest. So what does it mean, how is this interpreted then? Is this anymore useful property? I am not sure and i don't go into this here. All of these changes are coming from that you insert E_tot = muv^2 instead of E_tot = muc^2 during the derivation. Also some of the four-vectors changes, such as four-momentum: 5. four-momentum [math] P = (m \gamma v_{x} , m \gamma v_{y} , m \gamma v_{z}, i (\frac{v}{c})m \gamma v) [/math] (i am not sure about this) Also Lagrangian is now different. But i don't go into these. But i believe that everything is consistent. However most of these changes seem to be theoretical aspects without practical outcome. The rest here is my own thinking: ----------- An interesting physical quantity of mc^2/h ................................................................ What i think there might be new insight that the matter wavelength depends directly from the new total energy of the particle: [math] \lambda = \frac{h}{m \gamma v} = \frac{hv}{m \gamma v^2} = \frac{hv}{E_{tot}} [/math] [math] f = \frac{v}{\lambda} = \frac{m \gamma v^2}{h} = \frac{E_tot}{h} [/math] [math] f = \frac{m \gamma v^2}{h} = \frac{mc^2}{h}(\gamma - 1/\gamma) [/math] (note that phase velocity and group velocity are both v with this new total energy) Where is this frequency coming from? Could it be beating between [math] m \gamma c^2 / h [/math] and [math] mc^2(1/\gamma) [/math] ? That would mean that when particle is in rest, there is some kind of frequency that is related to its mass: [math] f_{rest} = \frac{mc^2}{h} [/math] This would be a kind of "rest frequency" of the particle - whatever it is. For some reason it divides to two components when particle start to move. It could be also a maximum of some frequency distribution that changes to distribution with two maximums when particle moves. This is just a schematic picture of how the situation would look like if you look frequency distributions. The spikes can be different kind of: There are two relativistic effects known to happen when particle moves: length contraction and time dilation. Maybe these components are related to these. This constant c^2/h has very high value: [math] \frac{c^2}{h} = 1.356 \cdot 10^{50} [\frac{Hz}{kg}] = 1.366 \cdot 10^{32} [\frac{Hz}{eV}] [/math] This frequency is very high even for lightweight particles such as neutrino which might have maximum mass of 0.120 eV/c^2. Therefore it is very difficult, if not impossible to observe. However the component mc2h(1/γ) could be observable since it goes down to zero when particle approaches light velocity. if [math] m = 0.120 eV/c^{2} [/math] => [math] f_{rest} = 0.120 eV * 1.366 * 10^{32} [Hz/eV] = 1.639 * 10^{31} Hz [/math] An effect of time dilation for solar neutrino on the frequency f_rest: [math] f'/f_{rest} = \sqrt(1-0.99999999995^2) = 10^{-5} => f' = 1.639 * 10^{26} Hz [/math] Wavelength of f': [math] \lambda' = c/f = \frac{2.998*10^{8} m/s}{1.639 * 10^{26} hz} = 1.829 * 10^{-18} m [/math] That is still small - about 1000 times smaller than the radius of proton. What does this frequency then cause? a second kind of interference pattern? I don't know. So if this thinking is right then there is some kind of frequency that is related to particle's mass and that divides to two components, and beating between these two components is somehow responsible for matter wave phenomenom. ----- this is all. I would say that there might be something interesting in this idea of different total energy. Whether it is really the total energy of a particle, i don't know.

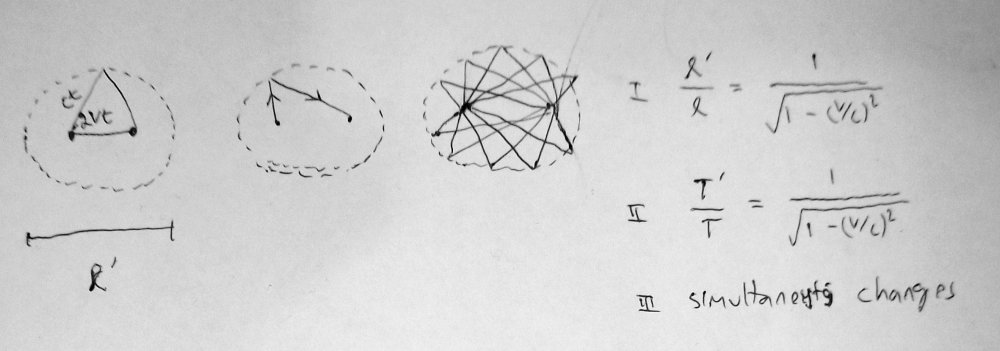
Hi all. I came up with this idea that could explain the theory of special relativity and more over the Lorentz covariance and Lorenz transformation. This reminds of one commonly known special relativity "problem" or thought experiment that is about mirrors. A. Lets assume that some physical entity does not consist of rigid constituents, but dynamic sub entities what i call "pulsating dots" or in other words, expanding and contracting spheres. B. Lets assume also that they pulsate between single point and some sphere such a way that the velocity of the frontier is always light velocity c. It may be possible that assumption A and B can be more general and that i don't need to assume that the pulsating dots are spherical but more like areas that change their shape with frontier velocity c. I don't go into what kind of dynamical laws there are for such dots. C. Next lets assume that in that some physical entity consists of these dots and every single point or place in x,y,z coordinates always is inside of some pulsating dot at any given instant of time - if you just zoom in to this point long enough. The pulsating dot around this point can for example be 10^-200 m (zero point 200 decimals) in diameter when it is at its largest. Or even smaller. Eventually, if you just go to enough small length scales and look this point or place, you will find a pulsating dot that covers such point. (D. It may be possible that Pulsating dot can be inside another pulsating dot.) I could also imagine that when you continue zooming the environment of that point long enough, i observe pulsating dot that has center exactly at that point. But this may not be necessary. Now, what happens if this entity is moving at a constant velocity v to some direction if you still demand that the frontier of the pulsating dots still is c? The answer is that 1. The form of the maximum frontier of every dot become ellipsoid that is larger by Lorentz factor 1/sqrt(1-(v/c)^2) 2. The period of the pulsation of every dot becomes longer also by Lorentz factor. But this 2. looks similar general change as relativistic time dilation of moving object in the theory of special relativity. I now attempt to raise question whether i can explain the Lorentz covariance and Lorentz transform assuming that the structure of all physical entities is dynamical this way - they consist of pulsating dots, when you just zoom in long enough. The entities are not static but undergoing continuos changes with frontier velocity c when you zoom in just long enough. Note that while they are not static they can still be stationary, or at least some of the properties of them can be stationary. Note that the entity in the picture above may not be stationary in its overall shape. I don't go here into the theory of quantum mechanics or generally standard model. I end here. What do you think?

This is a recent study that has observed that supermassive black hole growth could be coupled with cosmic expansion. This study seems to have gained much attention and also critizism. news article: https://www.ralspace.stfc.ac.uk/Pages/first-evidence-black-holes-source-of-dark-energy.aspx "Scientists find first evidence that black holes are the source of dark energy" research paper: https://iopscience.iop.org/article/10.3847/2041-8213/acb704 "Observational Evidence for Cosmological Coupling of Black Holes and its Implications for an Astrophysical Source of Dark Energy" (Published 2023 February 15) Shrinking matter theory explains this cosmological coupling by that when matter shrinks, the shrinking depends on proper time and black holes do not shrink since they have infinite time dilation. Therefore black holes appear to grow. There are some mistakes in my previous post regarding derivation of Friedmann equation and modification to stellar dynamics. The task is i think quite simple. i just have to somehow contribute both stellar dynamics and scale factor time evolution that all distances appear to grow by $$ r_(t) = r_0 e^{k(t-t_0)} $$ where k is parameter. There is still a problem with this theory: Earth-sun distance should appear to grow but it is observed to do that only 15cm/year.

About Friedmann equation and distance expansion: I couldn't manage to write correct equations. But i decided to write anyway what i am thinking about. My calculations were wrong. i cant multiply a with apparent a to get something like $$a_{obs} * a_{apparent}*a_{real}$$ this is wrong. I should start with stating that because matter is shrinking, all distances appear to grow by $$ r(t) = r_0 e^{k(t-t_0)} $$ This is the only thing i add to cosmological model at first. K is here a parameter. Besides the ordinary expansion of space, all distances just 'magically' appear to grow like this. (well not all - stars and planets do not expand) Maybe the law for planetary motion when matter is shrinking is then: $$ r_x(t) = r*e^{k(t-t_0)} $$ $$ a(t) = -\frac{GM}{r_x^2}$$ => $$ a(t) = -\frac{GM}{r^2}*e^{-2k(t-t_0)}$$ (?) IF thinking increments the orbital elements of the planet should be continuously changing such that the radial vector increases but velocity vector remains same. For this reason, the planet should gradually migrate away from the sun. I don't know does the eccentricity increase if you start with circular orbit. I know i could do numerical calculations by adding following increments: 1. Planet travels along Keplerian orbit a small increment 2.The radius vector gets increment but the velocity remain same - the planet is now in different orbit that is slightly further from sum but it has still exactly same velocity. I could look that situation on other perspective: as seen by non-shrinking observer. In this perspective the gravitation field of sun becomes different and shrinks. In Newtonian inverse square law gravitation, the observed change in gravitation is a' = La , 0 < L < 1. But what is L(t)? I know that L(t') = 1/LL(t') = e^{k(t-t_0)} and that t' = integral 0->t L(t) dt. Second question is - How to derive Friedmann equation from this new equation of planetary motion? I know that for Newtonian gravitation the first Friedmann equation in flat space becomes (Newtonian derivation of FE): $$ (\frac{\dot a}{a})^2 = \frac{8\pi G}{3}*\rho $$ On the other hand i suggested that in empty space the apparent expansion of scale factor is $$ a(t)= e^{k(t-t_0)} $$ Which is similar than in cosmological constant-only universe Could i from this correspondence just add A*k^2 to RHS of Friedmann equation? $$ (\frac{\dot a}{a})^2 = \frac{8\pi G}{3}*(\rho + Ak^2) $$ where A is certain constant (that i didnt manage to find from internet)? In this case the A*k^2 would be substitute to cosmological constant. I would need some kind of reference to make correct planetary motion equation and Friedmann equation that i failed to find for now. --- About the cause of shrinking (this is more open speculation but i write here some thoughts) I don't know the mechanism or process what would make matter to shrink. But i can describe how it could do that. It could be that if something happens to spacetime, for example the flow of time was accelerating could be the cause. Space or matter could be coupled with or stuck with flow of time that way, that it experiences shrinking when time accelerates. But what makes time to accelerate, i don't know. Maybe empty space differentiates but matter for some reason stay intact and shrinks. Space-time could be entity that is very dynamic in very small scales - i think that is what is needed. It could be full of continuously occurring events in a small scale. But not knowing this i can still describe something about possibility on shrinking of matter. What kind of principles hold. I selected to keep Lorentz covariance, constant light velocity, Heisenberg uncertainty principle, Photon energy law E=hf, De Broglie equation lambda = h/p and constant Planck constant. (Newton didn't know what causes gravitation but he knew how it works. He wrote mathematical description for it - the Newtons law of gravitation F = G M1M2/r^2. Now we know that gravitation field is not a force field, it is curvature of space-time. Newton's gravitation law is weak field approximation. And there is gravitational time dilation what newton didn't know also present in weak gravitational field) --- About curves I think if this theory gives almost similar Friedmann equation and time evolution of scale factor, then i think all other curves behaves also close to same way than in Lamda-CDM model. It will also give almost similar answer to nucleosynthesis.

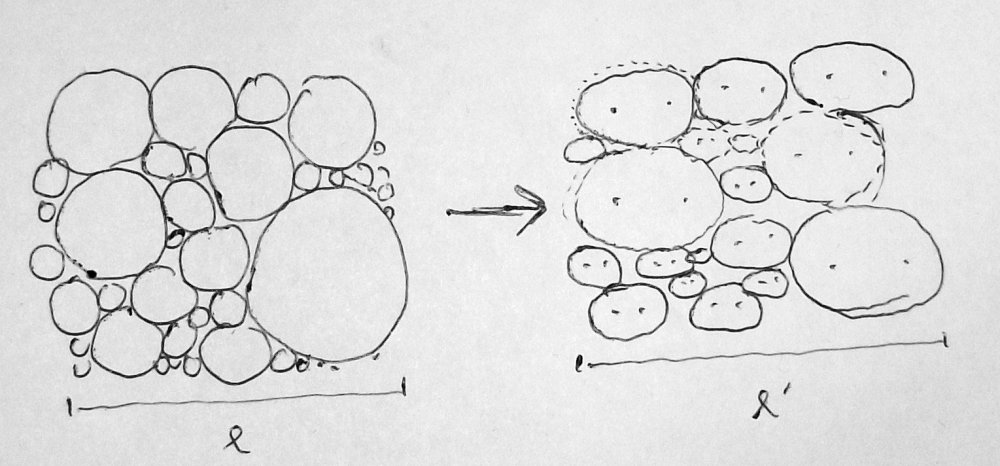
Thank you for those formulas and equations. I have to think about them for some time. (I hope the following math i represent does not disturb them.) I think this theory produces FLRW metric. But it is 'pseudo' metric or apparent metric, not real metric. (but only if all matter were shrinking similar way everywhere.) Actually the observed metric is combination of pseudo metric and real metric. But the Friedmann equation is slightly different. Since the metric is FLRW, solving the new Friedmann equation is i think quite simple. You just insert or substitute $$ a = a_{obs}/LL(t) = a_{obs}*e^{-k(t-t0)} $$ $$ \frac{da/dt}{a} = H = H_{obs} + k $$ to any kind of Friedmann equation or other equation or formula you have that describe expanding universe. k [1/s] is a parameter. I earlier guessed that k is a little higher than H0 but it could be something else as well. (But you have to assume that all matter is shrinking at equal rate in universe and the amount of black holes neutron stars and relativistic particles is negligible, and that there exist negligible amount of matter that belongs to different preferred scale - it would have different shrinking rate which causes that its gravitational influence and field changes relative to shrinking observer. All these matter would have different density components that depend differently from scale factor than ordinary matter) --- An example to solve Friedmann equation: I don't use here in this example Lamda-CDM what you did above - but old model where is no cosmological constant and i add shrinking of matter to it. Now i am considering situation where space is flat and there is ordinary expansion + gravitation + shrinking matter present in universe, but there is no cosmological constant - shrinking of matter is here a substitute for cosmological constant: $$ ds^2 = c^2dt^2 - a_{obs}^2(t)dr^2 $$ $$ a_{obs} = a_{real}a_{apparent} $$ $$ a_{apparent} = e^{k(t-t_0)} $$ $$ a_{obs0} = a_{real0} = a_{apparent0} = 1 $$ (Here i assume that apparent expansion that is coming from shrinking of matter is exponential) i mark: $$ a_{real} = a $$ in the following: $$ (\frac{\dot{a}}{a})^2 = (\frac{8\pi G}{3})(\frac{\rho_{m0}}{a^3} + \frac{\rho_{rad}}{a^4}) $$ First i get the ordinary Friedmann equation solution to solve a $$\frac{\dot{a} }{a} = H_0\sqrt{\Omega_{m0}(\frac{1}{a^3})+\Omega_{r0}(\frac{1}{a^4})}$$ $$\Rightarrow t = \frac{1}{H_0}\int_{0}^{a}\frac{xdx}{\sqrt{\Omega_{m0}x+\Omega_{r0}}} $$ (formula is taken from www.universeinproblems.com and modified by setting a/a_0 = a and a_0 = 1) Next step is just to insert to equation above the following: $$ a = \frac{a_{obs}}{e^{k(t-t_0)}} $$ With computer it might be quite easy to solve the inverse relation $ a_{obs}(t) $ numerically and then get other quantities and their curves (from 2nd friedmann eq and eq of state (?)) My guess is that all curves are just slightly different from benchmark model that has cosmological constant. (or are they?) --- About differences between this shrinking matter theory and expanding space theory: There are some significant differences in this shrinking matter theory. Some of them are in the range of observations. The differences of this shrinking matter theory from expanding space theory are: 1. Different kind of Friedmann equation - scale factor solution from 1FE is afterwards multiplied by LL-function that could be exponential LL = e^(k(t-t0)) , where k is parameter 2. Apparent expansion is velocity component - not a force - therefore it is not cancelled in solar system and milky way: R = R0*e^(k(t-t0)) 3. Black holes do not shrink 4. Neutron stars shrink less and old neutron stars start to have matter that has shrunk less than ordinary matter. 5. Relativistic matter shrink less 6. Gravitation of ordinary matter has very small delay effect by factor e^{kr/c} 7. There exist matter that prefers different length,time and energy scales. (I invented term 'scale difference' to describe the difference between two kind of matter that prefer different scales: There is matter that has scale difference) 8. Cosmological redshift - the apparent part - is now 'illusion' that comes from changes in shrinking observers meterstick,clock rate and energy units. However there may be ordinary expansion/contraction of universe present. 9. Universe can be contracting and at the same time it can look to be expanding in the viewpoint of shrinking observer. ---- 2,3,4,5 might be just barely in the range of observations 7 it may be possible to weight proton from meteorite sample - it is in range of measurements to see if it has more mass 7 may be difficult to observe from spectral lines since it is difficult to distinguish from doppler effect One problem with 2 : earth - sun distance should increase but it is observed that it does that only 10-15cm/year. ---

About nonlinearity: yes i know linear function is y=ax+b that is a straight line in xy-plane. This theory ,if there were only shrinking of matter present and universe is static, and assuming exponential apparent expansion, is similar than de sitter space and gives the age of CMB to be about 100 Byrs which is 7.5 times longer than current cosmological model gives. *** How i calculate the age of the universe in static + shrinking matter only universe: For age of CMB i solve following equation by assuming k = H0 = 0.0693 [1/Byrs] e^(H0(t'-t_now')) = 1/1100 => (t'-tnow')= Ln[1/1100]/(0.0693) = 100 Byrs (that is 7.54 times greater than in lamda-CDM model.) (I don't know can some observation verify that universe is 13.7 Byrs old, or is that extrapolation based on cosmological benchmark model. ) *** In that moment when universe is young i think some observables can go up but in this case they have gone long way. But they are nonlinear nevertheless. (I don't know which observations actually tells how old CMB and universe are or is it just extrapolation based on the benchmark model. I know that oldest known globular cluster stars are about 12Byrs old based on at least HR-diagram mainsequence cut-off.) If i add some amount of matter to this kind of space, it just pulls universe together and the observed apparent expansion is slower. (In principle gravitation could win the apparent expansion if matter is added to this universe more.) But if there were matter, radiation and 'ordinary expansion' but not cosmological constant and shrinking present in the universe the model gives somewhat similar age than benchmark model does. In this case the shrinking of matter would be substitute to cosmological constant. But the Friedmann equations are slightly different. Therefore i guess just in basis of the similarity with benchmark model - in this case all behavior of observables in early universe can go up when looking backward in time just the same way than in benchmark model. --- About the equations of change: I remind that L describes the factor of the shrinking - it is not directly scale factor (it is that only in the 'shrinking matter' + static universe). In order to get scale factor you need to solve friedmann equation. There is gravitation and may be ordinary expansion present in universe besides of the shrinking of the matter that also have effect on the time evolution of scale factor. LHS is dimensionless and L is dimensionless, also RHS is dimensionless. I think the correct way to write the equations with units marked in each quantity is: Equation for lengths : (s'[m])/(s [m]) = L Equation for duration of any event : (t')/(t) = L Equation for energies: (E'[J])/(E[J]) = 1/L Equation for masses: (m'[kg]/m[kg]) = 1/L and for example: Equation for forces: (F'[kg m/s^2] / F [kg m/s^2]) = 1/L^2 Equation for accelerations: a'[m/s^2]/a[m/s^2] = 1/L Equation for powers: (P'[J/s]/P[J/s]) = 1/L^2 m = meter s = second J = kg m^2/s^2 = Joule kg = kilogram --- About luminosity: You ask how does luminosity of distant star $$ L = F * 4\pi D_{L}^2 $$ appear to shrinking observer? -First In the viewpoint of shrinking observer, distant star has been bigger in past and has had 'expansion' by factor L>1 The light that was radiated by the star red shifted and the flux is time dilated which makes power go down like 1/L^2. 1) the power of that star is 1/L^2 times weaker Also since the meter unit of the observer has shrunk, the distance to that star is greater by factor L. 2) distance to that star is L times greater These two changes 1,2 makes luminosity of distant star to be 1/L^4 times weaker if it is observed by shrinking observer. Now if the universe was static, this 1/L would be exactly red shift: 1/L = proportional red shift ( in static universe, only shrinking of matter present in the universe ) --- About Temperature To get equation of change for temperature, i can look all the equations that connects energy,power,flux or wavelength to temperature. For example for black body radiation, Wien displacement law is lambda = b/T . I know that b must be universal constant and i get T'/T = b/(lambda'/lambda) = 1/L Or i can look Stefan-Boltzmann law flux = rho*T^4. Rho must be universal constant. Therefore i get (T'/T)^4 = flux'/flux = (power'/power)^2/(cross section area'/cross section area)^2 => T'/T = 1/L (Example: If sun would shrink by factor L=0.5, its mass gets 2 times greater, its temperature gets 2 times hotter and its flux becomes 16 times greater and total luminosity 4 times greater. However the nuclear reactions get only 2 times faster - all atom nuclei also change by certain ways: their interacting forces,radiating powers, masses, coulomb walls and binding energies changes also. But actually the star would behave as if light coming from it just have time dilation, redshift and that the star appears to been closer, in other words, the star just appears to behave as if the space was expanding and changing the picture and signal on the way)

About Rydberg emission lines: (This following may be unnecessary to write:) The Rydberg emission lines of hydrogen in distant universe should be similar than in expanding space theory. But now the light had red shift already when it was left from the matter in past. -atomic orbitals of matter have just been bigger in the past by factor (1+z), and the time needed for emission from exited state has been (1+z) times longer. The binding energy of electron would have been 1/(1+z) times less. But the velocity of light and velocity of electron have been exactly same. Planck constant has been exactly same also. it would be difficult to distinguish whether red shift is coming from expansion of space or from matter that has been bigger in the past. It is also difficult to distinguish whether red shift is coming from matter that is bigger or from Doppler effect. --- About non-linearity: You mean non-linearity = rapid spike up in the early universe in the redshift and temperature evolution curve? Both of the following are possible: 1 that there is ordinary expansion of space besides that gravity pulls matter together and matter shrink. 2 There is no ordinary expansion, only gravitation pulls universe back together and matter shrink. If 1 is true then i think the theory have almost similar non-linearity in very early universe than benchmark model. The difference is just slightly different Friedmann equations and their solutions. But if 2 is true i am not sure of that. In this case, non-lireaty can be achieved if the function LL(t') that might be something else than exponential, has been greater than exponential in early universe. But if not, then i think universe and CMB both should have been much older than about 13.7 Billion years. And that could have a problem or disagreement with current observations of early universe. I don't know which observations. If there were no gravitation present and 2 is true, the shrinking matter universe would be similar than de-sitter universe. (That is so if i assume that the factoral rate of apparent expansion is same all the time: dLL(t')/dt' = H_0 LL(t'). This assumption may be wrong.) In both cases 1 and 2 the universe would be asymptotically close to De Sitter universe in distant future. --- About equations of change: I think all equations of the change are correct, but i marked them in unclear manner. for example notation F'/F = 1/L^2 [kg m/s^2] means that when matter and its behavior changes, the ratio (new force/old force) is multiplied by factor 1/L^2 and what is in the bracket [] is just remainder that the dimension of force is kg m/s^2. The brackets is not a factor in equation. I could write the equation like this: new force = old force * 1/L^2 [remind that unit of the force is kg m/s^2] But it is a little more convenient to divide both sides by old force: new force/old force = 1/L^2 How do i calculate these equations? I start with these $t'/t = L$ $s'/s = L$ $m'/m = E'/E = 1/L$ I can end up to equation for force starting with: F'/F = (m'/m)(v'/v)/(t'/t) and i know already that m'/m = 1/L v'/v = 1 t'/t = L => F'/F = 1/L^2 and for example equation of change for power i start with P'/P = (E'/E)/(t'/t) and knowing E'/E = 1/L t'/t = L gives me that P'/P = 1/L^2 and equation of change for acceleration a'/a = (v'/v)/(t'/t) and knowing v'/v = 1 t'/t = 1 it gives => a'/a = 1/L ----- Actually (i forgot this statement earlier) if matter is shrinking continuously and the time is accelerating (duration of all events is all the time getting shorter) then equation t'/t = L holds only when t' and t are very short. The equation for elapsing time in this situation would be t(t') = definite integral(t1->t2) (1/L(t')) dt' (But who measures this kind of time t if all observers are shrinking?) ----- I earlier noted already that when i talk about apparent expansion of space observed by shrinking observer, i use function LL(t') that is not same as L. LL is a function that describes factoral change in photon wavelength or expansion of space if it was solely coming from the shrinking of matter - if they were observed by shrinking observer. LL is also expressed as function of the elapsing time of shrinking observer. I could mark this time as t'. LL(t') = e^[k(t'-t0')] LL(t0) = 1 If there were no gravity, a(t') would be just same as LL(t'). In this situation a(t') behaves exactly like in de sitter space. ---

Thank you for detailed answer! i am able to answer to 1,2,3 and maybe 4 but i don't understand 5. Actually the last paper considers black hole growth coupling with cosmic expansion and this shrinking matter theory also predicts that black holes should appear to grow when they are observed by shrinking observer. The following is quite lengthy and slightly repetitious. I think it is experimentally well established that: 1) If we do local observations, we see no change in standard model or gravitation over cosmological times. 2) If we do cosmological observations, we see -time dilation in signal -red shift in light -loss of photon momentum and energy -expansion of the space Also observations suggest that the velocity of light is constant, it does not accelerate. Light passes 299 800 km every second all the time. I basicly consider two things in this shrinking matter theory: A) I consider that the standard model and gravitation has a shift into smaller length scale as a whole. That would cause that fine structure constant and any of the coupling constants do not change over time if we measure things nearby us. B) And i consider that certain kind of changes in matter, standard model and gravitation, not only shrinking but multiple changes simultaneously, produces same cosmological observations that i mentioned above. But all these observations are now caused by that matter has been bigger and behaved differently in past - not by stretching the signal on the way. Therefore it only looks like that the space is expanding. these A) and B) gives that the effect of coupling constants remain the same except that the signal coming from distant place appear to have red shift and time dilation and space appear to expand. (and except that BH, neutron stars and relativistic matter behave differently) (It is possible that i did thinking error and The statement B) would not hold after all) Why these kind of things happens in matter? i don't know, but they might have some common cause. But what kind of changes are then happening in the behavior of matter? By demanding -that special relativity (for example factoral time dilation and factoral length contraction) holds -light velocity c = constant, -photon equation E = hf and -Heisenberg's uncertainty principle dp*dx = h/2pi with h being universal constant and demanding that all these three valid all the time and do not change, at least three changes should take place: 1)Matter shrinks (note that free photons do not shrink since they travel at light velocity) 2)time of the matter accelerates such a way that observation of light velocity stay constant 3)All energies, momentum and masses increase such a way that observation of photon coming from distant place appear to lose energy and momentum s'/s = L [m] all lengths t'/t = L - duration of all events in the behavior of matter E'/E = 1/L [J] energy of all events... p'/p = 1/L [kg m/s] momentum of any object or particle m'/m = 1/L [kg] mass of any object or particle ,where 0 < L < 1 is factor of the change (What do i mean by 'accelerating time of matter'? it is the shortening of duration of every event happening in matter, for example spin rate of the nucleus of deuteron and half life of carbon-14 and so on.) By doing dimensional analysis from the three statements 1),2),3) above, i also get following changes in dynamics of matter: f'/f = 1/L frequency [1/s] v'/v = 1 velocity [m/s] a'/a = 1/L acceleration [m/s^2] F'/F = 1/L^2 strength of local forces [kg m/s^2] P'/P = 1/L^2 power [J/s] T'/T = 1/L temperature [K] f'/f = 1/L^4 luminosity [W/m^2] where 0 < L < 1 (Note that any field like force field or potential would also shrink into smaller size) When this kind of change in matter happens it gives theory that passes so called Tolman test states that in expanding universe, surface brightness should be inverse proportional to the fourth power of cosmological redshift. This model gives this result. (except keeping in mind that compact objects shrink more slowly and black holes do not shrink at all) f = A/(1+z)^4 where A is some constant These kind of changes also produces Robertson Walker Metrics in flat space - which is now only 'apparent' metric, not real metric. ds^2 = c^2dt^2 - a(t)dr^2 There are important differences from expanding space theory. The three differences are that 1) expansion of distances is not a force - it is 'apparent growth' that can be seen as velocity vector. Also the Friedmann equations are different for the same reason. And 2) compact,relativistic matter and matter in strong gravitation field shrinks more slowly and black holes do not shrink. It is also now possible that 3) there exist matter that belong to different scale or have shrunk differently in the past. The time dependency of scale factor a(t) IS NOT LINEAR in this theory. IF cosmological principle holds, the observed change in distances if gravitys effect is reduced and photon wavelength are all the time same during same time interval of time of the shrinking observer. This would give exponential law: dL/dt = k*LL LL(t) = e^[k(t-t0)] = a(t) Note that L is now a function (of time of a shrinking observer t - not constant time t) and LL(t) > 1. it is not the same than L in the equations above. I used to mark this function as LL(t). But because gravity slightly pulls matter together, the real time evolution of scale factor is only nearly exponential, but it will eventually come close to exponential since the effect of gravity becomes all the time smaller. (this may be wrong pair of equations) 1)a(t) = a_f * e^[k(t-t0)] 2)((da_f/dt)/a_f)^2 = (3piG/8c^2)* rho(t) (Note also that the equation 2) does not need cosmological constant to get accelerating apparent expansion.) The rough linear approximation a(t) = 1 + H0(t-t0) i use only to get value for k that is close to H0 and to estimate how much distances should grow in solar system dr(t)/dt = (roughly) r(t0)*H0 This equation (that radius vector r increases or 'appear to increase' should be taken then account in numerical simulations. It does make escape velocity smaller like you said. --- I think nucleosynthesis and recombination are both possible in this shrinking matter theory and gives same results. Also inflation is possible - now inflation may be a period where matter shrinks very fast during short period of time. --- I hope this clarifies what i think of the possibility that certain kind of changes in matter, standard model and gravitation - simultaneously could give almost same cosmological observations than expanding space gives. .