Sarahisme

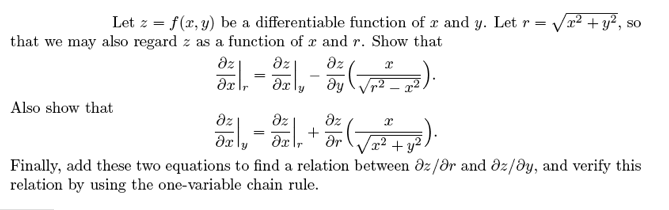
well this is what i have done so far, but i don't really know what i am doing... z = f(x,y) and [math] r = \sqrt(x^2 + y^2) \implies y = \sqrt(r^2 - x^2)[/math] so z = [math] f(x, (r^2 - x^2)^{0.5})[/math] so [math] \frac{\partial z}{\partial x}|_r = \frac{\partial z}{\partial x} \frac{\partial x}{\partial x} + \frac{\partial z}{\partial y} \frac{\partial }{\partial x}(r^2 - x^2)^{0.5} [/math] [math] = \frac{\partial z}{\partial x} - \frac{\partial z}{\partial y} \frac{x}{(r^2 - x^2)^{0.5}}[/math] but yeah... now i'm stuck

Hi all, i am having real difficulty doing this partial deriv. problem! would anyone be able to help me? (i am not even sure where to start really! ) Thanks Sarah

now for part ©: using the formula: [math] F =( \frac{n_1 - n_2}{n_1 + n_2} )^2 [/math] , where F is the fraction of power that is reflected back. we know [math] n_1 \ and \ n_2 [/math] from part (a) so plugging these values in we get: F = 0.0348 but only 1% of the input power makes it to the end of the fibre (that is [math] \frac{I_{out}}{I_{in}} ) [/math], so the amount of power reflected from the end of the fibre (as a fraction) is [math] F \frac{I_{out}}{I_{in}} [/math] So the amount of power reflected from the end of the fibre is: [math] F \frac{I_{out}}{I_{in}} = (0.0348)(0.01) = 0.000348 [/math] which as a percentage is 0.0348% now i just relised that there is another bit to part ©, which is that we also have to find the fraction of power that arrives back at the begining of the fibre as a percentage of the input power. so to do this: so we know only 1% of the power leaving one end of the fibre reaches the other end. so the amount of the reflected power that arrives back at the begining as a fraction of the input power is (0.01)(0.000348) = 0.00000348 = 0.000348% so how was that for part © ??

now for part (b): we are given that : [math] \alpha = -0.2 dB/km[/math] and since [math] \alpha = \frac{10log_{10}(\frac{I_{out}}{I_{in}})}{L} [/math] we want to find [math] \frac{I_{out}}{I_{in}} [/math] so rearranging gives: [math] log_{10}(\frac{I_{out}}{I_{in}}) = \frac{L \alpha}{10} [/math] plugging in for the RHS gives: [math] log_{10}(\frac{I_{out}}{I_{in}}) = \frac{(100km) (-0.2dB/km)}{10} = -0.2[/math] so [math] \frac{I_{out}}{I_{in}} = 0.01 [/math] and to get [math] \frac{I_{out}}{I_{in}} [/math] as a percentage, you multiply by 100 so therefore the fraction of power arriving at the end of the 100km fibre as a percentage of the power entering the fibre is 1% how was that for part (b)??

ok here is my working, (a) [math] L_1 = 100 \times 10^{3} m [/math] [math] \lambda_1 = 0.5 \times 10^{-6} m [/math] [math] \lambda_2 = 1 \times 10^{-6} m [/math] so [math] n(\lambda_1) = 1.459 - 0.002(0.5) = n_1[/math] and [math] n(\lambda_2) = 1.459 - 0.002(1) = n_2 [/math] now [math] n_1 = \frac{c}{v_1} \implies v_1 = \frac{c}{n_1} [/math] [math] v_1 = \frac{L_1}{t} \implies t = \frac{L_1}{v_1}[/math] likewise [math] n_2 = \frac{c}{v_2} [/math] [math] v_2 = \frac{L_2}{t} [/math] so [math] v_2 = \frac{c}{n_2} [/math] [math] L_2 = v_2t [/math] so [math] L_2 = \frac{c}{n_2} \frac{L_1}{v_1} = \frac{c}{n_2} \frac{L_1n_1}{c} = \frac{L_1n_1}{n_2} [/math] so the difference in length between the fibres is: [math] |L_1 - L_2| = 100 \times 10^{3} m - \frac{L_1n_1}{n_2} m [/math] plugging in the values gives: [math] |L_1 - L_2| = 68.63 m [/math] so how was that for part (a)?

oh i think what i've got means that 3.48% of the 1% of the power that makes it to the end is reflected...right? part (a) is the one i am most unsure about, to do that i just used the fact that n = c/v and v = L/t

???

Hey i am having a few problems with this question related to optical fibres. or at least i think i am . if i put up my answers, could someone who has a little bit of free time tell me if they are correct? Thanks Sarah Here is the question: my answers are: (a) [math] L_2 - L_1 = 68.63 m [/math] (b) 1% © [math] F =( \frac{n_1 - n_2}{n_1 + n_2} )^2 [/math] , where F is the fraction of power that is reflected back. so since [math] n_1 = 1.459 - 0.002(0.5) [/math] and [math] n_2 = air = 1 [/math] the plugging this into to F gives : [math] F = 0.0348 [/math] so therefore the fraction of power reflected from the interface as a percentage of the input power is 3.48 % how was all that?

right o , thanks swansont!

ok, cool, thanks! whats wrong with the units? their standard specific heat unit arnt they? (they use the same units for specific heat in the question)

hey i've got a bit of a tricky (i think it is anyway ) specific heat problem, and i just wanted to check that i'd got it right. so if anyone has a couple of mins free, could they please check my answer? Thanks -Sarah my answer is : [math]c_m = 450 \frac{J}{kg.K} [/math]

ok, its good, i've got it now! thanks for the help Tom

what does this notation mean? "P=[pij[/sub]]"

ok , yep i can do this bit, its the proof i ama having trouble with i think.... a non-diagonalisable 2x2 complex matrix is: [math]A=\left[\begin{array}{cc}0&i\\0&0\end{array}\right][/math] hows that? however i don't know where to start with the proof... :S

anyone?

nup its ok i know what i did wrong! i should have taken the unit vector qF!!!! woohoo i solved it! :):D:D:D:D:D:D

hey, i am not sure how to approach this problem.... any suggestions on how? Thanks Sarah

i hope this is this right graph....

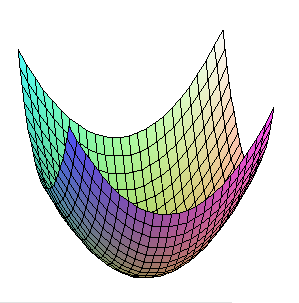
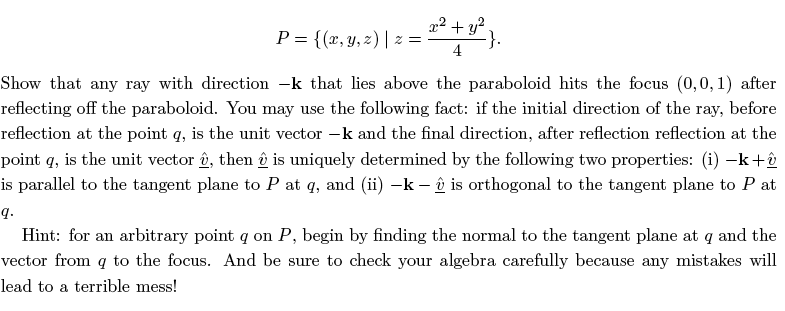
ahhh i don't see where i have gone wrong, i think i've made a small slip somewhere (either that or i am way off track ) anywho, heres what i did (the question is attached as a picture)... Let q = (a,b,c), so c = [math] \frac{a^2 + b^2}{4} [/math] now i get the tangent plane to P at q to be z = [math] \frac{a^2 + b^2}{4} + 0.5a(x - a) + 0.5b(y-b) [/math] and the normal at q to be n = (0.5a)i + (0.5b) -k then i call the vector qF the vector from q to the focus so i get qF to be qF = <-a,-b,1-c> then i figure all i need to do is show that (-k + qF) is parallel to the tangenet plane to P at q. That is that (-k + qF) is perpendicular to the normal vector at q. I then also need to show that (-k - qF) is orthogonal to the tangent plane. That is that (-k - qF) is parallel to the normal vector at q. To show that (-k + qF) is perpendicular to the normal vector at q i use the dot product rule so i need to show that (-k + qF).(n) = 0 likewise for (-k - qF), i need to show, using the cross product, that (-k - qF)x(n) = 0 and it almost works but i think the normal thing show have [math] \frac{1}{4} [/math]'s in it....anyway...does anyone know where i have gone wrong? or did i ever even go in the right direction to begin with? Thanks Sarah p.s. i also attached a picture of the parabaloid (in the next post) if that helps people ( i think its the right graph, i don't use maple much...) is it the right graph?

right ok, thanks

hello?

nevermind, i think what i've got is correct!

oh yeah, i just wanted to know if i had done things correctly? :S

[math] m = 0.1g = 0.1 \times 10^{-3} kg [/math] [math] \Delta t = 0.1 s [/math] PE of ball = [math] (0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m) [/math] but only 0.05% of it is converted into a sound wave so : Energy (E) = [math] (0.0005)(0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m) [/math] Now Power is Energy per unit time, so Power (P) is: [math] E = \frac{(0.0005)(0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m)}{0.1s} [/math] Intensity is Power per unit area (since it can be approimated to a point source of sound the area is the surface area of a sphere, [math] 4 \pi r^2 [/math], so Intensity is : [math] I = \frac{(0.0005)(0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m)}{(0.1s)(4 \pi r^2)} [/math] Since we are given that the lowest audible intensity is [math] 10^{-11} Wm^{-2} [/math] then we set I = [math] 10^{-11} Wm^{-2} [/math] so we have: [math] \frac{(0.0005)(0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m)}{(0.1s)(4 \pi r^2)} = 10^{-11} Wm^{-2} [/math] So then rearranging and solving for r gives: r = 200 m ( to 1 sig. fig.) now for part (b) we just set I = [math] 10^{-8} Wm^{-2} [/math] instead, so we have : [math] \frac{(0.0005)(0.1 \times 10^{-3} kg)(9.81 ms^{-2})(1m)}{(0.1s)(4 \pi r^2)} = 10^{-8} Wm^{-2} [/math] now rearraging for r gives : r = 6 m (to 1 sig. fig.)

anyone going to help me at all here?