steveupson

I’m quite sure that I understand what you’re getting at, and I think I understand the way that this should be properly explained (mathematically.) We consider the basis of the sine to be a ratio (mathematically) when in real fact it is based on an actual quantity (mathematically.) Work is currently being done on this front. The distinction is very subtle, hence your inability to pin it down any better. I just want to validate that what you say is correct, despite the reactions from the skeptics. The new geometry which includes the turn as a base quantity is called synchronous geometry. If anyone fails to understand the mathematical proof of the existence of a turn as a quantity, please feel free to ask questions. If anything that I’ve said here (or elsewhere) can be falsified by any method whatsoever, I’d sure be interested to hear about it.

and now for something completely different... This is the key identity: [math]\alpha={\cot}^{-1 }(\cos\upsilon\tan{\sin}^{-1}(\frac{\sin\frac{\lambda}{ 2}}{ \sin\upsilon}))[/math] We know that, since space is isotropic, the identity must hold true both locally and globally. This is not an issue in flat Euclidean 3-space. However, once we include time into the model and are confronted with relativity, some issues arise. How does the identity remain true both locally and globally? The answer appears to be that [math]\alpha[/math], [math]\upsilon[/math] and [math]\lambda[/math] are not only dimensionless quantities as we tend to think they are, but they are also actual quantities (or scalars, or numbers, or a measurable entity, or however you wish to say it.) Because this is the case, the addition of time into the coordinate system can be accomplished by understanding that, when this is done (when time is introduced), the coordinate system must wrap back on itself. This is an abstract solution, but it illustrates the problem with having this new identity hold true in the global and local domain. By this I mean that the equation holds true for both sets of points, those that are adjacent and those that are not. Once you grasp the significance of this reality it becomes clear that the dark energy doesn't exist in the "model" and that's why we don't see it. Once energy becomes oriented such that it is 90 degrees to the "model" it disappears because its amplitude goes to zero while its wavelength goes to infinity. It's a geometric artifact created by the way we choose to make the "model" mathematically. An alternative model uses time, length and direction as the three dimensions. We have coined the term "synchronous geometry" to differentiate this model from the standard model. In this model things flip symmetrically with the way they are represented in the standard model. In synchronous geometry the length triplet that is used to identify a point or event is replaced with a direction triplet. The vector quantity that is present in the standard model (direction) becomes the scalar quantity in the synchronous model. Following along, the scalar value (length) in the standard model becomes the vector quantity. In the standard model the direction has no magnitude or scalar quantity or length. In the synchronous model where things are swapped, the length has no direction (think of the unoriented radius of a sphere), since direction is the scalar quantity in this version of spacetime. Because this can be done, it becomes clear (or murky) that there should be an additional effect on the phenomenon that we call frame-dragging, which is more clearly another artifact of the way we are choosing to do the math. Rather than try and explain the math to you (since I don't have the algebra skills to write the expressions that would be necessary to get the point across to you), I can suggest another thought experiment. The rings of Saturn are orbiting the gas giant and are frame-dragging spacetime around with them. If we were to look closely at the interface between the plane in which the frame-dragging is represented mathematically, and the adjacent spacetime, we should see one of two things: either the spacetime that the gas giant occupies adjacent to this plane interacts identically with the frame that is being dragged, or, the two opposite sides of the plane interact differently due the effect on spacetime caused by the Sun's gravity. In other words, the advancing and retreating sides of the frame that is being dragged, relative the the Sun's field, will produce different effects. Doesn't this mean that the spacetime itself is rotating in the frame? I think it does mean that, except that I don't have the math skills to show it one way or the other. In any event, the dark matter question is another effect caused by the same issue with how we do the math. It isn't a trivial thing to understand this or to explain it to someone. It is impossible to explain it to anyone who doesn't understand the identity above, or what it means. My best description of it is to say that directions or angles can be used to construct a coordinate system in a symmetrically identical manner as what is done in the Cartesian system. I''ve been looking at how we model quanta mathematically and sure looks like the same question arises there as regards to how we are using the standard model of spacetime. In the synchronous model it is almost trivial to show how energy states transition at the rates at which they occur. There isn't really a step-function, but rather a hyperbolic function that goes to zero. Also, once direction is viewed as a quantity it become clear (or murky) that direction has no sign associated with it, which very likely explains spooky action at a distance. We have time and distance which each have signs associated with them and direction which does not have a sign associated with it. These are the three quantities that exist in synchronous geometry. When distance ([latex]l[/latex]ength) and [latex]t[/latex]ime are combined in spacetime the result is bounded by [latex]-c[/latex] and [latex]+c[/latex]: [latex]-c\leq lt \leq +c[/latex] When direction ([latex]\tau[/latex]urns) and [latex]t[/latex]ime are combined in spacetime the result is bounded by zero and infinity: [latex]0\leq \tau t\leq \infty[/latex] Note that the sign is actually associated with length rather than direction. This has an effect when spacetime is curved.

[tex]r_s[/tex] [math]r_s[/math] [latex]r_s[/latex] [math]\alpha={\cot}^{-1 }(\cos\upsilon\tan{\sin}^{-1}(\frac{\sin\frac{\lambda}{ 2}}{ \sin\upsilon}))[/math]

The list seems to be a mashup of length and time. There doesn't seem to be any pattern or symmetry to it. At L0 the constants at T0 are based on (time mass length), (mass), (time mass direction current), (length current) At L1 the quantities at T0 are based on (length), (time length) At L2 the quantities at T0 are based on (length) At L3 the quantities at T0 are based on (length) At L4 the quantities at T0 are based on (time length), (time current) At L5 the quantities at T0 are based on (length) (time mass length current) I don't understand the relationship between these first two columns and the remaining ones. I understand that there are fundamental properties or quantities that are used to express other stuff. Currently there are only seven fundamental quantities that can be expressed in base units. Currently, there is no recognized quantity for direction and we use pi, a dimensionless quantity, as a proxy for the property of direction. on edit> change T1 through T5 to T0

The list seems to be a mashup of length and time. There doesn't seem to be any pattern or symmetry to it. At L0 the constants at T0 are based on (time mass length), (mass), (time mass direction current), (length current) At L1 the quantities at T1 are based on (length), (time length) At L2 the quantities at T2 are based on (length) At L3 the quantities at T3 are based on (length) At L4 the quantities at T4 are based on (time length), (time current) At L5 the quantities at T5 are based on (length) (time mass length current) I don't understand the relationship between these first two columns and the remaining ones. I understand that there are fundamental properties or quantities that are used to express other stuff. Currently there are only seven fundamental quantities that can be expressed in base units. Currently, there is no recognized quantity for direction and we use pi, a dimensionless quantity, as a proxy for the property of direction.

Sweet!

Frame dragging is a very specific example of rotating spacetime. Are there any other examples that have been theorized or proven? The specific example that comes to mind is the effect of a rotating massive disk on local spacetime. For example, the rings of Saturn might cause a local rotation in the spacetime surrounding the planet and that this rotation interacts with adjacent non-rotating space in order to create unexplained high winds along the equator of the planet's surface. I often have difficulty when researching things like this because it isn’t much talked about, and if it has been explored then it usually goes under some obscure name that makes it even more difficult to find. Do you know if rotating massive discs have been looked at mathematically? The difference would seem to be, at first glance, that frame dragging occurs in the field outside of the rotating mass while this other type of rotating spacetime would occur in the field that is inside the rotating mass.

He doesn't have the courage or endurance to last out the week. He'll take his marbles and go home leaving a mess in his wake. He believes that he's too special to have to put up with being treated as if he isn't above the law. There'll be a mass of pardons for all his family and all his cronies on his way out the door. He never would have signed onto the job if it weren't for the pardon powers.

To explain how this pertains to the OP, it is necessary to return to first principles. Beginning with Newton, I'd direct your attention to the paper on the Origins of Torque that was linked in post # 97. http://www.scienceforums.net/topic/99258-how-does-a-body-know-how-to-move/page-5#entry950396 This is important because it tries to provide an explanation of how force germinates. Using their approach, they show that when we try and model a rigid two-body it becomes difficult to make the math work properly, based on our normal use of the definitions of vectors and geometry and such. In order to resolve the conflicts in the math, they assume that there must be some deep connection that doesn't allow for rigid bodies to occur in nature. This is a solution to their problem, and it's probably the most obvious, although I don't believe that it's the most elegant. There's another way to look at this question. Before I go too far into this, I need to reiterate that a change in position can be viewed as a change in either distance or direction, or both. This is important because if we were to disconnect distance and direction from each other, then we can come up with a plausible scenario where F=ma can be explained a little differently than using this non-rigid body approach. The acceleration is the rate of change of velocity, and the velocity is the rate of change of position, or, the rate of change of direction or distance, or both. Again, if we can break the mathematical connection between direction and distance, and if we can then tease out the direction from the distance, then we can have a change in position that is solely a change in direction, without any changes in any lengths. I know, it sounds odd, but it's only math. We've always seen direction as a purely ratiometric quantity, but that's only because its the way we've always done the math. What makes this relevant is that direction does parse out differently when we use ct,x,y,z coordinates. If we have a point mass at xyz at ct, and its still in the same location in space at ct' (or, in other words, if xyz = x'y'z'), then the point mass is moving through spacetime in a defined direction. If a force causing an acceleration occurs, or if gravity acts to change the magnitude of t, then the direction that the point mass is moving through spacetime will also change, regardless of what happens to xyz. The least action mathematically is to have the force (or gravity) cause only the change in t, and then this change in t is what results in the acceleration. This way of viewing how a body in motion knows to stay in motion leads to an observation that everything has a future that lies in a precise direction, and that this direction is all that needs to change, mathematically, in order to achieve the relationship F=ma. What makes this look like nonsense is only the fact that we have always dealt with direction as a ratio and not a quantity. Once it is recognized as a base quantity then it is a simple matter (mathematically and conceptually) to separate direction out from distance. This distinction has no relevance at all until it is applied to the spacetime construct. Directional relationships in Euclidean 3-space take on a different importance when they are combined with the direction of another quantity, which in this case is the quantity time. In another discussion at another site, the following explanation was produced, utilizing the rotational equivalent of the third law. I hope it's self explanatory as to how this relates to this question. http://mymathforum.com/physics/72236-physical-property-direction-7.html A close look at the method that was used in order to dodge the rigid body assumption reveals: [LATEX] = (r_1 - r_2) m_1 r_1 \alpha + r_2 (m_1r_1 \alpha+ m_2 r_2 \alpha) [/LATEX] Since this introduces a change in angular velocity, and since angular velocity is the derived quantity that determines direction, can angular acceleration be viewed as a derivative of direction? We understand that the direction of the angular velocity vector doesn't change with a change in angular velocity, but is that really the case? Can the direction become more so? I don't think that there's any proof one way or the other on this. There's simply the fact that we've always done it this way. Of course, the reason that we assume that there isn't a change in direction of the angular velocity vector could be caused by the way we apply Euclidean 3-space to spacetime. I don't think that there can be any difference between the two constructs in Newtonian spacetime, but it appears that there certainly could be a difference in relativistic spacetime. Most of the stuff that I obsess over has no real meaning in flat spacetime, but it could have significance when applied to curved spacetime. As for your suggestion about using a direction and a radius for identifying a position, there's a more interesting use for combining a direction and a radius. We can use a direction and a radius to specify a volume, and this raises some other issues that have to be explained somehow. The SI derived unit for volume is [latex]{m^3}[/latex]. But that's not the only way to specify a volume. If we know the radius of a sphere we can calculate the volume inside the sphere using a simple formula. But what happens if we take a partial area of the surface of the sphere and use this to calculate volume? The most straightforward method is to use the steradian (symbol [latex]sr[/latex]) as the partial area of the surface of the sphere. The steradian (see the SI chart in the above post) is referred to as the derived quantity for solid angles, but in this case its just as appropriate to look at it as the area of [latex]{r^2}[/latex] on the curved surface of a sphere. The procedure is to take the volume of the space that lies within the steradian and the sphere center. To make this into a volume we'll take the product of the angular quantity of the [latex]sr[/latex] and the length quantity of the meter. Well call this unit of volume the steradian meter, or [latex]srm[/latex]. There are [latex]{4}\pi[/latex] steradians in a complete sphere, so there must also be [latex]{4}\pi[/latex] units of volume that will be equal to the volume of the complete sphere. The first thing to notice is that the [latex]srm[/latex] can use either direction or length as the unit quantity. A quantity of [latex]3sr[/latex] where [latex]r=1m[/latex] is different than the quantity of [latex]1sr[/latex] where [latex]r=3m[/latex]. Mathematicians will probably know of a convention that can be used in order to capture this difference. If we put the number in the appropriate location to show which quantity is the unit quantity and which quantity the number is associated with, then the first case would be written as [latex]3srm[/latex] and the second case would be written as [latex]sr3m[/latex]. A little arithmetic reveals that: [latex]1srm = sr1m = \frac{1}{3}m^3[/latex] and also, for example: [latex]sr8m = 512srm = \frac{512}{3}m^3 [/latex] If space is simply an empty volume, these three examples of expressing a volume should have identical meanings. They should all be identical, both mathematically and conceptually. The problem is that there is a difference. The expression [latex]\frac{512}{3}m^3[/latex] has no spherical excess, [latex]sr8m[/latex] has a spherical excess of [latex]\frac{\pi}{2}[/latex], and [latex]512srm[/latex] has a spherical excess of [latex]256\pi[/latex]. I guess it would be possible to pretend that this difference has no relevance at all. Once again, I think were up against the same mathematical quandary. The difference between using unit length or unit direction to express a volume has no impact at all on Newtonian spacetime where ct is a constant. But it does raise a whole universe of issues once we try to use these two different methods to express a volume in relativistic spacetime. Some of this explanation (like the stuff about the direction of the angular velocity vector) is merely speculation at this time. The algebra hasn't been worked out for most of that yet. But enough has been accomplished to know that it won't be as simple as some of the stuff that has already been done. It would be necessary to show that gravity has an impact on the direction of the angular velocity vector and it looks as though this cannot be accomplished using conventional geometry. This is one of the reasons why a coordinate system that uses directions instead of lengths is being suggested. It appears that there's a portal that exists between Euclidean 3-space expressed as a system of lengths and Euclidean 3-space expressed as a system of directions. We should walk through it. On edit>>>> removed rant

A right angle isn't simply a condition where Pythagoras holds true. I mean, of course that's all there is to it in 2-space. In 3-space it's more than that because we can express the orthogonal angles as a interrelationship between all of the angles that make up the space. This description or definition will hold true both locally and globally. We don't need any length or what you're calling a metric in order to define orthogonality. The relationship that is expressed in the equation defines all angles in the system as a function of the orthogonal axes. And it makes all of the angles in the entire coordinate system relate to one another. This is independent of length or metric. It uses angles to relate all the angles to one another. In other words, the x, y, and z coordinates of a position should be able to be expressed as three directions and thereby represent a length that has no particular orientation (other than being positive or negative.) The radius of a sphere is a length like the one I'm talking about that has no particular direction. It's the exact inside-out version of using three lengths to define a direction that has no particular length. One more time, the best way to state this is to say that the equation expresses the relationship of all angles relative to orthogonality at once, or synchronously. I've spent some time studying the implications and I sure wish you would take a closer look.

Why don't you think it's useful? Can you explain why I'm wrong? The math is either correct or incorrect, isn't it? Show me the error. Explain the error to me. And, I don't know how to do any productive calculations using this new information. All I can do is show how and why the geometry is not correct when you try to do the math the way you are describing. It's an order of magnitude more complicated than that what you are doing. [latex] ds^2=(dx^0)^2-(dx^1)^2-(dx^2)^2-(dx^3)^2 [/latex] In this expression, the last three coordinates no longer contain the relationship that I'm talking about. The reason is obvious to me, but for some reason you cannot see it. Basically, when there is no curvature, the sine function is the dominate (or operative) setting for expressing physical phenomena that involve Euclidean 3-space, and so the expression is fine for describing Newtonian spacetime because in that case the first element doesn't vary. When curvature is maximum then the hyperbolic function dominates. This has to happen when you mathematically start to vary all of the components. Of course you have to understand the equation to understand why this is true. I'm sure you must have had basic trig somewhere in your studies. How do you explain a smooth function that maps the angles from 0°as a sine to 90° as a hyperbola? If you haven't seen this done before, why haven't you? And if you don't think it is significant that there is a way to map spherical excess as a curve, then why do you think it's not important? The physics we're trying to model is nature. The model that I'm talking about will do everything you want, and more. I just don't have the algebra skills necessary to communicate the math. You may recall that when we first started this discussion we were at odds because I couldn't show the algebra. Now I've shown the algebra. It was an effort. It would be nice if someone, anyone, would take the time to do the math and comment on the math. on edit >>>> Of course I see the problem. And I don't know the complete answer to it yet. The thing is, just as using a triplet of lengths yields a direction, a triplet of directions yields a length. http://www.scienceforums.net/topic/101726-formalizing-length-as-an-antivector-algebra-help-requested-once-again/page-2#entry986027 second edit >>>> Not to be snarky or anything, the mathematical rigor that I'm looking for is someone to look at the equation I've presented and then comment on what they think the equation shows. For me, I see the equation as a partial invalidation of your statement 3) in that if the coordinate system is to remain orthogonal then it doesn't work the way you think it does. If orthogonality isn't a requirement, then sure, your way works fine, but it isn't doing what you think it's doing.

Yes, exactly. The mathematical methods are important. The issue that I'm talking about has nothing at all to do with what you think it has to do with. If you cannot understand me when I try and explain the math that I'm talking about then at least let me know what you think the math is telling you. What do you make of the expression: [latex] \alpha={\cot}^{-1 }(\cos\upsilon\tan{\sin}^{-1}(\frac{\sin\frac{\lambda}{ 2}}{ \sin\upsilon})) [/latex] If this expression has no meaning to you, then you won't understand a word I've said. It is impossible to understand unless you do the math. on edit>>>>> In my childish way of speaking, the expression is a characteristic of orthogonality. This characteristic is not captured by the vector calculus that you are trying to explain to me. In my childish way of speaking, the reason this characteristic is not captured by the vector calculus is because the characteristic relates all three orthogonal directions synchronously, in a single expression. It's very similar to the mathematical distinction between finite rotations and infinitesimal rotations. This is a distinctly different characteristic and it hasn't been noticed until now. You cannot find this in any of the literature because it's new.

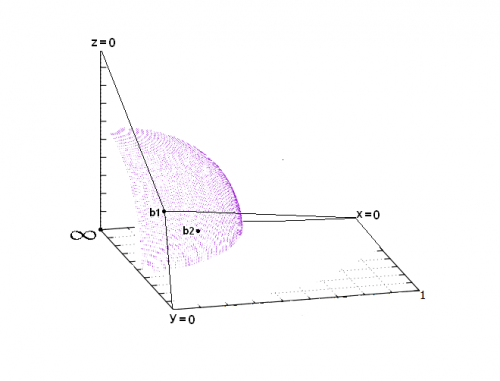
The legends for the Bizarro vector are not correct in the above post. In any event, the basic method is that there are angles [latex]\infty\,\,{x_0}\,\,{a_1}[/latex], [latex]\infty\,\,{y_0}\,\,{a_1}[/latex], and [latex]\infty\,\,{z_0}\,\,{a_1}[/latex]. When these are all expressed as the area [latex] A=(f)\upsilon [/latex] then the sum of these angles is equal to the sum of the similar angles for point [latex]a_2[/latex].

I’m pretty sure that I understand the principles (math and physics) that underlie this approach. The article is very good, and I think I understand the gist of how this approach is used. It is a much more straightforward explanation than what I’m used to. I feel as if we’re finally on the right track here. When you use the term spacetime curvature, I am assuming you mean the gradient between adjacent vector spaces. The way the math is accomplished this gradient is expressed as a relationship between adjacent points of a manifold, or at least that’s how it looks to me. The problem with this approach is that although it may connect space and time in a relativistic manner, Euclidean 3-space has a previously unknown relationship that exists between the spatial coordinates. This is due to them being mapped orthogonal to one another. The circumstance that is caused by them being mapped orthogonal is not differentiated in the method that is being used, and therefore it is lost. The space in spacetime that is being represented in this manner does not reflect real Euclidean 3-space. If you were to open any book on spherical trigonometry, all of the mathematics is based on the function given in the above post. The function is what makes Euclidean 3-space work with time to create spacetime. It explains how the curvature actually manifests, and how it is able to wrap back on itself. Since the relationship is expressed as a two-dimensional curve, I don’t know how it would be possible to differentiate it. I know that there has to be a way to show how Euclidean 3-space is slightly different than the way it’s being represented in relativistic terms. It has to be different than the approach of using 1-forms. I’m presently convinced that it involves calculating Bizarro vectors where coordinates are expressed as a triplet of directions. I don’t see any other way to proceed with this line of advancement, but then, I’m not a mathematician, either.

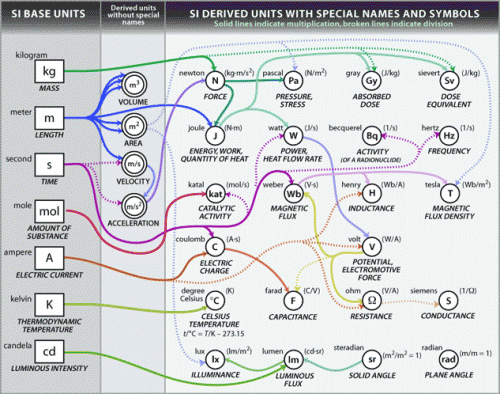
The boat knows the water is flowing because of friction. How does the particle know how spacetime is being bent? What could constitute the friction in your analogy? Every particle occupies a position in spacetime and every position in spacetime has a future. The manifestation of force can most simply be seen as a change to these futures. Gravity changes the velocity (combination of speed and direction) of spacetime locally. This is no different from the way acceleration occurs. In either case, the futures of events are modified in a systematic process. The process can be seen (mathematically and conceptually) as beginning with a change in the direction of the future of each event. This change occurs differently than the way we would normally view a change in position. We normally view a change in position as involving movement that can be expressed using the base quantity of length, in the base units of meters. When we view a change in position in this manner, we have no way of understanding what happens instantly upon application of a force. All we can consider is what happens during an infinitesimal. This limitation is caused by the way we do the math. There has to be another method of doing the math where we can see how the force acts instantaneously, or synchronously. The other method expresses positions in Euclidean 3-space (events in spacetime) as a triplet of directions instead of a triplet of lengths. When anything changes in this model, then all the directions specifying every other position or event in the laboratory frame must also change in a synchronous manner, both mathematically and conceptually. When you look at the illustration from nist, the diagram contains a derived quantity in the lower right hand corner that they call a radian, which is the unit of a plane angle. The correct representation would place the radian as the second SI base unit, and it would have its own dimension. The way it's currently done, the radian is the cheater’s way of representing a turn. A turn in Euclidean 3-space is different than simply a ratio between two lengths. The same problem exists with representing volume as a product of three lengths. This assumes that the three directions are “orthogonal” to one another. What does that even mean without a base quantity of direction? Orthogonal, but without a unit of direction? That’s the non-sense that I see. Maybe you understand this question better than I do, but I don't think that's the case here. I see things much too clearly to accept your argument. I know we disagree, but nothing that I've ever claimed to be true is in any conflict at all with state-of-the-art accepted scientific theory. As a matter of fact, it answers some questions that have been around for a long time.

And somehow the particles know how to determine what least action is, and then they know to follow this principle. The question seems to be "by what mechanism" does this happen?

I imagine the question in the OP could easily be restated to ask how all the particles in the universe "know" that they are supposed to expand. On-the-nose from my understanding of the OP.

My current understanding is that there will be one unique case in any reference frame where this relationship occurs. The triplet x,y,z can be ordered in any of six different ways and everything will still commute. When we add the time coordinate it doesn't commute. This is why I understand this relationship to be unique. If I'm wrong, I'm sure someone will correct me. If I'm right, I'm sure someone will still correct me. When I say that the coordinates commute, I mean that both length and direction commute. The problem with the two-dimensional ruler example is that in two dimensions length always commutes and direction is always its inverse. And that's exactly how we deal with it mathematically. The coordinate system that contains the x,y,z triplet has some previously unknown relationships that don't exist in two dimensions. All of the normal two-dimensional geometric rules still exist, only they must exist side-by-side with another three-dimensional set of geometric rules. Adding time to the manifold establishes constraints on this ability to commute, possibly due to the fact that time is divided into the past, now, and the future. All of the events that share the same now must have a geometric relationship to one another that is in addition to a common time coordinate. Also, everything that the skeptics are saying about me seems to be true, except for the parts where they claim that the math isn't the math. I honestly don't think they understand the math.

That is exactly my point. They are different things. But they do relate to one another in a spatial sense. Since both cases comprise Euclidean 3-space they each have a volumetric relationship with one another. Do you know what this relationship is? There hasn't been any response to my question. Do you understand the math that I'm talking about? If you don't understand, why not?

Look, there isn't any dispute that the corners of the cube are events in spacetime while at the same time they are positions in our reference frame. All that I'm talking about is the math that connects these things to one another. Have you seen the math? Do you understand the math? If the math is incorrect then, yes, the math is rubbish. If it's correct then it's simply math. I don't understand the hatred for math.

There is a set of directions in the local coordinate system, just as there is a set of lengths. We seem to pretend to understand the length contraction part by ignoring any questions about the direction part of the system. The two must jibe with one another. Anything that affects length will also affect direction. So the question is "how do all of the directions in the local coordinated system remain coherent when we throw length contraction into the mix?" This question can't really be answered by simply choosing between measuring or seeing. The effect of length contraction is a real effect caused by the requirement that spacetime has to be isotropic and time moves only in one direction.

What do you think happens to the cube when it undergoes length contraction? Do the corners remain at 45° referenced from the center? This question does have a correct answer.

I’m not convinced that this is the correct approach to looking at the question. Making this a question that involves only lengths doesn’t fully express the problem. Our official forum position is that spacetime is simply a volume with time added as an additional coordinate. I don’t think that anyone has much skepticism about this position being correct. The controversy is over the meaning of volume in this definition. What you are attempting with your experiment is to decouple the three dimensions that make up a volume into some two-dimensional components. I think this is intended to simplify the question, but is has a fatal flaw. Some of the characteristics of Euclidean 3-space cannot exist in a two-dimensional model. In other words, volume has some properties that are more than what can be attained by simply arranging three planes orthogonally. The two-dimensional question that you’re posing isn’t a valid one (except perhaps in some mathematical constructs that do not model nature.) This question is a better one because the cube represents the three-dimensional space of spacetime. Instead of looking at the edges, it may be better to focus on the corners. Each corner is a direction and distance from the center of the cube. The direction is JUST AS IMPORTANT as the distance. We tend to ignore this fundamental certainty, most likely because of the way we do the math. But in order for Euclidean 3-space to hold together, direction and length are inextricably interconnected in a unique way. This is a trick question. The correct answer is that she will not exist in our frame when she passes perpendicular to her direction of travel. That’s how spacetime wraps back on itself. Our notions about transverse Doppler only consider the distance aspect of the question. We need to consider the direction aspect, too.

Geometry exists. It's a description of something. That something must exist, at least in theory.

I understand how this is done, but how can we be sure that the volume of space is really nothing more than the combination of the three lengths, x, y, and z? How can we be sure that volume isn't something else entirely, for instance length and direction? edited to add>>> If we made up a new unit called a cubradian or cr and made this unit equal to the quantity of volume that exists between the center of a sphere and a steradian. One cubradian would be [latex] \frac{1}{4\pi}[/latex] volume of sphere then couldn't we specify volume by saying an angle times a distance? We could say approximately [latex] {.27} crm[/latex] rather than [latex] 1 m^3[/latex]. Is there anything wrong with that? (other than the fact that no one seems to like the question?)