sethoflagos

My point exactly. +1

I'm really quite envious of your expertise in this. I'm afraid that when I get into a topic that grabs my interest, I go into full focus mode and any considerations of not treading on other people's toes go out of the window. I've known my employer for over a quarter of a century and we get on very well. He says that when challenged my responses can be what he calls 'a little waspish'. We reconcile this with the fact that I'm a northerner and he's a southerner. It's just a clash of culture. Nothing personal.

For what it's worth, although there's some considerable overlap in our areas of interest, I've learnt to think very carefully before I post anything that may contradict something you've posted. Hey, none of us are perfect! But if we work together as a team and respect each other's core disciplines we have a chance of getting close to a viable conclusion.

I really like this! The ultimate goal of an education system is to teach you everything there is to know about nothing! Yes. it's a reductio ad absurdum but it's also very funny.

This sounds highly inferential. How would it work for say a Dutch poster who may not catch English nuances accurately and was culturally conditioned to respond in blunt fashion?

Some of you familiar with my posting style probably realise that my understanding of psychology is close to zero. Perhaps you can help raise it a notch or two. I'm sure all of us at some point or other have read a post that exposed our ignorance. @Mordred and @Markus Hanke for example frequently leave me feeling exposed as an idiot without seeming to pause for breath. But I do exit that thread a little less of an idiot. So why do a number of us seem to automatically assume that such exposure of our ignorance was intentional and malicious? No names no pack drill. Just curious.

It's quite reasonable I think to say something along the lines of 'the climate gets cooler as winter draws near'. But when we try to express such ideas in physics, we run into the conventional understanding that the total energy content of a material is always positive. At a fundamental level, if you accepted the concept of negative total energy, you would de facto be accepting the concept of negative mass. This is not observed.

I think it all balances up but I'd be grateful if someone checked it over.

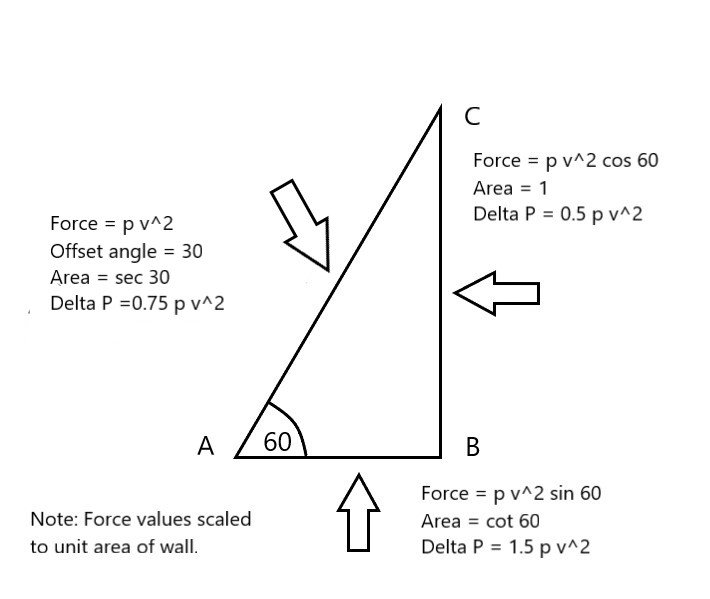
Just to tidy up, I forgot to adjust the ground load for the reduced area of ground relative to wall, so that figure is wrong. It needs to be divided by cot (60) (ie multiplied by 30.5). I make the triangle of forces to be as shown in the sketch:

It's many years since I used these methods. There are many hits for Runge-Kutta on the internet but I've no idea which would be best for you. I think the appropriate form to use is RK4. Maybe others can recommend an appropriate text book. It might help if you indicated your preferred language and level of maths education.

I think I've got this now into a form that doesn't involve some non-physical model. Deflecting the airstream upwards requires, as shown above, a force with h,v components of -p v2 (1 - cosA), p v2 sinA By Newton's 3rd Law that generates an equal and opposite reaction force of p v2 (1 - cosA), - p v2 sinA If we can reflect this reaction force back in the direction it came it will become its own source. I picture the means of transmission as a pressure wave (there are other valid pictures) To achieve the reflection necessary for a stable, steady-state system the wave must reflect off both the ground and the wall. It doesn't matter in which order but if say part of the wave 'bounces' over the wall, or strikes the ground ahead of the air deflection zone the momentum leakage will work to alter the angle of deflection of the airflow. A little study of the geometry shows that the wave path between ground and wall must be parallel to the deflected airstream ie This angle is self-maintaining as discussed above. As the wind picks up from still conditions (no air ramp), the growing pile up of air against the wall will naturally and very quickly construct its own 600 ramp and maintain it in dynamic equilibrium.

Does anyone under fifty understand 'punchcards', 'analogue computer', 'relay ladder logic', 'assembly language', 'ward leonard drive', 'pentode', 'cathode ray tube', even 'carburretor' and 'distributor'? All the stuff I must have spent years trying to get my head around when I was younger. Do you need someone who does? 😉

Okay, let's have another go at this, but taken in short (hopefully) simple steps. What do we know with certainty? We know the incoming wind gets over the wall in its entirity, there is no mass transfer in or out. We know that it gets over the wall very quickly (no time for meaningful heat transfer) and no external work is done on it. Therefore there is negligible energy exchange with the environment. By some means the incoming flow horizontal flow is deflected upwards at an angle we can call A. By conservation of energy horizontal velocity becomes vx = v cosA, and vertical velocity vy = v sinA By conservation of momentum the rate of change of momentum with the ground per unit area = p v2 sinA Ditto the rate of change of momentum with the wall per unit area = p v2 (1 - cosA) By conservation of energy (I think, help me out here), it is necessary that (1-cosA) = cosA hence A = pi/3 radians (60o) Pwall - P0 = 0.500 p v2 Pground - P0 = 0.866 p v2

The indo-european root of 'black' meant 'burn, gleam, flash etc', and also gave us the French word for 'white'

Have a look at http://star-www.dur.ac.uk/~tt/MSc/Lecture2.pdf It recommends Runge-Kutta for accuracy.

Where?

So my posts were 'wrong' because I failed to address all the safety factors the building codes include to cover for topological features such as wind funnelling? You'll notice that the expression for dynamic pressure is the same as the one I gave calculated at a standard air density @ 15 0C of 1.226 kg/m3. This standard doesn't appear to think the equation I gave is wrong. Unlike some other codes, this one factors the safety margin into the tabulated design wind speeds rather than use a crude drag coefficient. Ask yourself why the engineering codes use the incompressible form of the Bernoulli equation for a fluid that is demonstrably compressible. Noting that we can rearrange it in the form P1/P0 = 1 + p0 v02 / 2 P0 We can compare with the compressible form: P1/P0 = (1 + (k-1) / k . p0 v02 / 2 P0)^(k /(k-1)) So are the engineering protocols 'wrong' by your exactling standards? Or are they just simpler and conservative. Engineers are usually ok with that. Sorry for having to point out the fundamental flaws in your thinking earlier in the thread. I did try to do it as sensitively as possible - by addressing @joiguswho can handle differences of opinion in an adult manner. But you spat your dummy out anyway. You owe me and the other contributors to this thread an apology - but I don't suppose in a million years that we'll ever get one.

Let's let this thread die a well deserved death. The well has been poisoned by those unable to yield ground in their turf wars.

= Kinetically Imparted Common Knowledge

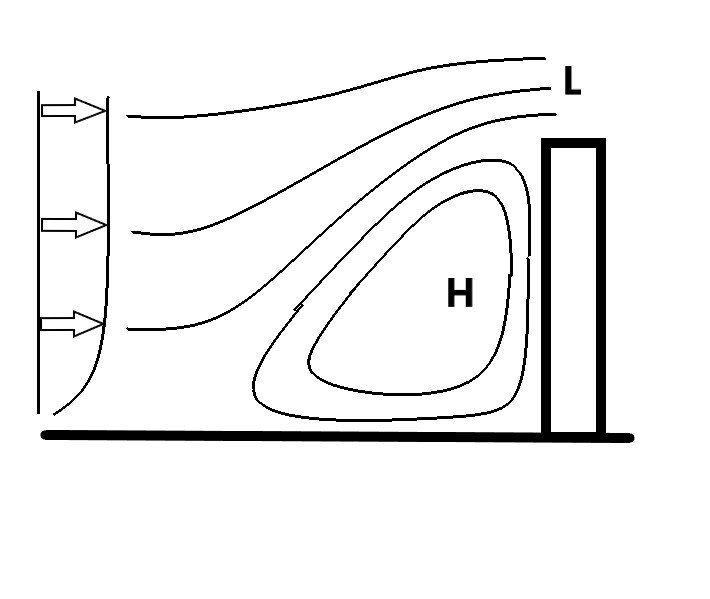
Firstly a word about deriving the result from Newton's second law. Maybe you missed the details of my earlier post: Please read the first calculation line carefully. I begin with rate of change of momentum, just as @swansont recommends, but since I can't integrate that directly I use the chain rule to recast the ODE into a simple form I can integrate. This gives the correct answer. Attempting crude numerical integration of the raw momentum equation via left Riemann sum as we saw yesterday gave a 100% overestimate, to which I suggested you try the middle Riemann sum. You are right to maintain some doubt over the validity of this procedure. It gives the correct solution but only by accident. You're working with at least one assumption that is profoundly unphysical. I made a little explanatory sketch of how I see air flow over a wall. (A small one - let's keep it simple) Interactions between the wind and wall are mediated by a clockwise rotating prism of air that acts as a ramp to accelerate the incoming airstream up and over the wall. It is clear that the main flow retains much of its horizontal momentum throughout - it doesn't dump it all into wall in fact it mustn't (continuity). But it isn't total momentum that is in the equation - it's rate of change of momentum that counts. So what happens inside the prism. Wind shear transfers momentum into the angled face accelerating it up to near bulk velocity. And that momentum is transferred via an exchange of angular momentum with a flow parallel to the wall rather than linear momentum from direct impingement. A similar effect happens on the ground providing the necessary reaction to generate upwards momentum. Almost none of the main flow gets anywhere near the wall. Anyway, this model works for me and is pretty close to what I've seen from wind-tunnel tests of blast walls and the like. Hope it helps someone out there, though I suspect the OP is long gone after yesterday's sorry show.

I'm sorry Josh, I missed the last bit. I took a little time out from the dog-piling to check my facts. I read the OP as 'What is the stagnation pressure acting on a wall normal to a headwind'. The response I gave is simply textbook fluid mechanics and is correct given the stated assumptions. I find it strange for once being called on to defend the Bernoulli equation, but then lots of things seem strange to me these days. Both viewpoints expressed comply with conservation of momentum. The key difference is that I reduce momentum incrementally into a dynamic pressure field while others drop all the incoming momentum at the face of the wall. Now I have an easy way of propelling the fluid up and over the wall: it's accelerated up and away by the pressure field built with the momentum of the incoming stream. Others seem to have stationary gas stuck to the wall and no source of energy to move it away. I look forward to some imaginative explanations.

The calculations you presented specifically state 'The jet strikes the plate and does not rebound but spreads sideways over the surface of the plate. The momentum normal to the plate is destroyed.' Sounds pretty inelastic to me. It seems quite credible. Just inappropriate to the OP. In particular there is no mention of the jet being slowed by an adverse pressure gradient prior to impact. In fact no mention of pressure at all. Which at least then avoids having to explain away the infinite pressure necessary to bring a jet to a halt instantaneously. I provided my own simplified calculation of 'the force of the wind' some dozen or so posts back. Perhaps you could tell me where exactly the mistake is?

What a very curious idea. Why exactly are inelastic impacts relevant to the discussion?

No worries. Just adjust the first line of your calc to dL = (v/2) dt and I think everything else clicks into place.

Of course Bernoulli is an energy equation - that's what you get when you integrate a force balance. If kinetic energy is conserved as you say, then where does the work necessary to compress the air come from? These things do happen very quickly but they are not instantaneous. The momentum exchange does not occur at constant velocity v. It occurs gradually over the velocity range v to zero. On average that's v/2. This is why your results are double what they should be. You need to integrate over a valid path.