Feynmanfan85

@swansont As the frequency of a photon increases, the number of wavefronts within the photon increases. The energy of a photon is in my model NE_0 = hf, where N is the number of wavefronts within the photon, and E_0 is the minimum quantized energy. As f increases, N increases. It's that simple. As a general matter, I really suggest you simply read the paper. The idea that I'm going to write a 60 page paper that's inconsistent with something obvious like Planck's equation is just not realistic. And I'm guessing by now you realize I'm not some crank. I have a significant investment in my professional reputation, and this stuff is not written anonymously. My model is consistent with every single test of special relativity that I am aware of. It's consistent with (though sometimes differs slightly from) every equation within special relativity that I'm aware of. The areas that I do not address are electrostatic charge, magnetism, and QM. I'm not saying these aren't important, they're obviously incredibly important. And perhaps my model runs afoul of something in these areas. But everything else is covered.

@swansont No no, not at all. Planck's equation is the root of my model of light, and matter in general. Think about what the equation says: energy is equal to a frequency multiplied by a constant. In layman's terms, energy is equal to the number of times something happens per second, multiplied by a constant. I take that concept and run with it, and say that what's actually happening in that equation is that the energy of a photon is literally the result of a frequency - the number of times a wavefront crashes into an atom per second, where each wavefront carries the same, discrete amount of energy,

My model treats light, and all particles generally, as waves. A photon is in my model a discrete set of wavefronts in series. Put informally, it's a "horizontal particle" that consists of some finite number of wavefronts. The tighter the wavefronts are packed into the photon, the greater the frequency, and the greater the total energy of the photon (each wavefront carries an equal amount of energy). To analogize, if you were standing at a beach, and a series of 10 equally sized waves in row all hit you, and then no waves followed afterward, that would be similar to what happens when a photon hits an atom in my model. It's a discrete amount of energy, but it's not all delivered at once, but is instead delivered over a very short period of time. So if, as in your example, an atom absorbs a single 1 eV photon, it means that a finite series of wavefronts with a total energy of 1 eV have smashed into the atom. If that atom is moving, then it can still eject all of that energy, but not as a single photon. That is, it will eject all of the wavefronts from the incoming photon, but over a longer period of time than it would have if it were stationary. As a result, the incoming energy equals the outgoing energy, but the amount of time it takes for the outgoing energy to get ejected is longer than it would have been if the atom were stationary. As a result, the frequency of the outgoing photons is lower than the frequency of the incoming photon.

@swansont That is exactly right. Energy is conserved in all cases, it is just a consequence of the frequency with which light is incident upon the detector. If the source is moving towards the detector, in the direction of its light, then the wavefronts will be compressed, causing a Doppler shift up in frequency. This doesn't change the amount of energy ejected by the source over any interval of time, it simply changes the rate at which energy is incident upon the detector (in my model each wavefront carries the energy of a lightwave). If the detector is moving towards the source, the same reasoning applies. That is, since the relative velocity of light is not fixed in my model, a detector that is moving towards the source will get hit by wavefronts at a faster rate, causing an increase in the observed frequency. This is consistent with the classical doppler effect, and therefore, my model makes use of the classical Doppler equations, even in the case of light waves. However, my model also implies that time-dilation will occur both in a moving source, and a moving detector. In the case of a moving source, the rate at which energy is ejected by the source will be decreased. That is, if the source ejects E Joules of light energy per 1 second when stationary, then it will take (1 + s) seconds for the source to eject E joules when moving, where s is the amount of time-dilation that corresponds to its velocity. The result is that the frequency of light ejected by the source is downshifted. A moving detector will also experience time-dilation, and the analysis is similar to a moving source. In the case of a moving detector, the rate at which energy hits the detector is obviously unaffected by time-dilation, since the motion of the detector has absolutely no effect on the source. However, I assume that the rate at which energy is exchanged by the atoms within the detector is affected by time-dilation, in the same way a moving source is affected. That is, it takes longer for the energy that hits the surface of the detector to make its way through the detector, since the atoms within the detector exchange less energy per unit of time, ultimately resulting in a lower observed frequency.

It is not the alphabetical characters that purportedly follow your name that concern me, it is substance that is missing from your words. You obviously lied about reading my paper. Why bother responding, just go do something else.

@Mordred To quote you: Yet you also say, I address both of these topics, so you obviously did not read my paper. In fact, the relativistic Doppler shift is addressed in the same section as the general Doppler shift. I've tolerated your uninformed belligerence, but going forward, I'm not going to respond unless you have something of substance to say, written in some comprehensible human language, and not the gibberish you've been spouting so far. That uninformed, anonymous people like you are allowed to pretend to be experts on anything at all is a serious problem for the dissemination of information online, because unlike me, most people would probably take you seriously.

@Strange There is a specific set of criteria that have been developed for testing Lorentz violations: https://en.wikipedia.org/wiki/Standard-Model_Extension I am saying that testing my model is much simpler, and requires only that we move a detector towards a light source. This is not a sophisticated experiment. If SR's predictions for the observed frequency are closer to the actual observed frequency than my model's predictions, then I'm probably wrong. However, this experiment has never been performed (to my knowledge) to the accuracy required to test my model. Your "10-35?" has no units, so I can't answer that question. For substantial velocities, and substantial frequencies of light, the difference between my model and SR is on the order of 10-6eV in the case of a Doppler shift. That is the range of accuracy that is required to test my model, which is certainly doable given current technology. @MigL @Mordred In Newtonian, and Relativistic physics, the quantity that you can't measure is velocity. That is, if you're in a given inertial frame, you can't know your "true" velocity, since all motion is relative. This has been the assumption for a long time, and I am very aware that it predates even Newton. In my model, energy is the "hidden variable" that generally cannot be measured. In contrast, I propose a method for experimentally measuring absolute velocity, by measuring the velocity of light, that makes use of time-dilation. This is set forth in Section 5 of my paper. Also, note that your statement that energy is "measurably" frame dependent is only true because you are using equations that assume energy is a particular function of velocity or heat. My equation relating energy and velocity is very abstract, and allows for different particles to have different relationships between their energies and their velocities, all using a single equation. For example, the velocity of a photon is fixed, regardless of its energy. This is generally not the case for most particles in the standard model. @studiot Apologies, I'm getting a lot of "dings" on this thread. What question would you like me to address? @Mordred Not in my model, it's simply dependent upon the observed frequency of light. If you're going fast towards a source, you will objectively experience a shorter amount of time between wavefronts. This is simply common sense prevailing over philosophy. If waves come in towards a beach once every second, and you run out into the water, towards the waves, you will get hit with wavefronts at a frequency that is greater than once per second. My model takes this same, common sense approach to light waves, and generates equations that are nearly identical to SR. I would suggest you just read Section 3.5 of my paper, as it is only a 5 or 6 pages long, and contains the bulk of the information that relates to your questions.

@Strange Again, I propose two experiments which would test all of these things, one of which would be very easy to perform in a reasonably well equipped lab. It is not necessarily Lorentz invariance that needs to be tested, but simple, direct measurements of photon frequencies. The differences from SR would be very, very small, and as far as I'm aware, well beyond the precision of any experiment that has been conducted to date. Also, my model is consistent with every experiment testing SR that I have come across, since the predicted differences are incredibly small. If you have a particular experiment in mind that you think disproves my model, please feel free to share it.

@Strange This is just a consequence of velocity being generally presumed to be relative, a topic I deal with at length in my paper. This has nothing to do with energy itself, but is instead a consequence of the formulas for energy in Newtonian and relativistic mechanics. As noted above, my model is more general, and does not need to make use of the same equations for energy. Also, motion is absolute in my model, not relative, and energy and information are similarly absolute, and not relative.

Hi @MigL Information is actually a conserved quantity in my model as well. And I present a theory of time-dilation due to gravity that is consistent with the general theory of relativity. However, my model of gravity is due to quantized "chunks" of energy interacting with systems. I think of my model as a potential "bridge" between SR, GR and QM, and if you have expertise in QM, I would greatly appreciate your insight. I'm not sure why energy or information has to be made frame dependent, so if you could expand upon that a bit I think it would be helpful Also, though I understand your initial skepticism, I think by actually reading the paper you'd find that assuming that energy is a substance explains quite a bit, though it may seem odd initially. @swansont I think this is the core of our misunderstanding: In my model, energy is a substance that contains information, it doesn't simply exist on its own. It's analogous to a unit of memory that contains information. Each "chunk" of energy is like a tiny writable disk. The properties coded for in the energy of a particle characterize the properties of the particle overall. So for example, in an electron, there will be some number of "chunks" of energy, each of which contain the information that characterizes the overall properties of the electron. This means that the chunks of energy within an electron will collectively code for charge, causing the electron to have charge. In that sense, all other properties are in fact properties of energy, but not in the traditional sense. Rather, these properties exist because the information contained in the energy of a particle causes these properties to manifest. If we "flip the switches" on the codes within an electron, it could turn into some other particle altogether. This is how my model views particle decay, photon photon collisions, and electron positron annihilations - in each case, the codes within the energy contained in a particle changes, causing the properties of the particle to change. It's like rewriting the information on a disk - the program / particle will behave differently, and have different properties, after its code is changed. Another analogy is DNA: the energy of a particle is its DNA. If you change the DNA, you change the particle. The amount of energy in a particle is, in my model, really just a measure of the amount of information it contains. Time-dilation in the case of particle decay occurs because it becomes less likely that these codes change as a particle gains kinetic energy. This happens because the particle's kinetic energy, which codes for motion, uses up the particle's "bandwidth", slowing down the processing of the particle's own information (which is contained in its mass). That's if we use the relativistic momentum - I just meant that the two formulas become consistent if you swap v with c, using the classical momentum of a particle. I'd state the formula from my paper, but it will look like nonsense without reading the rest of the paper. The gist is there's some minimum quantized energy in my model, just like there's a minimum quantized charge. Let's call this minimum energy E_0. If m is the mass of an electron, then it's mass energy is E = mc2. Assume the electron is stationary, with a kinetic energy of zero (motion is not relative in my model, so this means it literally has no kinetic energy). The number chunks of energy in my model is simply E/E_0. That is, you take the mass energy, and you divide by the minimum energy, and that gives you the number of chunks. Since each chunk of energy contains the same amount of information, the amount of information contained in an electron is given by M E/E_0, where M is a constant I discuss in my paper. This is the amount of information you need to "produce" an electron, generating a particle with the right mass, charge, spin, etc.

I think I see your point of concern, and the answer is yes: I know enough to not discuss the topic. My model uses information theory, just like statistical mechanics uses information theory, but the results are completely different, and flow from a completely different set of axioms. I don't rely on any precarious results in information theory that have ambiguous physical meaning. I use very basic, very concrete concepts, like codes, bits, complexity, etc, and use these concepts to build a combinatorial model of elementary particles. The similarities to statistical mechanics are in my mind superficial. That said, there could be deeper connections, but they're not necessary to achieving the main result of the paper, which is time-dilation. The concepts in my paper amount to an alternative to special relativity. If my model is a problem for statistical mechanics, then special relativity should be as well. That said, if you have an expertise in statistical mechanics, I would welcome your input.

Maxwell's equations are expressed in generic units of mass, time, and length. Using kg, seconds, and meters should not impact his analysis, so long as those units are used consistently. My question is, his analysis, making use of mass, time, and length, is that analysis viewed as incorrect or deficient? If not, then we necessarily have a connection between energy and magnetic pole strength, which I found intriguing. I am open to the possibility that his analysis is dated, which is really the question I am posing.

My background is in discrete math, not physics, which is why I ask these questions. I think my claims are limited to claims contained in reputable sources, other than my original research, which I think is well-reasoned, but I am of course open to criticism and suggestions. I am certainly not claiming to be some kind of king, but I am a published mathematician in the subjects of graph theory and information theory, so yes, I think I understand these topics quite well. I am aware of the connections between information theory and statistical mechanics. However, the point of my research is to show that information theory can be used to produce time-dilation. That said, I think someone with a background in statistical mechanics would likely find my work interesting, and will probably make connections that I have not made, that are unrelated to time-dilation.

Yes, thanks, I'm aware of the modern formulation. I was really more interested in the dimensional analysis Maxwell put forward. Working with his units, we obtain a relationship between magnetic strength and energy that I thought was interesting, and was wondering if anyone has carried that analysis forward. Do people view Maxwell's units as wrong, or deficient? Or have they simply fallen out of favor? For some reason I didn't get a notice about your response, apologies for the delay in responding.

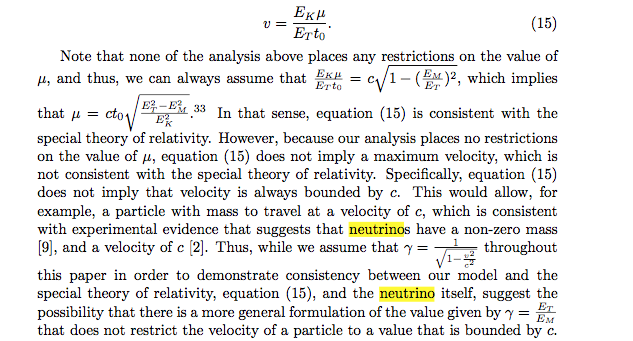
@Mordred As I noted above, my model is more general than SR, so a neutrino can have mass, and a velocity of c, as gamma = ET / EM, without any further restrictions on the value of gamma. Here is the section of my paper that addresses this topic, where v is the formula for the velocity of a particle.