-
Posts
569 -
Joined
-
Last visited
-
Days Won
1
Content Type
Profiles
Forums
Events
Everything posted by Kartazion
-
How can you know before I publish it? Precisely. It is all the interest of the gravitational potential energy of the mass to be above a gravitational attraction. Einstein gravitation appears to be affected by the Penrose-Hawking theorem, stating that if the dominant energy condition holds then the high density gravitationally-induced formation of singularities is inevitable; in turn this means that the avoidance of singularity formation due to gravity can be done only by invalidating the condition on the energy... Just the same, Higgs physics can change the energy tensor in its structure, so to make the dominant energy condition invalid, and therefore to have the gravitationally-induced singularity avoided. 1901.05295.pdf (arxiv.org) A few basics on Lagrangians will be enough: L = T(kinetic) − V(potential) I have already published: A tale of two disciplines. Physics can be a source of inspiration, with theoretical concepts such as general relativity and quantum theory providing an impetus for mathematicians to develop new tools. https://penntoday.upenn.edu/news/where-math-meets-physics PostScript: I do have an account on arXiv. I know it takes a lot of corrections, but we must get an endorsement from another user to submit an article to category physics. Can any of you sponsor me? I just wish publishing the basis of the gravitational oscillator adding to gravitational singularity avoidance. Thanks.
-
Yes swansont, physics of the zero-point field = implications for inertia, gravitation and mass. The term zero-point energy (ZPE) is sometimes used interchangeably with it are the terms zero-point radiation and ground state energy. The term zero-point field (ZPF) can be used when referring to a specific vacuum field. Specific vacuum? Possible vacuum? This is exactly what the title of the OP is about. So? And so on, and so on... Are you going to close this thread now? As usual ?
-
And? It is a link among the other published references. On the one hand you say that the center of the earth is not a black hole, and then you say that I am not talking about it. Yes ... No ... Maybe ... I don't answer the rest cause I know you and you never gonna understand. It is for the continuity of kinetic energy after x=0. Correct. The same singularity that Einstein talks about. "Einstein gravitation is known to give rise to the formation of singularities at high densities unless the dominant energy condition is made invalid by the occurrence of new physics: we show that such a new physics can be the already present Higgs sector of the standard model of particle physics." https://arxiv.org/abs/1901.05295 Yes, the sombrero potential is the sombrero potential and the singularity is the singularity. Gibberish? ... Yes ... No ... Maybe ... Physics of the zero-point field: implications for inertia, gravitation and mass Physics of the zero-point field: implications for inertia, gravitation and mass | SpringerLink
-
Supermassive black hole - Big bang For balance. After rotating up to 180° it is from the south to the north antiparticle. The ZPE corresponds to the inertia of the mass. Either orbit. The perturbation is the motion of inertia of orbit. It is the goal to be able to explain it in physics 101. I'm working on it. Postscripts:
-
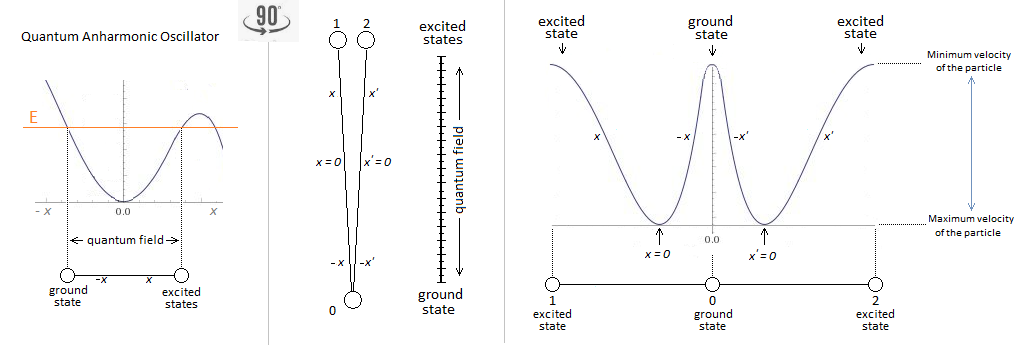
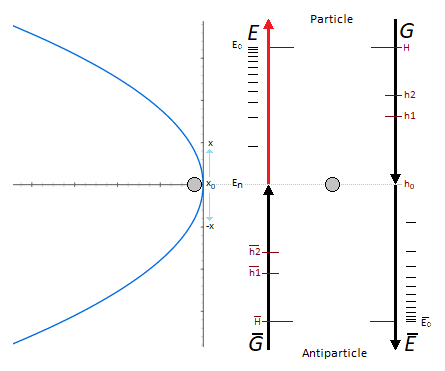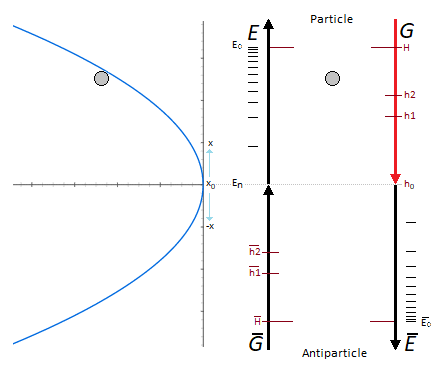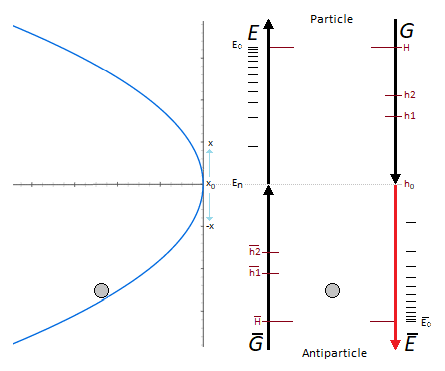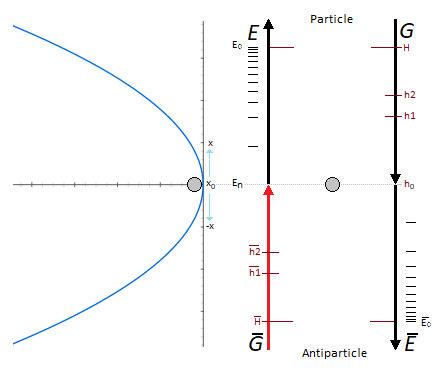
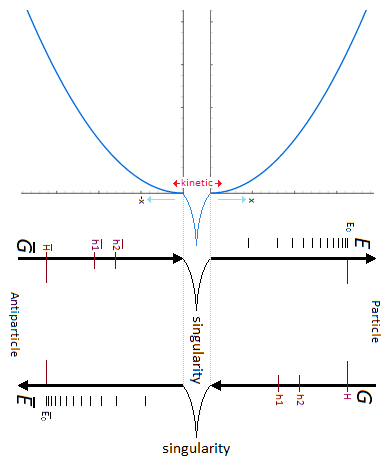
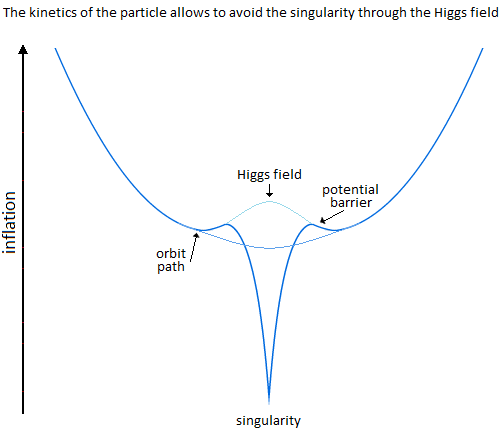
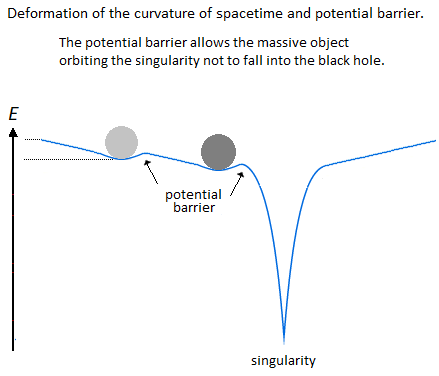
1 - The gravitational oscillator (Hole through the center of the Earth - http://hyperphysics.phy-astr.gsu.edu/hbase/Mechanics/earthole.html) gravitational potential energy - kinetic energy = 0 "If you drilled a hole through the axis of the Earth from pole to pole, and put a long thin vacuum chamber in it then dropped an object into one end of that chamber, it would fall down the hole, picking up speed. And it would be moving very fast when it reached the centre of the Earth so it would carry on going until it reached the other pole where it would stop, and then fall back down again. It would "bounce" back and to. If the density of the Earth was constant (rather than increasing as you go down) the body would exhibit simple harmonic motion." (Author - Bored chemist) 2 - The singularity avoidance At x = 0 when the particle is going faster (don't rely on GIF for speed), its kinetic energy allows it not to fall into the singularity. Kinetics of the particle at the bottom of the potential well, and avoidance of the singularity: I must specify that the avoidance of the sigularity, by the kinetics of the particle at the bottom of the potential well, also occurs when the particle is at rest in the false vacuum, namely the ZPE; And which corresponds to the same celestial mechanics of the orbit of the planets around the star. In other words when the particle is at rest at the bottom of the well and it undergoes the ZPE disturbance of the false vacuum, then we understand that the particle orbiting around the gravitational singularity, rather than a vibratory disturbance. Indeed for an observer the reproduction of the path of the particle is expressed by a sinusoidal signal in time or elliptical by its magnitude. True vacuum is total collapse. 3 - The Higgs field The kinetics of the particle make it possible to avoid the singularity through the Higgs field. The Higgs field corresponds to the path taken by the particle. In other words, the Higgs field corresponds to the path taken by the particle thanks to the kinetic energy and makes it possible to avoid the singularity. If the kinetic energy of the particle is sufficient and if the range of the energy condition allows to pass the potential barrier the singularity avoidance occurs, but during the attenuation of the kinetics of the particle, this causes by the quantity of lower energy to fall towards the singularity and to reach the true vacuum. In conclusion, the metastability of the vacuum is shifted and is represented in three parts. The first corresponds to the false vacuum of the Higgs field at the level of Spontaneous Symmetry Breaking, follows in two the true vacuum of the Higgs field which is in fact the Zero Point Energy and is therefore not the true vacuum since in three we have the true absolute vacuum which corresponds to the total collapse. Without any kinetic energy the contour of the potential barrier corresponds to the path of the orbit of the particle in Zero Point Energy in relation to its inertia. Application of conventional physics. Namely the kinetic energy of the particle for the harmonic or anharmonic oscillator, and inertia to simulate the orbiting motion of the particle or mass around a more massive object. According to the oscillator model that I propose, the orbit(s) is located at approximately at x=0 in the potential well of the oscillator, and corresponds to the Zero Point Energy. The initial Zero Point Energy disturbance (ZPE) corresponds to the movement of the particle located in the false vacuum in orbit around the gravitational singularity. The idea now is that the inertia of an orbiting body would correspond to the movement of its mass occurring by the force of gravity, but by an avoidance of the gravitational singularity thanks to the barrier of potential. In other words the particle slides along the barrier of potential and corresponds to the motion of inertia following the orbit in relation to the object with the greatest gravity at the center of the system. Indeed in the conventional illustration, the orbit is the closed curve representing the trajectory that a celestial object draws under the effect of gravitation and inertial forces. It should therefore be remembered that the own deformation by sinking of the celestial object in the curvature of spacetime creates all around it a barrier of energy potential from higher edges. The Higgs field and potential are also used well to be able to represent the metastability of the universe, as well as the quantum particle. Being able to make the link between GR and QM through the gravitational oscillator by explaining the Higgs mechanism becomes very interesting, even important. If we were to make the jump of a massive object over the potential barrier and the gravitational singularity, then we would draw through the particle, the curve of the Higgs field. It is therefore understood that in order to do this, the kinetic energy must be accordingly. We would therefore tend to believe that for a quantum particle that the step of crossing a black hole would become easier. I guess that its avoidance is related to the electromagnetic force. In a more speculative definition and given our knowledge of the gravitational sigularity followed by the time dilation, we could associate the center of the earth as such. Indeed for the quantum particle it becomes easier to imagine the avoidance of the singularity at the level of the terrestrial core through the gravitational oscillator. 4 - References: Black Hole singularity avoidance by the Higgs scalar field Einstein gravitation is known to give rise to the formation of singularities at high densities unless the dominant energy condition is made invalid by the occurrence of new physics: we show that such a new physics can be the already present Higgs sector of the standard model of particle physics. https://arxiv.org/abs/1901.05295 Black holes and Higgs stability We study the effect of primordial black holes on the classical rate of nucleation of AdS regions within the standard electroweak vacuum. We find that the energy barrier for transitions to the new vacuum, which characterizes the exponential suppression of the nucleation rate, can be reduced significantly in the black-hole background. A precise analysis is required in order to determine whether the the existence of primordial black holes is compatible with the form of the Higgs potential at high temperature or density in the Standard Model or its extensions. https://arxiv.org/abs/1606.04018 Unification of gravity and the harmonic oscillator on a quantum black hole horizon II: Perturbative particle scattering and Feynman amplitudes n Article I, a harmonic-oscillator model of a universe of n quarks is infinitesimally modified to eliminate the background reference frame. As a result, quark trajectories exhibit the unification of gravity and the harmonic oscillator near the horizon of a quantum black hole, a region that is approximately flat in space-time. Constituent quarks are confined to composite particles by cluster decomposition rather than a binding force. Here, the composite-particles are input for a perturbation model of particle-exchange interactions. https://arxiv.org/abs/hep-th/0307136 Gravitational and harmonic oscillator potentials on surfaces of revolution In this paper, we consider the motion of a particle on a surface of revolution under the influence of a central force field. We prove that there are at most two analytic central potentials for which all the bounded, nonsingular orbits are closed and that there are exactly two on some surfaces with constant Gaussian curvature. The two potentials leading to closed orbits are suitable generalizations of the gravitational and harmonic oscillator potential. We also show that there could be surfaces admitting only one potential that leads to closed orbits. In this case, the potential is a generalized harmonic oscillator. In the special case of surfaces of revolution with constant Gaussian curvature, we prove a generalization of the well-known Bertrand theorem. https://arxiv.org/abs/1305.3930
-

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
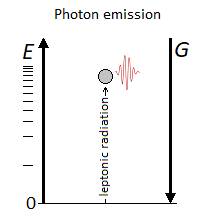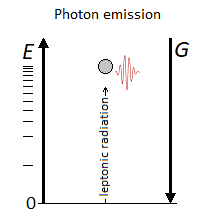
Yes that's right. But when the particle falls it becomes neutrino. The generation of the photon is done with the oscillation of the electron naturally in atom. Or the goal is to be able to plot the wave function with the paricle. The electric charge of the electron is used to generate EM field and becomes a neutrino. -

Particle Gravitational Oscillator & Wave Function
Kartazion replied to Kartazion's topic in Speculations
Hyperfine structure - Wikipedia The hyperfine structure gives the height, or the amplitude. The oscillation of the electron in the atom creates a photon of real shape in terms of waveform. The oscillation of the electron takes place with the Bohr radius from the center of the atom, to the electronic shells. -
Here I will develop how to plot a wave function with an oscillator. At first it is easy to understand that the oscillating particle traces this wave function. We can see that there are two types of wave function to create. The first is the simple plot of a non-sinusoidal x(t) type curve with the particle. The second uses an energy ratio plot. We can use the levels of the hyperfine structure to determine the amplitude in relation to the implusion to be given of the particle.
-

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
I use energy rather than mass to be able to move the electron to c. But to start I give a simple approach. The prediction is about the constant collapse of matter. The quantum gravity named z would then make the particle fall from the surface, to then reappear thanks to the energy. As much as the constant g has an acceleration on a classical object, then z would be an acceleration constant of the quantum particle to reach the speed of light. First, we can determine the amount of energy it would take to move the electron to c $$m = 9,11*10^-31$$ $$v = c-1$$ $$Ez={\frac{1}{\sqrt{1-({\frac{v^2}{c^2}})}}} \ mc^2$$ For an electron, the energy to develop is around 12,235,220 times its resting energy to reach speed c . From there inovation allows and depending on the height of the electron of its energy surface, to fall at the speed of light, to then reappear on the surface. Gravitational potential energy of the electron: $$Eu=mgh$$ $$z=\frac{Ez}{mh}$$ z is the constant it takes for the electron to reach the center of the earth at the speed of light. h = 6370 km The generation of the EM field is done by the oscillation of the electron into the atom. This is where we understand that the EM field of the photon is indeed independent of the particle, and without being a particle, and as an argument that it is no mass. I created a related thread Particle Gravitational Oscillator & Wave Function - Speculations - Science Forums -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
An electron for example 9,1094 10-31 kg. Photon? I'm going to open a new thread about this. -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
We already realize within the scope of the oscillator that the plot of the phase x(t) is anharmonic and is no longer sinusoidal because of g. The strength of the impact determines the amplitude. But I would open another subject related to the wave function produced by the oscillator. On the other hand, do not hesitate to talk about the principle of uncertainty for a particle at rest for example. Unlike the classical gravitational oscillator which experiences an acceleration of 9.806 65 m s − ², the quantum gravitational oscillator uses a particle of mass m to move at the speed of light where g determine its energy level. -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
But mathematically it is possible. -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
-

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
@studiot @SergUpstart The problem is that you apply a rotation θ that it doesn't have to be. My oscillator oscillates vertically. -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
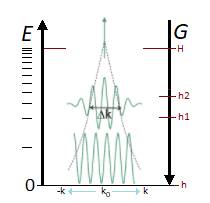
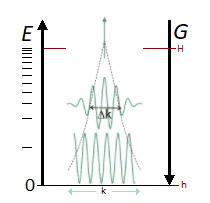
I did not understand the question. But ∆x corresponds to the width of the particle and the position of the particle is according to the path h, H and H, h. -

Particle Gravitational Oscillator & Heisenberg's Uncertainty Principle
Kartazion replied to Kartazion's topic in Speculations
1 mm is the size of the object. 100 mg. Coherent states are a similarity of state between the classical and quantum oscillator with which we can apply the uncertainty principle. $$\sigma x\ \sigma p\ =\sqrt{\frac{\hbar}{2m\omega}} \ \sqrt{\frac{\hbar m\omega}{2}} = \frac{\hbar}{2}$$ -
Here [1] is previously described a vertical oscillator of the particle following the vector of gravity. Indeed the energy is used to eject the particle of an impulse, and the particle uses the force of gravity to return to its point of origin. When the particle is at h at zero threshold and the impulse is given, then the momentum is high due to its high speed to almost zero momentum at H, then the particle falls back. This is what corresponds to the momentum seen from the uncertainty principle during the impulse, which is represented by k: I wish to continue with the Heisenberg's uncertainty principle for macroscopic objects of the order of millimeter. I recall the initial conditions that I wish: ∆x corresponds to the width of the particle at rest at h, that is E=0. For the moment we only have one exploitable interval height which is (H-h), H being the maximum height that the particle can reach. The particle therefore oscillates vertically from h to H and from H to h at a constant* width of ∆x. Also the impulse is constant and corresponds to the force of energy necessary to equal in terms of compensation the inverse speed of the classical gravitational acceleration (1 g = 9.806 65 m s − ²) ; Or quantum? Namely the gravitational wave at the speed of light. Everything is experienced in a vacuum. The units are meter, second, and kilogram. I now know that we have and for a particle of 1mm a scale of 10^-3 or 0.001 meter. $${\displaystyle \\ mv\ =\ \sqrt{2m^2g(H-h)}\ \\ v\ =\ \sqrt{2g(H-h)}\ \\ \\}$$ $${\displaystyle \\ \Delta v\ =\ \sqrt{2g(H-h2)}\ -\ \sqrt{2g(H-h1)} \\ \\ \Delta x\ =\ (x2-x1)\ = 10 ^- 3 \\ \\}$$ $${\displaystyle \\ \Delta x\Delta v\ \ {\ge } \ {\frac{\hbar}{2m}}}$$ * The question is and with the principle of uncertainty applied: will the particle oscillate transversely along x to give a value greater than ∆x if the height increases by speed? I recall that the particle travels its path from h to H and H to h having a width ∆x constant. But it is? I don't know if I correctly asked the question and the math problem. Thank you. [1] Reference
-
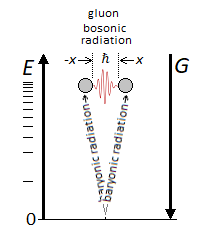
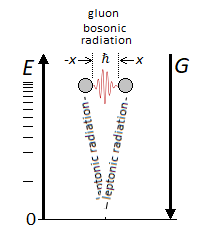
I made a naming mistake by using the word leptonic instead of baryonic for the previous diagram. Nothing contemptuous. If not that it should be emphasized. I therefore reiterate the message with the correct diagram: If the leptonic/baryonic matter is indeed radiative and vertical to gravity, then the bosons are only the difference in horizontal potential between two oscillations of the particle. It reminds me of the photon emitted by the oscillation of the electron. We understand that the particle collapses at any time thanks to gravity, and reappears thanks to energy. I take this opportunity to publish the leptonic oscillation with the following diagram: Vertical radiation = Fermionic matter = particle Horizontal radiation = Bosonic matter = wave I wish to continue with the Heisenberg's uncertainty principle for macroscopic objects of the order of millimeter. I recall the initial conditions that I wish: ∆x corresponds to the width of the particle at rest at h, that is E=0. For the moment we only have one exploitable interval height which is (H-h), H being the maximum height that the particle can reach. The particle therefore oscillates vertically from h to H and from H to h at a constant* width of ∆x. Also the impulse is constant and corresponds to the force of energy necessary to equal in terms of compensation the inverse speed of the classical gravitational acceleration (1 g = 9.806 65 m s − ²) ; Or quantum? Namely the gravitational wave at the speed of light. Everything is experienced in a vacuum. The units are meter, second, and kilogram. $$ {\displaystyle \\ mv\ =\ \sqrt{2m^2g(H-h)}\ \\ v\ =\ \sqrt{2g(H-h)}\ \\ \\}$$ $${\displaystyle \\ \Delta v\ =\ \sqrt{2g(H-h2)}\ -\ \sqrt{2g(H-h1)} \\ \\ \Delta x\ =\ (x2-x1)\ = 10 ^- 3 \\ \\}$$ $${\displaystyle \\ \Delta x\Delta v\ \ {\ge } \ {\frac{\hbar}{2m}}}$$ $$ and: $$ $$ {\displaystyle \\ \Delta p\ =\ \sqrt{2m^2g(H-h2)}\ -\ \sqrt{2m^2g(H-h1)} \\ \\ \Delta x\Delta p\ \ {\ge } \ {\frac{\hbar}{2}}}$$ * The question is and with the principle of uncertainty applied: will the particle oscillate transversely along x to give a value greater than ∆x?
-
@Enthalpy Very interesting this thread. I think the collapse of the charged particle, by its own mass, occurs effortlessly with the natural gravitational force. The charge is however not necessary. On the other hand, the emergence of the particle would then be the energy supplied, contrary to this gravitational force, but now with a charge. It would then take a vertical accelerator/collider to verify this.
-
It should be understood that when the particle is at h at the zero threshold and the impulse is given, then the momentum is high at the start due to its high speed up to an almost zero momentum at H, then the particle falls back. What it actually corresponds to when the impact is given, to the value of the momentum, which is equal to the k interval. We understand that the particle collapses at any time thanks to gravity, and reappears thanks to energy. If the leptonic matter is indeed radiative and vertical to gravity, then the bosons are only the difference in horizontal potential between two oscillations of the particle. It reminds me of the photon emitted by the oscillation of the electron.
-
I only said the unit was meter. After I specified 1 mm or 1 nm at your choices for the particle or quanta. We can also talk about 1cm. Indeed the interval Delta is x2=0,5 and x1=-0,5. But what are the different situations? You mean the principle of uncertainty which applies more or less depending on the size of the mass? This is true, because for macroscopic objects the principle of uncertainty is negligible. You have a width of 1 for x and y which oscillates more than 1 given the height. Which makes two dimensions with a surface x*y. However, it is simple if the observer is at the top at H there will be an oscillation of the particle in the form of a magnitude. As simple as that. But then what is the unit of x here in Heisenberg's formula? $${\displaystyle \Delta x\Delta v\ \ {\ge } \ {\frac{\hbar}{2m}}}$$
-
Meter. x corresponds to the width of the particle or the wave packet. If the unit is meter, then in this case it is 1 mm or 1 nm to choose from. With the uncertainty principle, the particle oscillates along y for a width of 1 along x. The principle of uncertainty is then real for an oscillation of magnitude, namely a greater appearance of the particle, with a decrease, or even disappearance of the visibility of the particle.
-
∆v = sqrt(2g(H-h2))- sqrt(2g(H-h1)) Then everything depends on h2-h1 and time t if x=1 for a macroscopic object with: $${\displaystyle \Delta x\Delta v\ \ {\ge } \ {\frac{\hbar}{2m}}}$$ The time of the duration of travel of the particle gives the height traveled. The longer the time, the more uncertainty is felt. Erratum: if x=1 then the uncertainty even for long distance is not felt. and ∆x = (x2-x1) = 1