frostysh
Members-
Posts
11 -
Joined
-
Last visited
Recent Profile Visitors
The recent visitors block is disabled and is not being shown to other users.
frostysh's Achievements

Quark (2/13)
0
Reputation
-

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
I am sorry, but my mission, at least for now, is not explaining fundamentals of classical and relativistic kinematics on science-popular level like a 'computer program' to the peoples that can only understand computer programs, but to understand some of this very fundamentals by myself. So corresponding to the present goal of my mission, I will not follow your suggestion and better will search the answer in some other places. But anyway, thank you, at least I have remind to myself some things when explaining it to you and to the forum users. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
1) The principe of invariance is not the same, invariant related to Galilean transformation is not the same as invariant related to Lorentz's transformation. At least I heard so, but my poor present knowledge of classical mechanics, and much, much more poor knowledge of relativistic kinematics. It's remarkably that formulas Doppler effect derived from Galilean transformation (as I have showed up above) is not the same as formula derived from Lorentz's transformation when speed is much more less the speed of light propagation in vacuum. If you think that invariances is the same and you can prove it? 2) Relativistic and Classical difference major is not in the speed of light finitiality, I think you are mistaken again. It's laying in two different groups of transformation Galilean and Lorentz's for an example. The branch of mathematics which is describing this difference called 'The Group Theory'. At least I have heard it in my University when study long ago. 3) I think you are not clearly understanding the formulas which you are typing (but of course I can be wrong), especially you are mistaken about physical meanings of that. It is a many works and articles in which is explained what I am trying to understand (and where you are totally mistaken, as I think), but the problem is me is in rural area, no job with money and I have no time to study so difficult to me subject for now, but I can provide you with some (there is actually a lot of) articles which can help, this one from google but in the large books is too lot on subject typed — On The Galilean and Lorentz Transformations (2013). (So horribly small pictures in the article.) Also there is some 'paradoxes' appears in relativistic Doppler Effect, and it cannot be so trivially described that you have tried. P. S. HOW to enlarge formulas on this forum? Like \large in Latex or something? It's looks not very cool and too small... Also in quotes is not good formulas representing, how choose so awful mathematics support for the scientific forum? *facepalm* -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
I mean hypotetically, it's just analogy that is less abstract to the one where we will imagine this very system of source-medium-observer, and the of infinite number of the inertial systems in the Universe. Of course I can type a nonsense, so beware, because I can create a new 'physics' and even believe in it, not saw my own mistake, it's sometimes happens... You are mistaken. You can imagine the standalone wave in medium without the source, and left the only observer and the medium, the wavelength is the length — no matter of observer speed (inertial frame) we will measure the same length. The dimension of wavelength can also torch up your intuition, it's — \( [L] \). Yes of course, but it's no so simple as can be on the first look, and paper not showing it in detail either (at for my opinion), there is a physical meaning in the model must be or will be no any point to speak about physics, it's will just abstract mathematical metamorphoses, when you saying "redshift" you must imagine the measurement without the very medium... And the inertial frames must apply somehow there, and so on, it's easily to mistaken there something, at least on my point of view. The point is the formula of Doppler effect derivated from the Lorentz's transformation have different physical meaning of inertial frame than the one from classical Galilean transformation. At least on my current level of understanding, because the both of this very transformations is more fundamental than the inertial frames in the physical models. I can mistaken, so if anybody knows something about this, actually not a trivial case, me very good to read it. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
Do you see formulas? Do you can disapprove it? You can try. Redshif is term which corresponds to the light, light have no require medium in which it propagating, we cannot use the classical theory there with a good physical meaning of this very theory because we cannot connect vacuum to the inertial frame... *facepalm* So your mention about red shift is meaningless too... -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
A good mention and try but we can imagine it from the less abstract way — assume that our model is a whole Universe, filled with this very medium (of course only theoretically, okay even hypothetically), and we somehow know the wavelength of source which is in the rest related to this medium — and the rest i connected to invariant, so it's privileged, no any other invariant with same value can appear except this will be equal invariants. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
Despite I very don't like a typing formulas on this forum, I will try. We have one-dimensional wave which have wavelength, frequency, and the amplitude which can be density for an example, the mathematics says that in simplest of situation we can describe this wave in model by the next equation: \[ G \left(x, t \right) = G_{max} \sin{2 \pi \left(\dfrac{x}{\lambda} - \nu t \right)}, \] where \( G_{max} \) — the maximum value of density in a waver, \( x \) — displacement from some particular point, in can be greater or less than zero. Let's say this density will be when we are looking on wave from an observer which is stationary related to the medium. Let's say we also have another observer which is moving with constant speed \( v \) related to the medium, and this observer see the density like that \[ g \left(X, t \right) = g_{max} \sin{2 \pi \left(\dfrac{X}{\Lambda} - \Gamma t \right)}, \] where \( X, \Lambda, \Gamma \) — displacement from the new point, new wavelength and the new frequency correspondingly. So what density will see the first observer in the point \( x \) after some time \( t \) and the second one in the point \( X = x - vt \)? Due to laws of conversation and time interval invariancy of classical mechanics this density must be the same of course, because the point on like is the same, and if we choose (we can choose point deliberately) with maximum, this is mean \( G_{max} = g_{max} \). Or will no be the any physical meaning at all. Okay, now we can make the equality, equation in this, very particular point \[ G \left( x, t \right) = g \left(x - vt, t \right) \] \[ G_{max} \sin{2 \pi \left(\dfrac{x}{\lambda} - \nu t \right)} = g_{max} \sin{2 \pi \left(\dfrac{\left(x - vt \right)}{\Lambda} - \Gamma t \right)}, \] of course the arguments of sinus-functions is not equal when the value of this functions equal! Bu we can chose the interval where it will be true, the point is the sinus function is so-called periodical one. And we can rewrite the very equation in the next way \[ \left(\dfrac{x}{\lambda} - \nu t \right) = \left(\dfrac{\left(x - vt \right)}{\Lambda} - \Gamma t \right) \] \[ \left(\dfrac{x}{\lambda} - \nu t \right) = \left(\dfrac{x}{\Lambda} - \left(\Gamma + \dfrac{v}{\Lambda} \right) t \right), \] let's make some reassembling \[ \left( \Gamma + \dfrac{v}{\Lambda} \right) = \Gamma', \] at least dimensional analysis saying that indeed it's some kind of frequency \[ \dfrac{1}{T} + \dfrac{\dfrac{L}{T}}{L} = \dfrac{1}{T}, \] and it's will be no other frequency than \( \nu \), because we have only two systems which making transformation and \( \Gamma' \neq \Gamma \) which is obviously, so let's rewrite our fine equation again \[ \left(\dfrac{x}{\lambda} - \nu t \right) = \left(\dfrac{x}{\Lambda} - \nu t \right), \] and, as easy to see the wavelengths is equal! Again, the wavelength is an invariant due to Galilean transformation, it's intuitively can be understand because the wavelength have dimension of \( [L] \) and length is an invariant the same as time interval. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
It's a good question, of course the Galilean transformation (so-called Galilean group in Group Theory) is far more general, and the 'break' is not the word. I mean some inertial systems which connected to the source will be privileged if medium exist, because we can connect the very relative speed related to medium with a very non relative and the very invariant wavelength. Cores is a modeling object in ballistic model of one-dimensional wave, it's mean a particular value of wave process which is travelling trough space (trough a line in one dimensional case), I don't know how to explain it to you more good, just google 'one-dimensional wave' or take any book about waves. I don't know what kind of error and code you talking about either, I just drawn a picture in GIMP, first one and the second one, no any 'errors' or you mean your formulas in post? I don't know why it is so, actually the formulas typing on this forum is a pretty awful to me at least... I have said clearly "we have a medium", in case of light there is entirely different physical meaning because we cannot connect inertial frame with a vacuum, it's obviously. Well, I am trying to understand it actually. Wavelength is NOT observer dependent, wavelength is an invariant, it's only source-medium dependent (that why I think Galilean relativity 'break'). You can realize it by yourself if you will do Galilean transformation of one-dimensional wave, it's the case like a source of waves disappeared and left only the medium and the very wave itself. I have done this transformation, and I will post it there, but after me will be familiar how to type formulas on this forum to avoid this errors and stuff. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
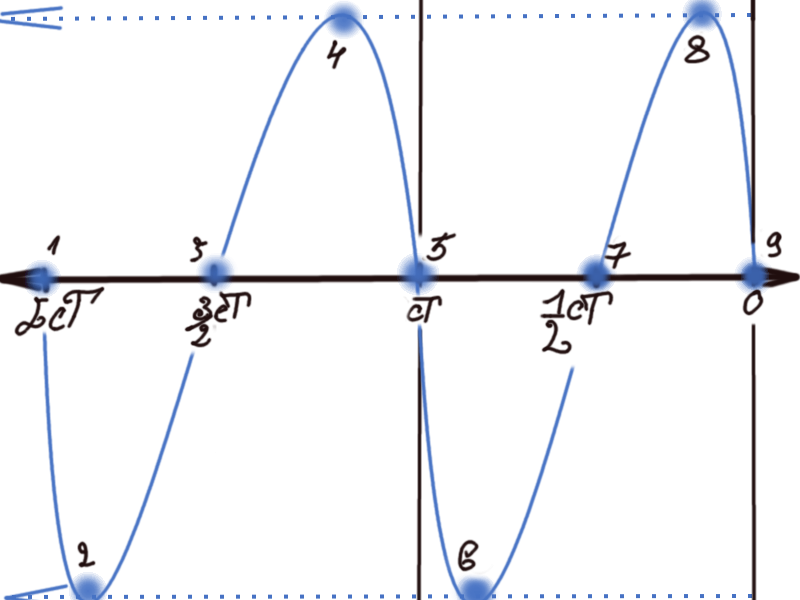
An abstraction means we are taking into account only very general properties or somekind — there is no matter the shape of the curve on the picture. It is one-dimensional wave, the picture is two-dimensional abstract model in which we are imagine the values of density of medium for an example as the shooting cores, when are too many core it will be a line. The curve is skew because me little bit fast trying to draw it, but as I said it's no matter. And why in your quote of my formula the displacement and wavelength is multiplied? The argument of sine-function must be dimensionless, it's so called transcendential funcion (OMG! I am not forget the whole university study which was long ago...), your formula that you quote have no physical meaning at all. You are particularly wrong about Doppler's effect, wavelength is changing when source is moving related to medium. And I think this process like an event, invariant, so it's breaking Galilean relativity. -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
Yes! Thank you. But what about the wavelength in the first case, the motion of source? I think movement of medium related to observer and source in the same frame will be equal of movement of source in one-dimensional case at least. If wavelength (which is invariant in any inertial system) is depends on relative speed of source related to medium, than we have somekind of event that can distinguish this, very particular inertial frame from the others, so it's looks like Doppler effect is an absolute of somekind. Yes, I have read Wikipedia somehow me poor around a week is trying to understand this effect and don't read Wiki about... So the question is little bit reorganized: if the wavelength is depends on speed, is inertial frame that connected to moving source can be assumed as privileged due to it's connection to invariant wavelength? This is because I didn't know how make formulas on this forum when I posted it, there is no matter. I draw it in GIMP. It's not a wave but an abstraction of one-dimensional wave which the mathematical description like that \[ \large A \left(x, t \right) = A_{max} \sin{2 \pi \left(\dfrac{x}{\lambda} - \nu t \right)} \] where \( \large x \) — is a displacement, can be larger or smaller than zero, \( \large \lambda \) — the wavelength and \( \large \nu \) — is a frequency. There is no wave pictured, the projection of that 'cores' on absciss axis (it is a distance line between source and observer) is a traveling in space values of property such as density. P. S. No working scaling formulas such as \large, \Large, etc... -

I need help with Doppler's effect understanding
frostysh replied to frostysh's topic in Classical Physics
Thanx, I will read and try to post more formulas. There is a way to include the pictures inside the text but not just to attach them on the bottom of post? -
First to say, how ridiculous that on scientific forum I am no see any things that can help me to type formulas... But instead of it a lot smiles. It is a very fun, but what about Latex or something? My second is connected on the first, I will try to formulate my questions in laconic way and without formulas: is wavelength changing when source of waves moving related to medium? The point is my 'plank-core' one-dimensional model (the ballistic one) is seems to say "yes it is", the wavelength changing, I have attached files that contain the picture that illustrating the rest and moving case of source, related to the medium. But the very Galilean transformation of the wave-function is saying "not it is not", the wavelength is no changing. Where is the truth? When answering considering that me is no good mathematical level, I was in past student and for now trying to refresh the knowledge and find a jog. Thanx for the future answers! Of course if it will appear...