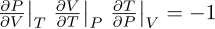-
Posts
4785 -
Joined
-
Days Won
55
Content Type
Profiles
Forums
Events
Everything posted by joigus
-
I am sorry but your thinking is not good question! Not sense make it now, not sense make it ever! Shut down or up, as you please!
-

kinetic energy and black hole formation
joigus replied to rjbeery's topic in Astronomy and Cosmology
Completely agree. That problem calls for numerical GTR. I was thinking along same lines when I said, On the other hand, linear accelerations for stellar objects are very rare phenomenologically speaking, I surmise. Plus any linear acceleration field would lead to accretion rather that inducing threshold trespassing, my intuition tells me, concurring with you, perhaps. But there could be an experimental/astrophysical context to do the trick perhaps. A neutron star approached by a heavy stellar interloper which induces strong tidal forces in it, as well as very intense centrifugal potentials. See if any such event of BH formation can be detected. But the acceleration field we're talking about would be very different, of course. -

kinetic energy and black hole formation
joigus replied to rjbeery's topic in Astronomy and Cosmology
Thank you for this pointer. I didn't know about geons. I'm very interested in self-consistent classical solutions. -

kinetic energy and black hole formation
joigus replied to rjbeery's topic in Astronomy and Cosmology
There are so many speculations about them. You're right. There's nothing common-sense about BHs. I think angular momentum may play a key part and, although Schwarzschild's solution is an exact one, only the Kerr-Newman maybe makes sense, the rotating one. It's in one of his video lectures. Don't know where it is though. I'll look it up. The thing about Susskind is he's so intuitive and pictorial. Even in the most abstract and difficult topics. Although he always discusses the Schwazschild solution. His lectures on supersymmetry, even though he's clearly unhappy at the end, are amazing. SS I think must be correct in some sense we haven't understood. A basic exposition like Susskind's I think is perfect for anybody young, without prejudice, that would like to have a go at a possible re-interpretation. But that's off-topic. -

kinetic energy and black hole formation
joigus replied to rjbeery's topic in Astronomy and Cosmology
Once it's fallen, there's no way back.That's what the theory says (Hawking radiation aside.) In fact, there is a very, simple, very nice Gedanken experiment explained by Lenny Susskind of a sphere of photons converging to a point, in such a way that a BH is bound to form, but even though you have the illusion that something could be done about it, there is a point past which the photons are doomed. I don't remember the details, but maybe I could find it. But I wouldn't bet my life on what a BH is actually going to do. On a rather more speculative note, I think gravitational horizons have something very deep to do with the problem of the arrow of time that we haven't understood very well at all. -

kinetic energy and black hole formation
joigus replied to rjbeery's topic in Astronomy and Cosmology
Very good question. It reminds me a lot of the twins paradox, but for GTR. The solution to the puzzle may go along these lines: If I understand you correctly, you've got yourself a bunch of matter that's stopped compressing just before it becomes a black hole, but it hasn't. (The Fermi degeneracy pressure is just enough to hold it partially outside of its Schwarzschild horizon.) Then you push it just enough from one side so that its energy density reaches the threshold, and it turns into a black hole. How could that be, if kinetic energy is --allow me to rephrase-- a frame-dependent concept? That means that if you go to a different inertial frame the kinetic energy, that in the first inertial frame looks like \[\frac{mc^{2}}{\sqrt{1-v^{2}/c^{2}}}\] would look like its rest energy \[mc^{2}\] Yes, but you've said that you're accelerating it, so there is no inertial frame you can go to where you can see it with constant zero velocity. In somewhat more technical words, you must include the fields that are accelerating your black-hole wannabe and either include them on the right-hand side of Einstein's equations (if they're other than gravitational) or in the Einstein tensor (if they are more gravitational fields). That's not frame-dependent, but covariant under general coordinate transformations. \[G μ ν =8 π G( T μ ν + δ T μ ν )\] Whether the system collapses or not would, I suppose, depend on the details of the dynamics. Maybe what you would do is save it from collapse. Many paradoxes arise in relativity (both special and general) when you forget that your reasoning requires non-inertial frames in order to make sense. Covariant means it is a tensor, and if a tensor is zero in one reference frame at a point, it is zero in every reference frame. So your force is not zero in any reference frame. -
Feynman Lectures on Physics, volume 1, chapter 1, Section 1-3. Atomic Motion. Go to your local library and start reading Feynman now. Then start mimicking Feynman in whatever way you can without giving up your principles, and maybe buy a pair of bongos. Just joking. But the key word in all of this is: Feynman.
-
That's probably because they eat their grandpa's brain for supper (true story.) They really are are very talented.
-
This very much converges with what I was thinking --even being an absolute nuthead when it comes to geology. The Himalayas are very much geologically active. They are very "plastic," so to speak. Erosion is at its maximum Earth-wise (you just have to take a look at the Kali Gandaki gorge.) I'm sure swathes of relatively young or "uncooked", sedimentary, non-metamorphic rock are being exposed too. But I must confess I'm not sure by any means... Eons of rock formation are being stripped away there. What do you guys think?
-
You are, as Endy0816 says, considering the work done by an ideal gas in an isothermal expansion or compression. Following your notation, \[\tau=-\int PdV=-\int P\left(V\right)dV\] So that, \[\tau=-\int nRT\frac{dV}{V}=-nRT\log\frac{V_{2}}{V_{1}}\] The reason why that procedure is only valid for reversible processes is that if you want to be able to guarantee that the equation of state \[f\left(P,V,T,n\right)=\frac{PV}{nRT}=\textrm{constant}=1\] is valid throughout the process, it must take place under equilibrium conditions throughout. In other words, the control parameters must vary very slowly compared to the relaxation times of the gas, so that it constantly re-adapts to the "differentially shifted" equilibrium conditions. Those are called "reversible conditions". Chemists use the word "reversible" in a slightly different sense, so be careful if your context is chemistry. Exactly.
-
I was quasi-quoting Dan Britt in Orbits and Ice Ages: The History of Climate. Conference you can watch on Youtube. You got me: argument of authority, I should be ashamed of. Conversations with you are starting to get very stimulating. Thank you very much for the references. I'll reconnect in about 5+ hours, then learn geology in about a couple of hours, and then keep talking with you, hopefully. The bio-data I got mostly from https://www.amazon.com/Life-Science-William-K-Purves/dp/0716798565 and a wonderful MIT course by Penny Chisholm.
-
I understand how you can say that. But it's not that clear to me. First, dinosaurs, like any other megafauna, are almost anecdotal in terms of primary production, carbon cycle, etc. To give you an example, there are about ten trillion tons of methane stored in the oceanic bottoms that can't get out thanks to methane-metabolizing microscopic archaeas that are keeping it at bay. And, mind you, methane is 25 times more greenhouse-effect inducing than CO2 is. If you want to understand ecosystems you must look at microorganisms. They don't look as pretty in a theme park, but are far more important for the global chemistry. Another question is the rate at which this is happening. Back in the time of the dinosaurs the conditions were quite stable, and many big animals (quite a big bunch of them in terms of animal biomass) may have been slow-metabolism. As to the dinosaurs, we don't really know if they were or how many there were. We do know that all the plants were C3, because C4 plants did not exist. How did that affect the carbon cycle? Be aware, e.g. that RubisCO, the carbon-fixating molecule, is the most abundant organic molecule on Earth by far. In fact, C4 plants, which are more efficient at sucking up CO2 from the atmosphere, precisely evolved to adapt to the new, slowly-changing, low-CO2 atmospheric conditions. And that's the observation that leads me back to the question of rate. Organisms need time to adapt, measured in tens of millions of years, not decades, for those paradises that you picture in your mind to establish themselves. We are now pumping into the atmosphere an estimated billion tons of CO2 per year. The Earth is 100 years within a Milankovitch cycle of glaciation, and yet the glaciers are clearly melting, and fast. We are really fortunate that the Himalayas are still pushing up, because this geological process sucks CO2 from the atmosphere at an incredible rate, and sends it back to the sea. The really big question now is what will happen when the ice sheet on Greenland sloshes down to the North Atlantic, as it is sure that the salinity will go down significantly and the conveyor belt that equilibrates the water temperature will eventually stop. It is estimated that that will happen by 100 years' time. Have you thought in any depth about these and other factors?
-
I totally agree. In fact, in the topics of physics that are dearest to my heart, it is my conviction that we must overcome this concept. I see your point. I went back to my sentence and I think what I meant (or must have, or should have meant) is "The culprit of all this is the fact that thermodynamics always forces you to consider energy." Instead of, No, I haven't, but from perusing the first pages --although the energy arguments weren't there--, it looks like a very interesting outlook. It reminds me of what Perelman did to solve the Poincaré conjecture: consider the Ricci flow to prove a topological statement. That's using a physical idea to solve a mathematical problem. I would talk more about this delightful topic, but my kinetics is forcing me to slow down. Maybe later. It's been a pleasure.
-
I have to agree with studiot's disagreement. That's one of the most common obfuscations when studying thermodynamics (TD). In TD you never go outside the surface of state, defined by the equation of state f(P,V,T,n)=0. That's why they most emphatically are not independent variables. This is commonly expressed as the fundamental constraint among the derivatives: which leads to unending "circular" pain when trying to prove constraints among thermodynamic coefficients of a homogeneous substance, for teachers and students alike. 'Kinetics' is kind of a loaded word. Do you mean dynamics vs kinematics in the study of motion, or as in 'kinetic theory of gases', 'chemical kinetics'? Sorry, I really don't understand. But I would really be surprised that a theory about anything in Nature missed the energy arguments. Sometimes you can do without it, but there are very deep reasons for energy to be of central importance. I would elaborate a bit more if you helped me with this.
-
"It is the customary fate of new truths to begin as heresies and to end as superstitions"
T. H. Huxley
-
I concur with swansont. Only, I think he meant, W = - delta(PV) assumes constant P when he said, as W = -P(delta V) is just the definition of work for a P, V, T, n system (the simplest ones.) And when n, T are constant ==> d(PV)=0 ==> W = -pdV = +VdP (for that case in an ideal gas.) Just to offer a mathematical perspective. If you differentiate (increment) PV=nRT, you get PdV+VdP = nRdT (d=your "delta"=increment, small change) or for varying n, PdV+VdP = RTdn+nRdT because, as swansont says, you must know what's changing in your process, and how. You see, in thermodynamics you're always dealing with processes. To be more precise, reversible processes (That doesn't mean you can't do thermodynamic balances for irreversible processes too, which AAMOF you can.). Whenever you write "delta," think "process." So, as swansont rightly points out, what's changing in that process? The culprit of all this is the fact that physics always forces you to consider energy, but in thermodynamics, a big part of that energy is getting hidden in your system internally, no matter what you do, in a non-usable way. This is very strongly reflected in the first principle of thermodynamics, which says that the typical ways of exchange of energy for a thermal system (work and heat) cannot themselves be written as the exchange of anything even though, together, they do add up to the exchange of something (here and in what follows, "anything," "something," meaning variables of the thermodynamic state of a system: P, V, T, PV, log(PV/RT), etc.) So your work is -PdV, but you can never express it as d(something). We say it's a non-exact differential. It's a small thing, but not a small change of anything The other half of the "hidden stuff" problem is heat, which is written as TdS, S being the entropy and T the absolute temperature, but you can never express it as d(something). Again, a non-exact differential. And again, A small thing, but not a small change of anything Enthalpy and Gibbs free energy are clever ways to express heat exchange and work as exact differentials, under given constrictions for the thermodynamic variables. And Helmholtz's free energy is something like the mother of all thermodynamic potentials and its true pride and joy.
-

Determinants : Proof det(AB)=0 where Amxn and Bnxm with m>n
joigus replied to Javé's topic in Linear Algebra and Group Theory
Yes, taeto, you are right, unless I'm too sleepy to think straight. The thing that's missing in your argument is the transformation matrix, which is, I think, what you mean by, I don't know if you're aware of it, but any Gauss reduction operation can be implemented by a square non-singular matrix. A change-of-basis or "reshuffling" matrix. Let's call it D. So that, AB = ADD-1B = A'B' The "indexology" goes like this: (mxn)x(nxm) = (mxn)x(nxn)x(nxn)x(nxm) The first factor would be an upper-triangular matrix (guaranteed by theorem that I can barely recall) but, as it has fewer columns than rows, at least the lower row must be the zero row, so that the product must have a zero row. Right? (AAMOF you can do the same trick either by rows on the left or columns on the right; it's one or the other. Then you would have to apply a similar reasoning to B instead of A, you're welcome to fill in the details.) This is like cracking nuts with my teeth to me, sorry. That's what I meant when I said, But that was a very nice piece of reasoning. It's actually not a change-of-basis matrix, but a completely different animal. -

Determinants : Proof det(AB)=0 where Amxn and Bnxm with m>n
joigus replied to Javé's topic in Linear Algebra and Group Theory
You may be right. Dimensional arguments could work. Let me think about it and get back to you in 6+ hours. I have a busy afternoon. Thank you! -

Determinants : Proof det(AB)=0 where Amxn and Bnxm with m>n
joigus replied to Javé's topic in Linear Algebra and Group Theory
Gaussian elimination does not help here. The reason being that it requires you to reduce your matrix to a triangular form, and in order to do that, you need the actual expression of the matrix, not a generic amn -

Can someone please explain galaxies moving 5 times light speed and
joigus replied to Angelo's topic in Relativity
My same impression. -

Can someone please explain galaxies moving 5 times light speed and
joigus replied to Angelo's topic in Relativity
How can a continuum be a constant? Could you elaborate on that? Maybe you're on to something. Can a stone be unhappy? See my point? If there is **one** feature of gravity that singles it out from every other force in the universe is the fact that you can always locally achieve absence of gravity (equivalence principle, EP). The only limit to this is second-order effects, AKA tidal forces. Jump off a window and you'll find out about EP. Get close to a relatively small black hole and you'll find out about tidal forces. Read a good book and you'll find out about how this all adds up. Oh, and mass is not concerned at all in GTR, as it plays no role in the theory. It's all about energy. It's energy that provides the source of the field. What you call mass is just rest energy, and this is no battle of words. Photons of course have no mass because they have no rest energy; and they have no rest energy because... well, they have no rest. Incorrect: Special Relativity (SR) says nothing (massless or not) can travel faster than the speed of light. Because GTR says geometry of space-time must locally reduce to SR, things moving locally can't exceed c. In other words: things moving past you can't do so at faster than c. People here have been quite eloquent so I won't belabor the point. I don't want to be completely negative. My advice is: Read some books, with a keen eye on experimental results; then do some thinking; then read some more books; then some more thinking, and so on. Always keep an eye on common sense too. Listen to people who seem to know what they're talking about, ask nicely for inconsistencies and more information, data. Always be skeptic, but don't just be skeptic. It doesn't lead anywhere. -
Depends on what they want to illustrate with it. Do you mean state of uncertainty? It's actually a paradox that cosmologists face every single day. No down-to-Earth physicists worry about it, because they use the quantum projection, or collapse, or wave packet reduction, as you may want to call it. They know whether the cat is dead or alive. As to cosmologists... who was looking at the universe when this or that happened, you know? It's not useful for anything, it's just there, looking us in the face. It's a pain in the brain. . Yes. No.
-

Tangent Space and Cotangent Space on a Surface.
joigus replied to geordief's topic in Linear Algebra and Group Theory
If you are a future mathematician, I would advise you not to try to think of the cotangent space as something embedded in the space you're starting from. In fact, I bet your problem is very much like mine when I started studying differential geometry: You're picturing in your mind a curved surface in a 3D embedding space, the tangent space as a plane tangentially touching one point on the surface, and then trying to picture in your mind another plane that fits the role of cotangent in some geometric sense. Maybe perpendicular? No, that's incorrect! First of all try to think in terms of intrinsic geometry: there is no external space embedding your surface. Your surface (or n-surface) is all there is. It locally looks to insiders like a plane (or a flat space). What's the other plane? Where is it? It's just a clone of your tangent plane if you wish, that allows you to obtain numbers from your vectors (projections) in the tangent plane. It's the set of all the vectors you may want to project your vector against, therefore, some kind of auxiliary copy of you tangent space. That's more or less all there is to it. Sometimes there are subtleties involved in forms/vectors related to covariant/contravariant coordinates if you wish to go a step further and completely identify forms with vectors when your basis is not orthogonal. That's why mathematicians have invented a separate concept. Also because mathematicians sometimes need to consider a space of functions and the forms as a bunch of integrals (very different objects). In the less exotic case, the basis of forms identifies completely with the basis of contravariant vectors. I will go into more detail if you're curious about it or send you references. I hope that helps.