joigus

You're welcome, and thanks for your appreciation. Both cellular respiration and photosynthesis are very old mechanisms for obtaining energy from the environment. Cellular respiration "sits on top" of fermentation, which is believed to be the oldest game in town. The take-home lesson being: Don't bury mantises in pots.

Infinite in time --although an idealisation-- is OK. "Infinite in space" requires some clarification: 1) Infinite in both the region of space the particle is restricted to move, and the proper length parameter (usually called s) 2) Finite in space (region where your particle is restricted to move) but infinite in the proper length parameter even though the particle may cover a trajectory over and over again (periodic motion) Your 2nd case corresponds to my 2nd case. In your 3rd case, the motion is finite in space and in the proper length parameter, but infinite in time in the mathematical ideal case. A good example is a particle moving in a viscous fluid. In the ideal case, the particle velocity is a decreasing exponential, and it would ideally take infinite time for the particle to stop. What one does in a practical case is to set up a time T such that the ratio between the particle velocity at time T and the limit velocity has gone down to a level that you would consider that it has effectively come to a halt. Say, \[ \frac{v\left(T\right)}{v\left(\infty\right)}<.99 \] where \( v\left(\infty\right) \) is the limit velocity. So, as @swansont said, the 3rd case is finite in space. It's also finite in time for all practical purposes. And in the real case too, as the velocity is well below the level of statistical fluctuations at some point.

Very nice. I think those fish are feasting on some stuff...

There's always a bad apple, isn't there? In this case a bad rafflesia. Very interesting.

Sorry I haven't been very helpful. To give you an idea of how central it is whether an element is a metal or not, or how much of a metal it is, is absolutely central to chemistry, here's a snapshot of the periodic table. Alkaline (also called alkali metals) Non-metals Other metals Transition metal Metalloid Rare earth Alkalie metal Halogen Nobel gas The concept of metal is so central to chemistry that you could understand a lot of what chemistry is about focusing on the concept. You would miss another important central concept, which is acid/base, and has to do with transfer of protons, rather than electrons.

They don't have chloroplasts. Even liverworts and mosses (the most primitive plants that still exist) have chloroplasts. Do mantises have chloroplasts? And That's why they're not autotrophs: They don't synthesise sugar from CO2, consuming water. Animalia (cellular respiration): C6H12O6 + 6O2 --> 6CO2 + 6H2O (many intermediate steps omitted) Plants: Cellular respiration plus, Photosynthesis: 6CO2 + 6H2O --> C6H12O6 + 6O2 which is (overall and oversimplifying) the inverse of cellular respiration. LOL. x-posted with @Genady

Not really that much of an insight TBH, but I'll take it anyway. Baez-Dolan cobordism hypothesis is used to clasify topological quantum field theories. What does it have to do with computation? I'm familiar with Baez's motto "A QFT is a functor." However interesting such statements may be in physics, what do these manifolds represent in computer science? Topological information on a manifold could be coded in topological invariants, like, ie., the Betti numbers. Is that the connection? Thank you, but no, thank you. I spent three months studying those under a teacher who was a mathematical physicist. Very nice, very good teacher. A fountain of mathematical knowledge in the vein of Baez. I thank him for the A he gave me, which I didn't deserve. But I don't know what the hell we were doing studying monoids and alphabets as a substitute for group theory for physicists. Apparently, no one in the department wanted to teach the Lorentz group because it's not compact, and that's very very bad, for some reason. I had to learn that from Weinberg's QFT Volume I. I tell you this just to explain I'm a person very much shaped by the effort of trying to free myself from the shackles of being exposed to layers and layers of abstraction that don't lead anywhere useful. As to, I don't know what to do with that, or in what sense it clarifies what information is, or how it's stored, deleted, encrypted, etc. It certainly doesn't clarify Landauer's principle to me. You might as well have given me the procedure to pluck a chicken. What particular aspect does it clarify in information theory? That's what I call an insight.

Yes, Giant Technicolor Squirrels Actually Roam the Forests of Southern India: https://www.smithsonianmag.com/smart-news/yes-giant-technicolor-squirrels-actually-roam-forests-southern-india-180971886/ Surreal Frozen Lake Michigan Turns Into a Magical Wonderland https://www.beautyofplanet.com/surreal-frozen-lake-michigan-turns-into-a-magical-wonderland/?fbclid=IwAR2nGvee4LVegUc7Y-2XD9gi6N2DaRmKKb-pP0A2EoIZ5mPSVUVgGs9sYsI

No, no. You go. I took Chemistry 101 many years ago. You tell me. 😇

It's statements like these that are a real timesaver for me when pondering whether to follow a certain line of reasoning. Thank you so much.

https://how-you-can-support-ukraine.super.site/

I'm going to build an argument that's completely isomorphic to this --no matter how scholarly-- silly idea: If sacrificing a virgin is the only means of avoiding a natural catastrophe that would wipe out thousands of lives, sacrificing a virgin should be used --and will be used-- to obtain the desired results... No one who doubts that this is the case should be in a position of responsibility. Another isomorphism: A chain is as strong as its weakest link. An argument is as weak as the validity of the "if" it rests on.

Agreed that this is a difficult topic. I think I understand pretty well what entropy is in the context of physics. Yet, I'm finding pretty tricky to build a completely unambiguous conceptual bridge from what entropy means in physics, and what it means in computer science. There may well be a good reason why that is so. While entropy is completely central to physics, it seems to be --unless I'm proven otherwise-- that it's not a matter of life and death in computer science, at least from the practical point of view. It's very tempting to me to start talking about control parameters and how they really determine what information is available to anyone trying to describe a system, but it would make it a discussion to heavily imbued with a purely-physics outlook. @SuperSlim, You've been very dismissive of every attempt at defining information, entropy, as well as of other qualifications, comments, etc. by the other three of us. I think you made a good point when you talked about evolution. I was kinda waiting for the illuminating aspects that were to follow. But they never came. Would you care to offer any insights? I'm all ears. And I'm sure @Ghideon and @studiot are too.

I think I can see at least three yetis there.

I don't know about Swansont & \( \Phi \) \( \forall \), but ask me anything about etc.

This is a selection of news during the last days on phys.org that have caught my attention in the last days, for one reason or another. Developments in Environmentally-Friendly Technology: New screening system may point the way to clean, renewable hydrogen power https://phys.org/news/2022-03-screening-renewable-hydrogen-power.html Sewer treasure: Engineers reveal how to optimize processes for transforming wastewater sulfur to valuable materials https://phys.org/news/2022-03-sewer-treasure-reveal-optimize-wastewater.html Archaeology: Origin of the 30,000-year-old Venus of Willendorf discovered https://phys.org/news/2022-02-year-old-venus-willendorf.html Cosmology: Experiment on reservoir in India fails to find evidence of cosmic dawn https://phys.org/news/2022-03-reservoir-india-evidence-cosmic-dawn.html More information, as well as criticism, welcome. Enjoy.

Sorry, I can't see a difference here. Yes. Infinitely many (ideally) copies of the system on which to perform the statistics. OK, I see. Thanks for the clarification.

We have. The jury went home very long ago, and the answer is no. Gravity is nothing like EM: non-polar, equivalence principle... High-energy experiments proved long ago that there are colour charges which are non-electromagnetic in nature. EM doesn't explain decays, which are explained by weak interactions instead.

Even if there is no evolution, any setting that involves probability requires an ensemble: An infinite set of instantiations of the system that justifies the use of probability. I wonder if that's what you mean by "globally." I look at it more from the side of physics, so there's a lot to learn for me. No rush. Interesting discussion is what's interesting. Have a nice trip.

Cut down on weed.

I don't think one can say that not having found squarks or photinos in LHC does away with M theory for good. There's a mixed blessing in superstring theory, which is: There's so much freedom in the theory that it's very difficult to rule it out completely --I think--, almost as much as getting concrete predictions from it. As to supersymmetry, my comment is: High-mass predictions of superpartners are based on masses arising from spontaneous symmetry breaking. That's the only game in town now. But, what if there are other ways for a symmetry to be hidden? Supersymmetry is a very compelling idea. It's not just about superpartners. I think it goes deeper. It's about symmetries of space-time and internal symmetries. It relates both. Very compelling. Maybe we need to understand quantum mechanics better in order to see what it's telling us. I'm not saying it must be pushed forward at all costs, but I don't think we're done with trying it out just yet. I think I can argue in favour of SS a little more, but not now. 😫

Yes. The ambitious (not toy models, like supersymmetric quantum mechanics, etc.) are called supersymmetric extensions of the standard model. There are several of those, depending on the number of so-called central charges. The simplest is known as minimal SS extension of the SM.

When people say "string theory" what they really mean is "superstring theory" or "M theory" with SS. A theory of strings without supersymmetry is a non-starter. One of the reasons for this is that you need the fermionic diagrams to correct for the uncontrollable bosonic diagrams, to remove the fine tuning in the Higgs mass. The other is that the best-behaved QFTs are those with supersymmetry. They can be exactly solved or perturbatively convergent.

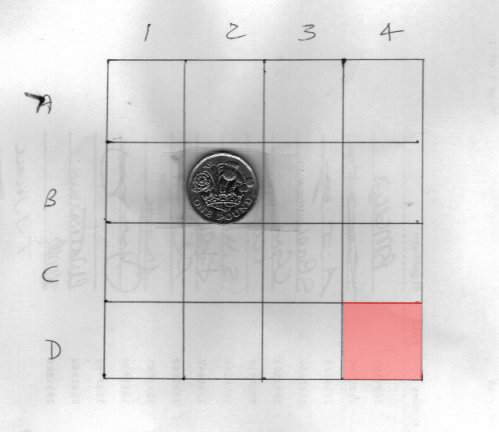
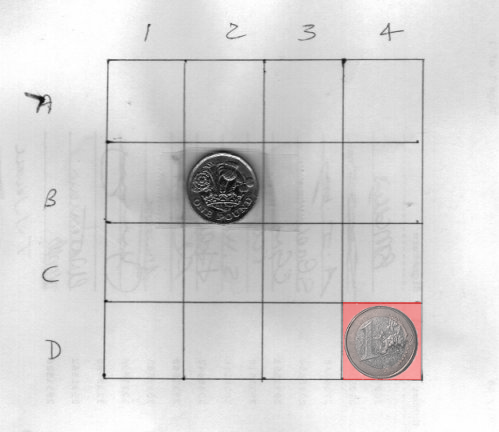
I think there are several aspects in which one could try to make this example more similar to what's going on in physics and, in particular, in computers. I'm no computer scientist, so don't take anything I say too seriously. I think interesting features are at play in thermodynamic systems that are not necessarily that relevant when it comes to computers. The most important one, IMO, is that computers are not generally set up in a way that they will end up being in a state of equilibrium. The other, perhaps equally important, is that the way in which circuits in a computer switch on and off is not ergodic, meaning that during a typical run of a program, the bit-states do not cover all possible configurations, let alone equally likely. I suppose --correct me if I'm wrong-- they will be more likely to run along some paths than others. And my intuition is that the sequence of configurations would have a strong dependence on initial conditions too, something that's not a characteristic of thermal-physics. Another feature that our toy example doesn't have, but both computers and thermodynamic systems share is, as @SuperSlim has been trying to tell us all along, evolution. So we would have to picture our system more like a table on which we're free to add and remove coins according to an updating law (evolution) that determines which square is occupied, and which is not, in an unpredictable way. If that's the case, I would find it easier to interpret Landauer's principle in the following --overly simplistic-- way. This would be a state frozen in time: Where you have to picture any number of coins compatible with available room as free to move about following an updating procedure. All squares are available to be "ocupied" with a microstate 0 or 1. At this point, the updating law (program) has yet to write a bit on a given square. Mind you, this concept could be applied to either RAM, ROM or SWAP memory. Now the program finally writes a bit. Whether it's midway during a calculation (for storing the value of a variable, for example), or to hard-disk memory, it takes some kind of process to hold the value there. It could be 0, or it could be 1. It's the holding that's important. I will denote this with a reddish hue: (Bit 0) Or: (Bit 1) At this point, the rest of the coins are free to occupy whatever other squares, as the case may be, except for that in position (D,4), which is occupied (whether it be 0 or 1 the bit that's occupying it), until it's freed again. This restricts lowers the entropy, because, for however long, the system has become more predictable. If I erase the bit, what does it mean? I think it means that I cease holding the value at (D,4) in position, the square being available again either for temporary use, or for more long-term one. I may be completely wrong, or perhaps oversimplifying too much, but please bear with me, as I'm groping towards understanding Landauer's principle on a physical basis. One final comment for the time being, I don't think you need evolution in order to define an entropy. Whenever you have a statistical system with a fixed number of states, as in @studiot frozen-in-time example, you can define an entropy as long as you have a way to asign probabilities to the different configurations.