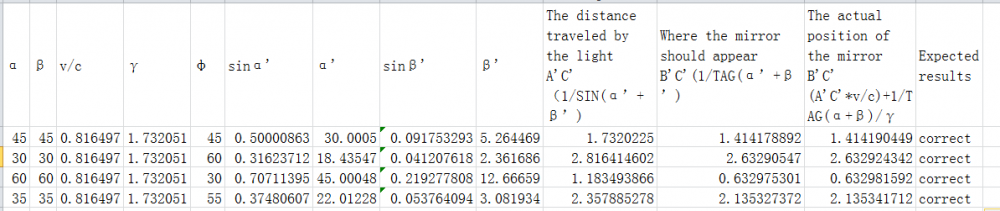
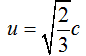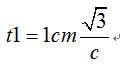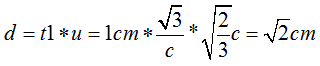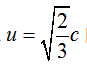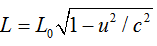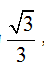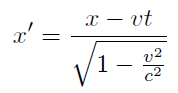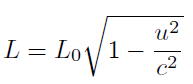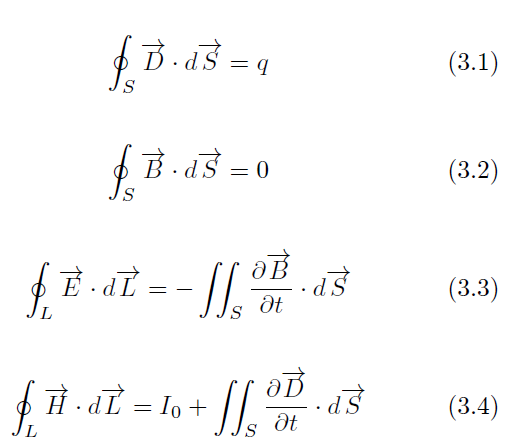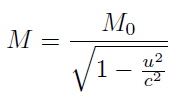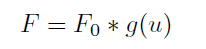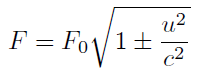The establishment of the Maxwell equations marks the perfection of the classical physics. But it is resolved from the Maxwell equations that the velocity of light is a constant. To explain the confict between the Maxwell equations and the classical physics, Lorentz revised the Galilean transformation to Lorentz transformation. Since the invariableness of the light velocity is veried by Michelson and Morley experiment, the light velocity has been accepted by people as a constant, and thus the special relativity put forward by Einstein.
According to the special relativity theory equation:
Suppose that R1 and R2 are both inertial reference systems whose relative velocity is u. If an object is static relative to R1 and the length of the object is L0 in the X axis direction. And in reference system R2, the measured length of the object is L. Then the relationship between
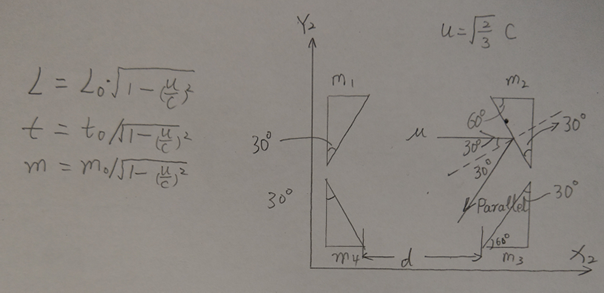
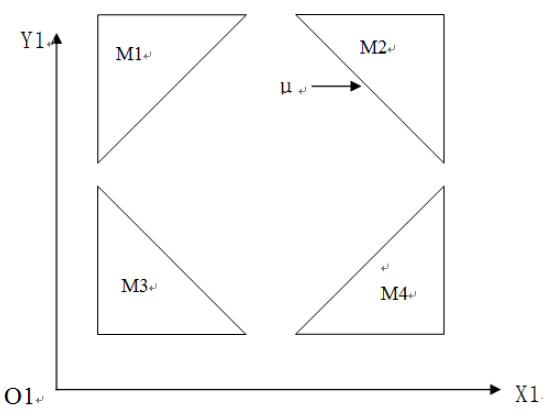
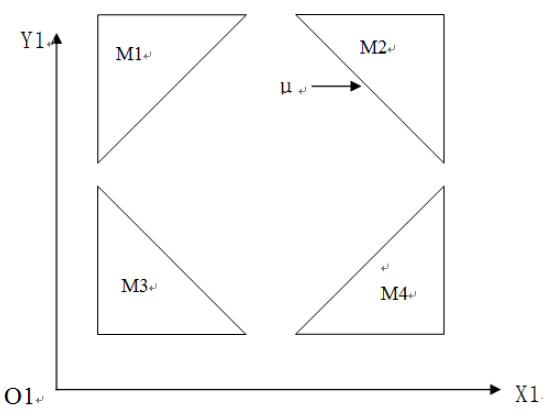
The following experiment is designed in the inertial reference system R1:
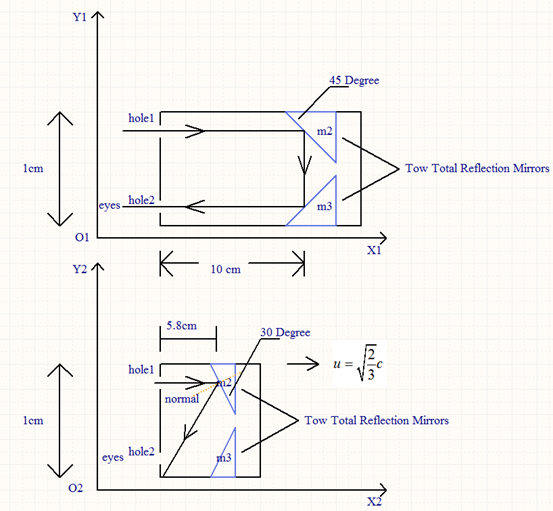
in above picture, M1, M2, M3 and M4 are four total reflection lens, whose cross-sectional shape are isosceles right triangles. Photonμis eradiate into the surface of M2 with the incidence angle of 45 degree. It will be reflected among the four lens. Assume energy is conservative during the reflection process, the photon μ will always stay in the cage of the four lens and vibrate forever.
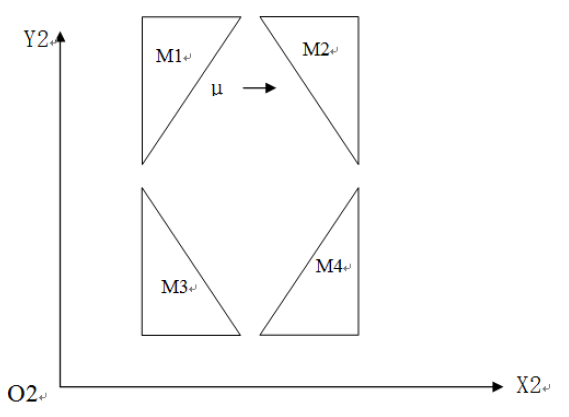
Now there is another inertial reference system R2, Which is moving at a constant speed in direction of X axis compared to R1. If observed in R2, according to the second equation the four lens of M1, M2, M3 and M4 shrink in the direction X and remain stable in direction Y as illustrated in the following picture:
Because the lens are deformed in R2, the Photon μ will not in parallel with Y after being reflected by M2. Thus the photon μ will escape from the gap between M1, M2, M3 and M4 after several times of reflection.
So in the two reference systems above, two different results will be observed for one thing, which is in conflict with the fact that everything has its uniqueness.
The essence of the Maxwell’s equations and the permanent principle of light velocity
The Maxwell equations are as follows:
Among which, the experimental basis for equation 3.1 is the coulomb’s law. The experimental basis for equation 3.2 is the Biot-Savart’s Law. The equation 3.3 is deduced from Faraday’s law of electromagnetic induction and the equation 3.4 is deduced from the Ampere circuital theorem and Maxwell’s hypothesis.
During the solving process of the equations, Maxwell did not mention the reference system. But the Coulomb’s law and the Ampere circuital theorem are the conclusions based on the experiment in the reference system of the earth. So the Maxwell’s equations cannot be regarded as have nothing to do with the reference system. It is not scientific to discuss the Maxwell’s equations apart from the reference system.
Now a new theory will be brought to explain the constancy of the speed of light and the experimental result of Michelson-Morley.
The experiments done by great scientists such as Coulomb, Ampere, and Faraday are two objects that remain stationary. We define this as the reference frame 1. If we observe at reference frame 1 in another relative motion reference frame 2, the experimental reference frame 1 has a certain velocity, but the two objects in the experiment remain relatively stationary, so I have reason to conclude:
The speed of the electromagnetic wave is constant relative to its emission source.
Electromagnetic wave comes from the emission source. The Maxwell’s equations did not mention the reference system. The experimental speed of the electromagnetic is in such accordance with the solution of the Maxwell’s equations. So it can be derived that the speed of the electromagnetic wave is constant relative to the emission source. The scientists are always relatively static to the emission source when testing the velocity of the electromagnetic wave thus the testing result are always and must be a fixed value.
From this theory we can deduce that the experiment data and conclusion of Coulomb, Ampere and Faraday are all correct. Because in their experiment the electric charge, the magnet, the conductor and the coil are static relative to the reference system of the earth. So the speed of electromagnetic wave is constant. The positon of the static charge and its electric field is fixed. The positon of the magnet and it magnetic field is also fixed. But if the observer is moving relative to the experimental reference system of the emit source, the tested velocity of the electromagnetic wave will not be a constant, which comply with the speed superposition principle. Thus the Doppler effect of Electromagnetic wave can be observed.
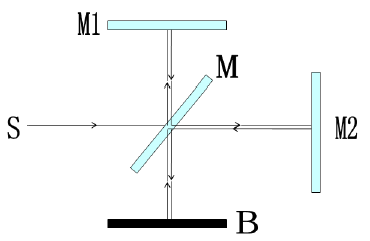
Now let’s go back to the Morley and Michelson’s experiment. To explain the result of the experiment, we need to rely on theory one. The relative speed of the reflected light to the reflector is constant. So in the Morley and Michelson’s experiment, the emission light source, stripe interference plate and the translucent glass in the middle are relatively static. That is to say,The geometric space formed by the objects in the experiment is a constant value with respect to the wave lengthλof the light. Thus no matter how the experiment system turns or no matter whether it moves with the earth, the shift of the interference figure will not be observed.
In the above figure besides the light source S, the pellicle mirror M and the reflecting mirror M1/M2 can also be regard as light source. So the speed of the light reflected or emitted by them are constant C (velocity of light).
The most important experiment to support the theory of relativity is that a charged particle can never be accelerated to the speed of light by a linear accelerator or cyclotron. The higher the speed of the charged particle is, the more difficult for it to be accelerated. Thus the theory of relativity is put forward:
According to the Hubble’s law, the universe is expanding and the farther the distance between the stars and the earth, the bigger the radial regression rate. Suppose there are two stars in the faraway place which are moving away from the earth with the velocity of light. The two stars are near to each other. Then seen from the earth the mass of the two stars are infinite in the process of high-speed regression. The gravity of them will be infinite too .Ultimately the two stars will merge together. Otherwise seen from the double star reference system which are separated with the light velocity, the earth and the sun will merge together by the appealing force of the infinite mass while it is definitely not true.
The essence of force is interaction. But without the interaction, we can never perceive the existence of the objects let alone to measure the force. In the view of the conventional concept, the interaction between objects is unchangeable. Now a new theory is put forward:
The interaction force between objects is changing with the relative radial velocity of the object.
g(u) is the transformation factor , which is the radial velocity between objects. The following transformation equation on F and F0 can be estimated based on equation below.(only I guessed)
In the above formula, F0 is the apply force with the relative speed of the accelerating field is zero. “+”stand for the backward motion to the accelerate field. “-”stand for the forward motion to the accelerate field.
Now we know that the particle can’t be accelerated beyond velocity c by an electric field accelerator in the earth. While in another reference system the velocity stack still exist which is to say in different reference system the moving speed of an object can exceed c. that is why it is not necessary to rectify the Galileo transformation to Lorentz transformation or add a reference system for the Maxwell’s equations.
Note that in the Theory of Relativity force is also a covariate. But the deduction is based on the change of the mass. The transformation formula of force is the derivation result of the momentum equation. In the Theory of Relativity many variables are covariate. While in this theory the mass, the time and the length are constant value.