JustSaying
Members-
Posts
4 -
Joined
-
Last visited
Recent Profile Visitors
The recent visitors block is disabled and is not being shown to other users.
JustSaying's Achievements

Lepton (1/13)
0
Reputation
-
Well, I've moved on to another, more suitable site. The misunderstanding was due to a failure to communicate. I'm anxious to answer questions and to adhere to scientific rigor guidelines, but when moderators pass judgements on how many posts are allowed to do that, it's seems juvenile to me. It is impossible to explain a major departure from legacy science and just jump into equations, without laying sufficient groundwork. There has to be more freedom for an author to prepare the reader to understand the change in concept, especially one of this scope. Honestly, how many of you understand what a system of theory is? My guess is none of you do, yet there was not one comment/question on my first post explaining it. There was only an impatient calling for getting to the meat of the theory. Something that, if I did, would be impossible to understand, without sufficient preparation. Big mistake. Anyway, no hard feelings. Just disappointment and frustration.
-
I just lost an hour plus work, because I'm not familiar with the site yet. I clicked on "view reply," while composing. Big mistake. Ce le vie. But maybe I misread things here, anyway. I thought it was a forum for speculations and pseudoscience presentations. When the critical judgements and cynicism are at the point of the spear, as if the moderators are competing to see who can expose the "malthinking" first, before an author has been able to say all of what he thinks needs to be said upon the topic, it's just not worth it to me. I'll see if I can find a more tolerable and patient site to do this. Sorry, it's just not a good fit for me. Bye.
-
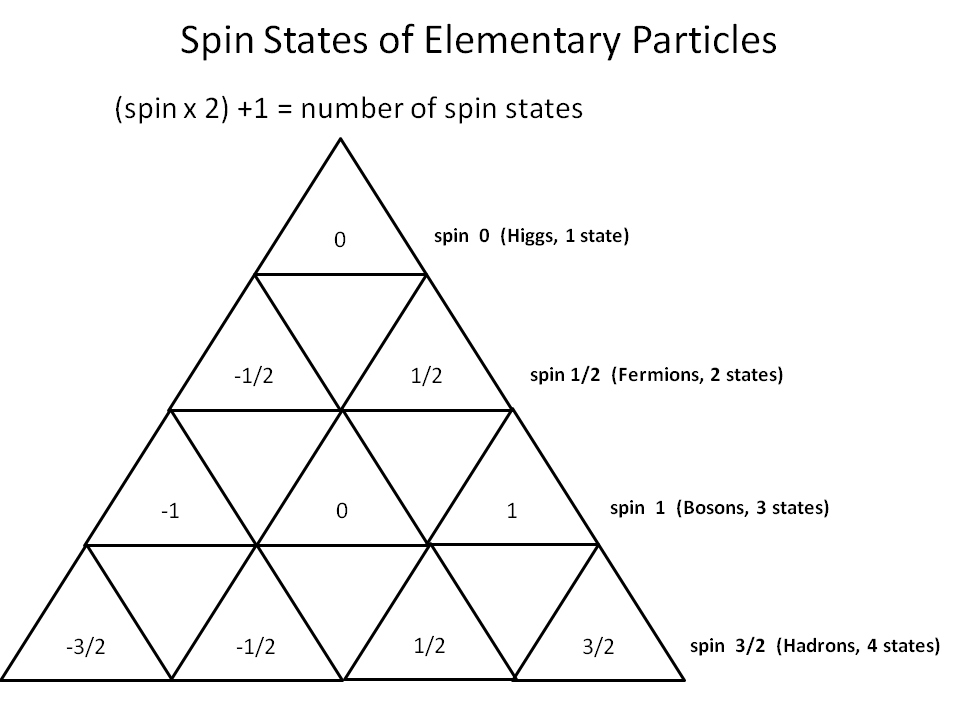
In the previous post, I explained what is meant by a system of theory, as opposed to a theory based on that system. I also explained that the RST is a new, deductive, system of physical theory that I have exploited to develop a new theory of the standard model of particle physics. Accordingly, it is referred to as an RST-based physical theory and denoted as RSt, with the lower-case t indicating the difference, thereby distinguishing the theory from the system, or program of research, it is based on. The RST is a deceptively simple system of research. It begins with two postulates from which a universe consisting of nothing but motion is deduced, by developing the consequences of the postulates (speculations). These two postulates are: 1) The universe is composed entirely of one component, motion, existing in three dimensions, in discrete units, with two reciprocal aspects, space and time. 2) The physical universe conforms to the relations of ordinary commutative mathematics, its magnitudes are absolute and its geometry is Euclidean (i.e. flat). Now, the moderators of this forum want me to lay out the evidence in one post that this is a valid, scientific, system for developing physical theory. I really don't understand the reasoning behind this requirement, but as a new-comer I want to comply, even though to me it's folly to think it can be done in one post. Nevertheless, assuming the reader understands what the evidence is actually establishing (i.e. the validity & usefulness of the system), here is what I will start with: Quantum spin and spin states: We all know how Pauli was unable to make the wave equation relativistic, if it were to incorporate quantum spin, yet Dirac not only achieved it, but his relativistic wave equation actually required it, astonishing the world even to this day. Nevertheless, as Bruce Schumm explains in his book Deep Down Things (page 187), physicists don't have "a clue about the physical origin of spin." It truly is a vexing enigma, because quantum theory would not be possible without this mysterious observable, and yet no one knows where it comes from, or even how it can exist (what cycle of rotation only completes in two, 2pi, rotations?). Happily, the RSt that I am developing explains quantum spin in terms of its discrete units of motion (more detail on these to come.) These units are actually discrete, oscillating, volumes (3d space/time ratios), which combine and interact according to varying degrees of freedom in the theory. A given degree of freedom determines how the units can possibly combine and begins with 1, then doubles to 2, then to 4 and finally to 8 unique combinations, as dimensions increase from 0 to 3. It's important to note that these four powers of 2 are inherent in the numbers of the first four levels of Pascal's triangle, which also happens to be isometric to the number of "directions" (poles) possible in each of the three known physical dimensions (four counting zero): 1st dimension = 20 = 1 (a mathematical monopole, analogous to a geometric point) 2nd dimension = 21 = 2 (a mathematical dipole, analogous to a geometric line) 3rd dimension = 22 = 4 (a mathematical quadrupole, analogous to a geometric area) 4th dimension = 23 = 8 (a mathematical octupole, analogous to a geometric volume) In QM, the possible spin states of the standard model particles are calculated by multiplying the spin of a particle type by 2 and adding 1: As it turns out, the combinations of the RSt's units of motion follow the same pattern of quantum spin and number of spin states, as shown above, indicating that these motion combinations follow the QM rules of quantum spin, as observed in physical observations. This is a good start to finding evidence that the system works , but many questions remain, which unfortunately can't all be addressed in one post. In the next post, I'll show how the existence of the theory's units of motion and their properties (including 4pi spin) are derived, leading inevitably to combined entities of these units that can be identified with the bosons and fermions of the standard model, having these identical spin states, if it's ok with the moderators to proceed.
-
Hello all, I'm new to this forum, and my purpose for posting here is to introduce a new system of physical theory to forum readers, for what it's worth. It's not that it hasn't been published/discussed previously, and it didn't originate with me, but I have applied it for years to develop an alternative physical theory to the standard model theory of particle physics, and I would like to receive informed feedback to the issues it raises here, if you don't mind. As you can imagine, it's a huge and very esoteric topic, not something one's friends and non-professional associates are anxious to engage. Since I am not a professional physicist myself however, I don't really have that many opportunities to share my work with professionals. The originator of the new system passed away about thirty years ago, but managed to self-publish several books on his system during his lifetime, but, while he too was what he liked to call an "amateur investigator," he was a very brilliant engineer. His name is Dewey B. Larson, and he called his new system of theory, the Reciprocal System of Physical Theory (RST). You may have heard of him and his work, but so many have regarded it as pseudoscience, including the consensus at Wikipedia, that it is mostly obscure to date. Nevertheless, as it turns out, it produces some startling results that go right to the heart of theoretical physics, which I intend to describe here (It was either do it here or in a peer reviewed journal - Ha!). Most readers will no doubt ask, "So what is a SYSTEM of physical theory? Mr. Larson explained it as the second system of physical theory since Sir Isaac Newton's, which is the system of theory that is normally used in today's theoretical research, where David Hestenes describes it as a program (system) of research the grand goal for which is to “describe and explain all properties of all physical objects... in terms of a few kinds of interactions among a few kinds of particles." (See his New Foundations for Classical Mechanics.) Of course, with the advent of quantum mechanics, Newton's program was modified, but its grand goal has not changed, nor has its focus on finding the fewest interactions among the fewest kinds of particles. As we know, the results of the research are currently found in the standard model of particle physics. Using Larson's new system, however, we find many of the same results corresponding to those of the standard model, but with entirely new equations of motion. The trouble is that, while the new equations of motion are spectacularly simple and useful, they are equations of a type of motion not explicitly dealt with in Newton's system of research. The new system postulates the existence of this unrecognized motion as the sole constituent of the physical universe, radically departing from the former system, where the observed particles of the standard model are deemed as elementary entities existing within a fixed background of space and time. This is of special interest, due to the fact that, currently, gravity cannot be theoretically explained, without warping the fixed background required by the standard model theory of Newton's system, making the two theories of the system incompatible with each other. The details require a book-sized treatise to develop them adequately, but suffice it to say that this is the crux of a great scientific controversy, sometimes referred to as "the trouble with physics." In the next few posts, I will describe Larson's new system of theory and also the beginning of the new mathematics it invokes, given my application of it. The results of this work will then be presented as prima facie evidence of the validity of the system (i.e. the validity of the RST).