Killtech

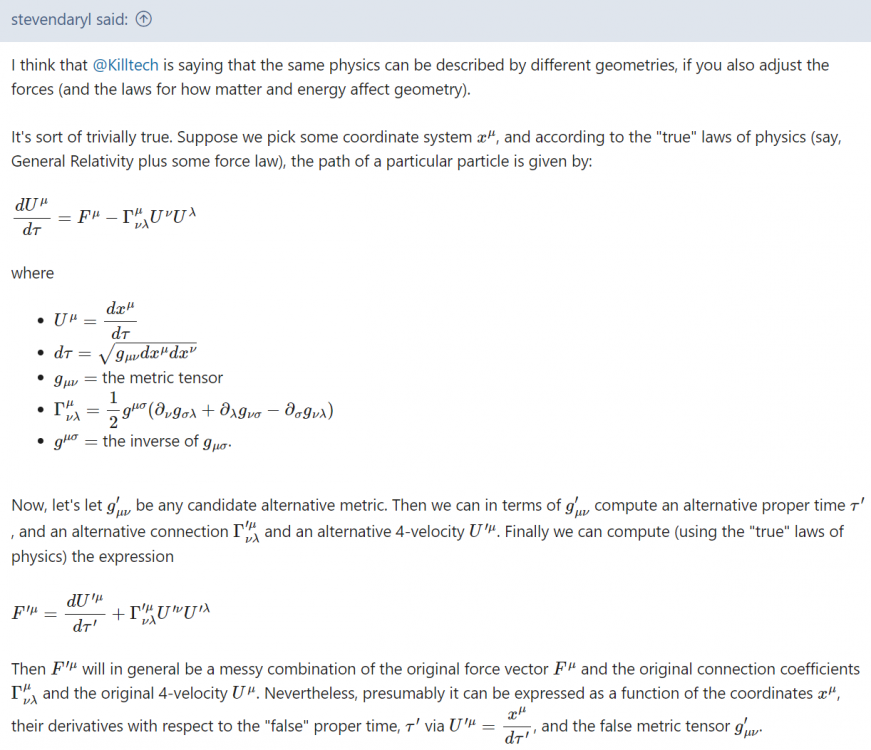
Functions of the form \(e^{ikx}\) are invariant under Lorentz-like coordinate trafos. This is a trivial mathematical observation that applies to any simple wave and their equations. So for an ideal uniform acoustic medium you can apply these and notice that the form of the equation remains invariant. This hints what definition of a time standard we have to use to achieve this in covariant form as well. In general we can use analogue construction of a geodesic clock from GR but utilizing acoustic signals instead of light. For the standard of length its pretty obvious: we just define length by the distance an acoustic wave travels in a fixed time - this is why the speed of sound must become a constant in any circumstance. For either standard we can construct devices that measure them in reality. Now we have discussed extensively what happens to the laws of physics when we do change the standards of time and length. Apply it to this case.

Of course. What i meant is that the definition of the standard for clocks and rulers is itself a significant degree of freedom, as it does not affect any real physics itself. yet massively reshapes the resulting model. I understood you. I am just not sure i would strictly call e.g. the form of Navier-Stokes equations - in their general form - to depend on location or the observers frame. There is also a general form for Maxwell equations under Galilean trafos. What is nasty though it the transformation between frames. But i know what you mean and you are right that in practice when describing e.g. physics in a medium there is always a frame for which the medium is (at least locally) at rest, making some terms to vanish and the equations of interest simplify. Not sure this is the case. For the corrections in questions it may not be possible to experimentally determine them by local means only, but involving global information, this should be possible to do without any knowledge of GR itself. For example the extremal clock signal a radio clock receives compared against a local running clock of same type allows to extract data about the gravitation environment the signal travelled and used that for corrections. Therefore we should be able to construct such u-standard clocks in reality even without the knowledge of GR. But since we do not want to experimentally and empirically discover the laws physics anew, we can deduct the new laws from GR, if we can express the correction in terms of GR. Once we know the equations of motions of all fields and the geometry in the new standard, we technically won't need any knowledge of GR any longer. The enthusiasm is indeed a lot on a theoretical level. because if the standard for time and length allows to rewrite the laws of physics with quite some degree of freedom, we could ask if we can postulate certain laws to have a certain form and then find a standard that makes these laws comply with reality. Applying this idea to Maxwell equation renders it infallible by construction and the nature of c becomes a different interpretation in this concept. Consequently its perfect constancy is a logical consequence independent of physical reality. But this idea seems to works for a broad set of wave equation without dispersion by frequency. So for example, if we take an ideal medium and look at the acoustic wave equations, we can find a time standard that allows to treat it with the same framework as SR - rendering the speed of sound a perfect constant which need to be set and perfect Lorentz invariance but around the set speed of sound. Even if we include regions with a non constant refractive index, in the new standard such local anomalies vanish from the wave equation in covariant form and instead wander into the geometry / are handled by the connection.

I am very well aware of that. The backtracing along world lines is however only relevant when translating results from u-standard model to t-standard experimental data. That is indeed expensive. Yet in the case we do for example numerical simulations of galaxies moving over long time spans, we can stay within one standard without the need for switching. Events like collisions will not depend on whatever time standard and its physical laws we use to calculate them - if they are consistent. This in particular would depend a bit what specific approach would make most sense for quantum field quantization. An isometry to either Minkowski or Euclidean metric would certainly make things simpler. An approach to change the geometry merely moves real physical information between geometric object and fields of similar nature. This is why it is guaranteed to produce an analogue of the Euler-Lagrange equations. But indeed all geometry dependent quantities are redefined by analogues and this of course includes energy and its conservation. I am happy that we finally start to understand each other. I find it very interesting as this shows how much relativity there is any model of reality, simply based on the standards and conventions required to map it into a mathematical description. This mapping is just highly non-unique, non-trivial and very influential in terms of the model it produces. I am aware how tedious this may look in the first place, yet on the other hand this line of thought also adds so much more degrees of freedom to model the very same reality. It also adds quite a different perspective on physics, as it is a lot more relative then one would think. I haven't yet concretized specific corrections. The Schwarzschild example i used was merely to demonstrate that i really want to change the definition of time standard and not just coordinates. For the concrete corrections, i was considering something along the lines of using a coordinate time concept like Barycentric Coordinate Time TCB and uplifted to serve as a time standard, or alternatively a clock concept basing the corrections on radio clocks based on a common specially placed signal source - both ideas are somewhat related. The latter would allow to determine the corrections experimentally, the former gives a recipe how to do it from the model. Either one should allow to find a relation between the regular GR geometry and the corrected one for any scenario, hence this would allow to deduct field equations / laws for all new physical fields from the Einstein field equations. I might note that the way TCB seems to be used in practice for calculation in the solar system is actually not that far from what i intend to do. And there are in fact practical reasons for doing that. It just doesn't go all the way. Weather laws of physics are differ depending on location is a thing of interpretation. state of physical fields and geometry differs by location but the overall laws won't if you keep your definitions of what is a force clean. Even if you would purely arbitrarily add some curvature to a region that is normally flat, in the resulting model it would look like there is some weird dark matter sitting there and messing up physical fields and geometry - yet apart from the concrete distribution of the dark matter in your model, the new laws of physics can retain the same form at all locations - everything just needs some special interactions with the dark matter when passing through. Finally, there is also another case of study for this idea and it goes the other way around - that is where physical laws appear complicated and location depended (in the sense of extra fields appearing), yet could be reduced in apparent complexity if a different time standard were to be chosen. Of course there are good reasons not to, but next time it might be of interest to discuss these situations to understand under which circumstances this is advantageous.

Naively i would think you should be able, since you know how \( a_{\mu \nu} \) changes in length in any direction. I would multiply some scalar function f and then try to find a solution such that \( \nabla_\lambda (f a_{\mu \nu}) = 0 \). Applying the product rule you get the covariant derivative of a as a term, which is known. So at least in the case of 1 dimension, this is a differential equation that can be solved. This gives you an adjustment f a that preserves length along lambda, which reconstructs a part of the metric. Anyhow, i dead tired and probably got something wrong. stupid of me to check the forums just before i was going to sleep.

See, this is unfair. I demand access to write latex formulas... no seriously, how do you do that around here? But i get what you are highlighting. in u-standards muon decay has a dependence on new physical laws and fields that appear in the new standard, hence the naive approach (utilizing classic/relativistic physics only) would indeed require an integration of the now location dependent decay rate along the worldline to get an answer. On the other hand, if u is not some arbitrary time standard but indeed obtained from pure gravity correction, then this question becomes quite a bit more interesting. As far as i am aware we have not much experimental data on if or how particle decay channels may change in strong gravitational environments - nor do we have a theory to make such predictions. But we do know particle decays change in say strong electric fields (think photon e+e- pair production). there is no obvious reason (for me) to exclude such a possibility for gravity. So, in u time standard, the corrections from gravity fields should result in producing some kind of physical 2-tensor field (at least) representing gravity and it should have interactions with both the EM-field and leptonic fields, hence after quantization (which should be straight forward in the flat spacetime u produces), we will get a very different Lagrangian. Around weak gravity fields this new u-standard-G+QED will then have to explain why (u-)gravitons slow down muonic decays. but around strong gravitational fields, i won't dare make any predictions. Maybe this makes my approach appear a little bit more reasonable? Well, in one sense there can be only one LC-connection at a time, as it is unique. But as it is bound to the metric, and we have two distinct ones, the meaning of LC becomes ambiguous. So in oder to keep it clear, we need to say either a g-LC or g'-LC connection. Note that for g-LC connection \( \nabla \) we have \( \nabla g = 0 \neq \nabla g'\). Yet \( \nabla' g' = 0\) using the g'-LC connection \( \nabla' \).

Sorry for the miscommunication and the wrong wording. Didn't know how else to describe it. Highlighting what this interdependence means is my main focus here and what it may lead to. The logical consequences of interdependencies are rarely trivial. Specifically what it means for the isotropy of light and the constancy of c.

One addendum though: in your example a(t) is the acceleration as defined in the previous standard. But if we think of proper acceleration and proper velocity, then those concepts are defined in terms of the proper time. Now as the standard definition of time changes, it reflects directly on proper time tau which is exchanged for tau' in the new standard. This in turn defines a new proper velocity in the new standard. same goes for the proper acceleration. So if the new standards dictates a change from tau to tau', it also requires switching from w to w' for the proper velocity and a to a' for the proper acceleration. And indeed a(t) != a'(t). Only s(t) = s'(t) is unaffected. Note that due to the change in geometry we attribute the same physical information differently between physical quantities and the geometry. So we have a'(tau') = d^2/d tau'^2 s'(tau') != a(tau') Now, where can i find how to use latext here?!?

Yes, this is exactly what i am doing. So far we agree what it means for the model. Now to the experiment: As @Markus Hanke stated the change of standard definition of time makes the laws of physics take a different form, so it is more then a mere change of units. On the other hand it does not change the outcome of experiments directly. In that situation the original experiment cannot be directly compared with the model - at least not without expensive transformations (like you stated correctly before). So we look at how we could adjust our experimental setup to make its measurements conform with the new standard definition of time to make it comparable again. In practice this means exchanging all measuring devices to new ones that adopt the new standard, including mainly clocks. Let's go through this process slowly, because it is fairly important to become aware of what it does. In an laboratory we can measure velocities by taking the time a test body needed from one point to another. A ruler gives us the distance between the points, a clock measures the time and the velocity is the quotient of both measurements. Now if change to the new standard where the clock in the laboratory have correction factor f(x) (which we assume is nearly constant in the area of the laboratory for this example), then this will result in obtaining a different numerical value for the velocity then in the original experiment, i.e. by a factor of 1/f(x). Similarly we can measure the acceleration as a change of velocity over time and with adopting the new standard we obtain a result differing by a factor of 1/f(x)^2. So if we were to measure the Coulomb force a charged plate capacitor applies on charged test bodies, we observe a slightly modified law Coulomb law, one that has an additional factor 1/f(x)^2 in it. Performing the same experiment in a different laboratory where the correction factor has another value f(y), we get observe the Coulomb force is affected by the physical field which we correct via f(x). However, as you stated yourself due to the change of the standard definition of time, the laws of physics take on a different form, and so this experimental outcome will be consistent with that. If our experiments uses the very same standards as our model assumes, we will not be able to create any testable violation between reality and theory. This is what is important for me to highlight. I am sorry to correct you here but that statement is false. On a smooth manifold, you can have a connection but without the metric you cannot classify it as LC, only as torsion free. There are many torsion free connections on a smooth manifold and they differ by what they do to different metrics. If you whish to contest me on that, then please provide some literature to back up your statement. I think you a mistake smooth manifold for a Riemann manifold, because then your statement would align with the fundamental theorem of Riemann geometry https://en.wikipedia.org/wiki/Levi-Civita_connection#Fundamental_theorem_of_(pseudo-)Riemannian_geometry. Two metrics which are not isometric towards each other produce different LC-connections.

Arg, no, that is not what i was saying at all. You misunderstand me. I am interested in exploring the definition of SI second and meter and how they affect measurements made by experiments, the laws of physics those experiments perceive when using those standards and finally how that shapes the entire physical model that we use. Also Einstein uses in his gedankenexperiments a lot of clocks, yet this object is never actually defined or meaningfully explored. Implicitly all his considerations assume there is only one concept of time, which all real world clocks have to measure. But there is more then one concept of time and of course we can build other real world devices that measure them (though maybe with less precision). But if we are not willing to even consider hypothetically what were to happen if we used those instead for all our current real world measurements, we become blind to how much the SI system and its standards for measurement determine. We won't be able to see that the invariance and shape of Maxwell as seen by experiments and theory is a logical consequence of SI standards and postulates underlying it. And because they determine both measurement and model in the same way, there is no possibility to create a violation. They cannot be proven wrong, as they are a convention. And we get stuck in using only a singular interpretation of spacetime which just isn't practical for a lot of problems, mainly in theoretical physics. Issues like failure to establish quantum gravity or the huge amounts of dark matter we need to postulate to make GR conform with observations may be related to this. Not sure if the latter two instances you do consider practical problems though. Let me be clear, if we just discuss calculating satellite orbits around earth, all the considerations discussed here are entirely useless. Only when we look at problems of galaxy rotation anomalies, or the issues of describing the quantum interactions in a black hole, things start to look differently. It is not like we can put a Caesium clock inside a black hole to get 'real world measurements' from it (that's like talking about invisible pink unicorns). Generally I am not convicted the SI standards are well definierend for that scenario. Maybe you can only describe the situation in there by extrapolating a definition of a hypothetical clock device that corrects for all gravity effects. Ah, this is crucial information and now i unterstand your prior statements. But your are using the diffeomorphism very differently then i do. Now a diffeomorphism can pullback (https://en.wikipedia.org/wiki/Pullback_(differential_geometry)) all objects defined on manifold, be it points, vector fields, metrics and even a connection. If you do that, the target manifold becomes really the original one as the diffeomorphism is used almost like a map itself, so this is almost a mere change of coordinates. But if we have two metrics, this doesn't help us at all, because such a diffeomorphism phi, the pullback metric g(phi) is not g' and same holds for the connection. Therefore in my case it makes no sense to apply the diffeomorphisms to any features linked to the geometry. We can only apply it to the points of the manifold but not even to the vector fields. And this is why: Imagine you have clocks that correct SI atomic clocks for some effects and you use those in your measurements. One way to measure a force, it to let it affect test bodies and measure their acceleration, which is by its units is space / time^2 and therefore have a direct dependence of the spacetime geometry. Any corrections made to the time measurement standard will therefore change the outcome of the measurement, as the acceleration measured using the corrected clocks is different and hence the force itself. In SI we can see the dependency of all quantities on the spacetime geometry right from its units. So no, a change of metric does not keep even vector fields invariant as due to the change of perspective those are related yet actually different objects. So we need to look a bit closer on the toolset of diff geo what it provides to handle this situation. So first let's have a look at the definition of a Riemann manifold (M,g): https://en.wikipedia.org/wiki/Riemannian_manifold#Definition - it is always a pair of two things and one is the defining metric. So if we have one smooth manifold M but two metrics on it, we have formally two Riemann manifolds (M,g) and (M,g'). When it comes to the connection, specifically Levi-Civita, we know that it is only defined on Riemann manifolds, as by definition it is explicitly defined via the metric: https://en.wikipedia.org/wiki/Levi-Civita_connection#Formal_definition. So two metrics, two Riemann manifolds, two LC-connections, only one smooth manifold M. When we use diffeomorphisms to transition between Riemann manifolds, we are no longer allowed pullback any objects that depend explicitly on the Riemann geometry. This situation is a part of diff geo that i haven't seen being used in physics, hence it won't be familiar for physicists to think in this way. Only distantly related, as it discusses using an alternative geometry and maybe the flatness, but everything else is based on a different concepts, hence resulting in quite a different setup.

You misread my statement or i do not follow you here. A piece of a sphere is diffeomorphic to a flat space of R^2 (calling the former a sphere and the latter flat does imply that i talk about more then there mere differential manifold). The coordinate maps themselves provide such a diffeomorphism (at least for piece of the sphere there is no problem with map singularities at the poles). So with the regular connection you have on the sphere you get a parallel transport like this: https://en.wikipedia.org/wiki/Parallel_transport#/media/File:Parallel_Transport.svg. On the flat R^2 you will not find a path where such a twisting will occur because it comes with a different connection. But of course you can pullback your connection from the sphere piece via the coordinate map to get a connection on the flat space which will also twists your parallels - and basically reinterpret the flat space as curved. Think of a balloon painted as a globus. Also paint the coordinate grid on it. Usually a balloon is spherically shaped. But we can cut a piece out of it, stretch it into a flat surface and pin it on the wall. All points of interesting like Greenwich, the Cape Town and Katmandu will remain at their locations as described via coordinates. But let's try a parallel transport between Greenwich, the Cape Town, Katmandu and back to Greenwich - in the original state and after the flattening. Apparently it now uses a different connection. Yet the piece of the balloon is the same smooth manifold regardless how it is shaped - it remains the same set of points and how they are linked with each other. Diffeomorphism, or more generally homeomorphisms is what we use in diff geo to reshape a geometry without changing its topology. You know, like a coffee mug is homeomorph to a torus (https://en.wikipedia.org/wiki/Homeomorphism#/media/File:Mug_and_Torus_morph.gif). The diffeomorphism will additionally make sure the reshaping does not produce any kinks or folds that would cause issues for differentials. But a diffeomorphism does not preserve the geometry, for that you need stronger things (https://en.wikipedia.org/wiki/Isometry#Manifold). In my case i have only one set of coordinates and never change it, because coordinates and equations purely expressed in terms of them are one of the few thing that remain unaffected under a reinterpretation of the geometry. Now imagine the balloon not to be made out of a simple fabric but its surface being a futuristic display that displays live physics interactions that happen on the globus (think of a live meteorological satellite stream). The physics displayed on that surface will have no idea about what kind of stretching and reshaping we did with it and act entirely independently from that. All the reshaping may however let the same physical interactions playing out on the surface appear to to subject to different laws of physics with things moving faster in the stretched regions and slowed in the contracted ones. Also our coordinates as originally painted as a grid onto the surface do not notice any kind of reshaping we do - they only look different for someone outside their world looking at the manifold. Yes, this you are absolutely right. When we stretch the spacetime geometry onto the flat, gravity must at least become similar to electromatic field which is a 2nd degree tensor. So unlike Newtons gravity there must be at least something like a gravity analoge of the magnetic field when represented in a flat spacetime. Probably even more then that. We have exchanged the clocks and rules and use those for our real-world measurements instead. Think as if you were forbidden from even using SI conforming clocks and rules and are forced to rely solely on the new ones for all experiments - i want to you understand what this situation mathematically means (not practically). In that case i don't have to do any additional mappings: because as you said, the correction we did coupled with the mapping back (which is the inverse) combined together yields f ° f^-1 = id. No additional work needed if i simply forget the old word entirely, both in terms of the the model and measurements in the real world.

But what does that even means "behave naturally as clocks"? Naturally i could just interpret the situation another way: Lets say I don't know much about modern physics and naively start my exploration of the world with radio clocks instead. those don't need any corrections and in a sense they do tick at the same rate regardless if they are in deep space or close to a black hole. If i compare their time with that of SI atomic clocks, i notice that those run inconsistently - and i can figure out a physical reason to attribute that effect to: gravity. Furthermore i can make the atomic clocks consistent to my radio clocks, if i were to correct them for that effect. Now if i have two clock types, ones that give me different times at different regions and another that show consistent results, which clock seems more like the natural choice for time keeping? If i was to run space ports around the universe, which kind of clocks would i announce my flight schedule with? Just to throw that in reminiscence of how connecting towns with the rail and trains required to make clocks at different towns to run consistently, i.e. introducing the railway time. It really depends on your perspective which clocks you declare to behave naturally. But yes, radio clocks have a significant drawback: they always have a preferred frame and that is that of their signal source. In empty space there is no natural choice of a preferred frame - and as you say eta and h there is some arbitrarily there. I am not sure if the presence of gravity may induce some kind of preference though. If you think of Poincarés corrected LET but now expand it with gravity deducted from GR - which is more or less what this approach leads to - it would require addition of density + current for the aether along with a flow equation. The choice of h would impact its form since it mostly would describe the aether, thus you would look for what makes its time evolution equation most natural. wait, we can use latex here!?! how?

Diff geo conforms with the general definitions of metric spaces, it's just the we have better means to represent the metric. But just to be clear, a diffeomorphism (see wiki definition) without any further specifications is just a bijective function which is smooth in both directions. A piece of flat space is diffeomorphic to a piece of a spheric surface - and i want to stress those are in no way unique. These spaces will have different connections though. Do you mean a situation where your diffeomorphism pulls back the connection, i.e. the connection of the target space can be expressed via the connection of the source space? Hmm, it's really neither of those two, though it is much closer to 1 except that your interpretation how this maps to reality/experiment might differ from mine. In a way you could call it a different physical situation with different laws of physics which despite this produces equivalent outcomes. We need to work on the interpretation of this. Lets go back to how we interpret GR and how we compare it with reality/experiments. More specifically, how does the spacetime show itself in reality? If we agree that the metric is a simple tool in our model to describe the spacetime, then there are a few things we can obtain from it that directly map to objects of reality, like the proper time maps to what clocks measure and proper length what rulers show. And there are also angles. And does this mapping work the other way around as well? i.e. given the tools like clocks, rulers and goniometers (for angles) is that enough to reconstruct the spacetime with its metric? So is this mapping kind of bijective and in some way smooth? ... can we treat this interpretation mapping between model and reality almost like a diffeomorphism itself, one pulling back a metric? What would happen to this line of thought if we exchanged the tools we use in reality (clocks & rulers) for a different set of tools wich additionally apply a correction for gravity? Let's assume the type of correction is such that these tools do fulfill all conditions to construct a mathematically valid space and metric. More specifically, lets assume they construct exactly the g' metric from my previous example in the Schwarzschild case. The benefits are hard to see from the tedious construction, but actually there are multiple reasons i am looking into this. But maybe I could throw in an appetizer for a potential silver lining: the quantization of gravity would greatly benefit if there existed a method to equivalently model GR physics in a flat space where gravity arises as a force. But i do not want to discuss such ideas further for as long as we don't have a common understanding. Lets forego that the definitions of one iteration second and meter were made such that to be consistent with previous ones, so that the original definitions weren't chosen with such a purpose in mind. But lets go to Einstein. He postulated c to be invariant and with it Maxwell. A postulate is just as much a definition, and so it was set as invariant. The definition of the meter was derived in parts on this idea. Lets not focus how this postulate was obtained but rather what the direct consequence of such a definition is. Lets just consider what would be if light somewhat tried to travel at different speeds (and for some reason no one catches it). even the prior part of the sentence already causes a lot of contractions with the meter. Because we measure distance in units of light travel time in vacuum. So we practically would try to measure the speed of light in vacuum in units of the speed of light, so we measure it moves at 1 c. It is by all means a tautology! It can neither logically deviate nor create any contradictions with reality. its just how we measure stuff. If light traveled slower in some region, it would cause Maxwell to look differently, but since the lengths that we use become shorter, plugging in the effect restores Maxwell to its well know invariant form. All effect you will see instead is that space will get a dent around that region - which is no contradiction with GR, since even for the worst case of a missing explanation we have dark matter. What this does it that whatever the reality is, the definition of meter and second guarantee that Maxwell in vacuum is invariant. At the same time there is nothing in this statement capable to produce a conflict with reality. For the meter, this tautological construction with SR is easy to see, but for the second it is far less obvious.

I think we are getting close to an understanding, yet I do not entirely follow how you use the term metric and what it means to you two of them. We know that the Christoffel symbols of a Levi-Civita connection can be expressed via the metric, i.e. the metric fully specifies all components of this special connection. That means that if we have two metrics, each produces a distinct LC-connection and those will not agree with each other in the general case. As for metric equivalents, i used the term they way i know it from metric spaces: https://en.wikipedia.org/wiki/Equivalence_of_metrics But actually, i should have said if the metrics are not isometric, that is the identify id:(M,g) -> (M,g') is a diffeomorphism but not an isometry. I think it's best to try an example to make things concrete. So let's start with the Schwarzschild situation: Let (x0,x1,x2,x3) = (t, r, theta, phi) as a usual choice of spherical coordinates, Let g be our regular metric associated with the standard clocks and rulers (SI second and meter). So in these coordinates we have a diagonal with g00 = -(1 - rs/r) g11 = 1/(1-rs/r) g22 = r^2 g33 = r^2 sin^2 theta The LC connection is given by Gamma^i_kl = 0.5 g^im(g_mk,l + gml,k - g_kl,m) (i use the comma notation for the derivatives) Lets assume the proposition of a alternative clocks and rules that correct for all gravitation effects. Lets g' be a metric associated with these and written in the same coordinates it is: g'00 = g11 = 1 (why not make it Euclidean if c is not preserved here anyway) g'22 = r^2 g'33 = r^2 sin^2 theta Now the Christoffel symbols Gamma' of this connection obtained from g' metric via the analogue formula. It is well known since these is the spherical coordinates of flat space. Obviously those two connections are different and disagree. However each is a Levi-Civita connection for its associated metric. And if we leave this as it is, it will break physics, so we have to do the real work. This is where we apply the scheme I quoted here: https://www.scienceforums.net/topic/135672-the-meaning-of-constancy-of-the-speed-of-light/#comment-1286826 We start at looking at the laws of physics. In the original space there were no extra forces needed as curvature did all. The new space with metric g' is however Euclidean, so it cannot work the same way. We look at the geodesic equation of a particle world line in free fall and try expressing it with the new connection - and we find not all terms can be expressed with the new symbols. The left-over terms have to be interpreted as a now appearing force resembling something like a Lorentz force in structure - thus quite a bit more complex then Newtons gravity. Yet any solutions expressed in terms of coordinates will by construction be the same as the original - we more or less just renamed/reinterpreted the terms, what is a force, what is the geometry/connection and all that. On that basis predictions stay the same, though doing all interpretation based on coordinates alone is nasty. When we fully want to compare the results with experiments, then these must be now preformed with clocks and rules corrected for gravity effects (conforming g') - because the laws of physics are now rewritten to have different invariances - which are based on the alternative second' and meter'. So we get an entirely different physical description of the situation, alternative laws of physics and a different interpretation yet all combined together still produce the same predictions. Think of it this way: the definition of the SI second defines the Caesium transition frequency as invariant (which is entirely electromagnetic in origin). Everything that behaves the same way, will become invariant with it. The SI meter defines c as invariant. With both the temporal and spatial part of the EM-waves set as invariant, Maxwell equations obtain their invariant nature. In this situation, experiments cannot even logically violate this part of the model, as any disagreement with the prediction can be interpreted as a failure to correctly implement the SI standards for time and distance measurement rather then a flaw of the laws. I suspect isotropy of space is purely a convention. Experiments cannot verify or disprove conventions. Conventions can be assessed in terms of practicality but not if they are right or wrong. What would an experiment be looking for to disprove the isotropic nature of space? The issue i raised with this thread about c is that a counterhypotheses cannot be properly formulated for experiments to check.

Nooo... i lost my post when i accidentally clicked the go back button on my mouse.... i suppose there is no cache i could get my post back? ugh.. Sorry, i missed a crucial world. We have two Riemann manifolds build on the same smooth manifold. A smooth manifold give you differentiable maps, that is enough to define affine connections and play with coordinates but not much else. A Riemann manifold expands this by defining a metric. This allows us to define things like curvature and also Levi-Civita connections only exist in this context as they are defined relative to a metric which they preserve. Every Levi-Civita connection is also an affine connection and in this sense we can look at it from the perspective of both Riemann manifolds and their metrics. If the metrics are not equivalent, then the LC-connection of one metric cannot simultaneously preserve the other metric. Now as for time- and space-like, these words are defined in terms of the metric, i.e. specific to the geometry. Remember X is defined as time-like if g(X,X) < 0. But if we have two non-isometric Riemann manifolds, then there can be X such that g(X,X) < 0 < g'(X,X). So if c changes, it is not because it changed but rather because c' ist not the same thing. And you are right in your suspicion that i use a different parametrization of world lines. However, this is how the situation looks when you stay on the original Riemann manifold. I do intend to treat the parametrization as a proper time - and for that i need a metric g' which produces this parametrization as proper time via the analogue formula. I think faster then i can type, so this leaves me often skipping crucial details. I am sorry for that. Thank you for bearing with me so far. Indeed, losing the isotropy of space is what is required, and yes, this has to end up in an aether-like physical model. The math reflects this then when we try to rewrite the Maxwell equation as it given with the usual connection of GR into another connection. If the new connection does no longer preserve the original metric (but some other), then it becomes a lot harder to express the Christoffel symbols of one connection with those of the other. In fact we need to introduce additional fields to be able to do that, one such field is c(t,x). But the geometry has a lot more degrees of freedom - 10 come from the metric tensor, so there are a lot of more fields that come out of such a transformation. A general formula cannot be written down without specification how the two metrics and their Levi-Civita connections relate to each other. That would needs a concrete proposal how to adjust the definition of the second (i.e. what clocks to use) and that i am not sure of yet.

Okay, i see a lot of misunderstandings here. I admit what i am suggesting here is so far from the usual of what is done in physics thus is quite natural to happen. But i understand where you are coming from and think we can work it out, if we both try to understand each other. You mention choosing a different connection - and that's what i am doing here. however in GR all such things are done only on a single Riemann manifold, and not between two different ones. This is why you state that we can exchange curvature for torsion and that is correct. In my case i utilize the very same underlying smooth manifold for both geometries (hence coordinates remain the same in both), each is equipped with a different metric tensor. And i do not mean rewritten in different coordinates. L1 and L2 are two disagreeing concept of clocks. I know the concept of coordinate time but this is not what i am referring to. In my case both L1 and L2 are associated each with the temporal part or their own metric tensor g and g' (g_mu,nu != g'_mu_nu in general) - since they represent the proper time of their geometry. For now i left out the discussion of what happens to the remaining complements of the L2 metric tensor - just assume it is constructed suitably. Given a metric tensor, there is a unique torsion free connection associated with it, the Levi-Civita one. We have two metrics, hence two manifolds, hence two LC-connections and each is torsion free on its manifold. On the other hand, since both connections are defined on the same smooth manifold, we can look at the L2 LC-connection from L1 geometry and will notice that it neither won't be torsion free nor more importantly it won't even preserve the L1 metric. The latter renders it quite easy to make c a function of something. I am not sure you are familiar with dealing with such kind of connections. L2 will break the usual parallel transport. It will yield different geodesics. It is entirely a different geometry. Yes, yes i hear all your alarm bells ringing - i know such a thing would normally break all laws of physics. But it is though to be used in accordance to constructing clock and ruler devices that conform to the L2 metric. And if we have both, then it is in fact just like you said, draw different types of maps over the same territory. To stay in your analogy, our maps are good for those that navigate via vision, but for bats that navigate via acoustics, a very different type of map may be needed. Not very revolutionary at all. And sure, so far mathematically this is still kind of trivial, even if it is used in an unfamiliar way. Indeed such a construction appears anything but practical for now. However, we are talking of what would happen if we were to exchange the clocks and rulers we are using in the real world for something else that is not equivalent. I do not mean just using different units. I mean changing such fundamental relations like A longer then B becomes A shorter then B - simple change of units cannot do that. A change of geometry can. Before you object that only one of the statements can be 'real', consider that in math we do often use different metrics or norms on the same space and they will disagree with each other without there being an issue. The reality is that there is more then one way to compare things. The gist of it the definition of the SI second chooses a very specific way to compare time, yet it is by no means a unique choice.

In my case the basis for the change of laws of physics is the consequence of switching between different clocks concepts with which observations and measurements are performed. Thus the change is not physically real, but is a pure result of change of conventions / definitions. Lets consider two particles Pa and Pb modelled in GR with different initial conditions but starting at the same space time location. Lets call this event S. Each particle follows a trajectory dictated by the laws of physics. Lets assume the trajectories are such that they eventually collide. Lets call this event E. Now lets consider two different clocks being placed on each particle, one is the regular SI clock type L1, the other a clock type L2 that corrects for local gravity field. the first clocks describes the regular proper time of GR for each particle, the other clocks provide another proper time which will disagree with the former. Let's assume that the flight time between S and E as measured by L1 clocks shows that Pa needed less time then Pb. Now L2 clocks tick rate will locally differ from L1, specifically let's assume Pb passes through a region where its L1 clock runs slower like a large mass. L2 clocks will adjust for the effect thus lets assume according to L2 clocks Pb needed just as long as Pa. Since L1 and L2 are different measures, there is no contradiction in that observation. In terms of Riemann geometry we can describe the equation of motion for each particle in either L1 or L2 proper time. However, L1 and L2 come with two different (1,3)-Riemann manifolds, metrics, and hence a different connections. These are different geometries. Expressing the very same physics in terms of two different connections will make the same laws of physics look entirely different for each. And they need to be in order to yield the seemingly disagreeing observations of L1 and L2. Yet their predictions, like the resulting trajectories and where they collide will still perfectly agree. This is what the transformation in the quoted post below does

Why would it not be a wave equation? How are Maxwell equations in a medium not wave equations since the speed of light in mediums does vary and is related to the refractive index? How are sound wave equations not wave equations? I fail to understand what you are trying to say. Also i am not transforming between frames. This has nothing to do with coordinates. It is far more fundamental then that. We are transforming between two concepts of proper time. Look at the formula. That is far more involved then a change of coordinates or something as trivial as switching between SI and natural units. Natural units still use the very same definitions of time and space as SI does but just scale them differently with some factors. But a change of proper time is like a local transformation of units. It forces you to rewrite all laws of physics. It changes what is constant and what isn't. Look at the equation and understand how massively it impacts the whole model but not the physics.

you forget an important aspect in your example. While the laws of physics will remain invariant under alterations of the iron-to-iron interatomic distance, the geometry of your space won't be. Lets for example assume the alteration only affects the distance in a local region but not around it. In the case we will measure that this region has a different volume then it would have it it were flat space, no? Therefore the effect is measurable even when using this specific distance definition but realize differently then one might naively think. to make your example more concrete, we can use a simplified temperature model as a factor to alter interatomic distances with the special assumption that our iron ruler always has already taken the environmental temperature before we use it to measure anything (and lets also assume there is no kind of hysteresis effect which would disqualify it from defining a valid distance - as needed for the mathematical definition of a metric space). In this example temperature will change geodesics, i.e. what the shortest path between two points is. Now coincidentally sound waves will follow these geodesics (under ideal assumptions)... therefore the sound wave equation would suddenly become invariant under local changes of temperature.

assume c(x,t), i.e. it may vary by time and region A simple approach for Maxwell with non constant c derived from current physics: SI second definition explicitly states that corrections due to the local gravity field should not be applied. Now define an alternative time measure and clocks with corrections for the local gravity field such as to enable clock in different regions to tick with the same rate. As clocks define the proper time this condition can be translated as to finding a correction such that curvature due to energy-mass vanishes. Defining a new clock and unit of time is so far a change of conventions, therefore does not affect real physics. However it changes measurement (and all SI units depend on the second) and the mathematical model of physics. Now transform maxwell equations from GR proper time tau to new proper time tau' and you get additional time and region dependent terms, one of which describes the wave speed and this we call c(x,t). These new terms reflect the physical degrees of freedom which GR describes via geometry. As a trade-off all physical degrees present in GR are gone, as it now becomes flat by construction. More specifically you do this: in short, the effect of this transformation is that curvature of space time transforms Maxwell into a wave equation in a medium where the medium is just an equivalent representation of what was previously embedded in the geometry. EM-waves represented via tau' now bend in vacuum because it gets a refractive index replacing curvature. it still the same physics, just a different mathematical model that yields the same predictions. To measure alpha, you need to do measurements and all those measurements use SI units, all of which depend on the second. While alpha has no unit itself, it still depends on the geometry of space time and since the definition of the second does define proper time, the geometry is a convention in this context. So no, in SI units alpha can never change, but other units that do not inherently assume c to be constant do allow it to change. You are exactly right, and so far you are the first person i meet that understand this. It however means that the constancy of c is more of a convention of our physical model and specifically our concept of measurement rather than fundamental law of physical. It would also make us free to change that convention, if there was a use case where a different convention would be more beneficial, no?

The constancy of the speed of light is a fundamental assumption in modern physics, built into both relativity and the SI system of measurement. I’ve been wondering: to what extent is this a fundamental property of nature, and to what extent is it a convention tied to our choice of units and measurement definitions? And does our current measurement framework even allow us to establish the possibility of it to vary in the first place? The Issue of Measurement The SI second is defined using atomic clocks based on the frequency of a cesium transition. The meter is defined in terms of the speed of light, which is fixed at 299,792,458 m/s by definition. Since c is numerically fixed, any potential variation in light’s speed would be hidden within changes in how we measure time and space rather than appearing as an explicit difference in measured speed. A Need for a Counterhypothesis To test for a varying c, we’d need a physical framework where such variation makes sense and is not simply reabsorbed into our measurement definitions. But how do we define an operational way to measure a changing c, when our time and length units are already tied to its assumed constancy? The Variation of c The fine-structure constant alpha is given by: e² / (4pi epsilon0 hbar c), meaning that if c varied, so would the constant. Since the energy levels of Caesium atoms - and thus atomic clock frequencies - depend on alpha, any variation in c would affect the very clocks we use to define the second. This creates a self-referential issue: if we use atomic clocks to measure changes in c, but those clocks themselves change due to variations in c, can we even establish whether c is varying in the first place? If c depended on location, it would cause clocks in different regions to tick at different rates. Since clocks define proper time, this effectively means that a variation in c would manifest as spacetime curvature. How, then, would we distinguish such an effect from gravitational time dilation caused by mass-energy? Back around 1900, Poincaré already recognized the subtleties in these assumptions and criticized how astronomers arrived at their conclusion that c was constant. In modern physics this consideration is even more interesting to explore. I tried this conversation with ChatGPT, and it was well versed to discuss the topic. Here is a link to that conversation: link deleted; violation of 2.13 I’d love to hear thoughts on whether this is a meaningful issue to explore.

Mass is a thing of reality, while energy is an abstract concept of our description of nature, that reality does not need to care about. the concept of energy arises conservation laws which are a consequence of symmetries, which again are arise from how we define time and space relative to natures laws. different definitions of the latter lead to different deductions of the former. Einsteins relativistic notion of space time (specifically his clocks) leads to the relation you mentioned, but if you were to use an absolute space time defined by a coordinate time and space and took the symmetries it is subject to, you get a coordinate energy unit with different relations. energy is an extremely useful tool for the calculus, but it is not real in the same sense as mass, space, time or change, which all come with their own unique units expanding the degrees of freedom of the universe, while the deducted quantities like energy, (angular) momentum all have units assembled from those fundamental ones and describe the constrains of the world.

F is a function of coordinates x and t, i.e. F(x,t) whereas F' is a function of coordinates x',t' i.e. F'(x',t') please look up how a change of variables works in math: https://en.wikipedia.org/wiki/Change_of_variables_(PDE) . the wiki article on Galilean invariance also explains it for the specific case of Galilean transformation. i really recommend you to read and understand it first. what you have written is a mixed picture using functions of old and new coordinates. you did not correctly/fully exchanged the variables.

lets take a combination of a uniform motion plus a translation like x′=x+vt+x0 t′=t+t0 then \(\frac{dx'}{dt'}=\frac{dx}{dt}+v\) and \(\frac{d^{2}x'}{dt'^{2}}=\frac{d^{2}x}{dt^{2}}\) therefore \(F'=m\frac{d^{2}x'}{dt'^{2}}\) you can go through the other elements of the Galilei group and check it remails invariant. EDIT: shouldn't have bother to write it down myself. here you have it on wikipedia, the Galiean relativity . also latex \frac breaks on editing

coordinates are needed to under the hood almost everywhere in physics. this is because in order to define differentials they are the minimum requirement. and even the coordinate free formulation of equations in geometry isn't entirely free of coordinates, because it still requires smooth manifolds to define them which in turn use atlases of coordinate maps for their definition. i am not sure i understand what you are trying to imply here. there is absolutely no physical phenomenon which depends in any way on your choice of coordinates. there cannot be, coordinates only affect the calculus and are entirely independent of physical predictions. the only thing that depends on the choice of coordinates is the length of your calculation needed to make a prediction - the result does not. Look up the Noether theorem. it's pretty standard. Specifically look up how (classical) energy conservation is derived in classical mechanics. the translation symmetry is needed to deduct the momentum conservation, while rotation symmetry gives you conservation of angular momentum. i hope you do not question those in classical mechanics? Show me what your textbooks says, so we can clear up the misunderstanding.

what you refer to is the geometric formulation using the metric. i covered that in the response above and saw no need to repeat. Classical mechanics are invariant to translations, uniform motion and rotations, the Galilean group. Noether uses that to deduct the corresponding conservation these invariances imply.