KJW
Senior Members-
Posts
480 -
Joined
-
Last visited
-
Days Won
3
Content Type
Profiles
Forums
Events
Everything posted by KJW
-

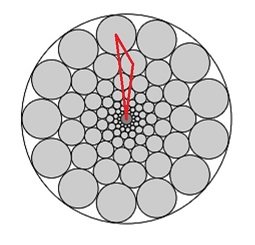
Proportion of the area covered by circles
KJW replied to Dhamnekar Win,odd's topic in Applied Mathematics
Not stated but used in the solution is the series expansion of [math]\cos \phi[/math]: [math]\cos \phi = 1 - \dfrac{\phi^2}{2} + \dfrac{\phi^4}{24} - \dfrac{\phi^6}{720} + \cdots = \displaystyle \sum_{j=0}^{\infty} \dfrac{(-1)^j}{(2j)!} x^{2j}[/math] For [math]\phi \approx 0[/math], the terms after [math]\dfrac{\phi^2}{2}[/math] can be ignored, and the closer [math]\phi[/math] is to [math]0[/math], the better [math]1 - \dfrac{\phi^2}{2}[/math] is as an approximation of [math]\cos \phi[/math]. In the limit of [math]\phi \to 0[/math] ([math]n \to \infty[/math]), the approximation is effectively exact. But [math]\phi[/math] can't be exactly [math]0[/math] ([math]\cos 0 = 1[/math]) because then the ratio of the total area of the small circles to the area of the large circle in the limit of [math]n \to \infty[/math] becomes indeterminate. Thus: [math]\cos^2 \phi = (1 - \dfrac{\phi^2}{2})^2 = 1 - \phi^2 + \dfrac{\phi^4}{4} = 1 - \phi^2[/math] for [math]\phi \to 0[/math] (ignoring the [math]\dfrac{\phi^4}{4}[/math] term) [math]2(1 + \cos \phi) = 2 + 2(1 - \dfrac{\phi^2}{2}) = 4 - \phi^2[/math] for [math]\phi \to 0[/math] Also, solving the quadratic equation involved carefully ignoring the higher order terms, leaving only the term that is linear in [math]\phi[/math]. -

Proportion of the area covered by circles
KJW replied to Dhamnekar Win,odd's topic in Applied Mathematics
I don't know where you got those numbers from, but have you interpreted [math]\alpha (R-r)[/math] as [math]\alpha[/math] as a function of [math]R-r[/math] instead of [math]\alpha[/math] multiplied by [math]R-r[/math]? [math]\alpha[/math] is the ratio of the radius of the second outermost circle to the radius of the outermost circle. It is also the ratio of the distance between the centre of the large circle and the center of the second outermost circle to the distance between the centre of the large circle and the center of the outermost circle. Thus, [math]\alpha r[/math] is the radius of the second outermost circles, and [math]\alpha (R-r)[/math] is the distance between the centre of the large circle and the center of the second outermost circle. I chose to define the ratio [math]\alpha[/math] rather than the radius and distance themselves. Much of the solution was about finding the value of [math]\alpha[/math], and even then, only for very large values of [math]n[/math]. It should be noted that the same value [math]\alpha[/math] describes both ratios because the second and subsequent outermost layers of circles are scaled down versions of the outermost layer of circles. -

Entanglement can be demonstrated by measuring the spin of a photon
KJW replied to Paulsrocket's topic in Speculations
I know it's off-topic, but I feel the need to say that the message of the article is for people to keep up-to-date with their COVID-19 booster shots, not that the COVID-19 vaccines do not work! -
The outer event horizon would expand in accordance with the extra mass of the infalling black hole, but the inner event horizon would remain intact as the boundary from which light can't even escape to inside the outer black hole.
-
The Proof of the 3X + 1 Conjecture
-
I have read the suggestion that the universe, represented by the Friedmann-Lemaître-Robertson-Walker (FLRW) metric, can be regarded as the time-reversal of a collapsing matter black hole. I don't know if this is true, but I do see it as plausible. It should be noted that the radial coordinate of a Schwarzschild black hole is timelike to an observer who is inside. Thus, to an observer inside the black hole, the singularity is not located anywhere in space, but in the future. Also, the FLRW metric is conformally flat, which is exactly not the spacetime of a Schwarzschild black hole. But the interior of an infalling matter black hole is not a Schwarzschild metric and may be more like the FLRW metric. A black hole can exist inside a larger black hole. Given that nothing special happens when an object crosses the event horizon of a large black hole, it is reasonable to conclude that a small black hole can cross the event horizon of a much larger black hole completely intact.
-
Considering the Schwarzschild metric, there is no upper limit to mass, and therefore no limit to the size and how close to zero the density can be. However, there may be limits at the cosmological scale. For example, I doubt that a black hole can be less dense than the universe as a whole. If the region surrounding a black hole is denser than the black hole, then the total mass of the black hole and the surrounding region would be large enough for the surrounding region to be also inside the black hole.
-

Proportion of the area covered by circles
KJW replied to Dhamnekar Win,odd's topic in Applied Mathematics
-
It's worth pointing out that although for a small black hole, the tidal forces may be so intense as to cause "spaghettification" well outside the event horizon, for an extremely large black hole, the tidal forces may be so weak that one might not even be aware that one has crossed the event horizon. Also, black holes have a peculiar property compared to ordinary matter: the mass of a black hole increases linearly with respect to radius, in contrast to the cube-power for ordinary matter. Thus, extremely large black holes have very low density. One only needs to accumulate enough matter into the large volume to form the large black hole... one doesn't need to compress anything.
-

problem with cantor diagonal argument
KJW replied to phyti's topic in Linear Algebra and Group Theory
No. This is what Wikipedia says about the use of "arbitrary" in mathematics: This is different to "random", which is associated with chance events and probability, neither of which apply to "arbitrary". Are you talking about finite lists of finite sequences, or are you talking about what Cantor is talking about... infinite lists of infinite sequences? It seems to me that you are talking about finite lists of finite sequences, in which case, you can't argue against Cantor, who is talking about infinite lists of infinite sequences. If you are talking about infinite lists of infinite sequences, then you are not taking into account the infinite nature of the sequences and lists. -

Proportion of the area covered by circles
KJW replied to Dhamnekar Win,odd's topic in Applied Mathematics
With the solution to the second problem in place, I decided to provide a solution to the generalised intermediate version of this problem, where the first and second problems are the two extremes. Because much of the solution to the second problem is unchanged in the solution to the generalised problem, I shall only include the parts of the solution that have changed. Let [math]\sigma[/math] be a parameter, [math]0 \leqslant \sigma \leqslant 1[/math], such that [math]\dfrac{\sigma \pi}{n}[/math] is the angle between the line joining the centre of the large circle to the centre of the outermost circle and the line joining the centre of the large circle to the centre of the nearest second outermost circles. Applying the law of cosines and continuing as shown in solution to the second problem: [math](1 + \alpha)^2 \cos^2\dfrac{\pi}{n} - 2 (1 + \alpha) (1 + \cos\dfrac{\sigma\pi}{n}) + 2 (1 + \cos\dfrac{\sigma\pi}{n}) = 0[/math] Note that the angle associated with [math]\cos^2\dfrac{\pi}{n}[/math] has a different origin to the angle associated with [math]1 + \cos\dfrac{\sigma\pi}{n}[/math]. [math]\phi = \dfrac{\pi}{n}[/math] [math](1 + \alpha)^2 \cos^2\phi - 2 (1 + \alpha) (1 + \cos\sigma\phi) + 2 (1 + \cos\sigma\phi) = 0[/math] [math]\phi \approx 0[/math] , [math]\cos\phi \approx 1 - \dfrac{1}{2} \phi^2[/math] : [math](1 + \alpha)^2 (1 - \phi^2) - (1 + \alpha) (4 - \sigma^2 \phi^2) + (4 - \sigma^2 \phi^2) = 0[/math] Solving the quadratic equation for [math](1 + \alpha)[/math] : [math]1 + \alpha = \dfrac{(4 - \sigma^2 \phi^2) - \sqrt{(4 - \sigma^2 \phi^2)^2 - 4 (1 - \phi^2)(4 - \sigma^2 \phi^2)}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = \dfrac{(4 - \sigma^2 \phi^2) - \sqrt{(16 - 8 \sigma^2 \phi^2 + \sigma^4 \phi^4) - (16 - (16 + 4 \sigma^2) \phi^2 + 4 \sigma^2 \phi^4)}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = \dfrac{(4 - \sigma^2 \phi^2) - \sqrt{(16 - 4 \sigma^2) \phi^2 - (4 \sigma^2 - \sigma^4) \phi^4}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = 2 - \sqrt{4 - \sigma^2}\>\phi[/math] [math]\alpha = 1 - \sqrt{4 - \sigma^2}\>\dfrac{\pi}{n}[/math] [math]1 - \alpha^2 = 2 \sqrt{4 - \sigma^2}\>\dfrac{\pi}{n}[/math] [math]\displaystyle \lim_{n\to\infty} P = \dfrac{\pi}{2 \sqrt{4 - \sigma^2}}[/math] For problem #1, [math]\sigma = 0:[/math] [math]\displaystyle \lim_{n\to\infty} P = \dfrac{\pi}{4}[/math] For problem #2, [math]\sigma = 1:[/math] [math]\displaystyle \lim_{n\to\infty} P = \dfrac{\pi}{2 \sqrt{3}}[/math] -

Proportion of the area covered by circles
KJW replied to Dhamnekar Win,odd's topic in Applied Mathematics
The following is a solution to the second problem: Let [math]R[/math] be the radius of the large circle. Let [math]r[/math] be the radius of the small circles in the outermost layer. Let [math]\alpha r[/math] be the radius of the small circles in the second outermost layer. Let [math]n[/math] be the number of small circles around the circumference of the large circle. Let [math]P[/math] be the proportion of the area of the large circle covered by small circles for [math]n[/math] small circles around the circumference of the large circle. Partitioning the large circle into [math]n[/math] sectors, the area of each sector is: [math]= \dfrac{\pi R^2}{n}[/math] The total area of small circles covering a sector: [math]= \pi r^2 (1 + \alpha^2 + \alpha^4 + \alpha^6 + \cdots) = \dfrac{\pi r^2}{1 - \alpha^2}[/math] Then the proportion of the area of a sector covered by small circles: [math]P = \dfrac{n r^2}{(1 - \alpha^2) R^2}[/math] [math]\dfrac{r}{R - r} = \sin\dfrac{\pi}{n}[/math] [math]r = (R - r) \sin\dfrac{\pi}{n}[/math] [math]r (1 + \sin\dfrac{\pi}{n}) = R \sin\dfrac{\pi}{n}[/math] [math]\dfrac{r}{R} = \dfrac{\sin\dfrac{\pi}{n}}{1 + \sin\dfrac{\pi}{n}}[/math] [math]P = \dfrac{n\>\sin^2\dfrac{\pi}{n}}{(1 - \alpha^2) (1 + \sin\dfrac{\pi}{n})^2}[/math] Let [math](1 - \alpha^2) = \beta \dfrac{\pi}{n}[/math] [math]\displaystyle \lim_{n\to\infty} P = \dfrac{n\>\left( \dfrac{\pi}{n} \right)^2}{\beta\>\dfrac{\pi}{n}} = \dfrac{\pi}{\beta}[/math] Applying the law of cosines: Note that [math](1 + \alpha) r[/math] is the distance between the centres of the outermost and nearest second outermost circles, and that [math](R - r)[/math] and [math]\alpha (R - r)[/math] are the distances from the centre of the large circle to the centres of the outermost circle and second outermost circle, respectively. [math](1 + \alpha)^2 r^2 = (1 + \alpha^2) (R - r)^2 - 2 \alpha (R - r)^2 \cos\dfrac{\pi}{n}[/math] [math](1 + \alpha)^2 \dfrac{r^2}{(R - r)^2} = 1 + \alpha^2 - 2 \alpha \cos\dfrac{\pi}{n}[/math] [math]\dfrac{r}{R - r} = \sin\dfrac{\pi}{n}[/math] [math](1 + \alpha)^2 \sin^2\dfrac{\pi}{n} = 1 + \alpha^2 - 2 \alpha \cos\dfrac{\pi}{n}[/math] [math](1 + \alpha)^2 \sin^2\dfrac{\pi}{n} = (1 + \alpha)^2 - 2 \alpha (1 + \cos\dfrac{\pi}{n})[/math] [math](1 + \alpha)^2 \cos^2\dfrac{\pi}{n} - 2 \alpha (1 + \cos\dfrac{\pi}{n}) = 0[/math] [math](1 + \alpha)^2 \cos^2\dfrac{\pi}{n} - 2 (1 + \alpha) (1 + \cos\dfrac{\pi}{n}) + 2 (1 + \cos\dfrac{\pi}{n}) = 0[/math] Let [math]\phi = \dfrac{\pi}{n}[/math] [math](1 + \alpha)^2 \cos^2\phi - 2 (1 + \alpha) (1 + \cos\phi) + 2 (1 + \cos\phi) = 0[/math] Also let [math]\phi \approx 0[/math] [math]\cos\phi \approx 1 - \dfrac{1}{2} \phi^2[/math] [math](1 + \alpha)^2 (1 - \phi^2) - (1 + \alpha) (4 - \phi^2) + (4 - \phi^2) = 0[/math] This is a quadratic equation to be solved for [math](1 + \alpha)[/math]. However, only the lesser of the two solutions is of interest. [math]1 + \alpha = \dfrac{(4 - \phi^2) - \sqrt{(4 - \phi^2)^2 - 4 (1 - \phi^2)(4 - \phi^2)}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = \dfrac{(4 - \phi^2) - \sqrt{(16 - 8 \phi^2 + \phi^4) - (16 - 20 \phi^2 + 4 \phi^4)}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = \dfrac{(4 - \phi^2) - \sqrt{12 \phi^2 - 3 \phi^4}}{2 (1 - \phi^2)}[/math] [math]1 + \alpha = 2 - \sqrt{3} \phi[/math] [math]\alpha = 1 - \sqrt{3} \phi[/math] [math]\alpha^2 = 1 - 2 \sqrt{3} \phi + 3 \phi^2[/math] [math]1 - \alpha^2 = \beta \dfrac{\pi}{n} = 2 \sqrt{3} \phi = 2 \sqrt{3} \dfrac{\pi}{n}[/math] [math]\beta = 2 \sqrt{3}[/math] Therefore: [math]\displaystyle \lim_{n\to\infty} P = \dfrac{\pi}{2 \sqrt{3}}[/math] -

problem with cantor diagonal argument
KJW replied to phyti's topic in Linear Algebra and Group Theory
The flaw is his definition of a diagonal sequence/string/s. It depends on all s listed, and differs from those. He defines the initial list as consisting of horizontal s. That's why the random properties of the s and the list which is itself a random s are included. If the s are parallel there can't be any influence of one on any other. I.e. they are independent. Where did you get the idea that the sequences are random? The more correct term is "arbitrary", which is not the same as "random". In fact, Cantor attempts to construct an infinite list of all possible infinite sequences. But regardless of how that is attempted (represented by a list of arbitrary sequences), the list will always be missing the sequence constructed from the negation of the diagonal. In the case of finite sequences, which Cantor does not consider, one can form a complete list of all possible sequences. But then the diagonal argument becomes establishing that the list of sequences is longer than the sequences themselves. It seems to me that you are placing too much importance on the structure of the list of sequences while not placing enough importance on what the diagonal argument is saying. False. I use the infinite set N. False. Where do you use the infinite set [math]\mathbb {N}[/math]? In an earlier post you said: "Per the constructivist view, no one can nor have formed an infinite list. No one can even form an infinite sequence!" If that's not denying the existence of infinite lists and infinite sequences, then what is? I see nothing wrong with the notion of going from the finite to the infinite. It's a qualitative transition that does not imply a "magic [math]n[/math]". Those are the only ones we can form. No one has or can produce an infinite list. It's not an abstraction but a fantasy. No one will ever see a list for the set N. I realised after had I posted that what I said was ambiguous. And you chose the unintended interpretation. I didn't mean that it was incorrect to apply Cantor's diagonal argument to finite lists of finite sequences. I meant that your application of Cantor's diagonal argument was incorrect. Specifically, you drew the wrong conclusion from the length of the list being longer than the length of the sequences. Also, because Cantor was dealing with infinite lists of infinite sequences, your dealing with finite lists of finite sequences is largely irrelevant. I should remark that although Cantor's diagonal argument applied to infinite lists of infinite sequences only produces one missing sequence (the negation of the diagonal), in fact the cardinality of missing sequences is the same as the cardinality of all possible sequences. Any list of sequences is a proverbial drop in the ocean compared to the set of all possible sequences. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
I should point out that I am considering what time is to be distinct from what time does, although it is understandable if people conflate these two notions. -

problem with cantor diagonal argument
KJW replied to phyti's topic in Linear Algebra and Group Theory
But you have not shown that Cantor's diagonal argument is false. All you have done is denied the existence of infinite lists and infinite sequences. And you are incorrectly applying Cantor's diagonal argument to finite lists of finite sequences. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
Another important consideration is that there are three space dimensions and one time dimension. If instead there were two space dimensions and two time dimensions, as indicated by the signs of the metric coefficients, then although space and time would still be distinct, they would be indistinguishable because the sign of a metric coefficient does not itself indicate whether the component is a space or time component. This would manifest itself in the solution of the wave equation. If my quest not was meaningful - it would be metaphysics. If my quest is meaningful - it is physics. Because physics is meaningful. In that case, time is what is measured by clocks. Can any other statement of what time is be truly meaningful? -

problem with cantor diagonal argument
KJW replied to phyti's topic in Linear Algebra and Group Theory
Thank you. The flaw in your argument is that Cantor was dealing with infinite lists of infinite sequences, whereas you chose to reject the notion of infinite lists of infinite sequences and instead deal with finite lists of finite sequences, and in doing so, misapplied the diagonal argument. In fact, your argument against the diagonal argument actually proved Cantor's theorem in the case of finite lists of finite sequences. In the case of finite sequences, one can always create a finite list of all of them. Therefore, if one applies the diagonal argument to a finite list of all the possible finite sequences, then naturally the negation of any diagonal will form a sequence that is part of the list not covered by the diagonal. That the total number of sequences is greater than the length of the sequences is actually what Cantor's theorem is saying in the case of finite sequences. But when one considers infinite sequences, the total number of possible sequences cannot be listed, as proven by the diagonal argument. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
Space and time are distinct in that the metric of spacetime has oppositely signed coefficients for the space and time components. The existence of an invariant speed of light (though not necessarily light itself) is a manifestation of this distinction. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
Time and space are different components of the one notion that we call "spacetime". If two observers are in motion relative to each other, then what is purely time to one observer is a combination of space and time to the other observer. Relativity provides geometry to spacetime. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
But saying that time is what is measured by clocks is not simply saying that time is measured by clocks. It is saying that is what time is. Time is a physical quantity and so must have a physical definition if it is to be meaningful. The problem with what you are asking is whether or not the answer you seek is truly meaningful. -

Why is it so hard to explain time? (What is time?)
KJW replied to chron44's topic in Classical Physics
First I have to "declare" that my quest here in this thread is the issue of what time is. -Not how we count it or how it works. This quest is on the agenda of modern physics (as well as the issue of what space is). Therefore I'm continuing in this modern approach of physics. I don't think you grasp the significance of what swansont said. Although it may not be philosophically satisfying, the statement that time is what is measured by clocks is in fact a genuine statement of what time is. Note that one can define a clock by instructions on how to build it. What you seek is ultimately not an explanation of what time actually is. The same can be said about other physical quantities... they are what is measured by their respective measuring instruments, given that those measuring instruments are defined by the instructions to build them. Unless the explanation actually connects to the physical realm, then you don't have an actual explanation of the physical quantity. -

problem with cantor diagonal argument
KJW replied to phyti's topic in Linear Algebra and Group Theory
This asked me to sign in to Google, so please present it on the forum. -
Ok, so go on, explain those things.