tar

Thread, Well if I might...the bringing up of male and female in the context of who is liable to troll is interesting in regards to the being able to join the club or not. The value of, or faults of testosterone could be debated, but it is difficult for females to have it, understand it, and use it, as well as a male. That is it is not easy for a female to join the testosterone club, or for a male to join the estrogen one. On the playground, it was found that females tend to play cooperative games that have no winners and losers, while males tended to play games that had teams and winners and losers. Such things as T-ball makes some high degree of sense to the moms, and absolutely no sense to the dads. Imagine, playing baseball, without 9 man teams, and strikes and balls and outs, rules, consequences, winners and losers. Does not fit with the testosterone based reasons to play the game in the first place. So, in terms of trolling, it is perhaps more likely to be engaged in by a male, because a male would be more likely to enter a village, look for the king, identify the king, and challenge the king for supremacy rights, than would a female, who would more likely come into a village, identify the king and seduce him so that she could have princes and princesses as children. For someone to enter a club (talkboard) with the sole purpose of disruption, sowing discord, unseating the king, or queen, or ruling parties, and replacing them, with themselves, they are probably going to be testosterone driven, to do such a thing. Lone wolves who don't mind choosing themselves captain of a team of one. Something I am quessing I could understand as a male, that a woman would see no value in, whatsoever. In the animal kingdom (a club we are already members of) Alpha male is a role more understood and aspired to by males than females. On the school yard, men choose captains, who choose teams, who then compete for victory. Who is being more tactical, and who is being more strategic is beside the point. It depends more on whose team you are on, and whether you are captain or not, and whether you are playing baseball or T-ball to decide when a troll has entered the encampment. Regards, TAR

Acme, I know my opinion is just that, an opinion. I know Mike Smith Cosmos and I have not "made our case". But I do not think you are attempting to look at this from the "requirement" side of the coin. Logically there must be a chain of mechanisms, of actual occurences that resulted in intentional beings, because there are such things as intentional beings, and no magic and no gods. Random chance mutations of dna is only half of the equation. The other half is the environment that an organism winds up fitting. A Sulpher based life form on the bottom of the ocean and a carbon based organism in a fresh water lake have attributes that suit their particular environment. Random chance alone would say that an occasional oak leaf should have feathers and eat worms, and the same tree has a equal chance of surviving 4 miles under the ocean surface next to a volcanic vent. Since the survivability of the Oak tree is dependent on its environment, and the survivability of the sulpher based lifeform next to the vent, is likewise dependent on its environment, random chance is not the only determiner. Location and enviroment and history, are very important. It does not sometimes, accidently rain cats and dogs, instead of raindrops. Regards, TAR

Acme, Nothing at all? I don't think that makes any sense. First of all, there is a posibility that time began, along with space, at the moment of the big bang. So happening from time to time is not necessarily something that this universe does. While happening the way it has happened IS exactly what this universe has done in the last 13.8 billion years.'' Now its just a by chance thing, because one thing leads to another, and makes another possible. You can only launch the projectile out, and have it come back around the Earth and hit you in the back of the head, because you have the projective, and the motive force, and an Earth to try and throw the thing off of, unsuccessfully. And once you start talking about life, it is not random chance that paints a Robin's breast the same color as his father's. What happened before, in the case of Robins is likely to happen again, and more than likely if you are in New Jersey and not in a busy city, you are liable to see some robins on a lawn here and there this spring. Happens like clockwork. Not a random chance kind of thing. Not at all. Its happened every year since I was old enough to know what a robin was, and I am now 60. And as for our exchange, that is neither random chance, and one can point to what came before and what might come after. People have written to each other in English before, and the words we use are not by chance but rather with intentional meanings. If you would like to imagine a cosmos that spawns universes from time to time, that is your perogative. My take is that this is the first time, this particular universe has gotten to this particular point, where there is an Acme and a TAR talking about it thusly. But it is not as if no two people never had a similar exchange. Not because pure chance would dictate that random letters would form into sentences with meaning on a page, but because human beings have been expressing ideas about what they sense of the universe, to each other for scores of thousands of years. That chance happening must have been involved in our biogenesis is rather likely, but there have been two or three generations of stars that have lived prior life on Earth, that cooked up the heavy elements like carbon, upon which life is based. Once you have the deck of cards to deal from, the queen of hearts is a possibility. Before that, random chance has no way at all, to ever come up with it. You need ink and paper and technologies and processes, all put together in an intentional way, to get a deck of cards. THEN you could pull her, by blind chance. Not before. Which means you can not pull a Queen of Hearts, by pure chance. There is no way that pure chance could have made a deck of 52 cards, with a queen in it, to pull. I suppose to you, that queens of hearts are just something that happen randomly from time to time. Bolderdash, I say. Bolderdash. As a universe, anything that we are capable of, as a species, the universe is capable of, by virtue of the fact that we are here, doing the stuff that we do, in the the ways that we do it. We fit the place, a it fits us. We can be no smarter than the universe that we model. We cannot be other than the thing that we are. If the universe is not capable of intent, then I could not intentionally put these sentences together. I just did, so you have to admit the universe, at least in the case of us, is capable of intentional behavior. And being that the universe is tremendously more capable than any single human, I would have to say that blind chance has very little to do with how the universe is currently configured. If you walked in a straight line, and kept swimming and walking in that direction for many months, you would wind up coming down your street from the other direction than you started out, without ever turning around. You could not do this by accident. You can only do this, because the Earth is already a sphere. Regards, TAR

Acme, Blind chance and foreknowledge are not the only two possibilities, there is a combination of the two, that seems to be important in everything. I would like to engage a favorite saying of mine, at this point. You can roll the dice as many times as you want, and you will only get a number of dots between 2 and 12. You will never get a queen of hearts. For that, you need a deck of cards. Regards, TAR If you launched a projectile at a high enough velocity it would fall toward the Earth, keep missing it, till it completed its orbit and hit you in the back of the head. Pure chance, or foresight, or some combination of the the two?

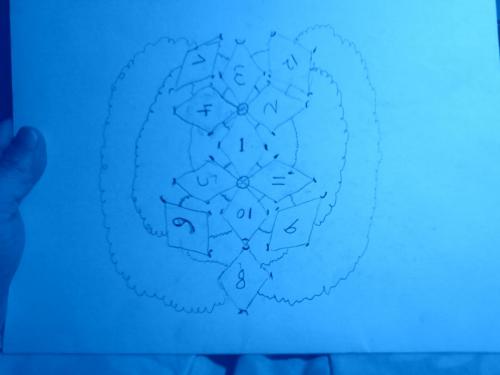
Janus, Wow, I was looking at your triangle ball with the nice complementary colors. I was noticing the eight (twelve rather) circles cutting through...and thought you had just sliced the aphere in half and then half again, and so on, from three different angles...then I saw you had taken the 12 diamonds and split them on the diagnonals. Interesting figure we are working with here. Certainly dual, if not quad or something. Interesting indeed. Regards, TAR Mike, Once they do another round of satellite photos, I will look for the great white mike smudge on Grand Canary. Regards, TAR

Vampares, Ah but the strategic pen hole is mightier than the slice of the steak knife (sword). And its the rendering program that is not worth a wit, without a wit to wield it. Regards, TAR Mike, Maybe you could use that white paint to paint some center lines on the Grand Canary mountain roads. From space (Google Earth) it doesn't look like there are any mid lines. Anyway I am considering reopening the case, so that 3meter diamond might still work. Or perhaps we can do something with those odd shapes in Janus's last rendering. In any case, I am rather sure that my original "Janus" sections of sphere are exactly 12th of a sphere, each. Having both 1/2th the surface area of a sphere, and being exactly 12th the solid angle of a sphere, each. If you divide one of these, exactly down the middle, with laser rays, eminating from the exact center of the sphere, the remaining two peices will each be exactly 1/24th the solid angle of a sphere, and , the curved surface area of each peice will be exactly 1/24th the surface area of a unit sphere. It does not mater, whether draw the lines, exactly in half this way or that, or along this diagonal or that, as long as you are making the cut with a laser from the center and keeping the laser on the same plane, as you make the cut (a straight cut), there is no choice but to split the solid angle, and the surface area in half, with the same cut. Won't result in identical peices, but the peices will each contain the same elements of solid angle, volume and surface area. I would think. How could it be otherwise? Regards, TAR Janus, Problematic, indeed. The diamonds on the other hand, staying on surface, stay diamonds when you half them from the sides and not from the point. You always have a diamond to work with exactly 1/4 the size of the parent. And the halfing lines do some nice things, as I mentioned. On the first iteration, the new lines describe six big squares and eight big triangles, exactly in the pattern of cutting the corners off a cube, like we started the whole process with. Regards, TAR

Janus, That is really interesting. Perhaps the four hexagonal planes (and the three square planes) at just those right angles to each other, conspire to equalize the vectors as Mr. Fuller noticed. Perhaps it really is as "magical" (meaning really really neat) a figure as I have sensed. Imagine what you did there. Cut twelve in 1//4s to get 24. Interesting indeed. On a parallel note, I was rethinking the cutting of the diamond into smaller diamonds. Granted it does not work so neatly if you are trying to make faces, but what if you stay on the surface? I remembered on the way home today, that when I drew my 48 and then 192 diamonds on my clay ball, I never broke the surface. The lines I was drawing where always exactly one radius away from the center of the sphere (cause they where on the surface). It is not a matter of figuring was surface area the shape is subtending, because its ON the surface and the area is exactly what the area is. In the case of the 192 diamonds, that would be 1/192nd the surface area of a sphere. Regards, TAR

MD65536 and Janus, But wait. The diamonds forever are out, but you both suggested the diagnoal route to four triangular faces, from the diamond. I did a little diamond cutting, trying to cut the diamond face flat, while staying true to the internal angles and attempting to stay normal to the center of the sphere, and like Janus says, the vertices are not on the same plane, so you wind up with a "cut" diamond, looking a little like a 4 faceted jewel. Regards, TAR I cut that one a little too deep, not knowing what to expect. It appears, to stay true to form, the exact center of the diamond should still be there as the only point on the original surface. That, and the four vertices of the original diamond shape. So the diagonal lines, leaving four triangles seems like it is the way to go to 48 divisions. But it doesn't look like the pieces with be identical. They appear to be, like Janus said, mirror images of each other. Same dimensions and angles, but one shape is lefthanded and the other right. So of the four, the catty corners are identical to each other, but the neighbors are mirror images, along the diagonals. Don't know if you can split these guys in 4, or 2 or 3. Maybe I'll wait to see if this way to 48 facets, with all vertices on the unit sphere, works.

Janus, Got it. I think my clay was too forgiving and the slivers could have fit anyway I wanted them to. Thanks for trying it out with real angles. Guess that brings the TaRadian to a screaching halt. If they don't divide into identical sections then the volume and surface areas can not be both 1/4ed with each successive division and the plan is poop. I was considering that if the solid angle at the center was quartered and the diamond shape was quartered, the interior angles would take care of themselves. Looks like I thought wrong. Anyway I really do appreciate you building out the ping pong ball arrangement, I have been trying to do that for a really long time. Still an amazing arrangement, just not as amazing as I was thinking it might be. Guess I will abandon the globe splitting quest. Without being able to reliably divide the solid angles in a manner that would keep the sections identical, there is no hope of using it as a measure of solid angle. Looks like we have the steradian for a good reason. Thanks again Janus. Was sort of exciting there for a moment. Regards, TAR

Acme, I am working on the previous suggestion to try the divisions out on the globe. It worked out really nice in preparation, my globe was about a foot in diameter so I took 6 inch peices of tape brought on down from the North Pole on the Prime Meridian, another on the date line, and another two between. (looks like a cross from the north pole. Did the same from the south. From the ends of the tapes coming from the poles made the wide angles of the four diamonds running lengthwise around the equator by simply putting the one end of the 6in. piece at the end of the tape coming from the pole, and the other end of the tape on the equator. It made the perfect angles. I aborted my effort. I have to take new pictures, minding my lighting and distance from the globe on each shot, and be a lot more careful photoshopping my sections, but here is a "rough draft" beginning of substituting globe sections for the diamonds in my diagram. Nevermind. Its incomplete and lousy to boot. I'll post something when its presentable. Could be a while.

Probably best to flip that diagram 90 degrees and put the four lengthwise diamonds along the equator, if one was to use it to divide up the globe, inorder to see the whole thing at once. Nice arrangement, as that it is not a projection, but an actual division. You could even leave the curvature and the terrain on a section, and it would still work to see the whole thing at once.

Imatfaal, I am just giggling rather hard at myself, having made 4 tetrahedron out of color clay balls and toothpicks and was in the process of trying to interlace them. I guess I am easily amused to find clay balls and toothpicks and my inability to sort out the pattern so hilarious. I took a picture, of the source of my amusement, which is definitely NOT your avatar arrangement yet. I'll post it in a bit. Just for fun. Regards, TAR ...WOW... Janus, You really have to do the cutting the diamonds in quarters thing. The new lines trace out the rhombic dodecahedron again. Or so it seems on my masacred clay ball. Regards, TAR well wait, not suprising at all. I drew the lines thru my pen holes. Duh. But I sure would like to see the Janus rendition of the resulting 48 sided figure. We could change the thread title into "dividing the sphere into 48 identical sections" Its neat. You can follow a pair of sides zigzaging all the way round the sphere and landing where you started. Then turn the big diamond 70.53 degrees (or 109.47 the other way) and follow pairs of sides around in the same zigzag. The only big diamonds you miss, doing this, are the two at the acute tips of your diamond. So you can follow pairs of sides around and see 24 little diamonds on each transit. The opposite big diamond (the opposite 4 little ones) you transit on both trips. The eight little ones off the tips of your starting diamond, you never transit following the pairs of sides around. Anyway, neat 48 sided figure, with some possibilities for further exploration. Sure would be nice to see that wireframe...(hint, hint) Here also attached it a first draft of laying the twelve sections of a sphere out where you can see the whole surface at once.

Thread, Although intent is hard to verify, it is usually communicated. I am not exactly sure how to verify this statement either, but I do believe we communicate, even on the internet, and boards such as this, on a personal level. While I am a lousy banterer, being very gulible and literal, I do, try on occasion to banter. Bantering, as in the above mentioned "posing as a creationist", is understood by the in crowd as having no malicious intent. However, if a person reading the exchange WAS a creationist, the banter would be offensive. And there is an implication, within the banter, that any creationist has to be a complete idiot, and any thought one might have along the creationist line, should be discarded to remain within the in crowd. With this general thought in mind, in regards to the definition of troll and the ethical requirement to not be a troll, I would say that a troll is anyone who seeks to attend a club meeting, without any interest in joining the club, or paying any dues. This shows disrespect for the club members, and is rude and inappropriate, and unethical in that you are speaking out of turn, without being recognized, and you don't give a hoot about the club, the members, or the rules by which they go. Regards, TAR

Imatfaal, Was wondering a few things. Looking at your profile figure, I noticed the four points at the top frontish seem to describe a square and the two of these, along with a point on the left describe a triangle. Is your figure, the same arrangement as the cubic octahedron? It looks like it is made of intersecting tetrahedra, but I would be interested to know how it is put together. And after looking through this link on the cubic octahedron http://kjmaclean.com/Geometry/Cubeoctahedron.html with the vector equilibrium idea, and the fact that it fit more snugly into a unit sphere, than the cube, I was wondering if we could come up with a regular strategy, based on this arrangement, to derive a figure that fit even more snugly into the unit sphere. If so, it might be possible, not to square the circle, but to sphere the cube, and thereby derive a function whereby the increasingly small diamond shapes of the same ratio, would have, at each iteration an exact number of which would complete the figure. If such a function could be described, when taken to the limit, to its smallest integral, approaching an infinite number of sides/diamonds, of increasingly small dimension, but the same ratio of diagonals...we would have a function that could sphere a cube. Leading me to wonder if we could find Pi in the ratio of the diagonals of the diamonds derived and depicted in my original clay figure. Regards, TAR or perhaps, not that we could, but that we should be able to find Pi in the ratio of the diagonals Janus, Can you do MD65536's divisions in quarters of the diamonds, keeping all the vertices on the surface of the unit sphere? And then divide each of the resulting diamonds in the same quarters, again keeping all the vertices on the surface of a unit sphere? We might already have the strategy. Conceptionally use the TARadian, whose solid angle is 1/12 of a sphere's, and the surface area of a Janus section which is 1/12th the surface area of a sphere, and divide the section in quarters, which will simultaneously divide both the solid angle and the surface area into quarters. Regards, TAR

imatfaal, Shaving off the vertices, in a manner normal to the center, til the new surfaces meet is the same way you get to my original figures arrangement, starting with a cube. I have not taken angle measurements on my clay bar, for obvious reasons, but noticed just before, a rather familiar looking diamond in the middle of the pentagonal arrangement on the right, above. Was wondering if anybody knows the angles of the diamonds in my original figure. The measurement of the obtuse angle, and of the acute, and what the ratio of the length of one diagonal is, to the other. Regards, TAR

Janus, But isn't the one on the right, our pentagonal arrangement, with the problems? I don't think you can use the one on the right, to seed a matrix with the "dual" characteristics of the one on the left. I know I had considered the one on the right. Probably could find a construction paper model like that (with flat faces) to prove it, if I had to, in some box or drawer. It just doesn't have the possibilities and is not quite so "loaded" with symmeties and nice finds, and "forced" qualities, as the one on the left, offers. My quess is, if pentagons were better than hexagons, we would not have honeycombs and snowflakes taking the hexagon route. All we have to back up the pentagon is starfish...well probably other things, but they do not come to mind. I was looking at some images on the web the other day, of crystals and electron microscope renderings and we have a lot of hexagonal stuff. I am trying to see what the geometric space rules are, that things tend to mind. To answer questions such as why electrons have the shell rules they have, for instance. Regards, TAR oh, and by the way, calling the figure "mine" is just a figure of speech, it is obviously "ours", and rather natural and evident and previously discovered, at that

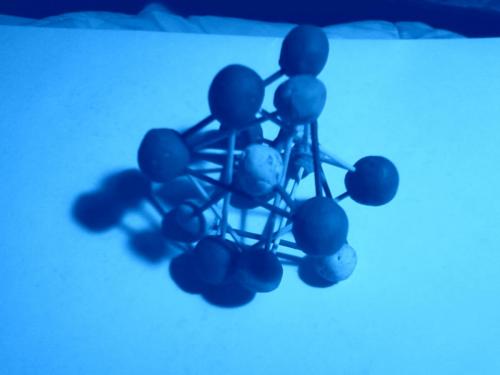
Janus, As I recall, the tetrahedra on the left do not complete the sphere. That is, if you make 12 regular ones and fit them together, they "look" like they are going to complete a "sphere", but they are a "little" off. Resulting in the reality, that if you are to join all the edges on the outside...you can't. The radii going toward the center are just a little too long...and you wind up with spaces. This would mean that twelve balls would not exactly fit around a center sphere of the same radius, in this arrangement. However, it appears that twelve balls do fit exactly around the figure on the right, and the edges shown are exactly one radii long. As are the internal edges of the six pyramids and eight tetrahedra, that point toward the center (not shown in the wire frame). This results in the fact that placing a ball at each vertices, each with a diameter equal to the length of an edge, and one in the center of the figure will result in the ping pong ball arrangement, and each ball will use exactly half its diameter to reach the outside edge of each of the twelve balls that surround it. Being that this positioning of balls is exactly the same as taking a regular cube and placing the appropriate size ball exactly at the midpoint of each of the twelve edges of the cube, and one of the same size in the exact center of the cube, the division of the cube into twelve equal solid angles, from its center point, is assured by this arrangement. As well, the division of the sphere in the center, into twelve equal solid angles is likewise insured. The diamond shapes in my original figure, are the result of forming the flat perpendiculars exactly half way between the adjacent pen holes(vertices). So in this regard, my close packing, wins, and space can be discribed, from any one point, as either 6 square areas and 8 triangular ones, in this arrangement, or the twelve identical diamond shapes that result from this arrangement. Regards, TAR

Janus, I am working with an old laptop, without its original keyboard, that my daughter used in highschool. She is now a doctoral candidate at VT. I have put so many things on it and taken them off, the registry is probably a tangled mess. Anyway, I will try an older version of the Moray. Do you have to link the POV to the Moray, or the Moray to the POV. And thanks a lot for chrushing my hopes with the pentagonal pack. Looks like MD wins the close pack challenge. Still I think the intersecting planes in mine are sweet. As I recall, I had built the pentagonal one and discarded it, for some reason. The multiple axis in the one I settled on, looked more promising. But non-the-less, it looks like the triangle wins over the diamond...when it comes to a close packed sphere. You have any problems building out the one on the left? There was some reason I didn't like it. Something didn't work out. Can't remember what it was. Regards, TAR

Thankyou Janus. That's the most beatiful thing I have ever seen. Its the figure I have been flirting with, but could never get a hold of. Thanks a million. (actually I have seen more beautiful things...but how the square planes and the hexagonal planes conspire at those angles to make each other, is really, really nice.) Symmetry to the max. Thanks a million. (by the way, my computer is way to underpowered for the POV and I couldn't get the modeling program to run, so your putting that together for me is PRICELESS.) You remain my superhero. Best Regards, TAR

Janus, Wonderful. The fliped third hexagonal plane though is more valid here, because it renders the exact arrangement of the pen holes in my clay sphere. And the pen holes are exactly in the center of each of the diamonds. It's THAT arrangement I desire to see the "next" layer of. I have been unable to achieve it in actual balls, but you can do it in virtual ones. So this is very exciting for me, as I have had it on my mind for well over a decade. The retention of the intersecting hexagonal and square planes is crucial. MD65536, "(if you cut down the middle of an edge, you get your rhombus shapes, but if you cut from the corners you can make triangular shapes)" I cut them in half the way you said, and then again. The rhombus shape is retained to the same proportions, and I think its great. I noticed that the long axis of the diamond appears to be 1/4 of a sphere circumfrence long, and I am guessing/estimating that the short axis of the diamond is 1/6 of a sphere. These are nice figures, considering the 60 degree matrices and the 90 degree matrices that this particular arrangement of pen holes around a sphere, sets up. Also gives some credence to entertaining the TARadian as a useful measure of solid angles as that the figures cut are 1 tr, 1/16 tr and 1/64 tr, respectively, and they designate 1/12, 1/48, and 1/192 of a sphere respectively. In a rather solid and sure and regular way that is easy to visualize and compute. Regards, TAR

Janus, That is fantastic. Except we need the top three flipped to get the intersecting hexagonal and square planes. That is, each ball on the first layer is in line with the center ball and a ball directly on the other side of the center ball. Where you see the two balls on the bottom three, you should see one ball on the top. In your build the top three were in the same holes as the bottom three. You have to rotate the whole third plane 60 degrees into the next set of homes. Then make the next layer. Regards, TAR You are still my hero. Can you PM me the rendering and modeling links? The priceless part I guess I will just have to borrow from you on occasion. (just for fun)

Mike, Kant, in his Critique of Pure Reason, establishes space and time as our two a priori intuitions. It is not unlikely that our models of the world, based on these two a priori intuitions have something to do with the empirical world. (if not EVERYTHING to do with it.) And we ARE postional, in relation to the empirical world, What we sense and interalize, is an anologue model of the thing, from our perspective. As we change our position, the model is continually and accurately adjusted to match what is sensed. My model of the world includes some of the roads and views in NJ and PA and NY and of less completeness those of all the states on the Eastern seaboard of the U.S. Plane trips to CA and HI as well as to the Yucatan and Japan and Germany include those positions in my model. Lived in Germany for two years and saw some of Austria, and a tiny bit of France and Switzerland as well. With Google Earth I have zoomed in on all these places and found their place in my model of the Earth. I am currenty in a basement room that few others on Earth have witnessed in its current configuration. Its not in anybody elses model, but a few repairmen and inspectors and short score of family members.. You can't see it from Google Earth. Its not in any videos on youtube. Perhaps there are some pictures, from this position in the room or that, that more people have seen, sitting on a clipdrive of the assessor, or some picture I posted on a thread. No two people hold the same model of the world, and each peice of their model is made up of views from particular positions that changed over time. And the model that each of us holds, has been built, over time. We are "positional" entities. We have built our models from Kant's two a priori intuitions. That of space, and that of time. Regards, TAR

Janus, That worked. Thanks. How much is that 3-D modeling program? How much is the Janus brain to operate it? I was wondering if you could do the ping pong ball figure. Another long term challange I have is to understand the shape you get when you build out the ping ball ball lattice, one "spherical" layer at a time. If twelve are on the first layer, how many on on the second? And the third? and so on. With this particular arrangement of "fitting" 90 and 60 degree angles, the intersecting hexagonal planes that establish square planes as well as you build out, I have a hard time visualizing what is going to happen. And have not determined what I should consider a complete "next layer". Seems its going to take a lot of ping pong balls and some correct decisions and insights...none of which I have obtained as of yet. Regards, TAR

Janus, I received a "this video is private" attempting to view the build. But you're still my hero. That is fantastic that you can do that. Here are the 4 identical sections that MD noticed.

Janus, That's great? How'd you do that? Is that actual or vitual? Regards, TAR Can we call that section a Janus if we find it does not yet have a name? Regards, TAR MD65336, Your'e right, we already have an SI unit for solid angle, that is nicely based on Pi so everything works out nicely already, nobody has the need for, or would care about a special unit. However, I still find it rather neat, and workable in visualizing how solid space fits together. It evovled, from an earlier "discovery" of mine drawing on clay spheres, and trying to work out the closest pack possible of same size balls. I know these things already have names, have been discovered and studied and measured and such, but it gives me a little sense of ownership to have found it out for myself. Here is my ping pong ball version of how space is put together. Twelve balls, fit exactly around a center ball, which results in some nice symmetries and intersection of hexagonal and square planes, when built out. Regards, TAR