Duda Jarek

"Researchers rush to test coronavirus vaccine in people without knowing how well it works in animals" https://www.statnews.com/2020/03/11/researchers-rush-to-start-moderna-coronavirus-vaccine-trial-without-usual-animal-testing/ Good video about mechanisms of COVID-19: https://www.youtube.com/watch?v=Eeh054-Hx1U

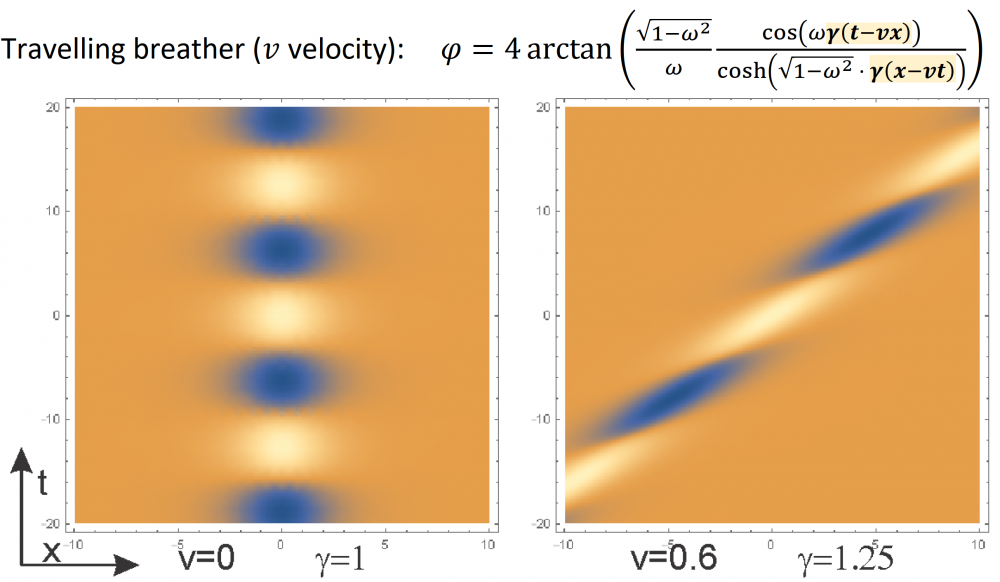
No, there is no superlaminal information exchange in EM, GR, or field theories I have considered. The goal of this thread was not solitons or virtual particles (separate topics), but to discuss a different possibility not to satisfy type of locality used in derivation of Bell inequality: by solving these models in symmetric way: through the least action principle, Feynman path/diagram ensembles. I was told by Richard Gill that it does not satisfy "no-conspiracy" hidden assumption - what agrees with https://en.wikipedia.org/wiki/Counterfactual_definiteness : " no conspiracy (called also "asymmetry of time")". While we could transform such solution to asymmetric picture: of Euler-Lagrange or Schrodinger, symmetric ways lead to solutions with different properties - due to using symmetric boundary conditions: in past and future. It is nicely seen in Ising model which is mathematically nearly the same (Feynman -> Boltzmann path ensemble), using more intuitive: spatial instead of temporal symmetry to get Born rule. For example probability distribution of value inside Ising sequence is Pr(i) = (psi_i)^2 due to symmetry: one amplitude comes from left, second from right. Sketch of derivation: Once again, by symmetry I mean time/CPT symmetry here: solving using the least action principle, path/diagram ensembles - please just think about finding probability distribution inside Ising model, e.g. in sketch of derivation above. I also don't like philosophy talk. To reduce the number of philosophical ambiguous assumptions in Bell theorem, I prefer to focus on simpler Mermin's - for 3 binary variables ABC: Pr(A=B) + Pr(A=C) + Pr(B=C) >= 1 which is nearly "tossing 3 coins, at least 2 are equal". Its derivation doesn't need any ambiguous "locality", "realism", just "there exists Pr(ABC) probability distribution" assumption: Pr(A=B) = P(000) + P(001) + P(110) + P(111) Pr(A=B) + Pr(A=C) + Pr(B=C) = 2P(000) + 2P(111) +sum_ABC P(ABC) = 2P(000) + 2P(111) + 1 >= 1 But this inequality is violated in QM formalism ( https://arxiv.org/pdf/1212.5214 ) . It has only one trivial assumption: "there exists Pr(ABC) probability distribution" leads to Bell inequalities, which are violated by physics - hence somehow this assumption is non-physical. And Ising model is a nice toymodel to understand how this assumption might not be satisfied: states are defined there with amplitudes on Omega instead of probabilities - to get probabilities we need to add over unmeasured variables, then multiply (Born rule). Exactly, all these considered field theories are Lorentz invariant, what already for sine-Gordon gives all special relativity effects, like scaling of mass/momentum, Lorentz invariance, and even time dilation for oscillating breathers ( https://en.wikipedia.org/wiki/Breather ) - their number of 'ticks' is reduced with velocity: If someone wants to understand special relativity, the best way is studying sine-Gordon.

It starts much earlier, e.g. with 1D sine-Gordon model: https://en.wikipedia.org/wiki/Sine-Gordon_equation Here is kink-antikink annihilation ( https://en.wikipedia.org/wiki/Topological_defect ) - their rest energy (mass) is released as massless excitations: Playing it backward we get pair creation - which is a continuous process: we can perform lower energy perturbation in this direction, what in perturbative QFT perspective would be virtual pair creation. ps. nice "rubber band universe model" video: www.youtube.com/watch?v=nl5Qq5kUbEE

Here is one of Faber's paper: http://downloads.hindawi.com/journals/ahep/2017/9340516.pdf Here in Fig. 4 is energy of electron-positron pair in his model for various distances - asymptotically Coulomb, deformed in tiny distances (running coupling): http://iopscience.iop.org/article/10.1088/1742-6596/361/1/012022/pdf

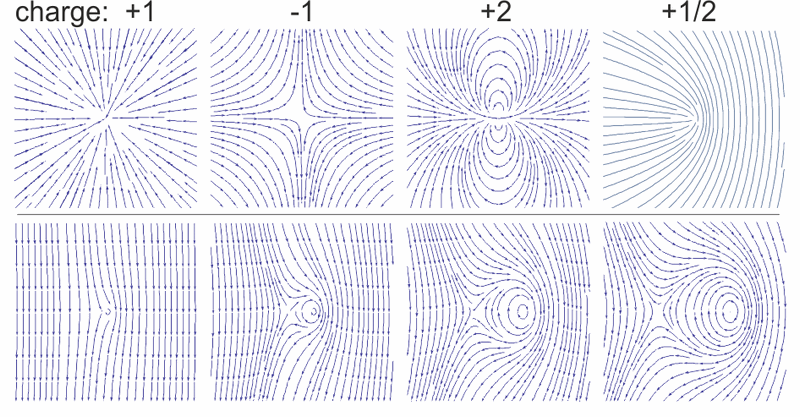
The soliton particle models I consider are based on Faber's model: repairing Maxwells' equations: to have built-in charge quantization (by using Gauss-Bonnet theorem as Gauss law - integrating field's curvature it gives topological charge inside: which has to be integer) and regularization of charge's electric field to finite energy (by using Higgs'-like potential allowing to deform electromagnetism into other interactions to prevent infinite energy in centers of particles). Anyway, this is just Lagrangian mechanics, which becomes Maxwell's equations in vacuum (far from particles) - it has all conservation laws including Noether: of energy, momentum and angular momentum. Performing second quantization we should get ~QED which might not require renormalization as electric field of charge has already finite energy here. This regularization also has running coupling - deformations of Coulomb for very close particles. Virtual particle is something different for topological solitons (like fluxons) - look at creation of minus-plus topological charge at the bottom of: Imagining it is electron-positron pair creation, the right hand side configuration uses fully formed particles: requires at least 2 x 511keV energy. But generally this pair creation process is continuous - the left hand side configuration might require much less energy. We can imagine e.g. vacuum polarization this way: brief fluctuations toward such left-most configuration ... in Feynman diagram representation it would be virtual pair creation, such brief fluctuation would not require entire 2 x 511keVs.

Mordred, once again - this was supposed to be Bell theorem thread, e.g. if it also disproves Lagrangian formalism models like EM, GR, QFT - which are realistic and local. But sure, I know especially perturbative QFT, and it is a very universal formalism - e.g. phonons are mechanical waves/distortions of atomic lattice, but QFT allows to treat them as real particles. Soliton models are in classical field theories, there is also performed second quantization for them. From other perspective, considering scattering of solitons, we need to consider various possible scenarios (e.g. kink-antikink pair creation): ensemble of Feynman diagrams from perturbative QFT. As QFT is generally built on classical field theory (e.g. EM -> QED), we should be able to understand fields corresponding to each Feynman diagram, like E ~1/r^2 around charged particles - I am only saying that we can also try to understand field for virtual particles, like in the bottom-left of soliton diagram above: just start of continuous process of pair creation.

Born-like formulas from symmetry in Ising model (Boltzmann sequence ensemble): Pr(i)=(ψ_i)^2 where one amplitude ("hidden variable") comes from left, second from right:

Mordred, I have meant time/CPT symmetry - which is at heart of fundamental models like QFT, and seems an alternative way to dissatisfy the contradicted assumptions of Bell theorem. Solving a model in symmetric way like Feynman path integrals (or the least action principle), the boundary conditions are symmetric: in both past and future, we would need two separate "hidden variables", what is a bit different than in Bell theorem. The best argument would be having Bell violation construction from solving in symmetric way - here is such construction for Wick-rotated: Boltzmann path ensembles, e.g. using Ising realization: spatial instead of temporal symmetry: Having a solution found with path ensembles, we can transform e.g. to Schrodinger, but its hidden state would be already chosen also accordingly to all future measurements - as in superdeterminism: https://en.wikipedia.org/wiki/Superdeterminism Sure, but asking about field configuration behind a Feynman diagram, e.g. with ~1/r^2 electric field around charges, what are virtual particles? We think about pair creation as 0/1 process, but e.g. topological solitons show that it can be a continuous process - e.g. from a few keVs there could start field deformation similar as in electron-positron pair creation, which quickly returns to the original state of the field. To consider e.g. scattering of topological solitons, we also need to use ensemble of scenarios - Feynman diagrams exactly as in perturbative QFT. Such fluctuation toward pair creation would correspond to virtual particle creation there, like in vacuum polarization.

While QFT is also usually solved in symmetric way (Feynman diagram ensemble: paths + more complex scenarios like decays), I didn't want to go there. The question of focus here is: what is really disproven by Bell theorem? For example classical field theories like electromagnetism or general relativity seem local realistic with field as "hidden variable" - do Bell theorem disprove them? What does it mean? My point is that maybe the misunderstanding comes from focusing on asymmetric ways like Euler-Lagrange or Schrodinger, which indeed satisfy assumptions of Bell theorem - should satisfy resultant inequalities, which are violated by physics - contradiction. Physicists believe that physics is fundamentally CPT symmetric, so maybe we should focus on symmetric ways of solving these models like through the least action principle, Feynman path/diagram ensemble - what I think don't satisfy Bell's assumptions, resolving this problem (?). Regarding virtual particles, to understand such abstract concepts I usually try to find analogues in topological solitons like sine-Gordon or 2D fluxons in superconductors. For example here are simple 2D solitons of field of unitary vectors (e.g. with (|v|^2-1)^2 Higgs potential to regularize singularities to finite energy). In the bottom half there is negative-positive topological charge pair in various distances - tension of the field increases with their distance, leading their attraction (e.g. F ~ 1/distance). What is crucial here is that such pair creation is a continuous process - we can invest much less energy than their mass to only start such process: fields deformation toward pair creation - what in Feynman diagrams language should correspond to virtual pair creation (?):

There are generally two basic ways to solve physics models: 1) Asymmetric, e.g. Euler-Lagrange equation in CM, Schrödinger equation in QM 2) Symmetric, e.g. the least action principle in CM, Feynman path integrals in QM, Feynman diagrams in QFT. Having solution found with 1) or 2), we can transform it into the second, but generally solutions originally found using 1) or 2) seem to have a bit different properties - for example regarding "hidden variables" in Bell theorem. The asymmetric ones 1) like Schrödinger equation usually satisfy assumptions used to derive Bell inequality, which is violated by physics - what is seen as contradiction of local realistic "hidden variables" models. Does it also concern the symmetric ones 2)? We successfully use classical field theories like electromagnetism or general relativity, which assume existence of objective state of their field - how does this field differ from local realistic "hidden variables"? Wanting to resolve this issue, there are e.g. trials to undermine the locality assumption by proposing faster-than-light communication, but these classical field theories don't allow for that. So I would like to ask about another way to dissatisfy Bell's locality assumption: there is general belief that physics is CPT-symmetric, so maybe it solves its equations in symmetric ways 2) like through Feynman path integrals? Good intuitions for solving in symmetric way provides Ising model, where asking about probability distribution inside such Boltzmann sequence ensemble, we mathematically get Pr(u)=(psi_u)^2, where one amplitude comes from left, second from right, such Born rule allows for Bell-violation construction. Instead of single "hidden variable", due to symmetry we have two: from both directions. From perspective of e.g. general relativity, we usually solve it through Einstein's equation, which is symmetric - spacetime is kind of "4D jello" there, satisfying this this local condition for intrinsic curvature. It seems tough (?) to solve it in asymmetric way like through Euler-Lagrange, what would require to "unroll" spacetime. Assuming physics solves its equations in symmetric way, e.g. QM with Feynman path integrals instead of Schrödinger equation, do Bell's assumptions hold - are local realistic "hidden variables" still disproven?

Sounds like you are writing about capsid (?), viral envelopes are rather not made of proteins: https://en.wikipedia.org/wiki/Viral_envelope They have some glycoproteins, which could be put on a liposome to get a fake virus ...

I am not saying about just releasing random proteins, but trying to recreate its viral envelope - doesn't it look like liposome? - part of the membrane of cell which produced it. Doesn't this viral envelope have something additional like proteins for targeting? If so, couldn't we just put these external proteins on a liposome to get a fake virus? Wanting to target enveloped virus in bloodstream, we need to target the envelope - or maybe it is just too difficult for immune system, so in practice it can only target infected cells? I thought ribosomes are mainly in nucleus and ER (...mitochondria, chloroplasts), that free ribosomes in cytosol are relatively rare in eucariote (?) and capsid's purpose is usually to get material to the nucleus(?) But still - how to get this mRNA into cells? If using other viruses, I believe it is extremely difficult/time consuming to make it work, and seems nearly impossible for mass production in millions (?) I have seen hypothesized that in November, being nearly identical suggests single point of origin - like jumping from an animal host (bat?). Fresh Science article: https://www.sciencemag.org/news/2020/01/mining-coronavirus-genomes-clues-outbreak-s-origins

Sounds interesting, but from one side delivering mRNA to cell's nucleus seems extremely difficult without viral envelope+capsid (?) Ok, they probably use envelope+capsid of other viruses, e.g. used in gene therapy (?) - but it's probably extremely difficult to take it scale required here (?) From the other side, this way immune system can only learn targeting infected cells (assuming they expose some material) - wouldn't it be better if it could directly target virus in bloodstream? Coronavirus is enveloped - where is the difficulty in just mounting its external proteins on a liposome to get a fake virus for immune system training?

Sounds interesting - some interfering RNA? The situation does not look optimistic here, exponential population growth continues ( https://gisanddata.maps.arcgis.com/apps/opsdashboard/index.html#/bda7594740fd40299423467b48e9ecf6 ) and standard vaccine development needs a year. In this moment the virus is still nearly identical: "The researchers also found that the eight complete 2019-nCoV genomes were more than 99.98 percent identical, " https://www.genomeweb.com/genetic-research/coronavirus-genome-sequencing-finds-distinct-genetic-differences-2003-sars-virus what is a big advantage for training immune response, but quickly mutations can start - especially with population growth. What other defense options are there? Wouldn't e.g. just putting its external proteins on a liposome work as provisional vaccine?

Developing a standard vaccine for coronavirus will take at least a few months - what might be too late. However, its sequence is already known, and is nearly identical - suggesting recent single point of origin for human host. So the question is if/how there could be quickly started production of some provisional vaccine - not perfect but fast to introduce? Also exploiting the fact that these viruses are now nearly identical. For example synthesizing its outside proteins and putting them on liposomes - would its introduction to blood have a chance to prepare immune system for the real virus?

Construction of Bell violation can be extended further to quantum-like computers realized as Ising model: e.g. by somehow printing on a surface conditions for solving a given problem by Ising's: Boltzmann distribution among possible sequences. Such Wick-rotated quantum gates seem a bit weaker computationally, but spatial realization allows to fix amplitudes from both directions: left and right, what seems(?) to allow to quickly solve NP-complete problems like 3-SAT (end of https://arxiv.org/pdf/1912.13300 ) :

Another paper with Bell violation using Ising model: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.123.170604

Quantum mechanics is equivalent with Feynman path ensemble, which after Wick rotation becomes Boltzmann path ensemble, which can be normalized into stochastic process as maximal entropy random walk MERW. But Boltzmann path ensemble has also spatial realization: 1D Ising model and its generalizations: Boltzmann distribution among spatial sequences of spins or some more complicated objects. For E_uv energy of interaction between u and v neighboring spins or something more general, define M_uv = exp(-beta E_uv) as transition matrix and find its dominant eigenvalue/vector: M psi = lambda psi for maximal |lambda|. Now it is easy to find (e.g. derived here) that probability distribution of one and two neighboring values inside such sequence are: Pr(u) = (psi_u)^2 Pr(u,v) = psi_u (M_uv / lambda) psi_v The former resembles QM Born rule, the latter TSVF – the two ending psi come from propagators from both infinities as M^p ~ lambda^p psi psi^T for unique dominant eigenvalue thanks to Frobenius-Perron theorem. We nicely see this Born rule coming from symmetry here: spatial in Ising, time in MERW. Having Ising-like models as spatial realization of Boltzmann path integrals getting Born rule from symmetry, maybe we could construct Bell violation example with it? Here is MERW construction (page 9 here) for violation of Mermin’s Pr(A=B) + Pr(A=C) + Pr(B=C) >= 1 inequality for 3 binary variables ABC, intuitively “tossing 3 coins, at least 2 are equal” (e.g. here is QM violation ) From Ising perspective, we need 1D lattice of 3 spins with constraints – allowing neighbors only accordingly to blue edges in above diagram, or some other e.g. just forbidding |000> and |111>. Measurement of AB spins is defect in this lattice as above – fixing only the measured values. Assuming uniform probability distribution among all possible sequences, the red boxes have correspondingly 1/10, 4/10, 4/10, 1/10 probabilities – leading to Pr(A=B) + Pr(A=C) + Pr(B=C) = 0.6 violation. Could this kind of spin lattice construction be realized? What types of constraints/interaction in spin lattices can be realized? While Ising-like models provide spatial realization of Boltzmann path integrals, is there spatial realization of Feynman path integrals?

It seems that condensed matter people usually just brute force use Monte-Carlo, but there are some subtle mathematical tools which might be worth considering, for example here is fresh paper with Mathematica implementation calculating in seconds parameters for Ising-like models with many digits of accuracy, also probability distribution of patterns or allowing to generate new uncorrelated field with single scan. 1) Hammersley-Clifford theorem ( https://en.wikipedia.org/wiki/Hammersley%E2%80%93Clifford_theorem ) saying that Gibbs fields are equivalent with Markov fields, which allow to simplify models, e.g. through local Markov condition: Pr(value in node | values in remaining nodes) = Pr(value in node | values in its neighbors) 2) Maximal entropy random walk ( https://en.wikipedia.org/wiki/Maximal_Entropy_Random_Walk ) provides probability distribution of patterns for Boltzmann ensemble of infinite sequences. Applying it to transition matrix (M_uv = exp(-beta E_uv)), while there are usually used its eigenvalues, here from its dominant eigenvector we get probability distribution of patterns e.g. in 2D Ising model: Pr(u) = (psi_u)^2 Pr(u,v) = psi_u (M_uv / lambda) psi_v Is there a literature applying any of them for Ising-like models? Beside Monte-Carlo and molecular dynamics simulations, what interesting mathematical tools are used in modern condensed matter physics? Diagram from the paper with concepts and plots of errors:

Both these topics are very speculative, - left-handed neutrino under CPT becomes left-handed antineutrino ... wouldn't it agree with hypothesis that it is a Majorana particle? I think we are very far from concluding something certain here, - also for baryogenesis (against baryon number conservation law) - can we be certain that it wasn't just Big Bounce: a fixed baryon number collapsing and expanding again? Accepting violation of baryon number conservation law e.g. in baryogenesis or Hawking radiation, means that proton decay is possible - this is a process we can now only speculate about.

Regarding neutrinos, I haven't heard of suspicion that they might violate CPT? Regarding baryogenesis as violation of baryon number conservation, it is a hypothetical process and could be avoided if assuming Big Bounce: maintaining fixed number of baryons before and after. Assuming baron number can be violated, baryons should be also possible to destroy (as in proton decay) e.g. in Big Crunch, they are also hypothesized to be destroyed in Hawking radiation: effectively transforming matter into massless radiation.

While CPT theorem suggests that all processes have time/CPT-symmetric analogues, there are popular doubts regarding some - starting with measurement: 1) Example of wavefunction collapse is atom deexcitation, releasing energy - it is reversible, but it requires providing energy e.g. in form of photon to excite back an atom. Can measurement be seen this way - that there is always some accompanying process like energy release, which would need to be also reversed? For example in Stern-Gerlach experiment: spin tilting to parallel or anti-parallel alignment to avoid precession in strong magnetic field - does it have some accompanied process like energy release e.g. as photon? Can it be observed? 2) Another somehow problematic example is stimulated emission used in laser - causing photon emission, which finally e.g. excites a target, later by light path. Does it have [urlhttps://physics.stackexchange.com/questions/308106/causality-in-cpt-symmetry-analogue-of-free-electron-laser-stimulated-absorbtion]time/CPT-symmetric analogue[/url]: some stimulated absorption - causing photon absorption, which e.g. deexcites a target, earlier by light path? 3) Quantum algorithms usually start with state preparation: all 0/1 qubits are initially fixed to let say <0|. Could there be time/CPT analogue of state preparation: fixing values but at the end (as |0>)? 4) One of cosmological examples is Big Bang: which hypothesis of the point of start of time seems in disagreement with CPT theorem - instead suggesting some symmetric twin of Big Bang before it, like in cyclic model of universe. Is hypothesis of the point of start of time in agreement with CPT theorem? Could these two possibilities be distinguished experimentally? What other processes are seen as problematic from time/CPT symmetry perspective? Which can be defended, and which essentially require some fundamental asymmetry?

I don't doubt that free-cell synthesis can get high production ... assuming you have a good source of e.g. polymerase, which are the real problem here - without mirror cells and ribosomes ... Beside industrial applications, mirror life will be also a crucial milestone in development of synthetic life - the first really different and reasonable (in contrast to e.g. additional nucleotides), and natural development will make it in reach in a few decades, e.g.: 2002 - synthetic virus: https://en.wikipedia.org/wiki/Synthetic_virology 2010 - synthetic cell: https://en.wikipedia.org/wiki/Artificial_cell#Synthetic_cells 2013 - synthetic ribosome: https://en.wikipedia.org/wiki/Synthetic_ribosome 2016 - large mirror protein (polymerase) Will we be really able to contain it then forever? - with human factors, antibiotics resistance, accidents, etc. ... it seems a matter of time when it will finally reach natural environment and start searching an ecological niche to populate, evolve, diversify ...

I don't have education in biochemistry (physics, cs, math), but it seems highly unlikely that you could produce macroscopic (e.g. grams) amounts of large molecules this way (?) Especially proteins requiring mirror ribosomes, often complex post processing, help in folding ... Cell-free synthesis might be useful for extremely rare diseases, but finding some promising drug for a common disease in this huge mirror world, there would be needed kilograms, tonnes to synthesize - what is completely unrealistic without mirror life ... which should become easier every year due to natural development of technology. Anyway, I think it is a matter of time (less than a century) when, due to ambition/money incentives/"because we can", somebody will open this Pandora box, e.g. secretly in a lab in China like for CRISPR babies ...

Hello, the Nature article mentions aptamers as direct application, which are length 30-80 oligonucleotides. Enantiomers of the small ones probably can be directly synthesized in negligible quantity. Now they have mirror polymerase allowing to speed it up, but being relatively costly to synthesize, how many copies can produce a single molecule of polymerase? For mass production there is needed mirror life. And aptamers are just the beginning - mirror life would literally double the space of possible large molecules we can mass produce. Starting actively searching this space, we can find many valuable ones. Especially enzymes - complex and effective nanomachines, optimized for very sophisticated tasks. Anyway, there are extremely strong incentives, not only financial, to go toward finally synthesizing mirror life - like CRISPR babies, there might be no way to stop it (?) What we can do is trying to prepare - understand it well, try to protect from the dangers. And there are many of them - earlier than mirror cyanobacteria dominating our ecosystem due to less prepare natural enemies, potentially killing us all in a few centuries. Bacteria has extremely fast evolution - already can consume l-sugars, and can quickly adapt to others. Mirror E. Coli might already find unusual ecological niches, disturbing ecosystem in an unpredictable way. I wouldn't be surprised if synthesizing mirror life was a factor in Fermi paradox - it is a natural possibility in development of civilization ... which might lead to its extermination.