timo

No, that's not what it means. The letter "m" means mass, not "matter". You cannot convert matter into energy in the same spirit that you cannot convert a block of iron into blue. It means that mass contributes to the total energy. I think that any reaction for which a) energy and momentum are conserved and the relationship [math]E^2 = m^2c^4 + p^2c^2[/math] holds for each in-particle and out-particle individually, b) the total number of quarks and leptons is conserved (anti-particles count negative), holds should be possible in principle within the standard framework of particle physics. In practice, the probability of a certain reaction to happen can be negligible, tough (rules of thumb: The less kinetic energy the reaction products, the less probable; the more reaction vertices needed in the smalles Feynman diagram, the less probable). The only reason E=mc² matters at all in the process is that it contributes to the contraint a). To answer your individual questions: An electron-positron pair (total lepton and quark number =0) can annihilate into 2+ photons (total number of quarks and leptons being zero, each). A single photon is not possible due to constraint a) - it might be interesting for you to figure out why. Same goes for quarks. The backwards-reactions are also possible except that here the photons must bring at least the mass of the leptons/quarks as kinetic energy to satisfy constraint a). Annihilation products do not need to be photons, in processes where the total quark or lepton number is non-zero it in fact cannot be photons only.

He he, that's what you get for posting lhc detector pictures and calling it your lab

More like 10^{-12} mm, I think. Dunno. Sounds somewhat reasonable, though. Orders of magnitude smaller than the size of a proton? I don't think that is true. About 10^{-7} would seem reasonable so maybe that just was a typo of yours. Why would a created black hole not move? It might be "effectively unable to have any influence". But to make this statement you'd need a scenario and a scale from which you can tell effectively irrelevant contributions from relevant contributions. I don't think you should throw around such strong statements without a context. As an analogy: One often hears that the gravitational force is much weaker than the electromagnetic force. In all generality that is crap, of course (think the motion of planets around the sun which is exclusively determined by gravity) but often stated in a context where it is true and the scale/scenario is obvious (e.g. chemical bonds in standard laboratory conditions). And these statements can then be backed up with rough calculations (e.g. by calculating the electromagnetic force and the gravitational force between two protons at 1 nm distance). It would probably be better to give an estimate of how big the radius of the event horizon actually is (just assume a standard black hole; the radius as a function of the mass can be found on Wikipedia, for example). Note that the gravitational attraction of a black hole does not end at the event horizon. Even assuming the BH was not moving: What if something flies in due to random thermal motion? Wouldn't that cause the BH to grow over time? Such qualitative statements have the problem that you do not know how much stuff is flying in in what time (not to mention that BHs are expected to radiate off). While it is true that the energies are negligible, the worry is that the BH starts to suck in other stuff and due to this process becomes large enough to have an impact. No, I think it misses the point. If you assume LHC forms a BH but that BHs do not evaporate (and I think the actual point is that forming and evaporation are tightly tied) then you do have a stable object that should grow over time. The only way you could argue that the growth is negligible is by actually doing calculations, i.e. integrating the attraction of the BH on other particles over the thermal averages of the particles in the surrounding and then deducting a growth rate from that. This then can be insignificant but there's no way to tell that without at least rough numbers. EDIT: Seeing ajb's post: Right, comparing to situations already known is an alternative (say "experimental") approach to the question. In fact -the problem that you should better convince yourself that the situations are comparable aside- it should probably be regarded the more reliable one. Scientific theories and calculations might just be wrong; nature is expected to always behave exactly like nature behaves .

Maybe this link works: http://mgmt.iisc.ernet.in/~piyer/Knowledge_Management/Organizational%20Learning%20Contributing%20Processes%202%201%20Organization%20Science%201991.pdf

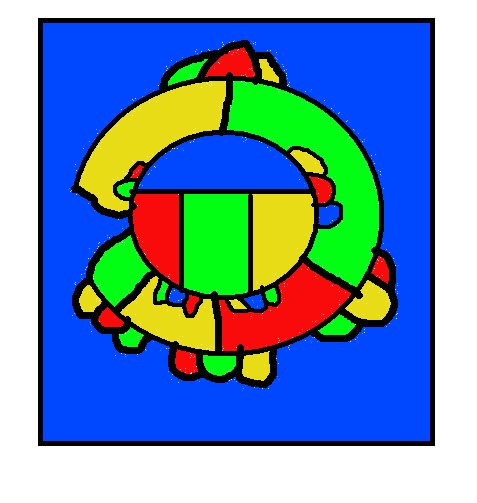
Unless I made an error, the coloring in the attachment should be a solution. Not super easy, but I got in on the 3rd attempt.

Well, to give you a start: The function is obviously differentiable at [math] x \neq 1 [/math]. It's therefore also continuous, there. So the only place where something weird could happen is at x=1. So what are the conditions that the function is continuous and differentiable at x=1? From this constraints, information on a and b should follow.

That seems to assume the people who introduced the concept were speaking English. While it may be (e.g. Newton) it may as well be wrong (da Vinci, Galileo, Gauss, ... whatdoIknowwhoelse). EDIT: Oh, and I now see that the OP seemed to also assume it.

I would assume that you simply state that (for any a) there is no x for which x>a.

Proteus starts with an equation probably involving a relativistic expression with m being the relativistic mass on the left side (the kinetic energy of a photon) and an expression with m being the rest mass on the right side (the potential energy in Newtonian gravity). At least that what it seems like to me. For me that seems like a case of not knowing what the letters stand for which would become more apparent if it was properly specified - it could well be that he wanted to couple the classical gravity field to energy rather than mass, or <something else>.

I think excluding the OP from the discussion is not really an option, big boy.

There's quite a few things that went wrong. The short version is: The equation you started off with is neither explained (what do the letters stand for?) nor justified (you seem to think in terms of Newtonian gravity when working on the prime example for gravity beyond Newtonian physics).

I am a bit worried that an ongoing high school teacher cannot solve a typical school exercise. But well, perhaps it's actually realistic to assume that few teachers (math teachers aside, of course) are capable of knowing the stuff demanded from their pupils. For the question: One statement that is helpful very often (like in this question) is that the probability of something happening plus the probability of this something not happening is 100%. This then means that the probability P that you know at least one of the units drawn is 100% minus the probability that you know none of the questions drawn: P = 100% - Q. The statement is so practical because the probability that you know none of the units drawn is rather easy to calculate. Assume the units are drawn one-by-one. The probability that you do not know the 1st one is (75-30)/75, i.e. just equal to the ratio of units you have not prepared. Since for calculating Q, you know that the 1st unit drawn must have been one you did not prepare the probability that you then also do not know the 2nd one is (74-30)/74 - the ratio of unprepared units in the remaining pool. The total probability that one event happens and then another (uncorrelated) event happens is the product of the individual probabilities for the even to happen (e.g. the probability to throw a 1 on a dice twice in a row is 1/6 (for throwing a 1 on the 1st throw) times 1/6 (probability on the 2nd throw) equals 1/36). Same goes for more than two, five in your case, events. That should be enough to calculate it. Sorry for not just giving the results or complete calculation; it really is a typical homework question. Anyways, if you just want the rough number: The probability that when having prepared 30 units you know at least one of the ones drawn is about 90%.

Speaking for me, none of Merkel's thugs visited me lately. I've just not found time to really read your 1st post and don't actually know if I really want to. One number that struck me though (you were interested in scientific opinions, right?) is the Plasma at 150 Kelvin. Is that a typo or supposed to be correct. Now, I am no expert of plasma physics but it looks strange that a substance is supposed to be in a plasma state in a temperature range where it probably doesn't even glow. On a personal note: Assuming you are serious here: Have you talked about this with your friends and family? If no, I think you should do that.

Well, a few random thoughts that come to my mind here. Note: I'll say "physics and/or math" a lot. While I do have some experiences with mathematics the stuff below primarily is from my experiences with physics. 1) If you are not amongst the top say 10% in math in your school then -provided an average school, not some elite school- you might be up to starting out as one of the worst when you start studying mathematics or physics in university. My personal experience is that most of the people I started studying physics with were among the top-5 in their school - 50% dropped during the first two years (but the old German system might have been somewhat rough compared to other countries, I think). That said (perhaps as a warning), I have a colleague who is doing a PhD over some really nasty theoretical physics who told me he wasn't really good at math is school (although I never asked him what exactly he means by that). So school grades do (of course) not say anything definite - but I think they are an indicator. 2) Talking about school grades: Unless you completely suck at physics (you don't seem to) the math grades are probably more important than the physics grades - even for aiming to study physics (for math that statement is a no-brainer, of course). 3) As a matter of fact, some natural ability to do physics or math is probably helpful for studying it - some intuition is certainly also required. But to my experience, working hard and challenging yourself has a higher weight (compared to natural skill) in university than in school. So in that respect you seem like a very suitable candidate for studying physics or math. 4) I do not advice to do physics because you find the sci-fi like topics that you hear about in the media to be cool, i.e. uncertainty, additional dimensions, wormholes, time travel, ... . If you are interested in understanding how things work and particularly if you are interested in figuring out (even when it involves at lot of hard and sometimes dull work) how things work, then that is a good motivation. If then, later, you find that the particular things you are interested in are wormholes, time travel, ... that is also fine. From my experience (as a physicist) interest in the methodology is more important than interest in a particular topic. I personally do not care too much about the actual topic but about the fun of working on it - for me almost anything is fun as soon as you dig deep enough (and still are not so far over your head that you don't make any progress). 5) Depending how serious your interest in physics is -and also depending on your parents' monetary situation- actual physics textbooks might be an alternative to finding info on websites. I am talking about into-courses to university physics and I really mean 1st semester books, not "introduction to quantum mechanics" or "introduction to string theory". Don't get too excited about that - it is just a random thought of mine. But if you are really interested in learning physics (beyond what you already do at school) then reading and (partly) understanding 1st semester books might be feasible (don't be worried if you try and don't understand anything). You are not in a hurry anyways and hopefully actually like reading such books and applying what you learned to exercises or self-imposed problems. You should probably not try this with mathematics except perhaps linear algebra. Note to other sfn-members: If you disagree on this point then please state it, I am merely proposing something that just came to my mind, here. Related to point 4: Why are you interested in physics, actually? I know it is quite a hard question to answer (in fact, I couldn't have answered it when I started studying it - I probably just wasn't) but I'd be interested to know.

I was not sure if I should comment on this or if at least I should do so in PM. But since it might also be of interest to others I'll do so in this post (despite being somewhat off-topic): 1) I do not see how that statement is different from your first one. 2) I really don't think a proportional constant depends on the units used! What you probably meant is that something like 10 m/s² can read 1000 cm/s², but that's just the same constant expressed in different units. In effect, I also doubt that there's any physics in the factor of 4 (which I expect to come from a squared R_s = 2M - see calculation below). It's just a factor that appears due to the canonical choice of setting physical constants to 1 (dimensionless). Not actually knowing the calculations I might be wrong on that, though. I was initially thinking in the lines of John in which case the question of micro states is either trivial in classical physics (mass, charge and angular momentum completely fix the micro state) or super-complicated (when you consider qft on curved spacetimes; dunno what happens there but I know that some of our aqft people are still trying to understand what temperature could mean on relatively arbitrary spacetimes so it's probably not easy). Introducing it because one introduced a temperature does sound somewhat reasonable. If (for some reason) I assume that Hawking Temperature is a temperature as in Thermodynamics (not sure to what extent that makes sense) then I could even reproduce the proportionality of S to R² with some (possibly oversimplified) easy calculation. I'll show it here, maybe someone would like to comment on it (let me emphasize here: I just pulled that out of my lowerback; it's not exactly backed up by serious literature). (1) Let's call the mass of the BH it's energy. I am not sure to what extent that makes sense - I don't even know to what energy makes sense as a global property in curved spacetime. But it's perhaps a start. (2) According to WP the Hawking Temperature is inversely proportional to the BH's mass [math]\stackrel{(1)}{\Rightarrow} T = \frac{\alpha}{E}[/math]. (3) Assume there is no pressure (whatever that would be) and that standard Thermo holds [math] \Rightarrow dE = TdS \Rightarrow dS = \frac{dE}{T} \stackrel{(2)}{=} \alpha E \, dE [/math]. Then, [math] S(E) - \underbrace{S(E=0)}_{=0} = \int_0^E \alpha E \, dE = \frac{\alpha}{2} E^2 = \tilde \alpha R^2[/math] where in the last step I used assumption (1) and the fact that the Schwarzschild radius is proportional to the BH's mass. I would not be too surprised if the mainstream derivation would be similar to my attempt - hopefully with more clarity about pressure and relation of energy to mass. Even if that was the case and those two points were clear then there's still one important caveat to this that prevents the calculation from answering the question what the entropy of a BH is: Most if not all people on sfn (including me, possibly excluding you) will not really know what the temperature of a BH is (except when tricked by seeing an allegedly familiar word and thinking they therefore understood the concept that it stands for). And of course, even then the so-defined entropy could be just a result of tossing around equations without any real meaning. EDIT: I indeed found that I could have saved some time asking Wikipedia first. Whatever, more fun trying it out myself. Merged post follows: Consecutive posts merged As said previously, that would have been my initial approach (before Andrew mentioned an access via temperature), too. The basic answer to your question is probably: No, no one on sfn can do it in a manner that you will understand. This answer is based on the following section I found on Wikipedia (and -as every WP reader- blindly believe): From that quote I guess that there is no calculation that does not involve mathematics and physics beyond a level either of us will understand. I'll gladly be proven wrong on this, though. So any stringer or other guy familiar with such a calculation is gladly invited to try

I'd consider not really working a pretty significant fault of a system.

That was the value (1/4 is also a constant, btw ). But for me the more interesting question is: What is the entropy of a black hole (or alternatively: Why would I expect it to be proportional to the volume and what is the volume)?

If you're looking to male teenagers in puberty that have to prove to everyone that their's is the biggest you should probably try some forum dedicated to an online RPG or something like that (assuming I understood the verb "to engender" correctly ). Don't you already answer your question yourself? If being true it would change the entire face of science, physics, ... then the current mainstream view is probably that it's not true, no? Probably not more than 11 of them, though. I am somewhat amazed that one can Google for Top Secret information; seems like pretty much the biggest contrast between concepts available.

As an even stronger statement: I strongly expect they'd merge into a single shell as soon as they touch. Reason: Consider the classical gravitational field and two equi-potential spheres around the centers of two circular masses. When the masses approach, the two spheres deform and at a closer distance merge into one. Since the event horizon is somwhat similar to an equi-potential area in concept and relativistic gravity is similar to classical gravity (admittedly: the similarity is not a quantitative one close to an event horizon) I'd expect the event horizons to behave similarly.

It's not absolutely needed and depending on the context either version could be preferable. But usually, the shorter an expression and the less frequent symbols appear, the better. OLD POST, REDUNDANT: Sure, I am just not sure how to explain it. Seems pretty obvious that the square root of a number squared gives the number. The square root is something like the anti-operation (the technical term is "inverse") of squaring a number. Would you agree that [math]\sqrt{x}^2 = x[/math]? In that case you can rearrange it as [math] \sqrt{m^2} = \sqrt{m\cdot m} = \sqrt m \sqrt m = \sqrt{m}^2 = m[/math]. Perhaps you should also just do some reading on what the square root is and how you rearrange expressions involving it. Or a somewhat more intuitive explanation: The square root of X tells me which value Y=sqrt(X) I'd have to square to get X. So which value do I have to square to get m-squared? m. Hence m = sqrt(m*m).

Here's a possible explanation. Feel free to skip any step if you find it unnecessary and also free to ask if one of the steps is not clear to you: [math] m \sqrt{ kT/m } = \sqrt{m^2} \sqrt{ kT/m } = \sqrt{ m^2kT/m } = \sqrt{mkT} [/math]. Edit: I was assuming that m is a mass, k the Boltzman constant and T the temperature and that hence all of these three variables are positive. You might have to be careful if some of them can be negative, e.g. m=sqrt(m^2) does not hold when m<0.

I think it is only fair to state that these "theoretical physicists" are probably about a dozen people (number guessed, I basically mean "few"). The most prominent explanation for DM is, as far as I know, still almost stable Neutralinos (or maybe other LSPs but I am not sure what other candidates there might be).

I am somewhat confused now. The factor should cancel in the nominator and the denominator if I am not completely wrong: x/y -> (x+y)/x 2x/2y -> (2x+2y)/2x = (x+y)/x In fact, 13*11 = 130+13 = 143, hence 143/88 = (13*11)/(8*11) = 13/8. Or should I better go to bed (or find a calculator)? What I said should also (in fact: especially) hold for integers, too.

I don't find this equal-spacing of "good" points surprising, at least if one assumes that the convergence is better the closer the initial ratio was. If you hit the point (x,y) then you probably come very close to the point (2x,2y) later (depending on where you start and how you increase) which has the same ratio. For the good points in between: You might hit a point (trunc(1.5x), trunc(1.5y)), 1.5 being an arbitrary number and trunc meaning some kind of rounding to whatever discretization grid you have chosen, which might be even closer to the golden ratio - note that due to the truncation it does not have the same initial ratio as (x,y). The likelihood to come close to (2 * trunc(1.5x), 2* trunc(1.5y)) later in equidistant steps should then also be there. So should the appearance of new even better in-between pairs. The explanation is a bit wave-handed, admittedly. But at least if you chose the grid for the two numbers equidistant starting from 0 (exluding (0,0), of course) that should make sense.

I can imagine this takes some changes to the system because you need to run some bot over all the accounts to adjust the rep points from time to time. You can get a similar effect with a more complicated but static calculation. Let [math]\delta_i[/math] be the number of points given on a single instance at time [math]t_i[/math]. Then, instead of calculating the total points as [math] P = 10 + \sum_i \delta_i [/math] you calculate them (at time t being "now") as [math] P(t) = 10 + \sum_i \delta_i e^{-\kappa (t-t_i)} [/math] where [math]\kappa[/math] is some decay constant ( the case of my previous proposal chosen such that [math]e^{-\kappa \, \cdot 2 \text{ weeks}}=0.9[/math]). Not sure how feasible that is but I wanted to mention it in case the approximate equivalence of these two approaches is not obvious.