Enthalpy

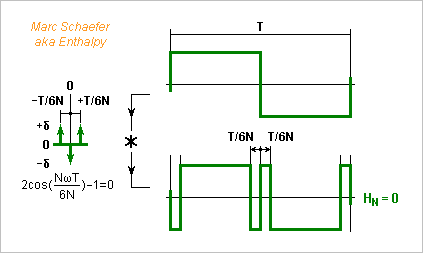
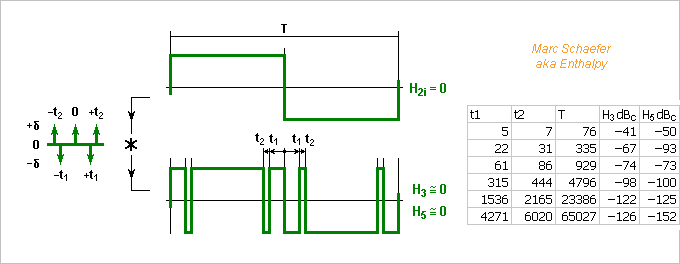
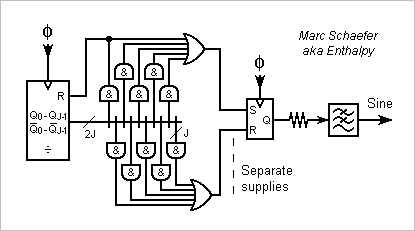
The previous method uses intermediate output voltages to suppress some harmonics. Alternately, the waveform can have more transitions between just two output voltages. This needs only one accurate output and no matched resistors, but reinforces other harmonics and has some limitations. I suppose much of that exists already. ========== To suppress one harmonic, three transitions per half-period suffice. Understanding it as a convolution with a three-Dirac signal spares some integrals and helps thinking further. Convolving multiplies the spectra. With the Diracs T/6N apart, the Nth harmonic cosine in 0.5 there, so its sum over the three positions (the Fourier integral) is zero. The sine is zero by symmetry, so is the power of the harmonic in the convolving signal, and in the output signal too. The transitions are placed accurately by a counter, for instance by 18 to suppress the 3rd harmonic, and the circuit fits nicely in a PAL for instance - with a separate CMOS flipflop for the output, fast to propagate the rising and falling edges with nealy the same delay, and having its own clean power supplies. With a simpler analog filter, it suffices already to measure the second and third harmonic distortion of an audio amplifier, detect the nonlinear antitheft magnetic filaments in goods at a shop, and so on. ========== Can we convolve several times by signals that suppress different harmonics? For the maths, we can combine the convolving signals first. Suppressing one harmonic more takes 3* as many transitions. An output signal of two values needs alternate +Diracs and -Diracs at the combined convolving signal, which happens if the suppressed harmonics' ranks differ by more than 2, annoyingly. For instance the ranks 3 and 5 need more output values hence summing resistors. Could the starting signal be unsymmetrical, like positive for T/3 and negative for 2T/3, to contain no 3rd harmonic? This helps little. One 3-Dirac convolution could then suppress the 2nd harmonic but not the 4th: if meant against rank N, it suppresses also the ranks 5*N, 7*N, 11*N, 13*N... and ranks 2 and 4 are too close for two 3-Dirac convolutions. Can 3-Dirac convolutions combine with the previous multi-level signal? Yes... Think with calm at what rank to suppress by which method so no new output level is needed. Combined solutions use to mitigate the advantages but cumulate the drawbacks. ========== Better, we can convolve the square by a signal with more alternated Diracs, putting more transitions at the output signal to cancel more harmonics. I take here the ranks 3 and 5 as an example (as the initial square already squeezes the ranks 2, 4, 6...) and extrapolate the observations to other ranks or more ranks. The seemingly harmless equation for the angular positions a1 and a2 of the -Diracs and +Diracs at the convolving signal are: 1-2cos(3a1)+2cos(3a2)=0 for H3=0 1-2cos(5a1)+2cos(5a2)=0 for H5=0 I found no analytic solution quickly. The deduced 5th grade equation with few nonzero coefficients may exceptionally accept analytic solutions, but these wouldn't help design an electronic circuit. Instead, I solved numerically a1~0.0656804005273 and a2~0.0925768876666 turns (of one fundamental period) with the joined spreadsheet. Programming, or Maple, or supposedly Mathcad, Mathematica and others would do it too. Squeeze35.zip (unzip, open with Gnumeric, Excel 97...) These parts of a period are not fractions, at least up to 66 000. Fractions can approximate them, and I picked some favourable ones from the same spreadsheet to put on the previous Png. The best denominators (clock pulses per period of output signal) against one harmonic are bad against the other, but I believe circuitry that places the transitions accurately needs one common counter, so I chose denominators decent against both harmonics. Such denominators are big: 4796 to exceed the signal purity of a Digital-to-Analog Converter. 23386 is manageable since the output signal is limited by the propagation time mismatch roughly to the audio band: clock around 23MHz for 1kHz output, and unusual but feasible 460MHz for 20kHz; the signal purity resulting from this approximation only, -122dBc before filtering, is excellent. The circuit to squeeze two more harmonics than the square signal does is simple, notably for programmable logic. The 65027 denominator needs a 16-bits counter with complementary outputs of which the And gates pick 16-bits configurations. Squeezing more harmonics, with more transitions per output period, would take more And gates. Denominators are supposedly less efficient or much bigger. Marc Schaefer, aka Enthalpy

Hello everybody! Areva is supposed to build a plant in China to reprocess spent nuclear fuel (agreement hoped in Spring 2018). I should like to remind that the radioactive 85Kr fission product, which stays in the fuel rods until they're opened, can be stored easily until it has decayed, as I described here (drawings on Dec 3, 2011) so there is no need to release it in our atmosphere. This operation is easier to design in a new plant, so it's a good opportunity for improvement. And: happy new year to everyone!

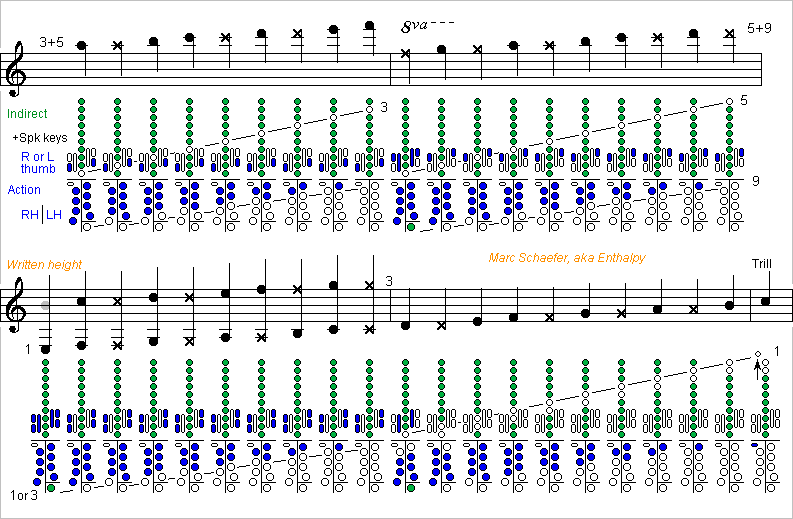
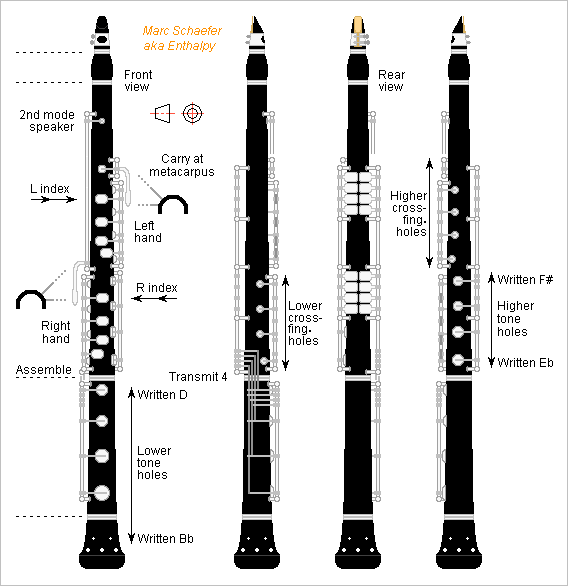
Here's a different attempt: a clarinet with automatic cross-fingerings. Other people proposed automatic cross-fingerings before. I suppose this instrument part wasn't from a flute http://memory.loc.gov/diglib/ihas/loc.music.dcmflute.1244/default.html but from such a clarinet, because (1) its 18 tone holes +end fit a clarinet (2) open holes some 9 positions apart make sensible cross-fingerings at a clarinet, not at a flute (3) the speaker key isn't desired at a flute but is at the usual position for a clarinet (4) a flute needs more flexibility to play the third octave (5) the missing length fits a barrel plus mouthpiece. This could also resemble a clarinet McIntyre system generalised to both hands, but movements resulting from two adjacent fingers suggest automatic cross-fingerings. I wish I could try the movements or have pictures from different angles. Opinions welcome! Here are already the fingerings I propose: The upper fingers close directly 8 tone holes, the thumbs on one more near the bell, all spaced by a halftone. The corresponding covers or rings act on more tone holes that I call "consequent": if the next higher hole is closed, an open finger hole permits to open two consequent holes 9 and 10 halftones higher. Mode keys at the thumbs choose which consequent hole to open, or both, or none. One open consequent hole 10 halftones higher vents the mode 5 in addition to mode 9 by the first open finger hole. This improves the reflection to play the highest register. One open consequent hole 9 halftones higher vents the mode 3 in addition to mode 5 by the finger hole. Upper second twelfth. Both closed to play the mode 3 by the finger holes. Lower second twelfth. Both open to play the mode 1 vented there. Upper first twelfth. Both closed to play the mode 1 vented at the finger holes. Lower first twelfth. At usual clarinets, the tone holes get narrow at the throat. They could be slightly wider as cross-fingering holes, and they should be to emit a clear upper first twelfth here through just two open holes. Though, narrow holes also increase the losses to match the reed and mouthpiece when the air column is short. I propose to put several smaller holes under one cover to reduce the inductance but keep the resistance of the higher holes - on other instruments too. Register holes are not displayed but necessary. The 10 halfnotes interval for mode 5+9 can probably emit a bad mode 3+5 too, so a register hole(s) shall impose the mode, just as for the lower second twelfth. More buttons at the thumbs can combine or not the action on consequent holes and on register holes. The lowest finger cover is also used alone or combined with one or both consequent tone holes. I'd put the same buttons at both thumbs, like the pinkies have at the Boehm clarinet. Some trills need extra hardware that paperwork can't determine. But one thumb tone hole more, plus its consequent hole, would solve that cleanly. Between modes 1 and 3, for Bb-C. One more hole above the consequent holes, own key. Between modes 3 and 3+5. Two more holes above the finger holes, with two keys? Between modes 3+5 and 5+9, combine the previous trill keys? These fingerings fit small clarinets better: the Eb, the Ab (or better high Bb for the repertoire). They need one joint for both hands, better including the barrel for a register key there. They limit the range to high D#, nice for a small clarinet but not for a bass. They let move 1+2+2 covers per finger, hence not too big ones please. And as small clarinets rely more on cross-fingerings, automatic keyworks with optimum venting ease them. With adapted intervals like modes 2+3 and 3+4, similar fingerings and keyworks would fit conical reed instruments: soprillo and sopranino saxophones, higher tárogatók, higher oboes, sarrusophones and rothphones, plus the ones I forget. Difficult keyworks hampered all attempts, but a sketch should come - later. Marc Schaefer, aka Enthalpy

Having read recently "refractory metals are hard and wear-resistant", I've made a table of refractory metals - because Nb, Hf and Zr are knowingly soft, and because Ta and Nb gall so brutally that wear performance is irrelevant. W and Nb may not be exactly cubic but slightly elongated. The yield strength of ever purer Re may well drop below 280MPa, so it would be soft. No relationship in this list between refractory, hard, brittle, nor the crystal form.

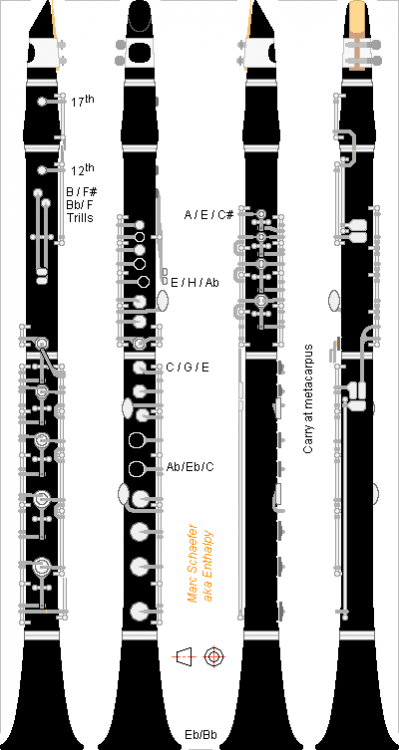
Here's a possible aspect of a clarinet with the Dec 25, 2017 fingerings. I miss the elegant and simple keyworks of the Boehm system. At least playing shall be easier. Projection follows the European method. Many background items are omitted. Right angles are only for simpler display. There are errors, certainly. Click for full size. The 17th hole is at the barrel's top as Sax and Marchi-Selmer did for the clarinet, not at the mouthpiece as Eppelsheim does for the soprillo. At the barrel, it is far too low for the top of 5*F register, but the 12th hole helps there. The Marchi system opened both keys and had a dual opening 17th hole: the reasons are expected to apply here. More register keys are possible, automatic ones too. The joints are as long as on the Boehm Bb clarinet with low Eb. The raise key, at the right thumb, is split in two but meant for simultaneous use: tolerances and assembling get easier, with only one transmission. The third button at right thumb closes the lowest cover. Some undisplayed means let hold the instrument at the metacarpus. The left hand is one tone higher than on the Bb Boehm, the right is as on the A boehm; it can be higher to shorten the upper raise key, but then the keys of right ringfinger and pinkie interact. At an Eb clarinet, some left fingers could close pairs of adjacent half-notes without covers, and the right hand use three rings. Though, I doubt a 17th key spares cross-fingerings at a small clarinet. Shallower or less narrow tone holes at the throat would ease the 5*F register without cross-fingerings, and split holes hopefully keep throat notes soft; this applies to the Bb instrument too. Lower instruments are easier, if accepting the range to altissimo C#. Marc Schaefer, aka Enthalpy

Happy new year! Bonne année! ¡Feliz año nuevo! Frohes neues Jahr! Feliz ano novo! Buon anno nuovo!

Hollow parts of thin metal let also design wider hence stiffer parts. For instance the arms that hold the biggest covers of the baritone saxophone twist easily, and some brands build two arms per cover; taller wider arms would improve and still be light. Long transmission tubes as well can be too flexible, especially on contrabasses, and wider thinner tubes would improve. The (piston or rotary) valves and slides of brass instruments may be worth a try too. Thinner metal, electrodeposited or catalytically deposited, would make the complicated shapes lighter. The parts must be ground and run in, but could still be thinner than the present brazed tubes. Can bells, other parts or complete walls be made by thin electrolytic or catalytic metal deposition? Complex accurate shapes are easy. But does some alloy (or sandwich of alloys, as already suggested here) make good instruments? Ni (and supposedly Co, Sn, Ag among others) can alloy electrodeposited Cu. Marc Schaefer, aka Enthalpy

Keyworks for woodwind (and brass winds) are made by casting (maybe forging too) of copper alloys: cups for the covers, arms... When the covers are large, notably on the saxophone, solid parts can be heavy. I propose to make them hollow and thin-walled hence light. Electrodeposition is a simple process for that, while catalytic deposition may be considered. It works easily with nickel, cobalt and their alloys, and more metals and alloys are possible, copper-nickel being known. The walls of almost arbitrary shape can be deposited on shapes of cast lead alloy that is later molten away. Other materials are used, including wax covered with graphite powder. Thickness exists down to 8µm but can exceed the mm. The process is easy enough for hobbyist to make parts for model boats, so a small music instruments company can learn it. Added layers can prevent allergies and corrosion, as is known. A first application could be the saxophone's neck octave key, which is presently slow because it's heavy, and rebounds sometimes. The musicians can replace it by themselves, especially if the pad is in place, so a company could sell the lighter replacement for instruments of varied brands. Marc Schaefer, aka Enthalpy

This is how the keyworks can raise the sound by a half-note on thumb action. Each of the eight upper fingers moves two linked covers to change the height by a full note. Additional holes make the half-notes; their covers are open at rest but often closed: when the thumb doesn't press the raise key; when the facing finger doesn't press its key; or when the next lower finger presses its key. The views use the European projection method. Rings can replace some covers, and some fingers can act at the higher tone hole position. The right thumb's button that closes the lowest cover is displayed but not its keyworks. The transition between RH and LH joints isn't accurate. All tone hole positions are open below the main transition. Zero or only one raising tone hole is open, if no cross-fingering is intended. Releasing one isolated finger for cross-fingerings would open two tone holes, or even three when using the raise key, bad - instead, additional speaker key(s) shall bring the altissimo register. Meanwhile I've read direct testimony that they do it on the Marchi clarinet and spare cross-fingerings. I've excluded some variants: The thumb must raise the sound when pressing. Release to raise would be inconvenient to play. But this demands springs acting against an other. The right thumb acts on both hands' tone holes, for easy transitions between the hands, and to leave the left thumb for the speaker keys. Opening all the raising tone holes below the transition would ease the keyworks, but the raise key's spring can't close eight covers. As described, it closes one, and the front fingers close the others - each front finger moves up to 4 covers. This system needs 1.5 tone hole per half-note. It seems to have more parts and links than a Boehm clarinet, but they are easily tuned, very uniform and mostly small and local. It saves most trill keys, the Boehm little fingers keyworks, has a single transmission between the RH and LH joints. The force needed from the front fingers must be optimized, and then this system looks very convenient to play. A clarinet drawing should come. Marc Schaefer, aka Enthalpy

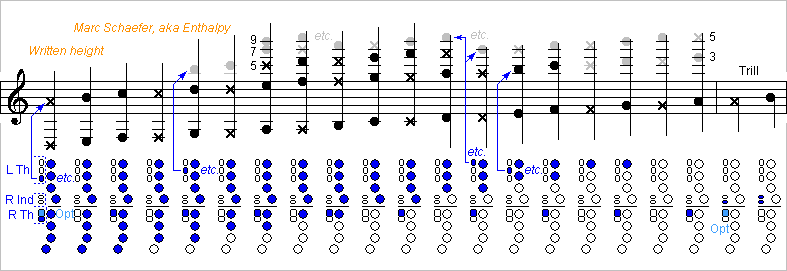
Here an attempt of fingerings for the clarinet family. The chart begins at Eb since most bass clarinets include it, and because a Bb soprano can then play scores for the A soprano. The tone holes are all open below the main closed-to-open transition. Timbre and intonation can be more even, and it solves the Boehm bass clarinet's flow noises, especially at the right middle finger's B. The speaker keys don't double as bad tone holes. Something lets hold the instrument at the right palm or at both so the thumbs are mobile. The left thumb moves no tone hole, only speaker keys, for instance three on the chart. Adolphe Sax had already put a speaker key at the barrel, outperforming the left index tone hole, and the Marchi system has it too with some refinements https://fr.wikisource.org/wiki/Page:Berlioz_-_Traité_d’instrumentation_et_d’orchestration.djvu/158 http://clariboles-et-cie.blogspot.de/2012/11/clarinette-selmer-systeme-marchi.html it shall be present here too. A single action could even open speaker holes near several pressure nodes if useful. Each of the eight upper fingers changes the height by a full note. The right thumb raises them by half a note, like the right index does for two notes on the oboe. A description of the keyworks should come. Register switches are easier than on the Boehm clarinet. Two trill keys are kept. All other trills use the normal fingerings. Only the thumbs must switch between buttons, the right one for two trills, which isn't difficult on the bassoon. Common Boehm clarinets use already the standard fingerings for the three first modes of the air column with good intonation: 1*F (fundamental), 3*F (12th) and 5*F (17th, opening the left index and right pinkie) up to the high F#. They use cross-fingerings for 7*F and 9*F, but the added speaker key on the Marchi system reportedly spares this: I'd like a fingering chart for the Marchi system, please! My fingerings are expected to ease the emission of 7*F and 9*F without cross-fingerings as they open all holes below the transition, but because they don't open isolated tone holes, they should make hypothetic cross-fingerings less efficient. If the wave reflection suffices, 5*F reaches the altissimo C#, excellent for the soprano. Marc Schaefer, aka Enthalpy

Updates yes, but not nice ones... The Navy told after two weeks that hope to find survivors had vanished, so the Government changed the operation to a search of the boat without rescue. The rescue means and teams have left, the ones for search continue, including international ones. Half a dozen potential objects were noticed, about three have been visited and were not the Ara San Juan, the others must wait for better weather. 100kg of explosives were detonated at 40m depth in the search zone to calibrate the seismic sensors that registered the previous explosion, as suggested on November 29 in the present discussion. https://www.clarin.com/sociedad/submarino-ara-san-juan-detonaron-dinamita-agua-comparar-dimension-explosion_0_HkOVtPm-f.html The newspaper tells "to compare the size of the explosion", which is already interesting to know. I suppose the experiment also let calibrate the propagation times and determine more accurately where the November 15 explosion happened. The newspaper doesn't report that, which would be a bit technical for the public.

Flexion damping by conducing heat through sheet thickness is inefficient because metal converts heat to work badly. Pure silver serving as an example: 1m*1m*1m heated by 1K stores 24kJ heat; It expands freely by 19µm or pushes 1.6MN if constrained, so it transfers 15J work or 600ppm to a matched load; Conversion from elastic energy to heat will be bad too and anyway <1; So the strain-to-strain conversion, which gives a damping with the proper phase, is tiny. Bad explanation of silver's damping on Nov 13 and 19, 2017. But the resonant frequencies stand. Since music instruments are full of excellent but unexplained features, the low-alloyed coppers and the alloy sandwiches may still be worth trying. Electrolytic deposition is an additional means to create a sandwich. Cu-Ni alloys are deposited by increasing the electrolyte's proportion of the less noble element and using enough voltage and current density Renata_Oriakova (425ko) Zr (-1.45V), Zn (-0.76V) and Cr (-0.74V) look difficult; Co (-0.28V) has nealy the same standard electrode potential as Ni (-0.25V) and should work too; Sn2+ (-0.13V) and Ag (+0.80V) lie closer to Cu (+0.34V) than Ni (-0.25V) is. I'd start from a laminated core and deposit the skins, which is decently quick for 100µm. Marc Schaefer, aka Enthalpy Thanks for your interest! Quantify how good, not really... It is a matter of individual and subjective perception, and a sound can be qualified as good for a bagpipe but not for a clarinet. Or the same saxophone sound can be considered good for classical music but bad for jazz. What's worse: we don't even know presently what physical attributes of a sound makes its quality. Helmholtz had claimed "harmonics" and everyone followed for a century and even now, but he was wrong. A few people know presently that a musical sound is, and must be, non-periodic, so its harmonics can't define it. The perception of sound quality should, to my opinion, be investigated with a high priority. It's uncomfortable because outside harmonics and frequency response of linear systems, the toolbox of physics is quite poor - but that's what is needed. Analysing harmonics and filters have brought some interesting results for violins and wind instrument, but now it seems complete, and we know that this approach is insufficient. So presently, our ears are the only judge.

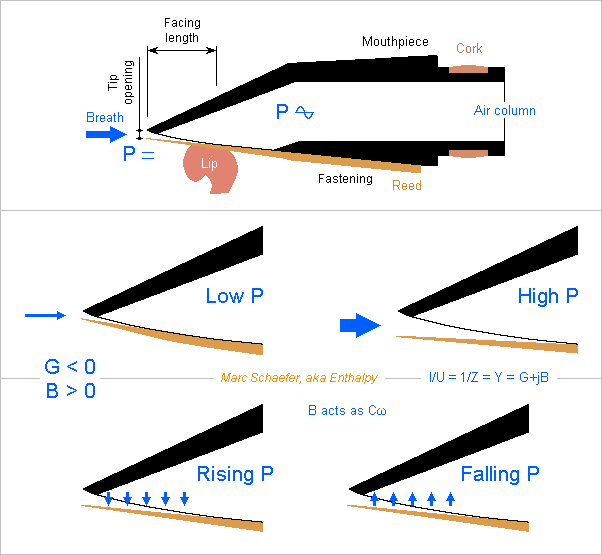
Hello musicians and everyone! About woodwind instruments, I mentioned sometimes the reed susceptance. Here are explanations. This sketches a clarinet mouthpiece and reed. A double reed works similarly https://en.wikipedia.org/wiki/Double_reed The pressure oscillations of the air column move the flexible reed. When the pressure is lower, it closes the reed. When it's higher, the more open reed lets more breath pass. More throughput when the downstream pressure is bigger is the opposite of a usual obstacle or loss: it's a negative conductance that provides power to the oscillation of the air column. That's known. The reed must be closed when the downstream pressure is low to sustain the oscillation. This needs that mainly its stiffness determines the position. Its inertia would close the reed when the downstream pressure is high, damping the oscillation. That is, the reed's resonance is higher than the instrument's notes. It can be heard as a high pitched hiss if the musician takes the mouthpiece much too far, and during articulation using an imperfect saxophone mouthpiece. Similarity of acoustics with electricity lets replace Pa by V, m3/s by A, then we use ohm and siemens: S=ohm-1. For sine U and I of given frequency, Y=G+jB where Y is called admittance, G conductance and B susceptance, while Z=R+jX where Z is called impedance, R resistance and X reactance, with j2=-1. We think of sine sounds and linear reeds to understand but none is. Horribly common. If a clarinettist blows 5L in 25s which the reed modulates by full 200µA peak while the pressure oscillates by 0.1bar peak, the reed's conductance is -20nS=-(50Mohm)-1. The air column of D=14.6mm has a wave impedance of 2.5Mohm and its losses are less than (50Mohm)-1 to oscillate with this reed, resulting from a strong resonance consistent with stiff intonation. As the pressure oscillation moves the reed, the sweeping area displaces air. Here pressure lets absorb a current (air throughput) when it rises, not when it's low or high, and lets provides a current when it falls. Like air's compressibility does, the reed adds a susceptance representable by a capacitor. I haven't seen this in books nor research papers, and at least one instrument maker ignored it. But bassoonists know it by experience while clarinettists may ignore it, not to mention organists. ========== A Bb clarinet mouthpiece can have 18mm facing length and 1.1mm tip opening, which the clarinettist reduces to a variable amount as he presses the reed with his jaw covered by the lip. Let's take 12mm width, remaining 15mm length and parabolic 0.8mm opening: the 38mm3 displaced by 0.05bar make 7.6pF, as compliant as 1.1cm3 of air, equivalent to 6.4mm length of air column; for a low note it's 1.2% of the length or 0.2 half-note. This is consistent with how much a clarinettist can pull the pitch with his embouchure. A bassoon's double reed can be 3mm open for low notes, mean 10mm wide and move over 10mm length. 0.02bar moving most of the 67mm3 make 33pF like 4.7cm3 of air. At the bassoon's narrow D/L=0.02 cone, it's as much as 0.36m from the apex or 12% of the length. Consistently, a bassoonist can pull the pitch by half a tone with his embouchure, and tunes the instrument by cutting the reed. When sounding a double reed alone, or a single reed on the naked mouthpiece, the reed's capacitance resonates with the outlet's inductance. Where the bassoon's bocal fits, L=20mm D=3mm make 3.5kH that resonate at 470Hz, not too bad estimate, and much lower than the cane's flexural resonance. At a double reed, the embouchure varies the tip opening and the mobile width, but the mobile length little. At a single reed, it varies the mobile length and tip opening but not the width. The equivalent capacitance can diminish much more with a double reed, possibly because the opening can reduce to nothing; one can pull the resonance of a naked double reed much more than of a single reed. The embouchure influences also the resonance mode of the air column - the register. By the reed's conductance or the susceptance, which are about as big? I'm not quite sure. The reed and mouthpiece must fit an instrument to sound its full range. At a bassoon, the better controlled reed lets play all the range without the lone register key, which wouldn't suffice for the range, is built little efficient and renamed "whisper key". As opposed, a clarinet would be unplayable without its speaker key. Marc Schaefer, aka Enthalpy

Kim Jong-un reappeared as the Hwasong 15 missile was launched.

The CTCBO has published a better estimate of the location of the explosion. Still nothing about a detection by their station on Tristan da Cunha, but they used the signals recorded by their seismic stations in Argentina and on the Falklands instead of the hydro-acoustic stations. In part because these stations are nearer to the source, the combed area is now much smaller. https://twitter.com/sinazerbo?lang=en https://www.clarin.com/sociedad/submarino-ara-san-juan-nuevos-datos-lugar-explosion-reducen-radio-busqueda_0_ryz0ouieG.html By the way, what I had called "hydrophones" are on the ground and they pick the ground movements created by Oceanic acoustic waves. The CTCBO distinguishes them from seismic stations, I don't understand the difference. Maybe a matter of frequency and of position nearer to the Ocean. In order to improve the accuracy of the seismic method and double-check it, it might be possible to create sound at a few locations near to the target and identify finely how it propagates to both ground stations. It doesn't need to be an explosion; anything with a broad band fits, and duration can compensate intensity. The propagation time will be known more accurately, the frequency response compared with the spectrum of the picked explosion sound would give additional information, and maybe echoes (if the received frequencies aren't too low) would tell more about the distance to the surface and the sea's bottom, the distance to the continental shelve. A helicopter-borne sonar can be there rather quickly: I don't know how flexibly they can choose an emitted signal. When used to communicate with submarines, they can emit for longer than a ping. Some active sonars are already in the search area, so if they can emit also in the frequency band picked by the seismic stations, it's the most immediate method to identify the propagation. Oil and gas exploration boats have sound sources too. Some years ago they were repetitive sudden gas expansions, that is, mini explosions. This would include the frequency band of the seismic stations. Maybe such means are available at the Falklands presently, or somewhere in the region.

Enrique Balbi, spokesperson of the Argentine Navy, excluded today that the own weapons could destroy the ARA San Juan, because the submarine didn't carry combat torpedoes: http://www.laprensa.com.ar/459901-La-Armada-dijo-que-sigue-sin-ser-detectado-el-ARA-San-Juan-y-suman-medios-a-la-busqueda.note.aspx Además, descartó que esa explosión o implosión pueda haber sido causada por armamento propio, ya que el San Juan "no tenía torpedos de combate". Single pressure-resisting volume too, if I read the drawings properly. This makes more desirable an explanation of the multiple bangs heard at Ascension. And more wanted, the records at Tristan da Cunha (if any) and at Crozet. It would help quench alternative explanation attempts, which include even an attack: Consultado sobre versiones que circulan en redes sociales, el portavoz naval subrayó que "ningún indicio" indica que "haya habido un ataque exterior al submarino ARA San Juan".

I read that there are no compartments with pressure-proof separations in the too small TR-1700 type of submarine. The source wasn't reliable and I don't find it again. At least, these drawings show no internal wall capable of resisting 70bar to make successive bangs: http://www.diariojornada.com.ar/200791/paismundo/confirman_que_el_ara_san_juan_sufrio_un_cortocircuito_antes_de_perder_contacto/ http://www.elsnorkel.com/2011/09/el-casco-del-s-42-ara-san-juan.html Only the front of the pressure hull, at the rear of the torpedo tubes, is round. Successive collapses would have been the most likely explanation for me too. Implosion versus explosion: at small distance it can be told, but at 6,000km I don't think so, because the distortions are too big. If the blue histogram is unprocessed, then the sound peaks at 10Hz with only 2.5Hz bandwidth, so it has several periods, and telling an overpressure from an underpressure is impossible. Looks like Cbcto told "explosion" and later it was deformed, including in wrong senses. The (processed) signal from Ascension shows at least three peaks at the event and none right before nor after. They are too many for one own torpedo explosion followed by one hull collapse. I don't imagine neither the hull nor the other own torpedoes resisting the explosion of one own torpedo - but I could be quite wrong since these warheads are designed for underwater effects and they would have been in air. Successive heard bangs being echoes on different water layers: 90s delay need 135km distance difference so they can't result from reflections at 3km seabed depth. Propagation through different layers? This would need several times 2% celerity difference. That's about the difference between -800m (1479m/s minimum) and -3000m (1506m/s in mean Ocean water). How would the sound stay in a faster medium? I don't know Ocean acoustics well enough. Multiple paths through different longitudes are hard to imagine. 135km more than 6000km need the sound to go 627km to the East or West on the first 3000km, then turn somehow to reach the listening station with almost the same strength. On the Cbcto's sensitivity map, shadows by islands are clearer than that, over long distances. Did you see the signal received at Crozet? Have a link maybe? Did you read if Tristan da Cunha received a signal? They should have, but I've read only about Ascension and Crozet.

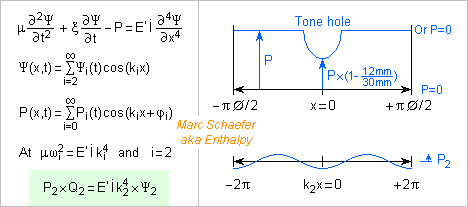
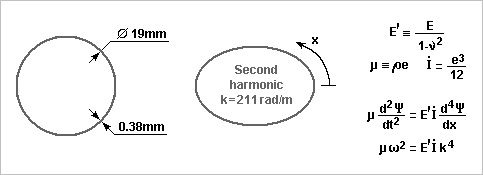
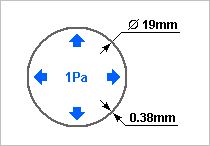
Here's a more formal model of the wall's elliptic deformation at the tone holes proposed on Nov 13, 2017. I keep neglecting the stiffening by the hole's chimney in metal bodies. If it stiffened perfectly 1/5 of the circumference on the whole body length, it would raise the resonant frequency by less than (5/4)2, nearer to 5/4. I keep the absence of pressure on the tube where the hole is, and because simplicity needs it, that the elliptic deformation is identical at the holes and between them. The deformation equation sums the forces on an element dx of the periphery for a unit length of tube. Zeta stands for the losses; heat conduction would include some complicated function of d2Psi/dt2 too, but later I represent anyway the losses by Q, the mechanical amplification factor at the considered resonance. The pressure felt by the walls, and the wall movement Psi, are defined over one circumference, so a Fourier series can represent them. Less usual than from time to frequency, this Fourier goes from the circumference position to the wave vector in rad/m. As the deformation equation is linear, the Fourier components of the movement and pressure distributions correspond, especially the second harmonic that makes the lowest resonance with an elliptic deformation. At a resonance, the µ*d2Psi/dt2 and E'I*d4Psi/dx4 compensate. If the mechanical amplification factor Q is not very small, the movement is Q times bigger than at low frequency where d4Psi/dx4 determines it. k4 comes from the differentiation of cos(k2x). A spreadsheet computes the second harmonic of the pressure distribution along the circumference for a 12mm hole in a D=19mm L=30mm tube section: WallsFourier.zip The sine peak value is P2=-0.098 times the air overpressure. Using: k2*pi*D = 4pi for the elliptic deformation, or k2=210rad/m; |P2|=-0.098 for 1Pa in the tube; E'=98GPa now for sterling silver and I=4.6*10-12m3 for 0.38mm walls; the wall moves by peak 0.11nm at low frequency and Q times more at a resonance. This is 1/4 the value estimated previously with a square tube model, and is possibly more accurate. ========== Mechanical Q=120 would now drop the sound's harmonics by 10% instead of Q=30. This isn't much for a metal: for instance a vibraphone bar resonates for seconds at hundreds of Hz, telling Q>1000 despite the radiation. Since we hear a tapped flute head joint of German silver resonate, a microphone and oscilloscope would tell figures. Marc Schaefer, aka Enthalpy

Hello everyone! The Argentine submarine ARA San Juan last reported on November 15. On November 23, the Navy published that two hydrophone stations operated by the CTCBO, meant to detect nuclear explosions, heard a non-nuclear explosion near the possible position of the submarine, suggesting a total loss of the ship. My information isn't at the source, alas - data from Twitter, and so on. Signals, positions of the hydrophones, timescales https://twitter.com/SinaZerbo/status/933745155399708674/photo/1 do you understand too that the three blue-to-red histograms are strongly amplified, hence the scale does not apply? I suppose the blue histogram maybe fits the scale in dB ref µPa2/Hz, the others not. How do you understand the several replicas after 90s? I can only image an artefact from signal processing. An echo would need a reflector >70km away, and then the echo couldn't have an amplitude similar to the first sound. Do you know if the hydrophones on Tristan da Cunha were active? And if yes, why didn't they pick the noise? They were nearer than the ones at Ascension and Crozet that picked it https://www.ctbto.org/verification-regime/featured-stations/types/hydroacoustic/ha09-tristan-da-cunhaunited-kingdom/ https://www.ctbto.org/verification-regime/featured-stations/types/hydroacoustic/ha09-tristan-da-cunhaunited-kingdom/page-1-ha09/ https://pbs.twimg.com/media/DPVIXa_W4AEyAcF.jpg and from the sensitivity map (look at the shadows by the islands), those on Tristan da Cunha seem as efficient as the others, to the West too https://twitter.com/ferencdv/status/933737271748050944 they were put into service before 2010. And if the hydrophones' sensitivity at the inferred loss location wasn't worse than 1t TNT or 4.2GJ (see map), it corresponds to 7MPa (700m depth to crush the hull) times 600m3, or 1/6th the vessel's volume (D=8.2m L=65m), so at least this would be consistent. It's consistent with other potential sources too. Thank you!

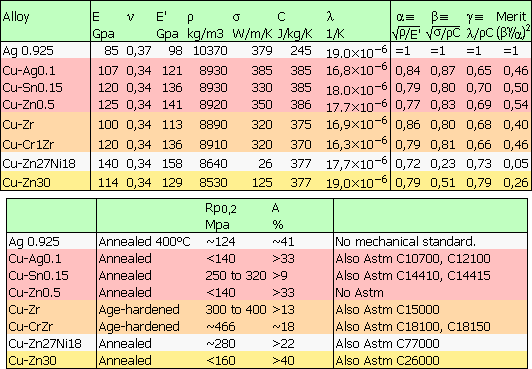
In the last message, I estimated a heat diffusion time through the whole wall thickness. But after diffusing through 1/3rd of it, heat reaches already a zone where flexion compresses and heats far less the metal, so this suffices for damping. Then, the diffusion time is only 1/9th or 0.1ms, which equals a quarter period for maximum damping at 2500Hz, in the resonance range of the 0.38mm silver walls. Strong coincidence again. ========== I've computed some figures of merit to compare alloys for damping resulting from heat diffusion. All refer to sterling (92,5%) silver, whose data comes from Doduco and Substech since mechanical engineering forgot to standardize it. Alpha tells how thinner walls can be if a stiffer or lighter material keeps the resonance frequency. Beta represents the heat diffusion distance at a given frequency. Gamma shall represent the heat-to-elongation or elongation-to-heat couplings. Possibly incomplete. The global figure of merit squares gamma since damping results from elongation-to-elongation, and also beta/alpha like a heat sine diffuses. From the table, sterling silver has the best combination to dampen vibrations by heat diffusion. Brass is bad and German silver much worse. Elemental silver's main advantage is the low heat capacity per volume unit, equivalent to a big molar volume for a metal: https://www.webelements.com/periodicity/molar_volume/ https://www.webelements.com/periodicity/youngs_modulus/ https://www.webelements.com/periodicity/coeff_thermal_expansion/ Strong thermal expansion goes rather against stiffness for pure elements, but atypical alloys exist like Invar, so it would be worth checking. Gold, platinum, rhodium are sometimes used, but their figure of merit is worse than silver, based on incomplete data. I've added high-copper alloys uncommon in instrument making. The last two need age hardening to conduct heat well; is it compatible with fabrication and maintenance methods? The figures of merit aren't as good as silver but far better than German silver and the alloys are cheap. Plated against corrosion, would they make better student's flutes? The company Gévelot supplied electric igniters whose wires could be bent sharply tens of times without hardening, while electric copper would break. I ignore the alloy, but instrument makers may like it or an adaptation. Some alloys in the table are too hard, so they could be less alloyed to improve the heat conductivity. Rolling a sheet 60mm wide uses affordable equipment, and a quick test would be to solder a tube and tap it to compare the damping with silver. ========== A sandwich can combine a stiff alloy as the skins, ideally with a big thermal expansion, and a conductive alloy as the core. In the above table, brass can cover little alloyed copper, with thicknesses like 15%-70%-15%. Deep-rolling hot sheets can join them besides explosion welding. The sandwich dampens hopefully more than brass and is stable enough for a saxophone. Ceramics are stiffer than metals and polymers expand more, but having both isn't obvious, and craftsmen prefer metals. A lacquer maybe, if easily removed and reapplied, and if some filler makes it stiff? ========== Other damping processes exist in alloys. For instance a Cu-Mn is known as a damper: try it a music instruments? Marc Schaefer, aka Enthalpy

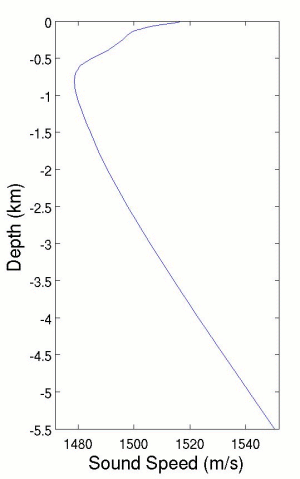
Can a physical model justify the wall material's debated influence? I take a flute as an example because I know dimensions; a piccolo or a saxophone would be interesting too. Most people consider a cylindrical closed tube: A usual argument is that 1Pa air overpressure in D=19mm and for instance L=30mm (a distance between tone holes) squeeze adiabatically 6*10-11m3 more air in the column, while the resulting 25Pa stress in the 0.38mm E=83GPa silver walls strain them by 0.3ppb and increase the contained volume by 5*10-15m3, or /104, so it's negligible. Here I suggest (I'm probably not the fist) a more significant process through resonance and oval deformation. I apply known models for flexural waves in sheet (E'=96GPa for pure silver, rho=10490kg/m3) to the cylindric wall. Its deformation is represented by a Fourier series where the fundamental is meaningless and the upper harmonics uninteresting, leaving the second, where kx covers 4pi in one geometric turn. With 0.38mm thickness (0.45mm is common too), the oval deformation resonates at F=2342Hz, just one note above the official range of the flute. So the fundamental doesn't excite this resonance (it can't be chance) but the harmonics may. How can such an oval mode couple with the air pressure? I exclude offhand the circle imperfection of the body, because tone holes offer a stronger coupling. Where the instrument has a hole, the wall doesn't receive a balanced force. It could be an open hole, if the air keeps a significant pressure at that location, or beneath a cover or a finger, which get a part of the force. As the pad or finger are much softer than the wall, they vibrate separately without transmitting the missing force to the wall. From 1Pa overpressure, 112µN push a D=12mm hole cover instead of balancing the forces on the wall. As balanced forces have no consequence, the effect is the same as 112µN alone pushing down on the wall's top, and since the whole body can accelerate freely, the deformation is similar to 56µN pushing towards the centre at the wall's top and bottom. The hole's chimney stiffens the body on 1/5 of the circumference and on 12mm over 30mm body length. This raises the resonances a bit, offering many proper frequencies to the sound. I neglect this stiffening for simplicity. Taking a Fourier transform of the force distribution and solving on the cylinder for the second harmonic would have been more elegant. Instead, I model the closed cylinder as a 15mm*15mm square of same circumference, slit where the forces apply. On 30mm length, 28+28µN and 7.5mm arm length bend E'I=0.013N*m2 by 0.12µrad over 7.5mm height, so the centre moves by 0.9nm - but half without the slit, or 0.45nm. The oval deformation changes the volume little at the closed section, but at the decoupled cover it makes 5*10-14m3. That's 1200 times less than the air compressibility, without resonance. Now, metal parts resonate, often with a big Q-factor. Take only Q=40: the volume due to the wall vibration is only 30 times less than the air compressibility. And at the resonance, the volume increases when the pressure peaks: it acts as a loss, not like extra room. This effect may be felt. With varied hole spacing, the flute offers many different wall resonances. The computed 2342Hz is for instance the 3rd harmonic of the medium G, so it has 12 quarterwaves in the air column. For this frequency and column length, the radiation losses alone give Q=60 and the viscous and thermal losses alone Q=138, summing for Q=42 without any other loss at the pads, the angles etc. If the wall resonance adds its Q=30 at one 30mm section from 440mm air column, the combination drops from Q=42 to 38, or 10%. Harmonics and partials change the timbre and the ease of emission. We're speaking about small effects anyway, so this increased damping of the harmonics may explain the heard and felt difference. ========== If you tap a flute's body with a plastic rod, German silver makes "ding" while silver makes "toc", a strongly damped sound. Silver's smaller mechanical resonance would attenuate the harmonics less, providing the reported easy emission and brilliant sound (...that I didn't notice at the Miyazawa headjoint test). This isn't necessarily a bulk property of silver. Thin sheets dampen bending vibration also by thermal conductivity: the compressed face gets warmer, and if some heat flows to the opposite side, less force is released when the compressed face expands. For 0.38mm thickness, a typical heat diffusion time is 0.8ms, just a bit long for the body's oval resonances, so thinner silver would attenuate more the mechanical vibrations hence less the sound's harmonics, but its resonances would fall too low. 0.45mm raise the resonance frequencies but strengthen the mechanical resonances. Again, this is consistent with the choice of silver for heat conductivity, and with the wall thickness. It's also consistent with the tried red brass for saxophones. But as PDM conducts probably less than sterling silver, it must bring other advantages. An alloy with a big thermal expansion (but good heat conductivity) should make a damping sandwich around a conducting silver core. I didn't find practical elements (indium 32ppm/K, zinc 30ppm/K) but alloys may exist. Laminate together with silver, or weld by explosion, as usual. Total 0.4mm stay good, most being silver. ========== Grenadilla (Dalbergia melanoxylon) is less stiff: 20GPa lengthwise hence maybe 2GPa transverse. But it's lighter hence thicker, like 1310kg/m3 and 3mm. The same resonance jumps to 14kHz. To my eyes, a good reason that high notes around 2kHz are easier. I've no data about damping at interesting frequency for transverse bending. Grenadilla gets scarce, and different wood is less stiff. Plain polymers offer isotropic 2 or 3GPa and the same density and thickness. Damping and lengthwise stiffness may distinguish them from wood. Polyketones are known dampers, worth a try? Polymers loaded with short graphite fibres are available industrially, notably POM and ABS. They offer 1470kg/m3 and isotropic 10GPa, very seducing. Graphite isn't very abrasive to cutting tools. Give them a try, including at bassoons and oboes? Long graphite fibres in epoxy matrix exist for flutes (Matit). If filament winding isn't already used, it's easily tried, since small companies make tubes on request. Marc Schaefer, aka Enthalpy

Here are some observations I made about wall materials for woodwinds. ========== The most reliable experiment was with flute headjoints on a concert instrument by Miyazawa, who sent the flute to a distributor in my city for the trial. Two professional flautists were invited together with me. They abandoned the trial and preferred a smalltalk after half an hour, so I could try the hardware alone for the afternoon. The room was mid-small, with carpet and some furnitures, at comfortable temperature and usual humidity. I was in an investigative mood, I believe without prejudice. Myazawa put at disposal a flute body with perfectly adjusted keyworks, whose intonation and emission beat the new Cooper scale, and three headjoints of shape as identical as possible, of - silver-plated German silver - plain 92.5% silver - their PCM alloy. All differences are small. The temperature of the headjoint is much more important than the material. Playing music wouldn't tell the differences within the test time: I provoked the known weaknesses of the Boehm flute. After 20 minutes, I could detect differences and reproduce them with confidence. Plain silver is identical to silver-plated German silver, or at least the differences are uncertain. Silver might make more brilliant medium notes. PCM improves over silver. The highest notes of the 3rd octave (and the traditionally unused 4th) are easier to emit pianissimo, and they sound less hard consequently. The instrument's lowest notes can be louder and their articulation is easier. I can't be positive that the medium notes are more brilliant. We avoided comments during the trial. One other flautist coincided exactly with me, the other had no opinion. So while materials do make a subtle difference, switching from German silver to silver headjoints as a flautist progresses is just superstition and marketing. Manufacturers may use silver for their better handcrafted products. I ignore if silver is easier to work and enables different shapes, but its acoustic qualities are identical to German silver, a cheap alloy of copper, nickel, zinc. The better PCM is darker than plain silver, rumoured to contain less silver and be cheaper. I believe up to now that the wall material matters most at the tone holes, hence at the body more than at the head joint. Testing that would be uneasy, since identical shapes are more difficult at the body, and the cover pads matter more than the walls. ========== I tried once a flute of gold, pure or little alloyed according to its colour. It was only a typical new Cooper scale, with very low 3rd G# and imperfectly stable 3rd F# - poorly made in France with very bad short C#. It didn't even have the split E mechanism, so the 3rd E was badly unstable. The lowest notes were weak, the highest hard and not quite easy. With such a thing, I couldn't concentrate on the claimed acoustic qualities of the metal and stopped the trial very quickly. At least, they didn't squander scarce wood for that. ========== Some piccolo flutes have grenadilla or silver headjoints, at Yamaha and elsewhere, on a grenadilla body. Wood is so much better that telling needs no frequent switches in a long experiment. The highest notes are easier to emit piano hence sound less hard. The lowest notes stay bad as on a piccolo. Wood (and plastic) offers other manufacturing possibilities than metal sheet. Especially, undercutting the blow and tone holes is easier. This may explain a good part of the improvement. The temperature profiles of the air column can't match between a wooden and a metal head, so "identical shapes" would be meaningless anyway, as harmonics aligned for one material would be misaligned with the other. Was the design optimized for wood and kept for metal? At least, the comparison stands for other manufacturers. ========== I tried a modern grenadilla flute from Yamaha around 2004. I found it fabulous. While metal concert flutes don't differ so much, this instrument has by far the strongest low notes of all the flutes I've tried - a very much desired improvement - and the easiest pianissimo on the highest notes. Its sound is very mellow, what soloist seeking a "good projection" hate but saxophonists switching the instruments like. Did the material alone make the difference? I don't think so. At Mönnig the wooden and metal flutes played about identically. This flute had also a new scale (holes' position and diameter) since its 3rd F# was more stable and its 3rd G# intonated almost perfectly. The mellow sound may result from the scale, as for a flute I tried in a Parisian workshop, and the stronger low notes from wood's workability like undercutting, or from a wider bore locally. ========== Despite playing the saxophone, I was once called to try a clarinet of thin injected thermoplastic. Its covers were of injected thermoplastic too, with modified movements, and I don't remember the more important pad material. The effect is huge, and people who claim "the material has no influence" should try that. The cheap and easy instrument offered as little blowing resistance as a soprano sax, consistently with huge losses, and couldn't play loud. The timbre suggested a clarinet, but, err. ========== Comparative trials abound on the Internet but many ones about flutes are obviously fiddled so the hearer notices a difference. Remember on the Miyazawa, it took long to notice any difference; much was about the ease of playing, and the subtle sound differences wouldn't survive computer loudspeakers. These shall be oboes of grenadilla versus cocobolo, both from Howarth https://www.youtube.com/watch?v=yVouYVlDZDY and if the construction is identical, then the material's influence is (not unexpectedly) huge on an oboe. In short, cocobolo makes bad oboes of clear and weak sound. Grenadilla (Dalbergia melanoxylon) gets ever scarcer while cocobolo (Dalbergia retusa) is abundent, but cocobolo slashes the density by 1.4, the longitudinal Young's modulus (I'd prefer the transverse) by full 2.0 but increases damping by 1.5 https://hal.archives-ouvertes.fr/tel-00548934/document (9MB, in French, p. 117)

Here's a possible aspect of the piccolo reed instrument. I've drawn a single reed instrument because the rare Ab clarinet achieves the target range and its reeds exist in catalogues, while I doubt oboists' lips survive one octave more. The apex of the conical bore must be truncated very little. The miniscule reed imposes some small susceptance, but the mouthpiece's volume must be reduced. A double reed would help here. Like a clarinet but unlike a saxophone, the mouthpiece fits in the body with no additional volume. The reed seat of an Ab clarinet mouthpiece may fit the task; the longer instrument permits more tip opening with softer reeds. To ease the piccolo flute's high notes, thick grenadilla walls beat metal, hence grenadilla here - or polymers, I still have no opinion. The section from the mouthpiece to the thumb keys is more easily bent, for instance to make a sopranino of decent size. Here a piccolo is as long as an oboe. The result resembles a narrow, higher pitched tárogató. Call it a gatito? I omitted on the sketch many items where partly hidden. Other are over-simplified, for instance four movements need four shafts or two pairs. And, well, there are probably some mistakes. The keys that make the cross-fingering holes closed at rest to ease the fingerings put the holes behind the hands on my sketch. Improvement would be welcome. Here 4 tone holes on the upper joint are closed at rest and 4 on the lower are open at rest. This lets transmit 4 movements. 5+3 holes are possible with a slight fingering change and a longer wooden main joint. The holes brought by Stowasser are closer to the bell's rim on this higher instrument. If the cross-fingering holes double-serve as tone holes, they can reach without gap the first mode pedal tones, but the instrument must favour the highest modes through the bore width, reed and mouthpiece size, position and size of the holes. Marc Schaefer, aka enthalpy

Hello everybody! The material used for the walls of woodwind instruments, and its real, perceived, imagined or absent influence on the sound and ease of playing, has been and is the controversial matter of recurrent discussions that I gladly reopen here. The air column is the essential vibrating element of a wind instrument, the walls are not, but this is only a first analysis. The walls are commonly made of wood (sometimes cane, bamboo etc.), metal, or polymer aka plastic, which manufacturers call "resin" to look less cheap. Mixes exist too, with short reinforcement fibres or wood dust filling a thermoplastic or thermosetting resin ("Resotone" for instance). I'm confident that long graphite fibres were tried too, as fabric, mat or in filament winding. The choice results from marketing, tradition, weight and manufacturing possibilities (a tenor saxophone is too big for grenadilla parts), cost - and perhaps even acoustic qualities. ========== Plastic is a direct competitor for wood, as the possible wall thickness, manufacturing process, density, stiffness, shape possibilities, are similar. As opposed, the density of metal restricts it to thin walls made by sheet forming an assembling, but permits big parts. Manufacturers typically use plastic for cheaper instruments and grenadilla for high-end ones - some propose cheaper wood in between, possibly with an inner lining of polymer. Musicians who own a grenadilla instrument disconsider the plastic ones; I never had the opportunity to compare wood and plastic instruments otherwise identical, so I can't tell if the materials make a difference, or if grenadilla instruments are more carefully manufactured and hand-tuned, or if it's all marketing. Two polymers are commonly used: polypropylene for bassoons, and ABS for all others, including piccolos, flutes, clarinets, oboes. These are among the cheapest polymers, but 10€/kg more would make no difference. They absorb very little humidity, but some others too. More surprising, they are uncomfortable to machine: POM for instance would save much machining cost and (my gut feeling) easily pay for the more expensive material. But ABS and also PP absorb vibrations while others don't, which I believe is the basic reason for this choice. They limit the unwanted vibrations of the walls. As a polymer that dampens wall vibrations, I should like to suggest polyketone https://en.wikipedia.org/wiki/Polyketone it's known to make gears more silent than POM and PA, its glass transition is near ambient temperature, its density and Young modulus resemble ABS, it absorbs little humidity. Still not widely used, it can become very cheap. Its creep behaviour and ease of manufacturing are unknown to me, but ABS and PP aren't brilliant neither. Marc Schaefer, aka Enthalpy

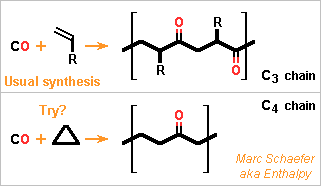
Failed! The usual polyketone is known to be ferroelectric, pyroelectric, optically nonlinear, etc. From: Asymmetric Catalysis from a Chinese Perspective, edited by Shengming Ma That's encouraging. The asymmetric one, with a C2 chain per period instead of C3, must be more strongly ferroelectric. I ignore if producing a polyketone with C2 chain per period is easy, nor if I did it, so may I suggest to try the polyketone with C4 chain per period? Less polar but unsymmetrical. The C3 chain per period is obtained from carbon monoxide and ethylene or propylene over a catalyst https://en.wikipedia.org/wiki/Polyketone so maybe cyclopropane instead of ethylene makes the C4 chain per period? One process sends the gases on warm solid catalyst, so more heat looks possible. Substituted cyclopropane can make a stiffer polymer chain. As usual, lamination helps put the macromolecules straight and parallel, drawing or extrusion too. Marc Schaefer, aka Enthalpy