Elen Sila

http://en.wikipedia....Volcanism_on_Io Would it be possible, through a combination of tidal heating, radioactive isotope decay, and the greenhouse effect, for a very large moon (like Titan) of a very distant planet (like Uranus or Neptune), to sustain earth-like temperatures and atmospheric pressure, as well as liquid water? How far out from the sun would this become infeasible? And, if feasible, how long could such a state exist, if sustained by an orbital resonance, as is the case with Io? A few million years? Billions?

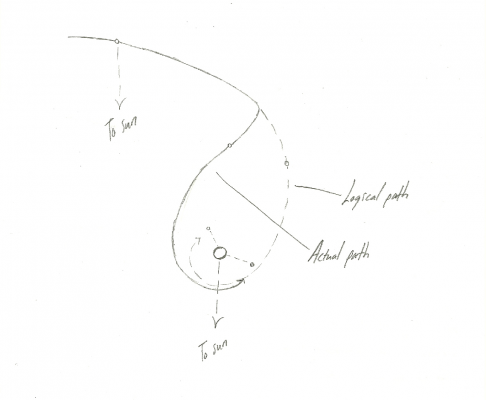
I'm aware that my conclusions are wrong. I am asking for an explanation of why they are wrong. Here were my two questions, originally, which I admit I did not enunciate very clearly. 1. Why do proto-planetary discs form in a prograde, rather than a retrograde, direction? 2. Once a prograde moon system has formed, why don't all of the moons fall into the planet due to head-on collisions with dust and rocks? My understand of your initial response, in terms of its practical application, is that objects being gravitationally captured do not follow the simple curves I described in my diagram, but rather perform a sort of "zig-zag" manoeuvre, where their motion relative to the planet reverses direction as they approach, due to some switch involving kinetic and potential energy that I don't really understand. Far moons would thus generally be hit in the face, dropping them in orbit, while near moons would generally be hit from behind, raising them in orbit, and resulting in the equilibrium you described. Is this a correct description of the mechanism of gravitational capture? Here's my attempt at a diagram. It shows the actual path of captured objects, as described in this thread, in a bold line, and and the logical path of captured objects, as described in my diagram, in a dotted line. If this diagram accurately expresses the system you've described, why does it work that way? What causes the reversal of direction?

They don't all have to be, they just have to mostly be. Since head-on collisions would be statistically more common, the moon would have a net loss of angular momentum. Our moon is a poor example, because it's so far out, and its orbital velocity is so low compared to the earth's (1.023 KPS versus 29.785 KPS), that it's actually usually moving approximately parallel to the earth around the sun, regardless of which way it's facing relative to the earth. Thus, it gets peppered on all sides roughly evenly. A better example would be, say, Jupiter's moon Io (17.334 KPS), or Saturn's moon Mimas (14.323 KPS), which both move around their primaries faster than they move about the sun (13.068 KPS for Jupiter, and 9.689 KPS for Saturn).

Why don't the moons always fall into the planet, as they lose kinetic energy to head-on collisions with dust particles, micrometeors, and asteroids? Is it because the angular momentum they steal from their primary's rotation makes up for it? Shouldn't one force or the other dominate the interaction, causing all moons to either break up or fly off, given enough time?

For reference, when I say "in the same frame", I mean "perceiving time at the same rate", as measured using the signal-timing method I described before in this thread.

No, I've totally heard of scientists creaming their pants over binary asteroids or double-dwarf-planet systems like Pluto and Charon. ... Yes we can know the masses of exoplanets. We just measure the back-and-forth motion of the star. How long it takes to move back and forth tells us the orbital period; and how much it moves back and forth tells us the mass. But wouldn't that just tell you the combined mass, as per g(m₁+m₂)/r²? Why would that tell you the ratio between the masses, as well, as I've often heard it's supposed to? OR WAIT. Of course you'd be able to tell the ratio between the masses! You'd be able to see the motion of both of them around the common center of mass; and then you'd just measure the ratio of their distances to the center of mass. Gosh, I'm dumb sometimes. Alright. I see what you're saying. Confusion cleared up.

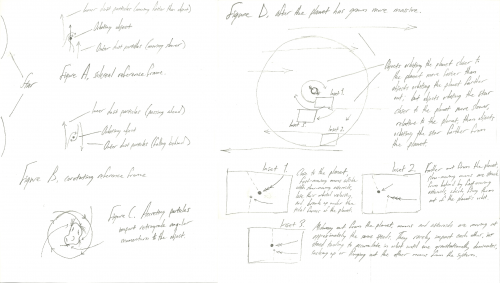
During the infancy of a planetary system, it usually consists of a young star, surrounded by a disc of spinning gas and dust. Eventually, turbulence in the disc results in the accumulation of clumps of matter, which over time grow into massive objects, which can eventually become planets, if they become so great as to achieve hydrostatic equilibrium and clear their orbits. Now, early on, before the planetlets have come into their own as rulers of a Hill sphere, the matter passing by them in adjacent orbits would be travelling faster if closer to the primary, and slower if farther out; thus, from a corotating reference frame, outer dust particles should be moving backwards, and inner dust particles should be speeding ahead. So, logically, per the diagram below, matter should, as it is accreting to the orbiting object, be imparting retrograde angular momentum to the object. Then, after the orbiting object has grown to the mass of a full-fledged planet, collisions and interactions with passing asteroids should destroy or eject all moons from the system, except those orbiting at approximately a certain ideal distance from the primary. Thus, every planet should, 1, rotate retrograde, and 2, have only one or two large moons, both of which should, 3, orbit retrograde. So why are none of these three things true? (In case you can't read my handwriting, here's a transcript of the diagram.)

Oh, whoa. I just realized. For any given object in keplerian freefall, the semi-major axis of the orbital ellipse is the distance at which centrifugal force exactly equals gravitational force. And, by extension, the gravitational mass of a planet is exactly equal to the sum of the mean orbital distance and the square of the mean orbital velocity of any given satellite of negligible mass. v²/r=gm/r² gm=rv² As I understand it, astronomers really like it when they find two objects of comparable mass, orbiting each other, because somehow it helps them determine the mass of the system. I would think it would be more helpful to find an object with a negligibly small object orbiting it; why is this not preferrable?

So, 0.026758–64.7^2/192000=0.004955; at Sylvia's equator, then, the effective gravity would be only five millimeters per second per second? On the earth, (398600.4418*10^9)/6378137^2=9.798285 meters per second per second; but 9.798285–(6378137*pi*2/86164.1)^2/6378137=9.764370 meters per second. The value Wikipedia gives is 9.780327 meters per second per second. The difference is small; but I'm not understanding where it's coming from: 398600.4418 is known to be the standard gravitational parameter of the earth to a very small margin of error; and 6378137 is likewise known to be the equatorial circumference of the earth at sea level in meters to within a very small margin of error; yet I produced a somewhat large margin of error as my result, both with centrifugal force and without it. I don't get it.

Can you put yourself in the frame of an object that is accelerating gravitationally, through orbit? Wikipedia says that freefall is inherently inertial. "Objects in free-fall really do not accelerate, but rather the closer they get to an object such as the earth, the more the time scale becomes stretched due to spacetime distortion around the planetary object (this is gravity). An object in free-fall is in actuality inertial, but as it approaches the planetary object the time scale stretches at an accelerated rate, giving the appearance that it is accelerating towards the planetary object when, in fact, the falling body really isn't accelerating at all. This is why an accelerometer in free-fall doesn't register any acceleration; there isn't any. By contrast, in newtonian mechanics, gravity is assumed to be a force. This force draws objects having mass towards the center of any massive body."

Hmm. I just came up with another scenario. Let's say Sam and Mary are both in space stations, orbiting the earth at the same altitude; Sam is several degrees ahead of Mary. In this scenario, Sam and Mary are not travelling in the same direction linearly, in the galilean/newtonian sense; however, they are travelling in the same direction geodesicly, in the einsteinian sense (at least, based on my understanding of the geodesy of spacetime according to Einstein, which I'm willing to bet is probably even worse than my understanding of time dilation). It seems to me that, in the curvature of spacetime by the earth's mass, Mary is following directly in Sam's path, and therefore her experience of time relative to him ought to be the same as if they were both travelling in a straight line, one after the other. Are they in the same reference frame in this scenario? Or am I totally misinterpreting the concept of spacetime geodesy?

... Wow. I'm so used to Wikipedia giving me the radii that I didn't even realize I was looking at the diameter. I hate diameters so much. Radii are so much more useful and relevant. I keep 2pi saved to my calculator as a constant, and almost never use my calculator's Pi button. Yeah, I get 64.6 meters per second for the equatorial rotation speed, at an equatorial radius of 192 kilometers. The orbital period at that distance would be 16831 seconds, and the orbital velocity would be 71.7 meters per second. So I guess the rotation speed isn't greater than the orbital velocity, and no negative gravity would be present. I'd still like to know how to calculate centrifugal/centripetal force on a rotating gravitational body, though. For the record, when you say "centripetal (inward) force", are you referring to, for example, the force of the floor of a rotating space station against the feet of an astronaut standing in it? Also, when you say "orbiting body", do you mean "body in freefall" or "rotating body"? (6.67384*10^-11)*(1.478*10^19)/192000^2, sure enough, is 0.026758 meters per second per second. However, 192 kilometers is only the asteroid's widest equatorial radius. Its mean radius is 143 kilometers, and its polar radius is 116 kilometers. If we plug in the mean radius, we get a surface gravity of 0.048237 meters per second per second; and if we plug in the polar radius, we get a surface gravity of 0.073305 meters per second per second. If we reverse-figure from 0.0729 meters per second, keeping the stated mass, we get a radius of 116.322 kilometers. I'm guessing the article is using the polar surface gravity; and since the polar surface gravity wouldn't be subject to the same centrifugal concerns, they wouldn't even have to factor that in. I'd still like to know how to calculate Sylvia's equatorial surface gravity though, accounting for centrifugal force.

So there's this asteroid called Sylvia. (It's primarily known for having two moons, Remus and Romulus, but that's beside the point.) Sylvia rotates about once every 18661 seconds, sidereally; and her mean radius is about 286 kilometers. That means that, at her equator, assuming approximate sphericity (obviously a big assumption, but that's also beside the point), she rotates at about 96.3 meters per second. But, assuming an object of negligible mass could revolve around her at a non-eccentric distance of exactly her mean radius (again, implausible, but let's not get hung up on that), that object would have an orbital period of (286000^3/(1.478*10^19)/(6.67428*10^-11))^(1/2)*pi*2≈30598 seconds, and an orbital velocity of about 58.7 meters per second. If Sylvia's surface rotation speed is greater than her surface orbital velocity, shouldn't Sylvia have a negative surface gravity? If an astronaut wanted to land on her surface, wouldn't he have to use mountain climbing gear just to keep from flying off into space? Why does Wikipedia list Sylvia's surface gravity as 0.0729 meters per second per second, instead of accounting for centrifugal force in that figure? And does Wikipedia account for centrifugal force in its figure for the earth's gravity (9.780327 meters per second per second at sea level on the equator)? If so, why the inconsistency? Anyway, my main question is this. I know how to calculate surface gravity on a non-rotating object. But how do you calculate Sylvia's (or any other body's) actual surface gravity, accounting for centrifugal force? By extension, how would you measure the artificial gravity in a rotating space station, in meters per second per second? http://en.wikipedia...._%28asteroid%29 Pic related, it's Sylvia.

I'm not even sure. I do know this, though: if the earth suddenly disappeared, leaving Sam and Mary floating in space, Mary would go flying off into the distance way faster than Sam would. That, at least, shows that they do not have the same inertial rest frame.

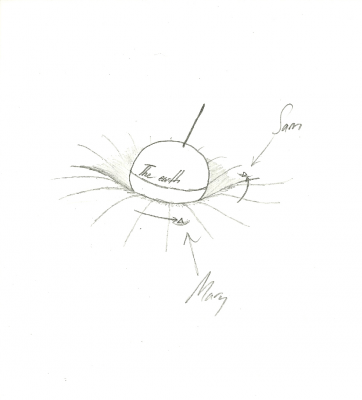
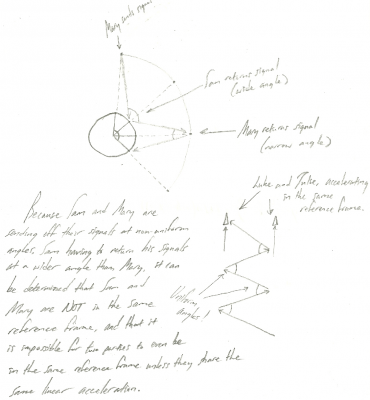
Alright. I suppose that makes sense. I still have a lot to learn, of course. But I guess my question appears fairly cleared up now. Also, while I was thinking about this scenario at school the other day, I came up with the following diagram, which actually kind of demonstrates that Mary and Sam must have a non-zero relative velocity. Let me know if this diagram is accurate, and really does have anything to do with the scenario and its solution. Again, in case you can't read my handwriting, it says, "Because Sam and Mary are sending off their signals at non-uniform angles, Sam having to return his signals at a wider angle than Mary, it can be determined that Sam and Mary are NOT in the same reference frame, and that it is impossible for two parties to even be in the same reference frame unless they share the same linear acceleration."

And that makes sense to me. What I don't understand is these statements. "Again, you must be careful here. Acceleration, in of itself, does not cause time dilation." "A Janus has pointed out, it's due to the speeds, which are not the same." I will explain exactly what's causing the confusion on my part. Velocity is not absolute. You cannot simply say "Mary is travelling faster than Sam", because she's not. Nothing travels "faster" than anything else, in absolute terms. You can only determine relative velocity, in which case, in this scenario, Mary and Sam have zero relative velocity, compared to each other. Mary does have greater acceleration, and Sam is standing deeper inside a gravitational well; so those are two absolute reasons why you could say that one or the other is experiencing time dilation relative to the other. But it seems to me that, in this scenario, the only time dilation that can be happening is acceleratory or gravitational. Yet the statements I quoted, from you and Janus, seem to indicate that time dilation is occurring for a reason pertaining to velocity, which, as far as I can see, cannot be the case.

Alright. Just answer this question then, I guess. If, when time dilation occurs, both parties view the other as moving slower, and difference in time elapsed only results when, through acceleration of one or both parties, the two reference frames are reunited, why should there be a difference in time elapsed if neither party accelerates to reunite with the other, and if they both maintain the same positions relative to each other, communicating remotely?

So, even if they account for the gravitational time dilation effect, there will still be velocity-based time dilation, even though Sam and Mary are stationary relative to each other? See, that makes sense to me. If the remaining time dilation in the Sam-Mary scenario, once gravitational difference has been accounted for, is due only to acceleration, then it makes sense.

So any 2:3 or 1:2:4 resonance will be self-correcting? The only requirement is the orbital ratio, nothing else?

I wasn't sure if this belongs in "Classical Physics" or "Astronomy And Cosmology". Anyway, according to Wikipedia, "In most cases, [orbital resonance] results in an unstable interaction, in which the bodies exchange momentum and shift orbits until the resonance no longer exists. Under some circumstances, a resonant system can be stable and self correcting, so that the bodies remain in resonance." I've been wanting to write a science fiction story for a while that takes place on a planet with two moons, and I wanted the moons' orbits to remain roughly stable over many millennia, but am unsure whether to attempt to fit them into a resonance, or whether this would detriment the stability of the system. I've been using approximations of what I want their respective orbital periods to be, and these happen to be fairly close to a particular integer ratio. I'm unsure as to whether I should actually have them be resonant with each other, though. How can I determine whether a given resonance between two moons of known mass, around a planet with a known mass, will be stable or unstable? What sorts of things will I need to consider? I'm imagining orbital inclination is probably important, as well as the planet's proximity to its sun. What are some factors that can contribute to a strong resonance? (The reason why I need to compute the system's stability accurately is because I need to design a calendar for the story.) (And yes, I will probably be developing a computer simulation of the system at some point; but I'd like to minimize trial and error as much as possible in setting it up.)

You don't seem to be understanding the scenario I've set up. Mary, upon achieving geostationary orbit, sends the following message to Sam, directly below her on the equator. "Dear Sam. My atomic clock will start running the moment I send this message. Please start your atomic clock the moment you receive this message." Sam, upon receiving this message, sends the following message back. "Dear Mary. Message received! My atomic clock will start running the moment I send this message. Please stop your atomic clock the moment you receive this message." Mary, upon receiving Sam's response, sends the following message back. "Dear Sam. Thanks! My atomic clock recorded that X milliseconds passed between my sending my message and my receiving your response. Please stop your atomic clock the moment you receive this message." Sam, upon receiving Mary's response, sends the following message. "Dear Mary. Good work! My atomic clock recorded that Y milliseconds passed between my receiving your first message and my receiving your last message. See you when you get back to earth!" If X is less than Y, then Y cannot be less than X. One of them must be perceiving time faster or slower than the other, or else they must not be experiencing time dilation.

Confusion as to how it can be real (albeit recognition that it is), and profound desire to one day meet a professor or layperson who can explain it to me in terms I understand. This desire manifests in the form of visiting science forums to ask people hypothetical scenarios, in the hopes that someone's explanation of one or more of them might be the explanation that finally gets my understanding to "click". Basically, I can understand time dilation as an effect of acceleration, or of being in a gravitational field. That would be some sort of absolute, physical effect. What I can't understand is time dilation as a mere product of being in different reference frames (except as a Doppler effect) – in particular, when you can compare reference frames that are in uniform circular motion, using the signal-timing method I've described in this thread. The signal-timing method I've described should allow for comparison of time passage without needing to change reference frames; and what, logically, it should show, is that, minus gravitational or accelerative effects, time dilation does not occur between people just because they're in different frames. Obviously, that's not what actually happens. And I recognize that, but don't understand it. I imagine any one of you could probably explain it to me if we were in person; I tend to be a pretty visual thinker (hence the uploaded diagrams). I'm trying my hardest to glean understanding from your responses, but I'm still just drawing a blank – hence, why I keep asking different hypothetical questions. Sorry if I'm wasting everyone's time.

But they're explicitly not doing that in my scenarios. Nobody is changing speed or direction. They're comparing passage of time by sending signals back and forth and comparing the time intervals they perceive between receipts of the signals. So, Mary sends a signal to Sam, then Sam sends a signal back, then Mary tells Sam how long it took him to respond, from her perspective, then Sam compares this to the time he measured between signals. Thus, they are able to compare passage of time without changing their speed or direction.

Sigh. I guess not. Alright. A couple miscellaneous questions then. 1. Would an atomic clock on the north pole and an atomic clock on the equator run at different speeds (as measured by timings of signal exchanges)? 2. Let's say the atomic clock on the north pole is located on a platform that rotates clockwise once every sidereal day, so that the clock is essentially standing still with respect to the center of the earth. Now let's say you have a second atomic clock on an airplane that's flying westward along the equator at an altitude of 10 kilometers and a speed of 1677 kilometers per hour, thus also standing still with respect to the center of the earth. If a system of radio towers is set up to relay signals back and forth between the plane and the outpost at the north pole, and the operators on the plane and at the north pole both compare their measurement of the timings of signal exchanges, will there be a difference in the perception of time between the plane and the pole?

And as per my previous posts, if the answer is an unqualified "no", then I don't understand relativity theory. Now, I could accept a qualified no. For example, time dilation in the first scenario could be justified in that the craft must be constantly accelerating by firing its engines towards the sun; and the time dilation in the second scenario could be justified in that the craft, while maintaining the same distance to the earth, is not constantly travelling in the same direction. These would be qualified reasons why the satellite and the earth would not share a parallel experience of the passage of time. Let's take a third scenario, much simpler and more topical than my first two. I am living in an observatory located in Ecuador, at zero degrees' latitude. Directly over my head, night and day, there is a geostationary satellite, hovering at an altitude of 35786 kilometers. At any given moment, if you were to draw my straight-line trajectory, and the satellite's straight-line trajectory, the two lines would be parallel; we are travelling in the same direction. Likewise, at any given moment, if you were to measure my distance to the satellite, and compare it to the distance between me and the satellite at any other given moment, the figure would always be the same. That satellite and I are maintaining a constantly equal distance from each other. Now obviously we're separated by 119 milliseconds of light-time (239 milliseconds both ways), so it's not fair to say we're in the same reference frame, per se. Nevertheless, by passing a signal back and forth between us, and comparing our measurements of the passage of time between receipts, we should be able to determine that, all other considerations (such as difference in gravity) ignored, we are experiencing the passage of time at the same rate, and no time dilation is occurring. Now, that's not to say there couldn't be a difference in time perception for gravitational reasons; after all, I'm about 6.6 times closer to the earth's center of mass than the satellite is; so the earth's gravity is pulling on me about 43.7 times harder, by the inverse square law. And it would make sense, if that geostationary satellite were going to be used as a global positioning satellite, to take relativistic time dilation into account, if for that reason only. But, after having accounted for gravitational time dilation, it doesn't seem to me like any time dilation should be incurred due to difference in relative velocity.