Acme

It would not be wise; it would be rather stupidly flawed. And what kind of parent kills their own offspring anyway?

How many words can a quick question have?

__Alrighty thens. Since last posting we have had to deal with a mole which did a number on the garden beds. Took 2 weeks, 2 kinds of traps (one useless), and poison pellets (also useless) to put the kibosh on the pest. __Then too, though not bothering the garden, we have finally managed to wipe out a family of rats that had taken up residence in the compost pile. Well, there might be a baby or two still around, but they won't last long. We took these out with a combination of stomping, whacking with shovel, pitch forking, and a pelletgun. Good times. __Anyway, here's a pic of today's harvest and I'm thinking of a baked ratatouille ala Acme. Bon appétit.

Whut guvnor!? She bloody well does sound like that.

The Americans told you Brits to quit taking and using what you don't have a right to. Kinda like those copyright pictures you posted. Some peoples' kids.

A pig by any other name would still smell as bad regardless of whether or not it is wearing lipstick. What a freakin' waste of our time and bandwidth.

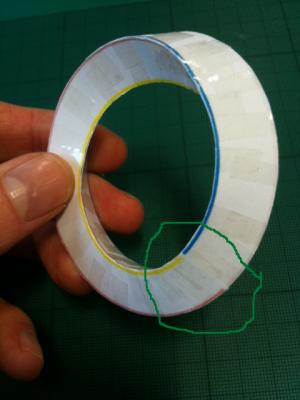
Okaly dokaly. I have my answer. First I should say I made a mistake earlier when I said: It is 2 bands interlaced, but with 2 twists each just as the Wiki article stated as the general rule for bands with even numbers of 1/2 twists. That out of the way, I managed to put together a copy of imatfaal's ring and then cut it. The result is a single ring with 2 twists. As imatfaal said, cutting it into a strip again and flattening, it looks like the starting strip but with some lumpiness. 'Course I haven't imatfaal's experience or skill with paper & tape and my starting ring is rather lumpy. So without further ado, here's a link to my video of the cut. (I started untwisting the wrong way at the end and decided to end the recording so as not to appear more of an idiot than I already do. ) Edit: Apparently Flickr has a 3 minute video length limit, so you don't get to see my fumblage. Video of Prismatic Ring Cut: >> https://flic.kr/p/ods5We

Yet another example of why a Religion section has no place on a Science forum. Get a clue.

Roger. But as your ring is hollow, it is not a lump of clay and so it is unique. And back to my other, still burning, question; how many twists in your 'set' when it's simply closed end to end? Also, when you assemble the ring do you join the ends first, or the edges first and then finish with the ends join? So, see attached images of imatfall's construction. I brightened the 3 colors on the unconnected strip pic where I was pretty certain of them. The green arrows that I added indicate a possible end-to-end join. Keeping these ends flat on the table and sliding them to join so that blue-goes-to-blue, there are no twists in the loop so formed. If a half-twist is made in the strip and the ends joined, the strip is now a Möbius band and there is no blue-to-blue match because 1 blue is up and the other is down. (Note the twist can be right or left and that is important because Möbius bands are chiral, i.e. there is a left-hand and a right-hand version.) If a full twist is made in the strip and the ends joined then you get a blue-to-blue mating. (This may or may not be chiral; it's not clear to me yet.) In case it's not clear I still haven't managed to get the damn thing together. So also on the strip image I added a green circle around an area where red, blue, and yellow end/begin. In the second image of imatfall's completed ring I have circled in green a similar area. Are these one-and-the same area? If so I might manage to start some edge taping there. If not, I'm screwed still. I do hope this post isn't appended to my last; I waited purposefully to try and prevent that. 'Course it's not like things have been going my way today, so I'm not holding my breath. EDit: Crap!! Didn't wait long enough. Foiled again.

OK Found the zoom. Still, there are a bunch of numbers within your color range that are uncolored in the lower 'half' of the plot. A garden path is as a garden path does, or... a mindless slave is as a mindless slave does, if you prefer. [if you are not aware of the idiom "led down the garden path", see here. >> garden path

OK good! Now my question is, with the set joined at the ends but not yet at the edge(s), how many twists in the set, i.e. is the set-joined-at-the ends just a simple loop with no twists, a Möbius band with 1/2 twist, a loop with 1 twist, or what? PS I think it's worthwhile noting that your ring actually has 2 surfaces and 1 edge inasmuch as there is an inside and outside surface. Or am I still missing something?

Thanks. I did see the faint color markings on the edge of your strip on the table-top photo, but I made my printout in grayscale and they didn't reproduce. Even looking at the color pic now I haven't figured out the assembly. (Add to that confusion my discovery a short while ago of a rat nest in the compost pile and chasing babes with a shovel, and it could be a while before I get this settled. ) What do you think is the result of the single cut I wanted? (presuming you understand now what I meant.) Was my guess right? Is the result equivalent to any 'simple' loop cut in my vid? Just caught your edit. Yes, realized the crossover required a filler piece and I got that made & put into the strip. I will have a go at enhancing the colors in your photo and transferring them to my strip. The cut I want is along the surface and parallel to the edge; follow Janus' ball-bearing with your scissor, then flatten the edge/vertex so it is a surface.

With all our British pals here, I was wondering how they felt about us celebrating giving ol' George III the... well uh, benefit of our opinion of him. British Thanksgiving? Next big holiday in US is Labor Day on September 1st.

Ack!! I can't quite get my head around it. Two cups of coffee and I still can't assemble my ring. So on just the first cut, -the one that I wanted- is the result like any of the cuts I did of 'simple' loops in my vid? (For some reason my cut of the double-twist got cut out of the Flickr version of my vid; perhaps a time limit.) Anyway, I guessed that cutting imatfaal's ring as in your #1 would be equivalent to my 1 1/2 twist cut [with the vertex flattend and becoming a surface]; was I right? Help me Obi Wans! Edit: Erhm...given your second cut along the vertex, am I supposed to be using 2 of imatfaal's planar strips to build the prismatic ring? Doble ack ack!! :doh:

As long as you let someone know where you are going so that when you don't return on schedule they will know where to look for you. Most of these unexplored regions do not get cell phone reception. Well, sorta like lower Wythoff insomuch as the large purple triangles go. But both Wythoff sets go to all purple and yellow on the 3rd iteration and non-polys don't go all purple & yellow until the 8th iteration. For some reason the hailstone numbers are incomplete/uncolored in that file. ?? I haven't figured out how to zoom in to read the values.

You're right; hard to see. As I earlier said, I'm doubtful these mappings 'mean' anything. Hiking the garden path is fun, but know when to turn around and head back. Did you model the set of not polygonal numbers in post #22? Your post #23 is empty.

Watch this video that I posted in post #10; it illustrates the cuts : >> https://www.flickr.com/photos/114331103@N07/14505951965/ My post #11 has a description of the cuts from the Wiki article on Möbius strips. I got out my printer and printed up several copies of imatfaal's strip. I have 1 cut out & patched and today I will try and tape the little bugger into the prismatic ring. Good times. Janus: Trey kewl POV! Can you model my proposed cutting of the prismatic ring?

You just said in that 'last' post: It's not a 'poorly worded' error by Bond, it's a giant blunderous misunderstanding of how cosmic rays interact with the Earth. Most of these rays -as well as other energetic particles that carry the Sun's magnetic field to Earth- are neutralized in the atmosphere and never reach the ground. Since your major premise seems to be that the Sun's magnetic field is inducing a current in the Earth's iron core and heating it, then thinking C14 supports this premise is unfounded. Spare me the usual wall of text rebuttal reasserting your erroneous & unfounded hypothesis and go ahead and get back to answering ophiolite's and billiard's objections.

I agree that the number of elements in the initial condition will have an effect on the ending. So much so in fact that I'm not sure there is much analytic value in these constructions, cool looking as they are. Muchas gracias. As I mentioned in the Primes thread, be sure to let out a string as you go so you can find your way back out. On the Polygonal note, here is the sequence of numbers not Polygonal. It contains all the Primes as they are never Polygonal as well as even and odd composites as I already gave earlier. Sequence of numbers not Polygonal: {7 8 11 13 14 17 19 20 23 26 29 31 32 37 38 41 43 44 47 50 53 56 59 61 62 67 68 71 73 74 77 79 80 83 86 89 97 98 ...}

The 'planar map' for imatfaal's ring is in post #5. My printer is put away just now but my plan is to print out a copy and try putting it together so I can cut it apart & see the result. For things like this my minds eye is not to be trusted. As to your clay constructions, I'd say they are substantially different than the paper constructions like imatfaal's ring as they have no interior, i.e. they are not hollow. ajb is our topology expert so maybe he will drop in to correct us or you could ask him directly if you like.

You da man! I like those last two a lot. I'm making up my sequence of numbers polygonal 5-ways, but I'm on beer #2 so give me a few minutes. . In for a penny, in for a pound. Automaton me! Sequence of numbers polygonal 1 way: {1, 9, 10, 12, 16, 18, 22, 24, 25, 27, 30, 33, 34, 35, 39, 40, 42, ...} Details: Sequence of numbers polygonal 2 ways: {15, 21, 28, 51, 55, 64, 70, 75, 78, 91, 100, 111, 112, 117, 126, ...} Details: Sequence of numbers polygonal 5 ways:{561, 1485, 1701, 2016, 2556, 2601, 2850, 3025, 3060, 3256, 3321, 4186, 4761, 4851, 5226, ...} Details:

. Addendum: Continuing with beer in hand, Wolfram is mightily screwed up me thinks. They say: 15 is only polygonal 2 ways. 15 2 [3, 6: 5, 3] (the 3rd 6-sided number and the 5th 3-sided number.) 16 is polygonal just once; a square, i.e. 4-sided number. Also they screw the 3-way, which may entertain & per se amuse you peepers no end. Here's my ménage à trois listing. Sequence of numbers polygonal à trois: {36, 45, 66, 81, 105, 120, 153, 171, 190, 196, 210, 261, ...} details: It says up to some limit, and the example simply stops at 4 followed by an ellipsis. .Addendum addendum: (curse you appending editor! ) Anyway, whether or not Wolfram is talking about something else than I is irrelevant to my sequences. Mine are correct as given. If Wolfram is talking about something else then by all means show how they generate the sequences and submit those sequences for Sunshaker's automaton.

I get the impression this thread has become peepers watching two addicts feeding the other's addiction in a perfect symbiotic relationship. I need a beer to wash some of this down. But first, this sequence is from my own research. I thought I had seen it at OEIS once, but searching just now they say no. Curiously, Wolfram on their Polygonal number page explicitly claim such numbers don't exist. Sequence of numbers Polygonal in 4 ways: {225, 231, 276, 325, 435, 540, 595, 616, 651, 820, ...} details:

D'oh! Trey kewl. How are you making these graphs? Would your error -if there is one- be just in entering the initial line? Here's some more sequences for you. Padovan sequence: {1, 1, 1, 2, 2, 3, 4, 5, 7, 9, 12, 16, 21, 28, 37, 49, 65, 86, 114, 151, 200, 265, ...} @ OEIS Beatty sequences including: lower Wythoff sequence @ OEIS {1, 3, 4, 6, 8, 9, 11, 12, 14, 16, 17, 19, 21, 22, 24, 25, 27, 29, ...} upper Wythoff sequence @OEIS {2, 5, 7, 10, 13, 15, 18, 20, 23, 26, 28, 31, 34, 36, 39, 41, 44, 47, ...}

Feeeed me! How about the sequence of Prime Gaps? These are distances/differences between Primes. {1, 2, 2, 4, 2, 4, 2, 4, 6, 2, 6, 4, 2, 4, 6, 6, 2, 6, 4, 2, 6, 4, 6, 8, 4, 2, 4, 2, 4, 14, ...} Longer list here: >> Prime Gaps @ OEIS . Addendum: Speaking of ending with 1, you may have some fun with the hailstone numbers. Collatz Conjecture The following set are the numbers that set records for number of iterations before reaching 1. {1, 2, 3, 6, 7, 9, 18, 25, 27, 54, 73, 97, 129, 171, 231, 313, 327, 649, 703, 871, 1161, 2223, 2463, 2919, 3711, 6171, 10971, ...} source @OEIS