studiot

So you are claiming that all those relativity calculations about spaceships travelling through 'empty space' are incorrect ? Think again more carefully. Isn't that way off topic ?

I don't see why this is off topic. It all depends upon the sense in which the ill defined OP was made. For instance, since the OP refers to both 'anything' ie something and empty space the empty space must be limited in some way. You cannot have 'anything' in empty space by definition. You could have something in otherwise completely empty space. Or you could have something surrounded by an extent of empty space or... This is a mind expanding question.

fazakerley But the screen and its display are not the mouse. And the mouse is very visibly (by other means) moving about in the air. (I am not distinguishing between air and empty space in this instance). As a matter of interest I have a mouse for presentations that does not do this but still moves the pointer across the screen.

Your mouse is obviously better trained than my mouse and equally obviously misinterprets what I said. Shame.

Although swansont and others are technically correct in one sense, In another sense, isn't that how a computer mouse works?

My dog changes from somnolent to very intelligent and communicates most successfully with me every mealtime and walkies time.

I would suggest the way the question is phrased misses a trick from our own historical experience. You suggest one language, and each language on Earth is unique because it doesn't need to be otherwise. Yet it is known both from deciphering codes and the experience with the Rosetta Stone that having more than one different representation of the sme thing in the single most useful thing to have. Consequently our communication attempt should contain not one, but many, 'languages' for comparison. Edit cross posted this this. +1

Thank you so much. +1 For a long time I have been trying to remember which character said that - I thought it was the walrus.

You introduced a Mars Rover. I asked you two straightforward uncontroversial questions and provided quite a bit of useful information about both planetary thermodynamics in general and its application to both Mars Rovers and this thread. You have failed to respond. Is there a problem ?

Apart from the fact that you claimed to understand when I started to explain about derivatives and began with the answers to these questions, I also suggested you should spend more of your time reading what was written properly and then digesting it. I have highlighted the difference between MigL's correct statement and your all-too-quick snap response. The difference between 'and' & 'or' is so huge they are nearly opposites. Not only that but this difference appears in many many places in logic, maths, physis and science in general as well as being one of the few special words that are exactly the same in plain English as formal scientific language. Please learn to walk before you try to run. You will make much faster progress that way. Another example would be that you did not pick up the difference between your opening statement and my correction for you.

No it doesn't work like that. Either "per second squared " or "seconds per second" note the plural in the first seconds in the second phrase. Both are correct and mean the same thing. but not second per second squared.

Did you say you were some sort of engineer ? Surely you understand that engineers use parameters that are single numbers to describe an environment or regime for instance Reynold's Number in fluid mechanics distinguishes between quite different mechanical environments in fluids. In the same way the average surface temperature can form part of another dimensionless environment number ( Xi) that indicates whether a planet has an atmousphere at all and, if so, what the gases are likely to be. [math]\Xi = \frac{{3{k_B}T}}{{4\pi G{r^2}\rho u}}[/math] where kB is Boltzman's constant, and u is the atomic mass unit = 1.66054 x10-27 kg Ref Douce : Thermodynamics of the Earth and Planets : Cambridge University Press. Douce gives a derivation and interpretation of Xi for all the planets and some moons on pages 616 -620 The other informatom contained in this parameter, I would suggest would be very useful for someone designing a rover as it contains the gravitational potential energy at the surface, (That is it compares the gravitational potential to the escape kinetic energy via Boltzman) Which I would think very useful in stability/ traction and other mechanical considerations for a rover, apart from the obvious materials considerations of the operating temperatures such as brittle transition temperature/ phase change temperatures etc etc. What were you saying about climate change and what does that have to do with a mars rover ?

Wouldn't it be nice to get AJB back, that's his field. Please note the ability of this site to do subscript and superscript This is unusual even in scientific and maths forums. Look for the x2 and x2 on the entry editor tool bar.

Data logging has been around almost since as long as computers. So clearly it was and still is a desirable objective. I remember constructing data loggers (for chemistry and other purposes) for my Commodore Pet and I still have the interfacing manual - it is a valuable source of information. When the small microcomputers (pic, arduino etc etc) came out, there were a number of projects in schools for single board loggers connected to all sorts of input sensors, including wet chemistry ones. I'm sure I still have many of those articles from elctronics mags before they stopped publication. So yes it can be done, it has been done but there is still plenty of demand out there so go for it.

Numbers are more fun than just N → Z → Q → R Consider the equation x2 - 2 = 0 This has no solution in N, Z, or Q In other words there is no rational solution But let us propose a solution and represent it by the character £ Now taking inspiration from the form of complex numbers (a + ib) let us consider expressions of the form (a + £b) We will find that we have a complete number system that follows all the four rules you mention and the equation has a solution in this number system! This number system is the usual rational numbers plus one irrational number, £. I have disguised the fact that we usually call £ the square root of 2. So number systems exist partway between Q and R. Our simple system does not contain a solution to the equation x2 - 3 = 0 as it cannot write the irrational number square root of 3 in terms of root 2.

A buffer solution is an equilibrium mixture of either a (weak) acid and its conjugate base or a (weak) base and its conjugate acid. So firstly you need to identify the acids, bases and conjugates in the two situations and whether they are strong or weak ? Have you done this ? Pudue University migh help you here https://chemed.chem.purdue.edu/genchem/topicreview/bp/ch17/mixtures.php

An orbital is not an electron and vice versa. An electron is a physical phenomenon that can be interacted with. An orbital is a mathematical description of a region of space defined not only by a general equation (the Schrodinger equation) but also by assiciated boundary conditions. The equation is the same (although coefficient values are different) but the boundary conditions are very different for the near and far situations you describe.

Well you really have got me there. What on earth do you mean ? 6 dimensions of the box?

Well the short answer is they don't need a very high level. This is compounded by the curriculum taught in too many places. I think everybody should learn how to wire a plug, change a fuse, read an electricty meter, a wage slip, a bank statement or a till receipt. None of these are commonly taught in school, as are not too many more scientific or technological life skills.

I think there are already too many vagaries in this thread.

Have you not answered your own question ? Surely the average is useful for some purposes but not for others in both cases. So clearly knowledge of the value of that average alone is worthless without knowledge of the intended use. I see that you joined 13 hours prior to this post and have already made 5 posts here. Welcome, but I expect you don't realise that new members are allowed only 5 posts in their first 24 hours. After that they can post normally. This is a (sadly) much needed anti spam measure So I look forward to your clarification of your topic in around 11 hours time. I don't agree that this conclusion follows from that premise for either the car or the Earth's average 'temperature'. Both contain internal heat sources.

To continue where I left of and pick up and develop joigus points about limits The f(x) and y =y(x) notation comes in useful if you want to get it all onto one line so the derived functions become f'(x) and y'(x). This is not directly related to any of the d notation variants and also becomes very clumsy when many variables are involved. So let us look at the d notation, which is, after all, what you are asking about. So we have Greek capital delta [math]\Delta [/math] Greek lower case delta [math]\delta [/math] Roman Capital D Roman lower case d Eighteenth century European stylised script d [math]\partial [/math] We have all of them because each perform a different operation (remember them) on the symbol that follows them. The first one is not important to us because we use it for the difference between two specific values of the variables represented by the symbol that follows. So the difference in height, [math]\Delta h[/math], between the ground and the gutterline of my house is 18 feet. [math]\Delta h[/math] may be large, but must be exact. That is it is a number. As it is a number it can legitimately be zero. The second one is important to us because it is not only about small differences in the values of the variable that follows it, it is about arbitrarily small differences. This means that [math]\delta x[/math] is not exact but may be made smaller and smaller indefinitely. So it is not a number it is a sort of function. Also since it is not a number it can never be zero, since zero is a number. This brings us neatly to the idea of limits. This is a sequence [math]1,4,9,4,16,...[/math] If we put in addition signs it becomes a series [math]1 + 4 + 9 + 16...[/math] And if we work out the differences between each term or the changes from term to term [math]3\quad 5\quad 7[/math] The three dots at the end is the convention for continuing indefinitely. It can immediately be seen that all the values for both the sequence and series and for the differences increase with each additional term added so each get larger and larger. This is called divergence and the sequence and series are called divergent. Such series do not have limits. If however we take the reciprocal of these sequences they get smaller and smaller, whilst the partial sums for the series gets closer and clsoer to a specific number (1.64) [math]\frac{1}{1} + \frac{1}{4} + \frac{1}{9} + \frac{1}{{16}} + ...[/math] [math]1 + 0.250 + 0.111 + 0.063...[/math] [math]0.75\quad 0.139\quad 0.048[/math] This behavious is called convergence. The specific number is called the limit. Because the process can continue indefinitely we write [math]\mathop {\lim }\limits_{n \to \infty } \sum {\frac{1}{{{n^2}}}} = 1.64[/math] Next time, this will lead directly into the limits [math]\mathop {\lim }\limits_{\delta x \to 0} [/math] and [math]\mathop {\lim }\limits_{\delta x \to 0,\delta y \to 0} [/math] So we have numbers, functions, variables, operators, limits sequences and series all mentioned before. An easy way to look at operators are that simple functions work on numbers to output other numbers and operators work on simple functions to output other functions. You will need to do some work on these to use all the to get a handle on what calculus is, what it can do for you and what it can't do for you. So please let us know any ideas in this development you didn't get a hold of properly so we can help correct that.

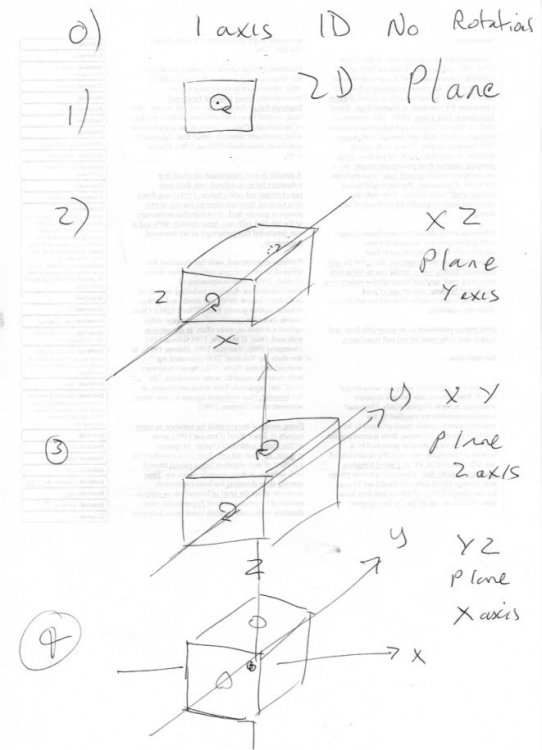
Gosh you are a hard person to keep up with on ideas. I see you have started another thread this morning. I seriously recommend you at least get to a sensible pause point with each one before moving on, we still have a long way to go in your calculus one. Anyway swansont has answered your question but here is more on my comment. Here is a brilliant experiment you can easily perform to gain insight. You will need a cardboard box with all six sides intact. Here is a quick blackboard sketch. Rotations can be represented by complete circles. Consider first one single space dimension. There is nowhere for rotations to occur. You have to leave the dimension (employ another one) to even turn around. This is Fig 0. Move up to two dimensions _ I have modelled this as a plane in two dimensions in Fig 1 You can have a rotation about any point in the plane. Draw this as a circle on one face of your box, as in Fig 2. But any rotation is about an axis which has to be a line in a third dimension. So if you extend a line through your point through the opposite side you have the z axis. You can draw a circle round it though any plane parallel to the first side like the opposite side. Now move up to 3 dimensions. You have to pairs of sides you can draw rotation circles on to generate two more axes, making 3 in all. As in Figs 3 and 4. I have shown the conventional right handed rectangular xyz coordinate system. Now comes the clever part - your experiment. Use the box to convince yourself that rotation on any plane at any angle has an axis within the 3D system. You do not need to leave 3D and have a rotation axis pointing into a fourth or higher dimension. This is what I mean when I say that 3D is complete for rotations. Let us know how you get on with your box.

I'm sorry to have to tell you that your video is a sorry mixture of fact and fiction that reaches some startling unsupportable conclusions. You will only confuse yourself at best and start to 'believe' junk Physics if you try to study from stuff like this. You question is, however a valid one that is worth a considered answer. The third spatial dimension is necessary in our physical world to achieve closure for a set of rotations. This is also why a fourth spatial dimension is not needed and we do not observe one.

I have to say that I am not a great fan of (formal) 'Logic'. I find it too narrow a concept. I much prefer the phrase, "rational deduction" which has a much wider scope. We had a thread a while back (not mine) debating whether 'Logic' is a subset of maths or Maths is a subset of 'Logic'. Of course, they have much in common but there are differences. A more interesting comparison, in my view, is between Maths and English and Rational Deduction.