studiot

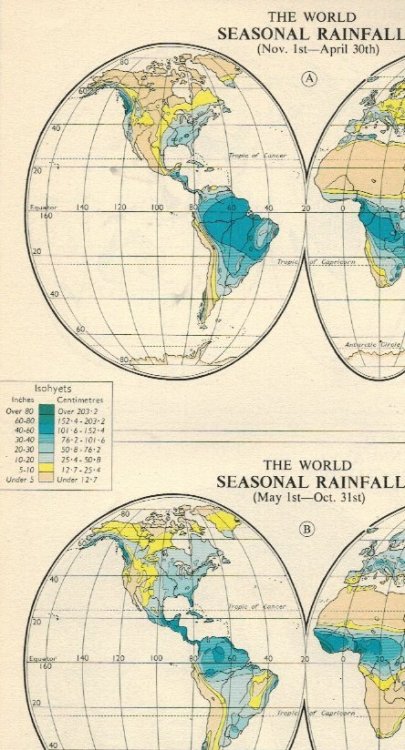
Thank you for that reply and information. +1 I see that if any, the prevailing winds are from the North in the vicinity of 40o N and there is a slight winter maximum to average rainfall. Since water dropped on your mountains cannot be carried and dropped elsewhere, can you say if there has been any rain shadow effect say to the South or in Utah ?

I'l just answer this one for now since it is an example of Caratheorory's formulation of the Second Law. You have correctly anticipated part of my answer. I do think our discussion is beginning to get somewhere now. +1 Perhaps you have come across this? Anyway here is an analysis of the system Suppose that when the piston is at the position shown, the system is in equilibrium. So the left hand chamber equilibrium volume is VA and the right hand volume VB. For the subsytems (partitions in your parlance) nearby states have volumes (VA + dVA) and (VB + dVB). So their entropy changes are [math]d{S_A} = \frac{{d{E_A}}}{{{T_A}}} + \frac{{{P_A}}}{{{T_A}}}d{V_A}[/math] and [math]d{S_B} = \frac{{d{E_B}}}{{{T_B}}} + \frac{{{P_B}}}{{{T_B}}}d{V_B}[/math] But [math]d{E_A} = - Pd{V_A}[/math] and [math]d{E_B} = - Pd{V_B}[/math] so [math]d{S_A} = d{S_B} = 0[/math] Therefore [math]dS\left( {system} \right) = d{S_A} + d{S_B} = 0[/math] So the entropy is the same in all positions of the piston. Thank you for the link I have downloaded the paper for later reading. I am glad you mentioned Turing because his machine is the crux of the difference between information entropy and the entropy of physical systems. So I will come back to this.

I don't understand. I quoted directly from your post before your last one. But my comment was a bit cheeky. I simply meant that thermodynamics was developed to enable us to predict (and therefore use) the time evolution of systems, including boxes of gas. It was not a criticism. Salt and pepper are part of the ncessary scientific apparatus for this. Take a sheet of paper and shake out some ground pepper over it. Mark where each grain falls on the paper. Dust off the pepper and take a ruler. You will find that a random ruler line matches the position marks of some of the pepper dots. Leylines are not magic. (I believe you recently referred to someone's experiment with random chords)

I really don't understand what you are getting at here. I am not saying the thermo partition is the only one. Quite the reverse. That is the whole point about my flats and pigeonholes analogy or chessboard squares, that you have yet to understand. Perhaps this statement of yours will help since I am matching the flats/pigeonholes or squares as a definition of particular classes (not of equivalence classes in general but different ones) There is a one to one correspondence between the state structure in thermodynamics systems following Boltzman's distribution and some information systems. Of course there is, the layout of available 'boxes' follows the same law for both. But the use of this is different and there are other laws which also apply to one or the other individually, which are different. Can you point to an emergent phenomen in information theory? I can offer you one from the physical world, that as far as I know, has no counterpart in informatuon theory. You have answered my question rather briefly Can you point to a QM law applied to information theory to produce QM effects in information behaviour? I am listening out for your detailed explanation of the anomalous ionisation energies and its alleged counterpart in informatuion theory. I am sorry if I misunderstood you but that was the impression I gained reading your previous posts. If I did please consider that other may do as well. However if you now confirm your view that two structures may have some similarities or identities but also some differences. I can happily accept that. My point then becomes, you cannot (necessarily) separate off the differences and declare them identical. Though you may, of course, take advantage of the similarities in using one to model the other. Hopefully the above now puts my view on this into context. Just as you have said that you didn't say there is only one law, I didn't say that all the laws of thermodynamics are different, I claimed that some are the same and some are different. Can you now offer me the same courtesy? In relation to this, have you hear of the division into "The Relations of Constitution" and the "The Conditions of Compatibility" ? If not it might pay you to study them a little. They are an important way of analysing things. @Tristan L and @joigus Surely that is the point of Thermodynamics - To model what a box of gas is going to do ? However I have not been following the runes example very closely, but perhaps I can offer my 'salt and pepper' set explanation of Leylines here ?

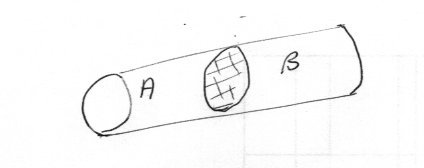
Here is another simple problem Suppose you have a sealed adiabatic tube containing an adiabatic frictionless piston dividing the tube into two chambers, A and B as shown. Let both sides of the system contain an ideal gas. Discuss the time evolution of the system in terms of entropy.

So what melts and what boils? So what is the liquid and gas comprised of? I already asked that question about the liquid and you have suggested it must be ions, in which case it is decomposed. You can't use the argument for one thing and not the other. You can you use your professional reputatuion to guarantee that no chlorine or sodium vapour is released. Did you read the RSC archive article? How does chlorine possess a vapour pressure if none is released? How does sodium possess a vapour pressure if none is released?

Congratulations, you have found a far better diagram. +1 And yes we usually ignore the effect on the Earth and just consider the effect on the body, be it book, table or ball.

Who said it does ? I see that someone who has not the guts to say why does not like my genuine attempt to help. I do apologise to Mica that it was not the best written of links. I did wonder why they talked about electrolysis, but what did they say that was factually incorrect ? In any event here is a better link. https://courses.lumenlearning.com/boundless-chemistry/chapter/electrolysis/ They clearly describe the industrial process of decomposing molten sodium chloride into metallic sodium and chlorine by electrolysis. The melting is achieved by heating, not electrolysis. OK so you are not going to electrolyse it so the question remains Does sodium chloride ionise when it melts ? Well most alkali halides apparantly do. If molten sodium chloride does not ionise (ie decompose on melting) how does it conduct electricity and what species are present in the melt? The was a study published in 1931 by Guthrie and Nance https://pubs.rsc.org/-/content/articlelanding/1931/tf/tf9312700228/unauth#!divAbstract It contains a discussion of differences of opinion from various authorities about how much chlorine is released. They mostly used a platinum crucible. Wikipedia shows pictures of melting halides on a charcoal block and blowpipe, a common past chemistry technique.

And I'm sorry to point out that you seem to me to be bent on finding fault with my attempts to explain my principle point to you, rather than understanding the point itself. As I understand your thesis here, you are proposing that there is one and only one Law or rule that applies to your partitions, that due to Boltzman. However it remains your responsibility to support your thesis so please explain the anomalous first ionisation energies of Nitrogen, Phosphorus and Arsenic in terms of your proposition.

This is the unaswered question. +1 Consider a system of molecules in some volume. Unless both molecules have the same velocity they do not have the same kinetic energy and therefore the internal energy is not evenly distributed between the molecules (maximum entropy). So the famous 'hot death' of the universe must be a static (as in unchanging) situation from the point of view of maximum entropy. But this view ignores the fact there are twin drivers in the thermodynamic world that often pull in opposite directions. The principle of minimum energy can be used to devise a system that will oscillate indefinitely at fixed (maximum) entropy.

Sensei said it better than the website I linked to. Sodium Chloride MP 801oC Sodium MP 98oC BP 883oC Chlorine BP -34oC So if you just 'heat it in a crucible' to 800oC + you will drive off the chlorine as a rapidly expanding highly corrosive gas. The sodium will be just liquid but close to its boiling point so will also be exterting substantial vapour pressure. So you will need more than just a crucible in a furnace. My comment was about safety.

Crucible material is not your only problem

The book Non- Euclidian Geometry by Manning was originally published in 1901. Here is the passage. I did actually try adding non euclidian and geometry, and boundary curves but can't remember if I just included mathematics. Also tried Wolfram but no luck there. Thankfully the theorory of horcycles appears in my Dan Pedoe's Geometry, a Comprehensive Course. Thanks again to you both.

Thank you for considering my comments. I'm sorry my analogy was not clear enough for you to understand. So try this one instead. Both chess and draughts are played on the same board. But they are very different games with very different rules, and different end results. Events can happen in chess that cannot happen in draughts and vice versa. The same can be said of the partitions of your master set. This carries over to the other part of your answer. There are umpteen relationships in physics where something is proportional to something else. And very often the contant of proportionality carries the units as in strain (a dimensionless quantity) is proportional to stress. But that does not mean we can disregard the constant and say therefore stress and strain are the same as thermodynamic entropies. Otherwise you could model one on the other, but if you tried you would obtain conflicting results, just as if you tried to play chess with a draughts set or vice versa. Information entropy and Thermodynamic entropy are not the same, or subject to the same laws (as in the rules of chess and draughts).

Many thanks +1 I will see if this fits the article I have.

Does anyone have any referencs to Oricycles ? I can't seem to find any. This is a (non Euclidian) geometrical question.

Didn't you miss something out here? Thermodynamic entropy has to have units of energy per degree of temperature. Other entropies, such as your runinations (pun intended) will have different units. In a block of flats there is (or should be) a one-to-one between the pigeonhole letter boxes at the entrance and the flats and their organisational structure. But would you rather live in a pigeonhole or flat? They are not the same.

That was part 1 - it was taking so long I though I'd split it. Part 2 is a bit less mathematical, but I'm gald to see you can cope with partial diffs.

Yes you are right thank you, though I thought I had spotted it and fixed it once. I hate LaTex.

Like it +1 @Nedcim I hope this helpsyou.

Yes and how many objects is 'objects' ? There must be more than one. But how many objects are there in a free body diagram ? Just one. Understanding this is the key to it all, as Ghideon so nicely told you three pages ago. and could you repair this English please / I do not understand the underlined bit. At the end I can see that it says that if A exerts a force on B then B exerts a force on A and I agree with that (as does everybody else).

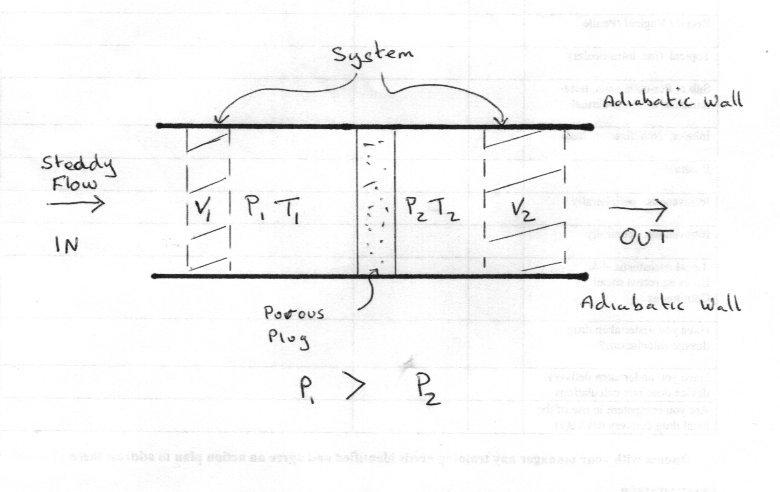
Part 1 The analysis if the Joule-Thompson or Joule-Kelvin flow or throttling is interesting because it demonstrates so many points in a successful thermodynamic analysis. Appropriate system description Distinction from similar systems Identification of appropriate variables Correct application of states Distinction between the fundamental laws and the equations of state and their application JT flow is a continuous steady state process. The system is not isolated or necessarily closed, but may be treated as quasi-closed but suitable choice of variables. It cannot be considered as closed, for instance, if we consider a 'control volume' approach, common in flow processes, since one of our chosen variables (volume) varies. By contrast, the Joule effect is a one off or one shot expansion of an isolated system. So to start the analysis here is a diagram. 1 mass unit eg 1 mole of gas within the flow enters the left chamber between adiabatic walls and equilibrates to the V1, P1, T1 and E1. Since P1 > P2 the flow takes this 1 mole through the porous plug into the right hand chamber where it equilibrates to V2, P2, T2 and E2. The 'system' is just this 1 mole of gs, not the whole flow. The system thus passes from state1 to state 2. The First Law can thus be applied to the change. Since the process is adiabatic, q = 0 and the work done at each state is PV work. E2 - E1 = P1V1 - P2V2 since the system expands and does negative work. Rearranging gives E2 + P2V2 = E1 + P1V1 But E + PV = H or enthalpy. So the process is one of constant enthalpy or ΔH = 0. Note this is unlike ΔE which is not zero. Since ΔE is not zero, P1V1 is not equal to P2V2 More of this later. Since H is a state variable and ΔH = 0 [math]dH = 0 = {\left( {\frac{{\partial H}}{{\partial T}}} \right)_P}dT + {\left( {\frac{{\partial H}}{{\partial P}}} \right)_T}dP[/math] [math]{\left( {\frac{{\partial H}}{{\partial T}}} \right)_P}dT = - {\left( {\frac{{\partial H}}{{\partial P}}} \right)_T}dP[/math] [math]{\left( {\frac{{\partial T}}{{\partial P}}} \right)_H} = - \frac{{{{\left( {\frac{{\partial H}}{{\partial P}}} \right)}_T}}}{{{{\left( {\frac{{\partial H}}{{\partial T}}} \right)}_P}}}[/math] Where [math]{\left( {\frac{{\partial T}}{{\partial P}}} \right)_H}[/math] is defined as the Joule-Thompson coefficient, μ, However we actually want our equation to contain measurable quantities to be useful so using [math]H = E + PV[/math] again [math]dH = PdV + VdP + dE[/math] [math]0 = TdS - PdV - dE[/math] add previous 2 equations [math]dH = Tds + VdP[/math] divide by dP at constant temperature [math]{\left( {\frac{{\partial H}}{{\partial P}}} \right)_T} = T{\left( {\frac{{\partial S}}{{\partial P}}} \right)_T}dP + V[/math] But [math]{\left( {\frac{{\partial S}}{{\partial P}}} \right)_T} = - {\left( {\frac{{\partial V}}{{\partial T}}} \right)_P}[/math] so [math]V = T{\left( {\frac{{\partial V}}{{\partial T}}} \right)_P} + {\left( {\frac{{\partial H}}{{\partial P}}} \right)_T}[/math] combining this with our fraction for μ we have [math]\mu = {\left( {\frac{{\partial T}}{{\partial P}}} \right)_H} = \frac{{T{{\left( {\frac{{\partial V}}{{\partial T}}} \right)}_P} - V}}{{{C_P}}}[/math] Which give a practical form with quantities that can be measured. [math]\Delta T = \frac{{T{{\left( {\frac{{\partial V}}{{\partial T}}} \right)}_P} - V}}{{{C_P}}}\Delta P[/math] Joule and Thompson found that the change in temperature is proportional to the change in pressure for a range of temperature restircted to the vicinity of T. The next stage is to introduce the second law and the connection between different equations of state and their meanings or implications. Edit I think I've ironed out all the latex now but please report errors to the author.

Yes I agree, Turing took one of the many steps along the development of IT, he did not invent the Von Neuman architecture (I wonder who did that ?) Although originally a theoretical mathematician, Turing was also practical as evidenced by rewriting the intensively theoretical Godel theorems into a practical (if gedanken) setting). But modern IT is about more than just about one thing. It draws together many disparate aspects of technical knowhow. But it is difficult to list the many who contributed to the drawing together of the many different threads without missing someone out, or how far back to go in engineering history. For instance what would the internet be like without the modern display screen ? Should we include the development of these or printers or printing itelf? Fax machine were first invented in 1843. What about control programs? Turing is credited with the introduction of 'the algorithm'. But Hollerith invented the punch card in 1884. You need The laws of combination logic (Boole, De Morgan) The implementation of these in machines (Babbage, Hollerith, Felt) Methods of communication between machines (Bell , Bain, Hertz, Marconi) The formation of 'words' of data from individual combinations. Standardisation of these words - the language (Bemer) Moving from mechanical to electromechanical to electronic implementations of data structures (Von Neuman) So here is my (draft) shortlist of the development, apologies for any omissions. Babbage (1791 - 1871) the analytical engine De Morgan (1806 - 1871) De Morgan's theorem. Boole (1815 - 1871) Boolean algebra Bain (1810 - 1877) the Fax machine Bell (1847 - 1922) The telegraph telephone Hollerith (1860 - 1921) punch cards Braun Berliner (1851 - 1929) microphone (inter machine communications) - 1876 Braun (1850 - 1918) cathode ray tube (inter machine communications) - 1897 Felt (1862 - 1930) Comptometer 1887 Von Neuman (1903 - 1957) Digital computer architecture. Bemer (1920 - 2004) Standardisation of digital words. 1961 Interestingly names beginning with the letter B predominate, perhaps that was Turing's crime - to start his name with the wrong letter.

Pematurely is the wrong word. There is no shortage of (sustainable) energy, just a vast shortage of the political will to use it. Like some many socially worthwhile changes (e g water supply) it is just a question of engineering.

That's only because the cops keep asking if you are old enough to smoke.