studiot

I know you haven't followed up any of the references I have already given but you might like to look at this one. Chapter 2 of Molecular Quantum Mechanics by Atkins and Friedman is largely about your current discussion with swansont. In particular they work out in great detail the difference between the classical harmonic oscillator and a quantum (probability) one with many plots and diagrams similar to the ones you are looking at. It would save you a lot of work and offers a great deal of explanation about what is and is not possible.

This thread isn't really a speculation, more of a personal statement so here are a couple of thoughts I have picked along the way plus a suggestion or two. I have read somewhere that the last man alive capable of knowing and understanding 'the big picture' was Archimedes. After him (he wasn't history's greatest genius) no man or woman had the capacity to look in every corner of Science, it had become just too vast. Of the many geniuses that follwed him I would place Newton at the top simply because he had not only to invent the Science he also has to invent the necessary Mathematics to go with it. Einstein at least has the luxury of better mathematicians to help him. OK, gloom and doom over here are a couple of suggestions. You do not need journalistic 'popsci' books, there are not many good ones and an engineering degree is already beyond them. You could try to follow Peter Collier, author of "A Most incomprehensible thing" ; Peter, like yourself, was a man who wnated to know more (about Relativity and Cosmology) so he set himself the task of learning the big picture as it applied there. It is a good read. He has to teach himself the maths of things like tensors to do this. But that is still a small corner of Science. A book your background should enable you to access is Molecular Quantum Mechanics by Professors Atkins and Friedman. The interesting thing is that they are Professors of Chemistry. Yet they develop relativistic QM, Group theory, Symmetry theory, explaining the necessary maths as they go along. It is the nearest thing to big picture that I know.

As a matter of interest, Professors Mott and Sneddon got there before you. Swansont asked you to use your equations to derive the energy levels of the hydrogen atom. Here is Dirac's solution. In particular see the notation introduced in (99) in the middle extract.

Gsoh, Mordred, you've outdone yourself there - must be a +1 Interestingly the only place I have seen Fields (as opposed to simpler things) tested against the postulates of Relativity is in Wangsness ("Topics in theoretical Physics" )

Here is a question just posted on another scientific forum, that you might like to consider with your mathematics since you have introduced eigenfunctions. Whilst we are discussing eigenstates/functions/values are you taking your start from Dirac's Bra and Ket ? That is where they come into their own in QM.

Well I've read it and I couldn't find the definitions which is why I keep guessing and you have to keep correcting my guesses. That is not a good way to connect with your audience. It is absolutely basic best practice that you define your symbols at the outset. That way you do not need to do it again. Of course we are criticising it (though we are still at the trying to understand it stage). That is the scientific process. We are looking for flaws and since the conjecture is not trivial we are trying very hard. If you genuinely have come up with a better formula I will be the first to congratulate you.

+1 I asked several times now for a list of definitions of your symbols. Is your gamma related to the normal mathematical use as the gamma function? Exactly, that is why I keep banging on about boundary conditions.

OK we have seen this before. Wasn't the poster eventually banned or something.

Finally some 'mathematics' Thank you. Did you understand the wave equation in my last post, you made no reference to it? The point is that it is a partial differential equation. I found nothing similar nor reference to such in your pdf. Are you aware of the difference ebtween partial differential equations and ordinary differential equations? In ordinary differential equations, adding a arbitrary constant to a solution creates a new solution, merely shifting the original solution on the axis. In a partial differential equation, you have to include an arbitrary function (2 for the second order one I displayed). This changes the shape of the solution plot. So there are at least as many solutions or shapes as there are arbitrary functions. So there are many solutions to both the classical wave equation and Schrodinger, Dirac et al. The point is that it is the boundary conditions that allow us to pick out a specific solution, which is why I asked about the boundary conditions early on. Boundary conditions are at least as important as the (differential) equation itself.

@John Henke You have answers from at least three widely separated continents from members who are capable of understanding higher mathematics. But I'm sorry to say that having watched the video I am no wiser since it is rambling and unstructured. It contains far too many "I don't knows" . Instead of proper explanations or shots of the maths it contains lots of waving over graphs of plain sinusoids and sinusoidal decaying curves. I did not see a single plot I would recognise from solutions of conventional quantum wavefunction theory. Your plots would only satisfy the non quantum wave equation. [math]\frac{{{\partial ^2}\Gamma }}{{\partial {x^2}}} = \frac{1}{{{v^2}}}\frac{{{\partial ^2}\Gamma }}{{\partial {t^2}}}[/math] if gamma is meant to be your 'wavefunction' what is the 'wave equation' it is meant to be a solution of? Also since you have used many conventional symbols perhaps for different purposes than convention, please list the attribution of your symbols.

Thank you for a good reply. +1 1) I hope not as I will have time to review it properly later on tonight. 2) This site uses MathML insert code as follows [math].......ML code....[/math] It used to use LaTex but that can now be unreliable. free sites for generating code (copy/paste) are codecogs (apparantly not working at the moment) so http://www.moreofit.com/similar-to/latex.codecogs.com/Top_10_Sites_Like_Codecogs_Latex/ sciweavers http://www.sciweavers.org/free-online-latex-equation-editor 3) thanks 4) noted 5) Note you have 5 posts available in the first 24 hours so no reply expected use your next 3 wisely.

Hello, John I see you are new here, have you read the rules ? In particular before the mods remove your half hour video can you pinpoint on its timeline where we might view the actual equation and the list of boundary conditions you are applying? Better still, simply post them here for discussion.

What is the ultimate beginning, middle, and inevitable destination of entities/objects/systems while in any equilibrium state as you said, also while in any type of relationship? Is there such an absolute and singular of entity/object/system that is absolutely independent of any type of relationship? Thank you for your reply. Sadly I didn't understand a word of it. Please explain to an idiot like me in simple baby steps how what you have said in any way answers my question ?

Take a pure liquid substance in a sealed container. What is the equilibrium state of the contents above the critical temperature? However I would admit that I was thinking about a ball perched on a hill top, rolling down to a bench partway down and at the valley bottom when I originally wrote that bit.

Science recognised many more types of equilibrium than the limited view you offer here. So Science distinguishes for instance stable, metastable and unstable equilibrium in one classification; static and dynamic equilibrium in another, further your system is stated to require two or more entities. What about the equilibrium of a single entity or system ? How does your assertion fit in with these ?

Since this is coursework, here are some hints. But since it is important to understand what you are doing, please ask if there is any part you do not understand. First it is important to understand the definition of solubility product. So ask if you do not. Solubility products are different from other constants in that they are not a ratio of concentrations. When a slightly soluble substance goes into solution, leaving some or most of its substance in physical contact with the solution the undissolved doesn not change in quantity. The solution is saturated according to the normal reaction equation for solution [math]Fe{\left( {OH} \right)_3} \Leftrightarrow F{e^{3 + }} + 3{\left( {OH} \right)^ - }[/math] With an equilibrium constant Keq given by (in your case) [math]{K_{eq}} = \frac{{\left[ {F{e^{3 + }}} \right]{{\left[ {O{H^ - }} \right]}^3}}}{{\left[ {Fe{{\left( {OH} \right)}_3}} \right]}}[/math] The bottom part is the amount of the substance that has gone into solution. The concentration of this is therefore the concentration of the ferric hydroxide that is in solution that you are given as 4 x 10-10M I have circled this value in pink. So to move on we multiply both sides of this equation by the concentration of the ferric hydroxide to get the solubility product, Ksp. [math]{K_{sp}} = \left[ {Fe{{\left( {OH} \right)}_3}} \right]{K_{eq}} = \left[ {F{e^{3 + }}} \right]{\left[ {O{H^ - }} \right]^3}[/math] Substituting values and forming the other equations of equilibrium should enable you to complete this question. Come back and let us know how you get on.

finding acceptable double arrows for chemical equations [math]Fe{\left( {OH} \right)_3} \Leftrightarrow F{e^{3 + }} + 3{\left( {OH} \right)^ - }[/math] [math]Fe{\left( {OH} \right)_3} \leftrightarrow F{e^{3 + }} + 3{\left( {OH} \right)^ - }[/math] [math]Fe{\left( {OH} \right)_3} \mathbin{\lower.3ex\hbox{$\buildrel\textstyle\rightarrow\over {\smash{\leftarrow}\vphantom{_{\vbox to.5ex{\vss}}}}$}} F{e^{3 + }} + 3{\left( {OH} \right)^ - }[/math]

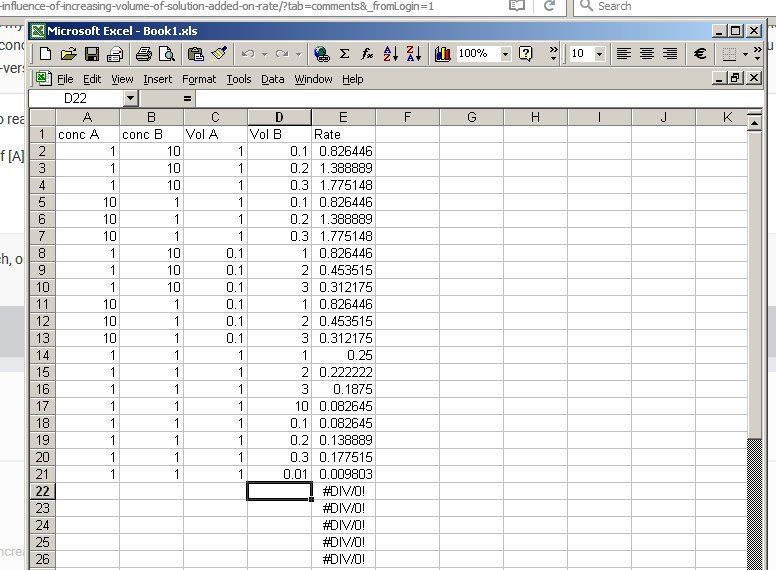
I had though you might like to reason the condition out for yourself. It depends both on the rato of [A] to and Vn to V1

So the solution is not packing D Trump off in a spacecraft. +1

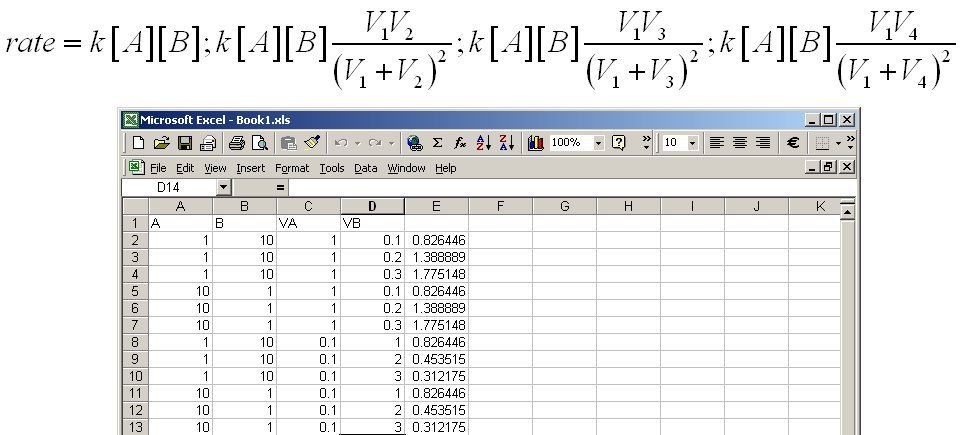
I see. So the answer now is it depends on the values. So take the rate R = k[A] and compare rate when you add V2 < V3 < V4 volumes of B to 3 samples of V1 volume of A. Then the total volumes are now V1 < (V1 + V2) < (V1 + V3) < (V1 + V4) Which clearly dilutes [A] since you have added no more Ab ( that is V2, V3 and V4 contain no A) So the initial concentrations for A to put into the rate equation are [math]\left[ A \right] > \left[ A \right]\frac{{{V_1}}}{{\left( {{V_1} + {V_2}} \right)}} > \left[ A \right]\frac{{{V_1}}}{{\left( {{V_1} + {V_3}} \right)}} > \left[ A \right]\frac{{{V_1}}}{{\left( {{V_1} + {V_4}} \right)}}[/math] For B the situation works the other way round since each adds a large quantity of B to the total So the initial quantities of B to put into the rate equation are [math]\left[ B \right] > \left[ B \right]\frac{{{V_2}}}{{\left( {{V_1} + {V_2}} \right)}} > \left[ B \right]\frac{{{V_3}}}{{\left( {{V_1} + {V_3}} \right)}} > \left[ B \right]\frac{{{V_4}}}{{\left( {{V_1} + {V_4}} \right)}}[/math] So the rate varies depending and I will leave you to think about where it goes up or down. But below is a spreadsheet tracking variations in [A], and the volumes of A and B. [math]rate = k\left[ A \right]\left[ B \right];k\left[ A \right]\left[ B \right]\frac{{{V_1}{V_2}}}{{{{\left( {{V_1} + {V_2}} \right)}^2}}};k\left[ A \right]\left[ B \right]\frac{{{V_1}{V_3}}}{{{{\left( {{V_1} + {V_3}} \right)}^2}}};k\left[ A \right]\left[ B \right]\frac{{{V_1}{V_4}}}{{{{\left( {{V_1} + {V_4}} \right)}^2}}}[/math]

the answer is still no. If the concentration is fixed (ie constant) and the rate is proportional to the concentration how can the rate vary IOW [math]\frac{d}{{dt}}\left( {\frac{{d\left[ A \right]}}{{dt}}} \right) = 0[/math]

I logged on just to say +1 for this.

Yes, but it is also true that many ( actually an infinity) other classifications are possible. So how is yours useful? By the way 'group' is another very well defined term in Mathematics, how and why is your definition different? I too am also waiting to learn how one set can 'oppose' another. I do wonder if you are referring to the conventional 'complemetary set' since you are partitioning your universal set into two parts.

It is extremely bad form and very unhelpful to readers to answer quotes in this fashion. ie by placing your reply within the quote attributed to another member makes the reply from that member appear different from what it really is, both in content and date/time. (I have changed your word colour to red to show this) Above the second quote is the first attempt to quote your words, using the forum quote function. It is completely blank. Please separate your words from those of other members. This example quote from Strange shows how to do it properly. Your insistence on "inferior divisors" appears to be redundant.

Yeah good advice, we don't know how much is lost or bungled in translation. +1