studiot

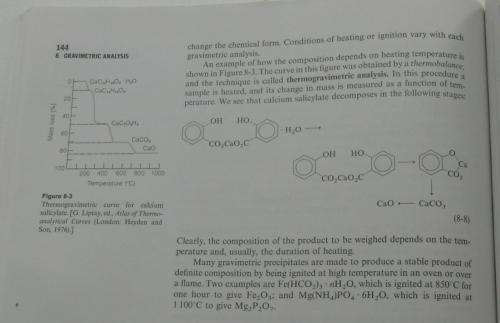
I know next to nothing about calcium acrylate chemistry, but there is clearly significant knowledge about. https://www.google.co.uk/search?q=calcium+acyrylate&rlz=1C1AVNG_enGB673GB673&oq=calcium+acyrylate&aqs=chrome..69i57.9069j0j8&sourceid=chrome&ie=UTF-8 I would hazard a guess that some intermediate reactions as with this TG analysis of calcium salicylate

You are testing a carbonate/polyacrylate mixture are you not I don't know what the decomposition temperature of the polyacrylate is but for silicone engine sealant it is around 300o C and you are testing much higher than this?

Where there's a will there's a whirl.

I find the quote system cantankerous at best but one tip I have discovered Write some text, any text you can always delete it later, before you invoke the quote function. You can then come back, at any time, and paste into the quotes at will.

To borrow a phrase "Be careful what you measure, It may come true!" When I was growing up there was a black and white TV programme called "Stranger than fiction" Later I worked in the Saudi desert where survey parties observed (measured) negative vertical angles at both ends of some observation lines. Reality was of course, somewhat different.

Rather than following a flippant treatment such as presented in your post#15 quote, I suggest you look up the following An angle modulated radio/light wave may not be sinusoidal, or expressible as a linear combination of them. What is the waveshape of light received from a body which is continually accelerating away? Solitons (solitary waves) as applied to (modern) optics. The difference between dispersion and dissipation as applied to waves. Gibbs phenomena in periodic functions.

Thank you for the positive and frank discussion so far. I have tried to encourage others (who have read this judging by the viewing numbers) to participate, not least the OP, moreno. I'm sorry you have limited your interest since I was preparing the next stage of my discussion, viz the transition from the 'little balls theory' to quantum theories. I will again post a few summary observations in case there is further interest. When we consider the bonding in alkenes we discus the pi bond and these carry over to polymer dienes. These pi bonds, which are formed as hybrids from the bonding atomic obitals have a definite shape and locality in space. This describes where we can expect to find the electron and where we can't. When we consider the same process in metallic bonds the bands that result are not like this and the band structure is not equivalent to a series of pipes through the lattice. In particular they exist in phase space, not xyz physical space. So the 'curvature' you describe exists on graph paper, not in real space, unlike the curvature of p orbitals and pi bonds. An electron in a band does not move about like a little ball but once it enters a particular band it can be anywhere within the range of that band. The electron acts a wave that spans the entire band but can only appear in one particular phase space sub orbital according to Pauli rules. I was preparing a comparative table of orbitals here are a few. We can discuss the implications if you wish. Note that Lithium Sodium, Potassium, Rubidium, Caesium, Copper, Sliver and Gold have a full shell plus an outer S orbital with a singleton electron. They all have 'normal' Hall coefficients. Beryllium, Cadmium, Tungsten and Zinc have 'anomalous' coefficients and have that outer S orbital fully occupied. Borderline cases, magnesium, indium and aluminium have the full S orbital, but also have a singleton outer p electron.

D is wrong because it looks at independent causative variables. The third variable is due to a variable common to both the measured ones.

Yes a selectional bias can create a false correlation, because the sample population may be drawn from only from those with the tested characteristic, by the selection process. Since this is homework help it is good to do more than just ask us to check you answer to boost marks. (you didn't do that did you?)

A few calculation and references to support your statements would be nice. Looking at my diagram here is something to ponder. We are discussing conductors and semiconductors, that is conductivity current as opposed to displacement current, not the special states that exist in superconductors. All conductors have some resistance (conductivity) and require an electric field to drive the current (even if small). Any explanation that states 'It's due to negative mass guv, honest' must also explain why the main current proceeds in the normal expected direction and only the hall current/deflection is reversed. Edit If you are going to offer the negative effective mass explanation, your explanation is insufficient, particularly if you wish to reject the deifinition I gave which is entirely conventional. Your statement is basically that of Dr Steve B here http://physics.stackexchange.com/questions/10800/how-can-the-hall-effect-ever-show-positive-charge-carriers Whilst I have great respect for Steve, his explanation was as lacking as yours as to why there should be 'curvature of the bands' or what that might mean or signify. There is something more fundamental going on that I was leading up to.

I haven't used any diagrams yet so here is one that I think is clear.

Most of what you have said in post#11 I agree with but this bit needs some thought. Not exactly. If hole movement was the same as electron movement then current could be described in terms of electrons only. But the holes and electrons are often in different bands. Anyway that does not explain negative effective mass. This is because mass is a non-negative isotropic scalar. Effective mass is much more complicated and is in fact a tensor. Effective mass in two or more dimensions can be positive or negative and vary in value. I don't know if either moreno or anyone else is still interested but I am going to start at a very basic level and expand some things normally just stated as granted in textbooks. So here is a gentle introduction to the implications of this. First let us consider speed and velocity as a simple example to set the scene. Speed is a non-negative isotropic scalar. A body travelling at a speed of V travels at V, whatever direction it is heading. Velocity is a vector. Velocity in two or more dimensions has positive or negative components that vary in value, depending upon the direction. So a velocity V due East has a component Vcos (45) in the North Easterly direction and -Vcos(45) in the South Westerly direction. And yet both speed and velocity have the same units or dimensions - metres per second or LT-1 Sometimes speed and velocity can be used interchangeably, sometimes they can't, as in the case of the calculation of momentum, which is always speed times mass, but you need to take all the velocity components into account if you calculate mass times velocity. Speed and velocity are generally well known and understood. Mass, reduced mass and effective mass less so. Especially as some authors in quantum theory call 'reduced mass' a form of effective mass and there are yet other definitions in other circumstances. So what definition of effective mass should we use in the Hall effect and what are the consequences? Well I will just outline the definition in this post and fill in derivation and detail later if anyone is interested. We define effective mass as the ratio of the square of Plank's constant to the second derivative of the particle's energy with respect to its De Broglie wave vector. [math]{\rm{EffectiveMass}}\quad {\rm{ = }}\quad {{\rm{m}}^*} = \quad \frac{{{{\left( \hbar \right)}^2}}}{{\left( {\frac{{{\partial ^2}E}}{{\partial {k^2}}}} \right)}}[/math] To see why it is called effective mass look at the units/dimensions. Energy has units Joules or L2MT-2 [math]{\left( \hbar \right)}[/math] has units Joule-seconds or L2MT-1 the wave vector, k has units: per metre or L-1 So the second differential has units energy per metre per metre or L2MT-2LL or L4MT-2 Putting this together with the square of the reduced Plank's constant we have [math]{\rm{Dimensions}}\;{\rm{of}}\;{\rm{effective}}\;{\rm{mass}}\;{\rm{are}}\quad \frac{{\left( {{L^2}M{T^{ - 1}}} \right)\left( {{L^2}M{T^{ - 1}}} \right)}}{{{L^4}M{T^{ - 2}}}} = M[/math] This has the same dimensions as the scalar inertial mass. So why is this a tensor? Well look at the bottom of the fraction. In the wave vector we have the cartesian product of two vectors, ie a tensor. Just like the simple example with a vector velocity the tensor has different components in different direction. These components are conditioned by the environment, not the electron but that is the subject of another post.

I see that you were online after I posted yesterday so presumably you saw my reply. I note also that you are a biologist, although you have posted this in the Chemistry section so I if you have any questions arising, don't hesitate to ask. Some further notes. If the temperature is not constant then you also have to integrate the SdT term. This can be performed at constant pressure. My analysis was for gases and the pressures are therefore partial pressures if there is more than one. Pressures are a measure of (molar) concentration and the reaction quotient or coefficient, q, can also be described in these terms, which is good for non gaseous components. You have used Q for the quotient, which is becoming common, when K used to be used. There is a danger of confusing the q used in the First Law with Q which is (partly) why K was originally chosen.

Hello Nikolai and welcome. Some questions for you to think about. If a moving electron creates a wave, where does the energy for the wave come from? Does the electron loose energy as a result? Can this be measured? (Look up Bremsstralung https://en.wikipedia.org/wiki/Bremsstrahlung ) If the wave is 'extinguished', where does that energy go to?

This is an important question so I am going to work through it at some length. It is important to distinguish between the various Gs and G0 s and their deltas. G on its own refers to the Gibbs Free Energy function, which is a function of state. G0 is just the constant of integration, and is made specific to each reactant and reaction by choosing a stated condition. The integration is not of ΔG = ΔH - TΔS as you thought but of either Maxwell's relation [math]{\left( {\frac{{\partial G}}{{\partial P}}} \right)_T}[/math] Which brings out the fact that this is developed for gaseous reactants at constant temperature or of the equation [math]dG = VdP - SdT[/math] Since T is constant, SdT = 0 and we are left with the following [math]{G_T} = \int {Vdp = nRT\int {\frac{{dP}}{P}} } = nRT{\log _e}\left( P \right) + {\rm{constant}}[/math] The state function of temperature has a value at any T given by integrating the above Working per mole n = 1 and we set the constant equal to G0 Thus [math]{\rm{Constant}} = {G^0}[/math] and [math]G = RTLo{g_e}\left( P \right) + {G^0}[/math] This is the general thermodynamic equation. To apply it to a particular chemical reaction say [math]A + B \to C + D[/math] we calculate the change in G as ΔG by summing the values for the reactants products and subtracting the values for the products reactants Edit oops ! [math]\Delta G = {G_C} + {G_D} - {G_A} - {G_B}[/math] Each of these four components individually follows the general law so substituting this in [math]\Delta G = \left( {RTLo{g_e}\left( {{P_C}} \right) + G_C^0} \right) + \left( {RTLo{g_e}\left( {{P_D}} \right) + G_D^0} \right) - \left( {RTLo{g_e}\left( {{P_A}} \right) + G_A^0} \right) - \left( {RTLo{g_e}\left( {{P_B}} \right) + G_B^0} \right)[/math] Collecting terms and rearranging [math]\Delta G = \left( {G_C^0 + G_D^0 - G_A^0 - G_B^0} \right) + \left( {RTLo{g_e}\left( {{P_C}} \right) + RTLo{g_e}\left( {{P_D}} \right) - RTLo{g_e}\left( {{P_A}} \right) - RTLo{g_e}\left( {{P_B}} \right)} \right)[/math] This shows the various reactant and product contributions to [math]\Delta {G^0}[/math] in the first bracket Doing some algebra on the logs yields [math]\Delta G = \Delta {G^0} + \left( {RTLo{g_e}\left( {\frac{{{P_C}{P_D}}}{{{P_A}{P_B}}}} \right)} \right)[/math] Defining the reaction coefficient, Q as [math]Q = \left( {\frac{{{P_C}{P_D}}}{{{P_A}{P_B}}}} \right)[/math] and substitution yields [math]\Delta G = \Delta {G^0} + RTLo{g_e}\left( Q \right)[/math] Which is your equation for this particular reaction. Remember always that G on its own is general, but introducing G0 makes it specific to a particular reaction.

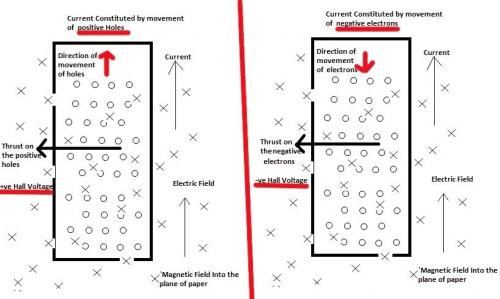
I'm having some difficulty with this statement. As I understand the Hall effect, positive and negative charge carriers are swept in the same direction by the Lorenz force. This results in either a positive or negative Hall voltage depending upon which carrier predominates. My calculations to support this are as follows Establish a right handed xyz coordinate system. Charge flows along the y axis with velocity V; electrons flow -y direction and holes flow in the positive y direction. and the applied magnetic field (B) is directed along the negative Z axis. This results in Lorenz force, F, directed along the negative x axis as follows The resultant Lorenz force on charge q is given by F = q(V x B) - a vector equation - and resolves as follows Fx = q(VyBz - VzBy) Fy = q(VzBx - VxBz) Fz = q(VxBy - VyBx) Applying values For holes q = +e ; Bz = -B ; By = Bx = 0 Vy = +V ; Vx = Vz = 0 Fx = +e{(+V)(-B) - 0) = -eVB Fy = +e(0 - 0) = 0 Fz = +e(0 - 0) = 0 For electrons q = -e ; Bz = -B ; By = Bx = 0 Vy = -V ; Vx = Vz = 0 Fx = -e{(-V)(-B) - 0) = -eVB Fy = -e(0 - 0) = 0 Fz = -e(0 - 0) = 0 It can be seen that once all the signs are taken into account both holes and electrons are driven in the same direction. I look forward to your calculations supporting the opposite view. Edit Of course this describes the standard Hall effect. It does not explain the anomalous situations moreno asked about. These cannot be satisfactorily explained by simple free electron theory.

As always you hold good conversation and make excellent points. Thank you. Yes indeed you could consider the clock defective whilst it is running slow. But my point is simply that put by Russell a century or so ago. We have already met processes that can produce the results listed in the OP and many others in ordinary everyday experience. So we should not be so surprised when Physics uncovers another. Russell further establishes the point that many of the apparently strange results of einstinian relativity could (and should) have been anticipated pre M&M. Indeed Newton knew some. The greatness of Einstein was in drawing all this together in so simple and compact a way.

Thank you for your reply, that is pretty much what I thought you would say. Now suppose the observer with the watch and standard rule slept whilst the changes occurred. When he awoke he would have an unsynchronised watch and clock and a displaced ruler that still matched his standard. How would he be able to tell whether the effect was due to relativity or heating and cooling? In other words, the result or product of the process is the same, at least for the clocks. IMHO there is too much attention paid to process and not enough to product these days. Note my other examples in post40 about chemical v mechanical degradation and points of view v relativity.

Disappointing. You are completely missing my point. I did not say that is was an example of relativity. In fact I definitely stated and repeated that it was not. So why contest? Chemical wear and degradation is not an example of mechanical wear , but the effect is very similar. This is the point I was making. This is following in the footsteps of Bertrand Russell's delightful book on relativity in which he is fond positing that we should not be so surprised/frightened about the effects of einstinian relativity as other, more familiar, mechanisms can have similar effects. Instead of attacking, why not try to help another understand? Michel, Following my theme to swansont here is another example of non relativistic observer dependence. An observer has a field of vision, say x by y units or xy square units. Let us suppose he wishes to place a fig leaf to cover the bottom half of his field. We both know that the required minimum size of the fig leaf depends upon its distance from the observer. This is because distant objects appear smaller to an observer. This example is a bit artificial, but computer programmers do exactly this when constructing onscreen CGI. PS I've got your six, you get it back when you respond.

Please explain An uncompensated clock runs more slowly in the presence of a gravitational field. (relativity) An uncompensated clock runs more slowly in the presence of a thermal 'field' (classic) Is there a difference?

Space and time are different. They behave differently classically, so why should you expect them to behave the same relativistically? I gave an example of time accumulation following dilation, but not space accumulation following dilatation by classical means in my post 19, but no one has thought to comment.

We should not call in either the old ghostbuster squad or the new one whenever we are faced with an einstinian relativity issue. We should call on common experience and realise that such changes also occur for other, well known, reasons. We should also not be surprised at the results when we take two variables that are not independent and plot an effect against them as though they were. Space and time are not independent. Consider the following. But the end of a ruler against a wall. Hang a pendulum clock on the wall above the ruler. Synchronise it with your watch. Now heat both clock and ruler (but not the watch) by some means, then allow to cool to original temperature. The ruler no longer abuts the wall and the clock and watch are no longer in synchronism.

I would say that Moreno has been chased off by your condescending attitude, when you could have offered explanation. I will take the first part of your last post as a reasonable question and try to expand the explanation I gave before. The mechanics of the 'free electron model' is Newtonian in that the response of a particle is proportional to an externally applied force. The constant of proportionality is called the mass and is the same for all sorts of applied forces in all sorts of situations. Electrons in a lattice are not actually free, but subject to constraints due to the lattice. As a result of these constraints the electron in a lattice does not respond in the same proportionality to an applied electric and or magnetic force. To a first approximation we can replace the free electron constant (mass) by a new constant and we call this the effective mass to distinguish. But it should be stressed this is a measured or observed value not a calculated or derived one. This is why I say the effective mass is a consequence, not a cause. To fully explain this phenomenon we need to delve into quantum band theory, but here is a simple explanation that might help. If we accept the idea of bands, without too much detail, we can observe that if a band is full the electrons cannot move in response to an applied force so no drift or driven current can occur. This is because an electron can only move into a vacancy. If there are none available it cannot move. This of course describes a perfect insulator. Conversely if the band is sparsely populated with electrons then these can move quite freely about making the material a good conductor, since there are many vacancies available. Now consider what happen is the band is almost full and short of exactly one electron. We still cannot have an electron migration current, but we can have a 'hole' migration current, which is, of course, of the opposite sign to the normal electron current. This is essentially the situation for the 'anomalies' asked about, but life is, as always, really much more complicated.

Negative effective mass is not the reason it's the consequence.

The history of plant sizes is outlined in The Emerald Planet, which I have referenced several times. A word about geological timing is in order here. The superlarge plants were a particular feature of the carboniferous period 300 - 350 MYA At this time athe first large animals appeared and a flourishing ecosystem developed. These animals were not dinosaurs. They were entirely wiped out at the end of the Permian in the PT mass extinction 250 MYA The dinosaurs developed subsequently in the lateter Jurassic and cretaceous and were themselves wiped out in the KT mass extinction 60 MYA