studiot

Hello, Saby and welcome. The first term in your expansion is $\frac{{{\mu _0}I}}{{4\pi r}}\oint\limits_{} {dl}$ Remember what dl is. It is the physical spatial displacement vector. (not the electric displacment vector D) When taken round a complete loop it must end up where it started. That is the total displacement must be zero. so $\oint\limits_{} {dl} = 0$ I didn't get why the last thing (the nabla opened thing) was zero... If you want to display math here go to this (free) site and type it in. It will generate either Latex or a gif for you to paste in. Edit forgot the links, sorry. http://www.sciweavers.org/free-online-latex-equation-editor or https://www.codecogs.com/latex/eqneditor.php

Yes I quite agree and I was just thinking of posting to invite cladking, who introduced the question, to say what he means by reality and exists. I was thinking about a test for existence and came up with the question Can something that does not exist interact with/affect reality? I thought I had it but then I realised that non existent things do indeed affect reality.

Look up mica, graphite & clay mineral structures. The layered structure affects physical and electrical properties.and produces anisotropies in these properties. Graphite is used as a lubricant. Find out if the bonding in graphite is ionic or covalent then look up its electrical properties Mica can be cut to ultrathin sheets Clay minerals have a hugely rich structure and hold pore water in soils.

Yes , you said that before and it is quite true, but I don't see how that is relevent? For instance how many natural numbers are there? Yet every one of them is finite. Consider further Enumeration is now considered one of the basic physical 'dimensions', along with mass, length, time, etc. Energy is not so considered.

Of course, but the original purpose of this model was substantially more exacting than the question we are discussing here. Surely it is enough to know that 1) An electron can be removed form a hydrogen atom 2) Whiilst bound within the atom the electron has a finite number of energy states available to it (neither the exact number, nor their exact values are relevent here) 3) Once the electron has left tthe atom, leaving a hydrogen ion behind, it is in a continuum and has surely therefore has an infinity of states availble to it. So is this not an example of physical realisation of infinity?

Thank you ajb, I see I did not explain myself very well since you have picked up far more complicated cases than I envisaged. I was not thinking of anything so complicated as the band structures in solid state physics, just a single atom or molecule, possibly the simplest ie hydrogen. In any event band structures still have a finite number of states available. I was just thinking about the morse curve http://www.chemicool.com/definition/morse_potential.html and the ionisation energy, when the electron breaks free of a simple molecule to form an ion and a free electron, which is in a continuum of energy states.

ajb, This really is the key point and one I seem to have to keep making (but not for your benefit). It does not matter if a theory gives us infinity for a 'value' of something we would like to meassure, which is usually the result of taking limits either in terms like 1/r or in terms of power series, this is interpreted as 'pushing the theory too far'. Singularies can appear in very simple models, like electrostatics or much more complicated theoreis in quantum field theory. They just show that the theories we have are not complete and that physics beyond these models is needed. ajb, I would really value your comment on the situation posed at the end of my post#56? ajb In truth it seems to correspond to the scientific standards of this forum and in particular the quality of the posts of the members. However, collecting lots of negative points quickly is usually a sign of poor attitude and civility. I tell you this because we know that collecting negative points is an indicator of a member quickly becoming banned for breaking the rules. I do not wish that to happen to you. Once again, +1 for displaying far greater tolerance and patience than I can.

Yes seems a reasonable if pessimistic summing up, with the exception that many think the underlined words are obvious. What's more because reality is more that our (best) models (or to put it another way reality is our best models and then a whole lot more) it surely must include infinity since the models do so.

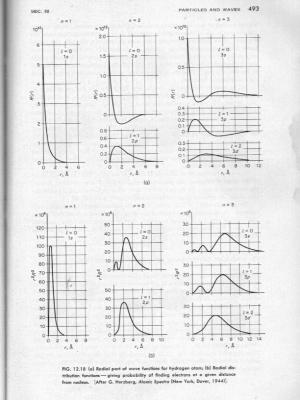
I'm sorry I put that rather badly, I was watching a 4 beer DVD at the time. The cumulative probability for the integral over all space is obviously 1 (The electron must be somewhere). r then runs from zero to infinity and these are the limits of a cumulative integration. The formula $4\pi {\left[ {R\left( r \right)} \right]^2}{r^2}dr$ can be broken down into a point probability P at r = r multiplied by an expanding volume dr. That is Pdr, whre P is $4\pi {\left[ {R\left( r \right)} \right]^2}{r^2}$ This is what is plotted on your graphs. The y value at some r give the point value of P at that r. So the hump corresponds to the max P and the Bohr radius for the 1s orbital.

One thing I didn't make clear is that the probability is not a point function, it is cumulative. It is not the probability at a point, but the probabilty that the electron will be found within a certain region of space. The region is defined by the limits of the integration over the volume. For spherical symmetry this is particularly easy since the limits are from r = 0 to r = r and define a ball or spherical shell anywhere within which the electron may be found. (Note the volume integral is the area of a spherical surface (4pir2) times a small radial distance dr) So as we move out from r = 0 the cumulative probability is the integral described for a sphere of radius r. The significance of the shape is that the probability starts off low and rises until you get to the hump. A bit before the hump it is significantly less so the value of r at the hump contributes the most to the cumulative probability. Your library should have a copy of Moore, it is one of the best textbooks at this level. Sleep well.

Here is some bedtime reading then, (from Physical Chemisry by Moore)

You haven't indicated the level of science or maths this question is for. Because the (electrostatic) potential energy of an electron is a function only of its distance from the nucleus; that is the force field is spherically symmetrical, it is possible to express the total wave fucntion as a product of three functions, each involving only one of the three coordinates in a spherical coordinate system. Two of these coordinates are angles and the third is the radius. Thus $\psi = R\left( r \right)\Theta \left( \theta \right)\Phi \left( \phi \right)$ Where capitals represent the function and lower case represents the variables. Theta and phi are the angles, r is the radial distance from the nucleus. For all s electrons the angular part is constant for all values of of the angles. This is because the s orbitals correspond to the zero value for the subsidiary quantum number, l So all s orbitals have spherical symmetry, so only the radial part R® need be considered. The probability of finding an s electron in a small element of volume dv at a distance between r and (r+dr) from the nucleus is then ${\left[ {R\left( r \right)} \right]^2}dv$ This is the same regardless of the direction (ie the angles) of the radius vector. The total probability of finding an electron anywhere between r and (r+dr) is found by integrating the expression over a spherical shell between r and (r+dr) ie replacing dv by the total volume of the shell. This volume is $4\pi {r^2}dr$ So the integral now in terms of r becomes $4\pi {\left[ {R\left( r \right)} \right]^2}{r^2}dr$ This function is known as the radial probability distribution, which is plotted on your y axis. Are you with me so far?

I assume the quote from your question was a misprint and you do indeed mean radial, not radical. That makes sense. Yes they are the correct graphs. Important features. Well maxima or minima are usually important featuresof interest in a graph. Alice the camel has 2 humps. How many humps has the 1s, 2s and 3s? Are they all the same size? What is plotted on the axis X axis? Hint hence the radial distribution Y axis ? Note the function plotted is (proportional to) the square of the wave function - What does this signify? What do you thing the r2 is doing in the y axis function?

Yes you are probably much happier than any citizen has any right to be. I will dispatch the thought police immediately.

I wondered if you envisaged more turns when you first posted and I can see the machine you are describing as a combined motor and generator with alternate turns connected to the motor and the generator. This would work, but it is not in contravention of Lenz law, which I think you misunderstand. Both the motor windings and the generator windings are subject to a generated emf, known as back emf. However the back emf serves different purposes in the two sets of windings. In the motor section the back emf serves to limit (reduce) the 'applied voltage' and thus the drive current. It does indeed opposes the driving emf directly. In the generator section there is no driving emf or applied voltage. There is only mechanical driving of the shaft by the motor section. The Faraday voltage generated obeys Lenz law and provides the emf to power the external load lamp. This explanation from 'Nightingale' may help. Exactly what happens will depend upon the relative circuit values; the driving battery voltage, the circuit resistances in the motor and dynamo circuits, the lamp load resistance. However there are devices known as rotary converters which can convert (battery) DC to other values of DC or AC supply. These are less common today with modern electronic alternatives, but are still used for very high powers. Rotary converters work on essentially the same principles as we are discussing.

I suggest that a fruitful line of enquiry into the reported differences between gender differentiated performance in doing maths and reading (and perhaps even other activities) is to look at the questions Why do people read? Why do people do maths?

In defence of models, their very imperfection is their greatest strength. A perfect model matches every characteristic of that which it models in every detail, both discovered and yet to be discovered characteristics and possesses no unmatched details of its own. So the only perfect model of say an electron is an electron. Our imperfect models are much simpler and only match some (hopefully desirable) details over some limited range. But they also come sans the details that are not of interest and do not therefore need wasting effort on. As regards infinities, mathematics in this discussion has become more technical than the OP was able to support and I commend the discussion in appendix IV of Hardy's famous book (A Course of Pure Mathematics, Appendix IV, The infinite in analysis and geometry) where he discusses the Aristotelian idea of real and potential infinites in the light of 20th century mathemnatical knowledge. As regards infinities in the real world, I have a question for consideration. In quantum theory an electron has many but finite energy states available to it whilst it is part of an atom. According to quantum theory, these energy states become closer and closer together as energy increases until the energy reaches a point where the energies are contiguous and a 'continuum' of energy states arises. So the question arises what is the enumeration of the energy states within the continuum?

That's why it's called pop-sci.

Not so. I did not originally offer comment on the data but summarised the survey conclusions for others to comment. The thread was later widened by others to include reading and I do not object to this. In fact I welcome it as a natural extension to other observed gender differences. This is the second time to have serouiusly misquoted my statements. I did not claim this at all. Strictly I claimed that girls appear to buy more comics than boys. Nothing at all was said about their preferences. In my turn perhaps I did misunderstand some of what you wrote. I apologise for any of this. Data should be tested not only against the original collection but also against other known or observed data or theory. If a conflict is found the proper conclusion may well be that further observation is necessary to answer the discrepancy. Data, after all can be sometimes misleading and sometimes actually false.

Nice work if you can get it. But if you live in the EU, particularly in the UK, you are required by law to do many things online these days. from driving licence to tax to farm movemnt orders to all the other umpteen government forms we have to deal with. Business is also trying to force this more and more.

That is not a justifiable conclusion. I have not disputed the data collected by the surveys. However I am fully entitled to dispute any subsequent, analysis, interpretation or explanation. It is for the surveyors to show that that any other explanation is invalid, I merely have to find one, I don't have to prove it as I am not offering it as gospel.

Far too prescriptive. No it is not my interpretation , I attributed it to your own goodself. No it does not follow that what people (of any age or gender including children) do is the same as what they say the would prefer to do in a survey. This was my principal point. I do not have total circulation figures for comics for boys or men comics v those for girls or women, I did however observe that there is a greater selection of girls and womens which, being subject to extreme commercial pressures, would suggest that girls and women buy more comic reading material and men and boys. That too is data. You cannot pick and choose which data you like and which data you don't, but you can try to understand the data you have and its progeny, before drawing invalid or unwarranted conclusions. How does that play with a survey of 'preferences'?

Go well with your chemistry studies, Dan, but please remember there is much overlap with Physics (and other sciences). Sometimes, however, there are distinct differences. Energy is very important in Chemistry, but beware sign conventions. Chemists use the very understandable convention that energy out is negative and energy in is positive, whatever form that energy takes. So they write the law of conservation of energy (or the first law of thermodynamics) dU (or dE) = q + w The change in internal energy is the sum of the heat supplied to and the work done on a system. Physicists use an older, more complicated convention. With them , heat evolved is negative but work evolved is positive. dU = q - w This causes an enormous amount of confusion for the unwary when reading the literature. The Physicists' convention is also used by engineers since they developed it for heat engines where heat was supplied (+ve) for the engine to do useful work (also considered +ve)

That doesn't prove they read anything at all. The comment was specifically about UK teenagers, what relevence is the Pell Institute of Washington DC? Of course it was relevent. You claimed or inferred that boys preferred comics and girls preferred more meaty text. Yes boys like comics, but that does not mean boys don't like more substantial text. Perhaps boys write a greater quantity of substantial text, we have just had the 400 anniversary of Shakespeare; Shakespeare was a boy. The relevence is that I suggested that (in the UK at least) publishers under considerable commercial pressures disagree in that they off rather more girl's comics than boy's and extend this into adulthood. I would agree it is a commonly held perception, but is it true?

Yet if you go into any UK newsagents and look at the teenage section. How many comics for boys are there and how many for girls? Further how many girls carry this on to to later life, only the comics are now called magazines?