-
Posts
18483 -
Joined
-
Last visited
-
Days Won
108
Content Type
Profiles
Forums
Events
Everything posted by studiot
-
Minette doesn't only appear in the sout west UK, it also appears in the south west US. https://books.google.co.uk/books?id=d_mqypQ0YgAC&pg=PA236&lpg=PA236&dq=minette+rock&source=bl&ots=XjhTkm4DCE&sig=8XzgvEGWh9FSPaICK1P73YphnHQ&hl=en&sa=X&ved=0ahUKEwiI-7aX8rfJAhXMOhQKHZm8BYsQ6AEIQTAK#v=onepage&q=minette%20rock&f=false
-

Does mathematics really exist in nature or not?
studiot replied to seriously disabled's topic in General Philosophy
The only thing that is clear here is that you have either not read the original post properly or that you have ignored it. To refresh your memory the question was and still is "Does Mathematics exist in Nature?" If Nature does not exist then nothing it contains can exist and we have no discussion. So for the purposes of having this discussion we need to assume the existence of Nature. For those who think that the original question did not include the possibilty that some part of the whole of Mathematics is manifest in Nature and wish to stick to numbers, why would you expect counting in Nature to be the same as counting by humans? For one thing counting by humans is not always accurate, humans make mistakes. Animate entities already discussed also make mistakes. But inamate Nature can count with 100% accuracy. For example when sodium chloride or calcium chloride dissociate into ions, exactly the right number of electrons is always transferred. Never ever one too many or one to few or 1.3 times the correct number. That fellow fallible humans is counting. -

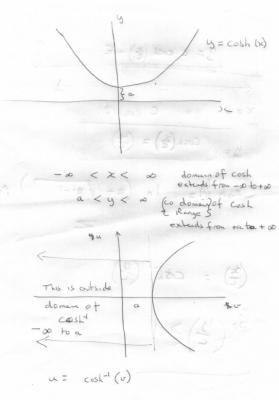
Catenary solution problem solving for constant 'a' in cosh formula.
studiot replied to Mike_B's topic in Applied Mathematics
I noticed a small error in line 3 of post#4. Perhaps some kind mod would put it right. It should read [math]\sinh (w) = \sqrt {{w^2} - 1} [/math] Of course it should be noted that these equations only apply when the cable is uniformly loaded throughout its length. This is sometimes the case but not for engineering structures such as bridges and cable stayed/supported structures. Telephone wires and some washing lines are heavy enough for their own weight to be sufficient loading, but for lines that approach weightless the curve approaches more and more nearly a parabola. The above is the mathematical approach, a good treatment of the engineering approach to simplify things is given in Pippard "Analysis of Engineering Structures" -

Does mathematics really exist in nature or not?
studiot replied to seriously disabled's topic in General Philosophy
Although I have previously discussed counting and number in this thread I really can't see why members are so obsessed with restricting Mathematics to number. I have, in my library a book entitled The Mathematical Description of Shape and Form. It is a most useful and practical textbook for the subject, which is a part of Mathematics at least as old and important as counting. But here's the rub. There is all this nonsense about order and pattern being spouted, as though there were shapes and forms that were mathematical and those which were not. Mathematics can and does describe any shape whatsoever, as well as regular ones. So the shape of say the earth is well, Earth shaped. That shape is unique but appears in the set of all possible shapes in Mathematics and in the Earth in Nature. I think we can take it as read that we are not debating the existence of the Earth in this discussion. If the very existence of Nature itself was under debate then there would be no point in this thread. -

Catenary solution problem solving for constant 'a' in cosh formula.
studiot replied to Mike_B's topic in Applied Mathematics
Let [math]x = {\cosh ^{ - 1}}\left( w \right)[/math] Then [math]w = \cosh \left( x \right)[/math] So [math]\sinh \left( x \right) = \sqrt {{w^2} - 1} [/math] Therefore [math]{e^x} = w + \sqrt {{w^2} - 1} [/math] Take logs [math]x = \ln \left( {w + \sqrt {{w^2} - 1} } \right) = {\cosh ^{ - 1}}\left( w \right)[/math] as required. The attachment shows the domain of cosh(x) and its inverse The arc length, is geven as a function of the slope, theta and a. [math]s = a\tan \theta [/math] differentiate [math]\frac{{ds}}{{d\theta }} = a{\sec ^2}\theta [/math] also [math]\frac{{dx}}{{ds}} = \cos \theta [/math] So [math]\frac{{dx}}{{d\theta }} = \frac{{dx}}{{ds}} \bullet \frac{{ds}}{{d\theta }}[/math] [math]\frac{{dx}}{{d\theta }} = \cos \theta a{\sec ^2}\theta = a\sec \theta [/math] Integrate [math]x = a\int {\frac{{d\theta }}{{\cos \theta }}} = a\ln \left( {\sec \theta + \tan \theta } \right)[/math] Similarly [math]\frac{{dy}}{{d\theta }} = \sin \theta a{\sec ^2}\theta [/math] Integrate [math]y = a\int {\frac{{\sin \theta d\theta }}{{{{\cos }^2}\theta }}} = a\sec \theta + D[/math] Which gives x and y as functions of the slope. Constants c and D can be made zero by suitable choice of coordinate axes, as with the other solution. -
This thread belongs in speculations, if anywhere.
-

Catenary solution problem solving for constant 'a' in cosh formula.
studiot replied to Mike_B's topic in Applied Mathematics
What are you using your catenaries for? Do you have a derivation for my logarithmic formula and have you seen the alternative secant solution to the catenary? -
Macroscopic is a term that is relative to the wavelength, ie much bigger than the wavelength. The wavelength of a 100Hz soundwave is 3.4metres.
-

Catenary solution problem solving for constant 'a' in cosh formula.
studiot replied to Mike_B's topic in Applied Mathematics
It is not clear how you are solving your equations. However there are restrictions on the domain of the inverse hyperbolic cosine in particular for any w [math]{\cosh ^{ - 1}}\left( w \right) = \ln \left( {w + \sqrt {{w^2} - 1} } \right):w \ge 1[/math] Given these restrictions let us consider your equation [math]y = a\cosh \left( {\frac{x}{a}} \right)[/math] [math]\frac{x}{a} = {\cosh ^{ - 1}}\left( {\frac{y}{a}} \right)[/math] [math]\left( {\frac{y}{a}} \right) \ge 1[/math] [math]y \ge a[/math] -

Is logic a branch of philosophy or maths?
studiot replied to andrewcellini's topic in General Philosophy
Yes I awarded you +1 for staying awake for 48 hours, but had to take it away again for rambling whilst in that condition and particularly because my lotto numbers didn't come up. Sorry. There are several things wrong with your rambles. Here is the shortlist. No I do not think that Mathematics is constructed to explain the universe. There are plenty of things and matters that are outside the boundary of my Venn diagram. So there are plenty of things in the universe that methematics does not address. This, of course, is also true of Logic and Philosophy and indeed every other discipline. Mathematics and Logic do not set goals. That is an important statement because that is why human activity is not constrained by either. As an aside, nor does Physics set goals. That is part of the reason they both address the question of 'how' rather than 'why'. Human activity also includes rational thought, sensible sensible thought (and their opposites). These are not the same as the logic.discussed in this thread. You should perhaps read the scifi novels about A and NullA by AE Van Vogt which explore the boundaries of formal logic. https://en.wikipedia.org/wiki/The_World_of_Null-A It is also instructive to study how electronics engineers have diverged from formal logic. Firstly the customary presentation of formal logic requires two connective 'and' & 'or'. Theoretically this can be cut down to one, 'nor', but this is rarely done. Electronics engineers however have built a huge industry based on the nor gate. (Yes I know that and & or gates are also available but nor gate implementations are usually more efficient.) But they have gone further and implemented a whole family of devices and system of logic known as 'tristate', which extends the formal logic discussed above. I look forward to more accurate predictions for next week's lotto. -

Is logic a branch of philosophy or maths?
studiot replied to andrewcellini's topic in General Philosophy
Agreed, although rather than say 'true' which opens another can of worms, I prefer to say 'is compatible with the stated axioms of the discourse, along with their derived theorems' For example the fundamental theorem of algebra asserts crudely that every polynomial has a root (and therefore 'the existence' of complex numbers) So [math]\exists z:z = \sqrt { - 1} [/math] Means that the square root of minus 1 is compatible with the rules of algebra when we include the complex numbers. -

Is logic a branch of philosophy or maths?
studiot replied to andrewcellini's topic in General Philosophy
Perhaps you are not aware that the statement "There exists" has a very precisely defined meaning in Mathematics, as well as its own symbol. And this meaning is different from the definition used in other disciplines, including general English and in Philosophy. Yes it is a separate discipline, but it is nonsense to suppose it has any desire or will or capability to supplant any other discipline. Actually it deals with aspects of chance, not dealt with by Mathematics. I would say that Philosophy does not deal directly with the numbers (probability), that is the province of Mathematics. -

Is logic a branch of philosophy or maths?
studiot replied to andrewcellini's topic in General Philosophy
Because there are elements of mathematics that are not reflected in philosophy (treatment of chance for example) and elements of philosophy that are not reflected in mathematics (discussion of what is existence? for example) -

Trouble with Ohms Law and some advice please
studiot replied to ohdearme's topic in Classical Physics
OK, we have established that you need a more robust 16 volt DC power supply. Since it seems to be a waste of money getting a Logitech one, you have several options. 1) Some older laptops have 16V DC supplies. More modern ones are in the 19 to 21 volt range. I have PM'd you a link to an IBM 4 amp one for £1.20. Or look in a computer shop. 2) Some Yamaha music equipment uses 16 volt DC 2 -3 amp adapters. So look in a music shop. 3) A universal adapter around 2.25 - 3 amps capacity and set it to the correct voltage. However some cheap versions of these don't last any better than the Logitch variety. A couple of further points. Make sure you have a decent UK mains plug connection, some click together ones are poor to dangerous. Make sure you have the right plug connector on the output unless you are prepared to change the one that is there. This is a disadvantage of the universal type. the click together all purpose connectors can easily become undone or just loose. Cheers -
Useful summary Sensei, +1
-
It is about probabilities, not certainties. There is a possibility that the EM radiation from a cellphone will activate circuitry in an unwanted way. Therefore there is a finite probability of this. Imagine, for a moment, that you are blind and standing by the side of the road to cross. You could simple march across, like small animals do. There is a possibility that you could get across without being hit by traffic and a possibility that you could become roadkill, like many small animals. Would you take that chance? Back to the cellphone. Electronics works on electrical energy, and as the industry has developed the amount of such energy needed to create an electronic action in electronic devices has fallen dramatically. Even the device leads and connections on a circuit can pick up EM (electrical) energy that is passing through. Mostly this pickup falls beneath the threshold to alter circuit action , but as electronic devices uses less and less power, the gap between pickup and that threshold grows ever smaller. However in order to communicate with a cellphone mast, the phone must broadcast a minimum quantity of EM energy. This is not really getting smaller these days. Further many other electronic devices nowadays operate in the same frequencies as cellphones, so pickup will be more efficient. So there is a real possibility that ever more sensitive equipment will pickup a small pulse of EM energy from a cellphone suddenly communicating with a mast, and then malfunction. Again would you like to take the risk of being connected to that most modern piece of medical gadegtry at the time? Incidentally it has always been known that not only EM radiation, but ionising radiation and even neutrinos can affect computer memory by changing the charge on a single memory cell. And the actual charge a memory cell requires to change/ indicate state is incredibly low these days. So, even without cellphones, computers are vulnerable to memory degradation from passing radiation. And medical devices (like many others) routinely incorporate memory these days.
-
Yes, I looked up the Mandelbrot reference for you and I didn't realise how long ago his famous book (in English) came out. 1982. You really ought to read The Fractal Geometry of Nature. It is not too mathematical and I don't think there is even any calculus in it. However the book is a masterpiece. It answers many of your questions about fractional dimensions, the nature of dimensions, and even (if I remember correctly) discussues the Peano space filling curves I referred to. It has lots of pictures and diagrams , which should keep Mike Smith Cosmos happy as well. Be aware the the word point as used in set theory is just another name for an element or member of a particular set. So in the set of all numbers, each number is called a point, even though there are are an infinite number of them in any interval. Finally your thinking about points is perhaps closer to the ancient Greek thinking Euclid definition: A Point : That which hath no part. A Line: A breadthless length. Note that Euclid regards lines as starting and finishing so had at least two points (each end). Modern maths regards a line as going on forever, without end points. Further there was a religious dimension (aspect) to ancient Greek mathematics. They regarded geometric figures, points, lines etc a somehow 'perfect' or embodiments of perfection as achieved in whatever heaven they believed in. Our real physical world was regarded as just a pale shadow or copy of the 'real' thing.
-
Quickly since you are still online. I think you have misunderstood my comment about limits (though ajb did not) I mean the formal mathematical limit the we use [math]\mathop {\lim }\limits_{\delta x \to 0} f(x)[/math]
-
Exactly. So it depnds upon which set, which was the essence of my post. So by choosing an appropriate set you can endow your point with any number of dimensions you care to, according to any definition you choose to use.
-
I think there is rather more to it than this. We are again talking about the difference between the value zero and nothing (= no thing). So let us consider density = mass /volume. What sense does it make to state "the density at a point is"? A point has zero volume so you are dividing by zero. Yet the applied maths world happily uses density every day. We get around this conundrum by taking a limit. Of course the same issue applies to other properties besides density, pressure for instance. Pressue is Force/Area and we need the use the same limiting process when we consider pressure at a point. So should I consider a point as a line with zero length, a square of zero area or a cube of zero volume? These have 1, 2 or 3 dimensions respectively. To make matter worse we can also consider the reverse situation. How many dimensions has a cube? Am I bid 3? What about (1 dimensional) Peano curves then? At this point (pun intended) you need to ask the Hausdorf/Mandelbrot question What is a dimension?
-
36grit, You might like to know that the Logitech Z320 speakers we are discussing in this thread http://www.scienceforums.net/topic/92308-trouble-with-ohms-law-and-some-advice-please/ include a pair of back to back mounted speakers to create their so called omnidirectional response.
-
Yes there you are the earliest reference I have is The Acoustic Labyrinth B Olney Journal of the Acoustical Society of America Vol 8 , No2 1936 So at least 80 years ago.
-
Right at the beginning of your link. 'transmission line' A La Bailey et al. Bose are very late at the gate.