-
Posts
18483 -
Joined
-
Last visited
-
Days Won
108
Content Type
Profiles
Forums
Events
Everything posted by studiot
-
Thank you for bringing this theory to our attention. Perhaps if it was couched in less sensationalist language that accorded with current defintions of specific technical words it would garner greater credence. For example I have no idea what this means simply because all the technical words used clearly have different meanings from currently accepted practice. What is your definition of vector? What is energy momenta? What is a single quanta of energy? What is the meaning of will associated with energy? What is the definition of diverge in this usage? What is the definition of neutralize in this usage?
-
I found this at the top of the reference readily enough. Personally I regard the Preamble to the American Constitution as one of the masterpieces of political and social thought in English. I don't know much about Jefferson though.
-
Thermodynamics was originally developed to study the relationships between thermal and mechanical properties, but was later extended to include other properties involving energy. Its laws and methods may be applied anywhere energy is involved. Thermodynamic theory divides a universe into two parts: The ‘system’ and the rest of a universe. I say ‘a universe’, not ‘the universe’, because this includes everything of interest or relevant in a particular thermodynamic discussion, both inside and outside the system., but may exclude other parts of the Universe at large. It displays an identifiable boundary between these two parts. This boundary may be fixed as in a chemical reaction flask. Or it may be part moveable, part fixed as in a piston in a cylinder. Or it may be wholly moveable as in a balloon. Further it may have physical concrete existence as in the above examples or it may be a theoretical surface such as the space surrounding a molecule. Incorrectly/inappropriately specifying the boundary is a common source of difficulty. So when considering the thermodynamics of heating some reagents in a flask or braker to form products, The bunsen burner is part of the rest-of -the-universe (not the system). The flask is the fixed part of the boundary and the liquid surface the moveable part. Finally a particular Thermodynamics discussion deals with a specified ‘Thermodynamic Process’ of interest. This may be a single stage process and may involve the entire system. For example blowing up a balloon. Or there may be several processes in action in different parts of the system. In this situation the system may be broken down into smaller subsystems, each with its own process, and sub boundary. A process may be one-time or cyclic or may even be stasis (=Thermal Equilibrium). Once we have defined the system, its boundary and the process involved, we can study the system properties. There are directly observable variables such as pressure, volume, temperature, number of molecules, phase (solid, liquid, gas, dissolved) etc. We also identify derived or calculable variables such as internal energy, entropy, enthalpy and so on. These variables are calculable using some of the many thermodynamic relations or equations that are known. Some variables may be mathematically treated as constant for a particular system. Examples would be specific heat, density, redox potential, ionisation potential. A complete list of the values of the properties is called the State of the System. We may equally apply this to the rest-of-the-universe and talk of the state of the rest-of-the-universe. Since the above variables are define the state of the system they are called state variables. Yet more variables have a particular and very important role to play. These do not apply directly to either the state of the rest-of-the-universe or to the state of the system but to exchanges between the two. These are hugely important and form the basis underpinning thermodynamic theory. These variables act across the boundary so linking the system to the rest-of-the-universe. Originally they were heat input and work extracted, but now the list has been extended to other forms of energy such as electromagnetic. It is important to realise that heat and work are not state variables, and by themselves cannot define the state of anything. Nor are they properties of the system. On to part 2
-
Bomb the iraq oil fields? Isn't that what Saddam Hussein did to Kuwait and, at least in part, why we went to war there ?
-
If you bothered to read my post173 before you grumped this might be a more convivial thread.
-
What people have been trying to tell you, here and elsewhere, is that mathematics has very specific definitions of (1), (2) and even (3). Using these definitions mathematics can even offer a meaning to (4). So the rest of the world considers these matters of mathematics, not philosophy (5). You seem to have different personal definitions for (1) (2) (3) and most particularly (4), so it is not suprising that your conclusion (5) is not in accordance with the rest of the world. Until you can bridge that gap you will never communicate, for certainty the rest of the world is not going to change to accomodate you.
-
Yes, but we don't use gamma rays to carry signals. No there is no law concerning this. There is no reason to assume any upper limit, just because we have not yet encountered such waves. We normally take zero (the stationary steady state) as the lower limit for real waves, but negative frequencies may be used in mathematical theory. Negative frequencies again have no lower bound.
-
The energy lost to sound is negligable in a jackhammer and inherent in the mechanics. Muffler arrangements do exist, but they simply absorb the sound, they do not and cannot prevent it. Having said that some hammers are noisier than others.
-
Here is my simplified take on the subject, based on the trickle-down model. At one end of the scale the pute mathematician like to generalise. This means she doesn't like to solve specific problems like : solve x+4 = 6, but would rather solve all such problems and say that The solution to equations of the form x+A = B is x = (B-A). Another example would be the general statement that a function f(x) is maximum if f'(x) = 0. Enter the Mathematical Physicist who says Ahah that's interesting so if I have a specific formula such as the power transferred to a load R in an electrical circuit is givne by the equation [math]P = \frac{{{E^2}R}}{{{r^2} + 2rR + {R^2}}}[/math] Where E is the source voltage and r the source resistance And I differentiate and set to zero [math]\frac{{dP}}{{dR}} = 0[/math] This leads to [math]R = r[/math] Which is a formula for a general property of electrical networks derived from an even more general formula in mathematics. Long comes the Engineering mathematician and says. Oh that's interesting I have a need to maximise the power transfer from magnetic pickup heads to an electronic circuit. The value of r for my heads is between 20k ohms and 100k ohms so if I make the input R for my amplifier 47k ohms that will be good. And that is reason, o best beloved, why magnetic pickup inputs on audio amps are (usually) 47k. (Have you ever read Kipling?)
-
Coincidentally I have been reading a mediocre science fiction thriller that turns out to be in part about aliens dividing by zero. Deep Storm : Lee Child : 2007
-
You have already wasted money, time and effort on the wrong equipment because you have tried to tell electrical experts what you should have. Instead of saying "I want to stabilise my mains to 220 volts" or even My mains varies and sometimes drops as low as 180 volts. Say I need to supply the following equipment { Insert List} and if possible why you think 220 volts is so critcal. Then let the experts ask the questions designed to point you at the appropriate solution. Also if I understand you correctly you are saying that you run power devices in the 10 amp range at 220 volts without any safety precaution (ie no earth) I would advise you this is very dangerous and against Pakistan Electrical codes since at least as far back as 1937. https://www.google.co.uk/#q=Pakistan+electrical+earthing+code
-
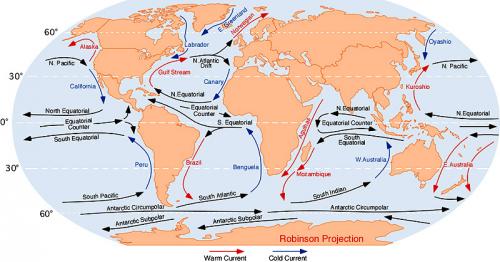
An ocean has several characteristics that make it self contained. One of these is ocean currents. You can see from the picture (courtesy NASA) that the Southern Atlantic Ocean and the Northern Atlantic Ocean are effectively separate oceans in this respect. And of course the Indian Ocean is another. The currents circulate the water within the given ocean basin. Note that the S Atlantic current is cold and the Indian O current is warm on opposite sides of Africa. Further to the south lies the Southern Ocean that surrounds Antarctica and the current there is known as the circumpolar current because it goes all the way round. Shouldn't this be in Earth Science, not Chemistry? Note this is a simplified explanation, but a good start.
-
Way back at the beginning you were dismissive when I commented that many others had had similar ideas before you and that a great deal of work had already been done and suggested that you could save yourself effort by tapping into this and adding your two penn'rth to the world wide effort. To save Professor Strange some hair note that pairs and larger groupings of numbers are identified with many mathematical objects, each with unique useful properties, such as vectors, complex numbers and quaternions, Bra and Ket, to name but a few. When you have more than two, more complex groupings are possible as with Matrices, Tensors etc. The correct word for this is n-tuple (where n is 2 in your case) https://en.wikipedia.org/wiki/Tuple Since there is already a well developed mathematical framework for these things, it is encumbent upon you to develop your system in line with this framework (extending it as necessary) and all the (mathematical) world will applaud if it turns out that you have genuinely thought of something new.
-
Has Christmas come early this year?
-
There is an awful lot to get your head around in this part of physical chemistry and it takes a lot of time and effort. But you seem to be making real progress. The thing to remember about energy is that there are lots of forms of energy and most can be converted from one form to another. However Nature is not that generous because it exacts a charge for doing this and that is what quantities like Gibbs and Helmhottz energy and entropy are all about. Ask about these when you are ready for a gentle introduction.
-
Think about Mt Everest as a self supporting skyscraper of height just under 9km. The mountain is basically solid (ie has no internal rooms). But to 'stand up' it sinks into the crust of the Earth many km. Many being 5.6 times its height http://geoscience.wisc.edu/~chuck/Classes/Mtn_and_Plates/mtn_roots.html see the section marked implications for mountain ranges.
-

The "Whatever Theory" Identifying The World...
studiot replied to whatever theory's topic in Speculations
I have already proposed more modest goals , and this was accepted. Thank you for your comments, I need all of the nudging I can get. -

The "Whatever Theory" Identifying The World...
studiot replied to whatever theory's topic in Speculations
You still have not appreciated the implications of what I said about digital phtographs and programs. Programs change the actual RGB values of pixels to suit their purpose. I would add to Strange's list that error of continually introducing new material (examples) before fully dealing with existing ones. With every point you introduce new photos and new goose chases. Small wonder you are 'running round in circles'. I posted that one single photo as it contains examples of almost all the effect I and others have mentioned, including the most recent that of the influence of adjacent colour. But don't follow string junkie's advice and give up. Reappraise and regroup and follow at least some of the sound advice given here. Here is a good site to find out a huge amount about the vagairies of digital photography and colour. They have lots of tutorials. http://www.cambridgeincolour.com/color-management-printing.htm Look up Antialiasing Subpixel rendering https://software.intel.com/en-us/articles/conservative-morphological-anti-aliasing-cmaa-update -
For those of us that don't know or are just as thick as me can you tell us what an atmospheric river is please.
-
Of course it does. The rate of charge entry equals the rate of charge exit. So the rate of accumulation of charge is zero. The summed charge is not the same as the accumulation of charge; the summed charge must include all charge entering and leaving the control volume in the time period.
-
You have not responded to my first answer.