studiot

Be warned I find this information highly unreliable. Software glitches ensure inconsistencies between the various times presented. You can often see a post attributed to a member who was also recorded as having logged out 3 minutes earlier. If you two (or more) members are shown as viewing a thread at the bottom, this does not change for a long time after one logs out. I have often scrambled to post a reply whilst I thought the other guy was still online, only to find he had logged out a quarter or half an hour before I finally posted.

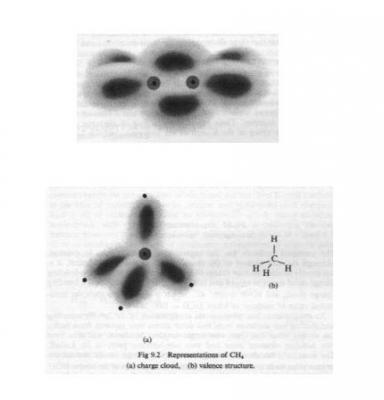
No the methane pics both represent a 3D picture. Pretend the carbon atom labelled C is a blue ball and the hydrogen atoms, labelled H are red balls and the tapered lines are sticks. Then you have a ball and stick model. I did say to ignore what was inside the atoms and neither of my pics attempt to show the details of the connections in the way the Lewis representations do. None of these are Lewis representations. To understand these would mean going into the structure of the atom and considering some sub atomic 'particles', we call nucleons and electrons. The Wiki article shows some 3D structural (ball and stick) models at the top which is misleading because these are not the Lewis representation. A Lewis example of this appears about halfway down the page with two images of the nitrite ion. Lewis examples are all flattened to 2 dimensions and are used to show the nature of the chemical bonds involved, as well as the electrons not involved in the bonding process. They are, of course, an example of a different model appropriate for a different purpose. We have been talking about structure which, loosly speaking, is about the distribution of things in space and to a lesser extent what is directly connected to what.

What do you mean charges don't cancel? Water is electrically neutral. If you are referring to the eletrical dipole moments please expand as this could be confusing.

It is more like the second one, but you have the general idea. This does not mean the ball and stick model is wrong or redundant. That model is useful for other purposes. Real world molcules are more complicated and unlikely to be symmetric. Many are three dimensional. This fog approach is called the charge cloud representation. Here are the charge cloud representations for oxygen and methane. Compare the ball and stick with the CC for methane.

Be aware of the difference between force and energy. Magnets do not have energy flowing into or out of them. Magnetism is a force. Exerting a force may or may not involve energy. For example the Earth continues to exert a force on you through gravity, but no energy flows into or out of the Earth, unless you fall out of the sky. In the same way a magnet may cause another body to move, doing work in the process. But something moves or holds the magnet. This is like poking something with a stick so it moves or drawing it along with a string. Either way the stick or string exerts a force on the object, but something else has to push or pull the other end. If the object does not move then the force can remain exerted till kingdom come and no work is done, so no energy flows.

My comment about atoms and molecules was meant to be a helpful simplification (particularly as you will meet technical terms as your reading progresses) So I am interested in what you made of it. As to space. Think carefully what how you mentally picture an atom (from a distance, lets leave the inside till later) You must have seen the 'ball and stick' models of molecules (there you go, a technical term) made of coloured balls to represent the atoms making up a mlecule and the sticks to connect them. So when they are close enough to be joined together there are 'connection sticks' in the space between the atoms. These are not solid or other atoms so how do they work? Well instead of picturing the atom as a solid ball, think of a mist or fog. As you walk through the mist some patches seem thicker, more dense than others. The more tenuous parts may even thin out to clear air. Furthermore if you walk far enough you will exit the fog and enter clear air. Now think of an atom as being a clump of particularly dense fog but thinning out towards its edges, and think of two such clumps coming close together. As they appraoch each other the thin edges will touch first, forming a thicker zone between the two clumps. This thicker zone corresponds to the sticks in the ball and stick model. It is formed by overlap of two fuzzy edges of the atoms. How are we doing so far?

For the most part we cannot answer a question "What is XXX ?" The best we can do is create models. We obtain observations and measurements and call the sum of all htis the real world. Clearly since new observations and measurements are continually being made the 'real world' is continually being updated (like Microsoft Windows ) We also create completely abstract constructs with artificial rules and relationships. Many are mathematical. Some of these can be used to model observations and measurements in the real world and even make predictions. But none of these models are exact or perfect. Magnetism is a case in point. We know today that the source of all magnetic effects is electric in origin. But a simple model, That of the separate existence of a magnetic force affecting certain objects for some purposes will make the required prediction easier. On another forum I posed a calculation question about lifting a car in a scapyard with a crane magnet. Members there disputed non electrical explanations and one member with a PhD and many years experience in electrical and magnetic matters finally showed several pages of vector mechanics to calculate the selfsame result I had achieved in three lines. I employed what is known as the method of Virtual Work, and did not need to mention electricity at all. So your question really need lots of context to provide a sensible answer. What are you actually seeking to know?

Well I was referring to the connection between straight lines and geodesics in Newton's First Law. I don't know if Galileo ever referred to the important concept of The Right Line

For static charges or steady current, the statements:- The E field is conservative, has a scalar potential, V (as introduced by imatfaal), has a zero curl, has a line integral around any closed curve equal to zero, are all equivalent statements that are proved by simpler means before Maxwell is introduced. Since these are all mathematically equivalent only one is chosen as the basic and the others follow mathematically. One of the difficulties in replying to this is avoiding a circular argument. So where does your course start? Also where do you stand on vector algebra and vector calculus in 3 dimensions?

Was this a quote from Euclid or Newton Or..............?

I expect that was a simplification imposed by your teacher. What did she have to say about Helium and Avogadro's Hypothesis? Historically the concept of atoms was introduced followed by the concept of elements and finally the concept of compounds. Three laws were enunciated The law of Definite Proportions The Law of Constant Composition The Law of Multiple Proportions These codified the idea of 'pure substances' that could be formed from or reduced to simpler forms of matter (called elements), which could not be reduced further. The natural question arose "If an atom is the basic form of an element, what is the basic form of a pure substance?" This lead to the idea of a molecule, after quite a few false trails and some obstruction by some older scientists, lead by Berzelius. The concept of a bottle or bag of a pure substance containing multiple instances of identical units is simple and alluring and offers a working explanation for many physical observation. But it is only a model and not always reliable. For instance what does a molecule of pure diamond look like? If you are still reading this rather than moving on I was going to discuss your view of the space between atoms with some hopefully helpful comments. Are you still interested?

I'm sorry I didn't make myself clear. I was not asking how the temperature changes were deduced. Your vertical axis plots change of temperature. Change from what temperature? Is the change from a common base, an average or some function of the previous temperatures? Also what about the particulate counts?

The fraction by weight of a particular constituent in a given precipitate. For example the chemical factor for Fe in Fe2O3 is 0.6994. So if we have a precipitate of 10g of ferric oxide it contains 10 x 0.6994 g of iron Good gravimetric analysis texts offer tables of chemical factors. You can also use stochiometry of the reaction to calculate the fraction yourself, if you do not know the factor. Can you work out how to do this?

I'd be interested to learn more about those graphs. Pavel. What does the temperature variation $\Delta T$ vary from? And what is is the variation in temperature of? The carbon dioxide levels are self explanatory. The dust levels are stated as ppm dust levels in what, thae atmosphere, the ocean? And ppm measured how? The correlations are interesting, however, and hopefully less distorted than the Gore efforts. Many thanks.

So did I, isn't it?

Not to contradict ajb, but to add: It should be noted that the value of something may or may not be equal to its limit, if it only has one limit.

Although I realise you are interested in testing out Maxwell, you don't need Maxwell and vector calculus to solve this one. Indeed having a physics feel for what is going on is important. With a steady direct current, the electric field situation is the same as a line of charges, viz the charge/current density never changes and the field has radial symmetry. The E (and D) field lines must be at right angles to the B (and H) field lines. As you rightly observe the B field lines form concentric circles around the line of the conductor. How would another set of concentric circles be orthogonal to these? You asked where the flaw in your reasoning lies and I pointed to the step from 3 to 4. In step 3 you correctly say that if the E field is circular then curl E is non zero.. The error is in chosing the circular diagram, not the star in the top left of your attachment. The electric field cannot form closed loops. It starts or ends on a charge or goes to infinity. The magnetic field, however cannot have ends. It can only form closed loops. You can also apply Maxwell to show the consistency of this.

Ever the too practical Scot.

Edit sorry I missed a minus sign $\nabla {\bf{.J}} = - \frac{{\partial \rho }}{{\partial t}}$

Where have you accounted for continuity in step3 to step 4? $\nabla {\bf{.J}} = \frac{{\partial \rho }}{{\partial t}}$ Note continuity is not one of Maxwell's equations. In free space you also have the conduction current J = 0

At least show that you can correctly add two forces.

Ah magic, I should have known it was not real science. Sorry I am in the wrong forum.

Vacuum cored Tx?

Sensei and strange, sounds like you two are quibbling. This is meant to be basic and elementary to present concepts and promote understanding. What exactly did you not understand?

yahya, Did you miss post#4 or are only certain members allowed to comment?