-
Posts
18484 -
Joined
-
Last visited
-
Days Won
108
Content Type
Profiles
Forums
Events
Everything posted by studiot
-

Should we change the forum's name to Religous Forums ?
studiot replied to studiot's topic in The Lounge
So someone finally spotted my irreverend deliberate spelling mistake. The point I am making is that the % is too high IMHO. Other sites seem to have lively technical without religious overtones. Ask Dave, his last site simply bans the subject and is technically lively and friendly with only light touch moderation needed. I have seen folks happily sending speciality technical components and information halfway round the globe, just to help others (and have done so myself), courtesy this site -

Should we change the forum's name to Religous Forums ?
studiot replied to studiot's topic in The Lounge
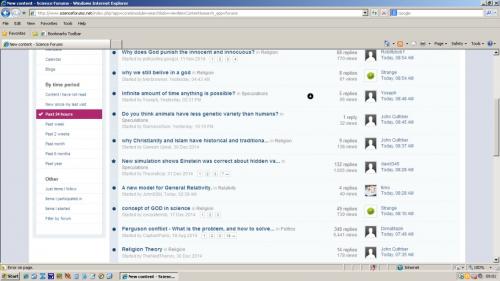
Unfortunately the figures in my screenshot don't (scientifically) bear this out. View totals by category Other : 348 Religion : 154 Science : 136 With science coming in a poor third. -
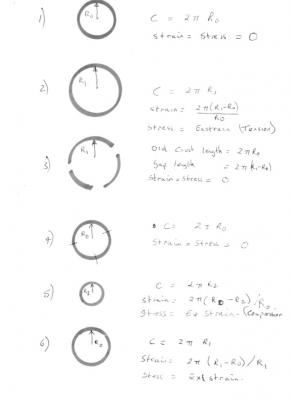
Again good morning, arc. You still have not understood my point so here are some facts and figures for you. Please understand this is the very crudest 'ball park' analysis that would need some refining. If you wish to start expounding on shell theory I suggest you study the classic book by Timoshenko Timoshenko : Theory of Plates and Shells : McGraw- Hill With reference to my sketches 1) As the ancient 'Earth' cooled the outer layer deformed continuously and plastically until it became solid enough to support direct loads. I will call this the base radius R0 and observe this is in the unstressed state. 2) Following your scenario the Earth expands to radius R1, straining the periphery as shown in the equations and inducing a peripheral hoop tensile stress. In my simple model there are no shear stresses at this point. 3) At some point in the expansion this peripheral stress is sufficient to fracture the crust by cracking. Since the crust is no longer continuous the hoop stress is relaxed. ( I have simplified this to zero). This relaxation induces shear stresses between the crust and the supporting interior. The shortening of the old crust leaves gaps in the periphery. 4) The Earth contracts back to its original radius R0 and the gaps disappear as the disconnected segments of crust rejoin. Note these are unstressed. The shear stresses are reversed but there can be no hoop stress since until the gaps close there is nothing to 'buttress' -your term is as good as any' the disconnected segments. 5) One alternative would be that the Earth contracted to a new radius, less than R0. This would indeed induce compression in the periphery as it subsided under gravity. This is the mechanism that could lead to oscillation. 6) Another alternative would be if the gaps were filled with new solid material, before the contraction began. In this case hoop compression would be induced in the periphery as the Earth contracted. You should note carefully, however that the strain would be different for the contraction and expansion so the compression would not exactly mirror the tension, since the definition base length for strain is different in the two cases.
-

Should we change the forum's name to Religous Forums ?
studiot replied to studiot's topic in The Lounge
This will happen more quickly if you don't feed the _____. -
-
With the greatest respect, you mentioned polishing the concrete in the first place. The only way I know to polish concrete is with commercial grinding equipment, just as any other form of industrial 'polishing' for finishing something. You can obtain a glass like finish this way. If you just mean apply wax polish and buff well sorry if I misunderstood. So commercial grinding would give you whatever finish you seek (it's a dirty, dusty process unless you use spongejet http://www.spongejet.com/technology.php) But you must remove any loose material or the resin will not adhere. Some resins are acid resistant, some are not you need to consult the manufacturer. You can also get acid resistant floor paint systems, sorry I can't remember the names at the moment. Beware all resin coatings generate a lot of heat while setting. Follow the instructions exactly and do not be tempted to apply more thickly than recommended. I have seen some resins get so hot they literally caught fire because the contractor tried to put them on too thickly, instead of building up in layers.
-
3. something else
-
Simplified? [math]\sqrt {{V^2} - 2gtV\sin a + {g^2}{t^2}} [/math] Good luck in your exam.
-
presumably d is given in m/s since it is a velocity. Have you drawn a diagram aand calculated the time of flight? Velocity at time t has components Vcos(a) and [Vsin(a) -gt] Hence total velocity at time t is [math]\sqrt {{V^2}{{\cos }^2}a + {{(V\sin a - gt)}^2}} [/math] which can be simplified.
-
Stop spilling acid Ha I like it. To dream the impossible dream. I further assume that the resin coating is to protect the concrete against further damage and will itself be acid resistant. Therefore in the future spilt acid will remain unneutralised and a potentially hazardous. In those circumstances, bicarbonate neutralisation and washing off would be beneficial.
-
The problem with using water is that the OP has said there is limited time. I expect the resin coating they intend to apply requires a perfectly dry surface to bond to or it will fail. I cannot honestly see the need for the bicarbonate. We used to use sulphuric acid to clean off concrete from tools and it acts very quickly. So quickly that there will be no caustic residue left on the concrete. Any damage will already have been done. I further assume that the grinding is to remove the loose (damaged) material to provide a sound surface for bonding. So once again, why not just grind it out?
-
No it was not too long, but I'm afraid it did not address my point. I have raised and lowered several bridges in my time and can assure you that your slabs of concrete would not suffer additional compression, merely from raising and lowering. That would require an external agent. I do not know if the Earth pulsates over a long time period or not, so I am genuinely trying to help you make your model work. This is a discussion not an attempt to prove you wrong or right.
-
So were you able to complete the question? Since the OP has left the scene without further answer and for the benefit of others who might be interested. [math]\frac{1}{{\sqrt 3 + \sqrt 2 }} = \frac{1}{{\sqrt 3 + \sqrt 2 }}*\frac{{\sqrt 3 - \sqrt 2 }}{{\sqrt 3 - \sqrt 2 }}[/math]
-
I can't find any reference to radar in any of my posts, although I suppose I could design a radar system that interfered with itself, whatever use that might be. Nor did I say that all systems were self referential, just that there was an abundance of such systems to be found in reality. I further tried to offer a wide spread of examples to illustrate the point. My words were particulary specific. I should not need to defend myself against retorts, since all I have done is ask questions. So there should be no retorts, just civil answers, politely put. You are the party making the claims so it is encumbent upon you to substantiate them.
-
I said nothing about awareness, or self awareness, and I certainly can't find reference to it in your opening post. Strictly, an 'observation' is made when any object, animate or inanimate, interacts with the subject in a way that would not have happened if the subject's actions had not taken place. The subject's actions would include merely the presence of the subject if something different would have happened had the subject not been present. Several posters have tried to explain this to you, so there is no call for the rudeness of your last paragraph. As a matter of interest it is the easiest thing to prove that dualism is an incomplete and therefore inadequate universal philosophy. But that should be the subject of another thread. So I still await a proper discussion concerning my point.
-
What happens if you bring the square roots to the top of each fraction? Do you know how to do this?
-
Why can't you just polish the stain out? The concrete will have neutralised any acid. Have you a photo?
-
I am still waiting for your proof of your claim that you can't be both observer and observed. I also note that several others have offered views on this.
-
Sauce for the goose..................
-
First welcome, voultsi, and congratulations on your English. I can just about recognise the Greek letters. There have been huge changes in the career paths for both scientists and engineers over the last 50 years. I expect the next 50 will also see great change. You should only think of doing science or engineering if you really want to, not for the money. The money is poor compared to what a clever person can earn pursuing other careers. Any difference between science and engineering is becoming less. People measuring water quality or air quality are considered applied scientists, not engineers. But really all engineering is just applied science. You may have seen CSI or other forensic science programs on TV. This is a growth area to consider. What interests you finding out or doing and making?
-
Hello studiot, welcome back to my thread. I will be a more gracious host this time. My apologies again for not responding properly in the past. There was and is no excuse. And I extend this acknowledgment to billiards as well and anyone else who feel I acted improper towards them. My apologies to them also. '''why the crust enters compression when the core shrinks," Well, gravity. Just as the skin of an apple will wrinkle as the surface area of the hypanthium or flesh, to which it is attached, shrinks, the Earth's crust that is much more rigid will in turn begin to respond with similar compressive energies as the gravitational potential energy slowly increases from the inward displacement of the mantle. The only solution to this growing energy level is for the crust to go downward as subduction, or to go upward as mountain structures. Good morning arc, I nearly missed your reply in the flurry of activity after the disappointing closure of the matter continuum thread (not yours). I don't think you quite understood my comment, so I will try to expand. If you inflate a (semi)rigid skinned balloon (eg a metal foil skin) to put the skin into tension. All that happens if you then remove the internal inflating force is that the balloon resumes its former diameter. The skin does not enter compression. At the beginning of the cycles the perimeter 'crust' is neither in compression nor tension. If you stretch a body, and therefore put it into tension, and then release that tension, the bodydoes not automatically enter compression. It simply returns to its unstressed state, unless it has something to convert the strain energy of tension to, in which case can oscillate between tension and compression using the something as an intermediary between tension strain energy and compression strain energy. For example a mass on a spring converts the tension strain energy first to the kinetic energy of motion and then converts that to the strain energy of compression. So it oscillates about its mean unstrained, unstressed, position. To avoid the complication of gravity, that is how a loudspeaker works. Now in your model earth, internal forces stretch the perimeter into tension and then the tension is released by cracking. So there is no strain energy available to convert via an intermediary into compression. You may wish to say that the perimeter is now longer than before, by virtue of the material that has welled up into the cracks and solidified. This this longer perimeter would enter compression if it tried to shrink inwards. If you wish to consider the Earth as a sphere, pulsing in and out of its unstrained radius the very simplest formula for the period of oscillation is [math]T = 2\pi \sqrt {\frac{m}{k}} [/math] Where T is the period, m is the oscillating mass and k is the spring constant. You can estimate the mass of the crust, but what you would do about the spring constant, I don't know.
-
Three hints in the form of questions At what distance travelled does the block reach its final speed? In what direction do we take the velocity be for the purpose of the equations of motion? Velocity is a vector do we need to resolve it in any particular directions?
-
This is now the third time I have asked what you mean by a continuum (posts 94 and 108) Were they so impolite you did not deign to answer?
-
I asked the question because you made the claim that you can't be both observer and observed. But Reality is full to the brim with self referential systems Self replicating autonoma, positive feedback, autocatalysis, self fertile plants, to name but a few. So your claim that this must be so is defeated by counterexample.