studiot

Yes it does. Well done you have produced a different method than mine for both parts of this question, although I hope I have helped. +1

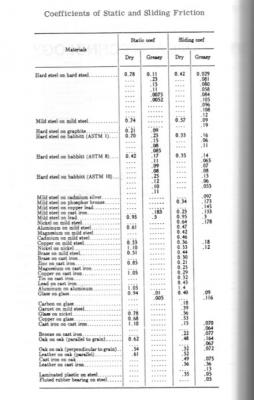
Really? Then I would say it is pretty good. This table from the International Handbook of Mathematical, Scientific and Engineering Formulas, Tables, Funtions, Graphs, Transforms (Fogiel) makes interesting reading There are also extensive tables of special conditions that can reduce some of these towards 0.1

Yes this is perfectly true and you have already used it (indirectly) in solving the first part of the question to get to the 50 days. The original fraction I wanted and why I said Now you found a and have to find b. Since A completes the task in 50 days If B completed the task in 1 day he would be 50 times as fast. If he completed it in 10 days he would be 5 times as fast. So B is a/b = 50/b times as fast as A Which of course means that if b =50 (it does not) the B is exactly as fast as a since 50/b = 50/50 = 1 This ratio or fraction is important because it connects B's contribution to A's contribution so that we can add them together as the next step.

If you can explain it better, please do, you are welcome. But please don't disrupt the thread.

Can we go we the idea that if C works twice as fast as A he takes half as long?

Surely it would take B 15 days to do 15 days work? This is not much better than your last comment, which I pretended didn't exist. Just bear with me and follow through what I have already said and we will get to the end.

Well I like this bit.

OOh, can I make a bid of $0.00 (disallowed) for one?

Looking at the end of your working surely [math]\int {\frac{{a + bx}}{{cx}}} dx[/math] = [math]\int {\frac{a}{{cx}}dx} [/math] + [math]\int {\frac{{bx}}{{cx}}} dx[/math] Where a, b and c are constants. is a very basic integral

Unfortunately I have to agree (and sympathise) with you. +1

With the greatest of respect, old bean, this displays your ignorance. And all ajb was trying to do was explain that relativity is about relative velocity, not 'velocity', funny how that word relative creeps in. FYI, 'linear' and 'vector' are synonymous as they refer to the same thing.

Let us do the second part of the question in two stages and it should become clear why they are working at different rates. Let us first consider the situation where m workers are working and all m workers work at the same rate. So if you like they are all clones or copies of each other. So if one worker is working then the task takes T days. If two workers are working the task takes T/2 days If three workers are working the task takes T/3 days If m workers are working the task takes T/m days. We can consider this as one worker worker working m times as fast. So one worker working m times as fast will complete in T/m days So if one worker works 1.5 times as fast m = 1.5 and will complete in T/1.5 days So days = T/m or m = T/days In our situation, T =50, days =15 so m =50/ 15 = 10/3 times as fast (note this is not a whole number don't worry it will become clear)

More or less exactly what I was considering saying +1

Yes and no. There is not "a (single) state of" time dilation, that implies too much (or perhaps too little?) We call the measurement made by an observer about his own time (his) "proper time". Any other observer in the universe would observe a dilated time if that second observer was in steady motion relative to the first. Since there are an infinite number of possible relative motions (all not greater than c) there are an infinite number of possible "states of dilatation". It does not actually need a real observer for this to be true, just the fact that there could be such an observer.

But A and B work at different rates so each accomplishes a different amount in 6 days or 50 days or 15 days. We have calculated that Mr A accomplishes the job on his own in 50 days. If Mr B worked at the same rate he would also finish in 50 days on his own so together they would finish in half that time ie 25 days. But we are told they finish faster in only 15 days so Mr B must work faster than Mr A. So I ask again how much faster does Mr B work than Mr A?

Yes correct. You are getting there, although I wanted you to say that A working alone takes 30 days to complete 9/15 of the total [math]\left( {\frac{9}{{15}}} \right)a = 30[/math] so [math]a = 50[/math] This was the point of my first question in post#2 We need to do some more work on this part to find b. Please use my 'a' and 'b' notation from post#2 as it will make the next bit easier. Now that we have found 'a', can you answer my second question in post#2?

Well yes that is what it comes down to, but you need to understand the answer to If it takes 15 days to complete the job what fraction of the job is complete after 6 days? and also What fraction of the job remains to be completed?

Unfortunately not. Neither the 1/9 nor the 1/6 is correct (do 1/9 + 1/6 make 1 ?) Edit divide the '1 whole' into days. How many days were there when the job was completed when they were both working together?

Better would be if you told me what fraction of the work remained when B fell ill.

This problem is about ratio, proportion or fractions - whatever you like to call them. You have nearly done the first bit, I will start you off with some notation Let 'a' be the number of days A takes to complete alone Let 'b' be the number of days B takes to complete alone So taking the information you have deduced from the problem can you think of any equation involving a fraction and 'a'? Also can you tell me what is the ratio of speeds at which A and B work?

Yes you could call it that.

That's exactly right! Edit : Did you want to discuss it further?

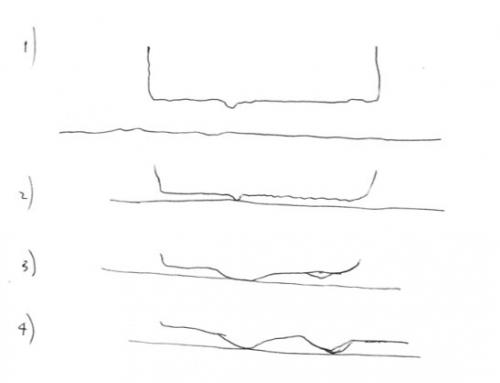
Hello again, Nicholas. Don't be sorry it is a perfectly good question. I have drawn some very quick pics to show what I mean. It is possible to develop this to a numerical theory that predicts friction coeffiients with reasonable accurcy for many metals. All surfaces are rough at some level of magnification. In (1) we are lowering a block, magnified until a bump shows. In (2) the block touches the table and the bump is the forst point to touch (there must be one) All the weight of the block is now resting on that one point, so it deforms as it cannot support such a load, as in (3) This continues untill a second (third, fourth etc) bump joins and the total surface area of the bumps is just sufficient to support the block as in (4)

After breaking my neck reading the scrolls you posted i thought that they first one gave you the equation and the second one gave you some figures to plug in. So what have you done so far and what do you not understand?

@jajrussel We are all agreed that for a relative speed of 60mph the difference is very slight. I have based my figures on the travellers measuring the distance across america as exactly 3000 miles and therefore measuring their transit time as 180000 seconds and their speed as 60mph. You have mentioned 'invariants'. Invariants means the same for all observers, not just the two in relative motion but others (eg in a helicopter flying above the vehicles) There is only one such invariant that is c the speed of light. However the 60 mph is the same for both the observers in the cars and those at the side of the road. Both observers subject to the same relative motion will observe the same speed (apart from the opposite signs) ie 60mph. So this is not exactly invariant but it is the same for both observers here. So swansont and JohnG will observe the transit time being a tad longer (as noted) and they will measure the distance across America as a tad longer than 3000 miles so that the figure of exactly 60mph is maintained. It is important to realise that both distance and time must change to maintain this equal value of relative velocity.