-
Posts
18258 -
Joined
-
Last visited
-
Days Won
104
Content Type
Profiles
Forums
Events
Everything posted by studiot
-
Classical thermodynamics requires a temperature difference for heat to flow. All curved motion can be constructed from two linear components in 2D or three in 3D.
-

How important is calculus for Comptuer Science?
studiot replied to aimforthehead's topic in Computer Science
Never forget that calculus was born out of discrete maths. The man (Newton) who invented calculus invented both and made great use of finite differences. Obviously many others have made great contributions since then. Oh and congratulations on your results, keep up the good work. -
Interesting video. But the effect is a surface effect (capillary waves) not a body effect so I don't see the NS equations as prominent. What keeps the drop together? I speculate that its size is important (for a given fluid density and surface tension coefficient)
-
Surface tension is a separate treatment from Navier Stokes. We combine them by introducing ST into the boundary conditions of the NS equations.
-
What I found interesting was that the article said the drop "bounced up and down" when it hit the liquid. That implies that, although of the same material as the liquid, it did not coalesce with it. The drop must have been in some way identifiable as a drop. I am still pondering the implications for the mechanics, in particular the surface tension.
-
I fear something important has been lost in the editing. But thank you for raising the subject.
-
Many folks misinterpret this statement and imagine it means that there is no shear stress in a fluid. This is far from the case. A solid can 'support' shear stresses without deformation, in which case it is totally rigid. Or it deflects a certain amount, but no more, under a given shear stress, in which case one of the responses -elastic, or some other applies. On the other hand The shear stress in a fluid at rest is zero. But the response of a fluid to imposed shear stress is movement. A fluid in motion suffers shear stress.
-
If you are going to invoke the Navier Stokes equations, please note they are plural. The other half of the pair to the version you have quoted is [math]\nabla .v = 0[/math] Please also note that the one you have used is for incompressible flow. However the other material in your post suggests you are continuing your investigation of airborne phenomena. You should therefore ensure that incompressible conditions apply in any such use of these NS equations, which sometimes happens in airflow, but not always. As to the apparent specific question The p terms refer to normal stresses, the T terms to tangential (shear) stresses.
-

Similarities between the theories of electromagnetism and gravity
studiot replied to kostik's topic in Classical Physics
If you want to study the comparison at undergraduate level I recommend the book by Professor Hammond of Southampton University Electromagnetism for Engineers. His treatment uses the similarities (and differences) between gravity and coulomb's law to introduce electromagnetism. It is very readable. You should look for the SI/Metric edition. -
I haven't seen the paper that swans refers to but he mentions the word 'model'. This idea is all to often forgotten or ignored. It is a philosophical question "Is mass a fundamntal property?". From the model point of view it can be regarded as simple a necessary constant in a model equation. As such there is more than one possible source of 'mass'. A rough rider's guide is that there is what we observe as the inertial mass model but there is also mass due to the (currrent) energy of the particle. A photon is allowed zero inertial mass, therefore any mass due to any other source eg motion is appreciable.
-
Well my first degree was applied maths and I am the first to admit I understand some areas of maths better than others and some not at all. It's a vast subject these days. Take heart.
-

Was the Law of Conservation of Energy ever proven for electricity?
studiot replied to Windevoid's topic in Speculations
I don't know what you mean by simple symmetric processes (or systems). Consider, for instance, the question "What is the kinetic energy of Mars?" Well to know the KE, you have to know the velocity and the mass. But someone on Earth, Jupiter, Alpha Centauri or some nearby black hole would all disagree on the velocity, although they might agree on the mass. So how can you tell if the KE is conserved if you don't even know what it is? I'm sure there are equivalent electrical questions. COE works well when we consider the change of energy from one form to another, which was why I mentioned processes. So you could ask Does COE hold for generating electrical energy from steam? or in the electrical heating of my bedroom? or perhaps can I transfer all the electrical energy from one battery to another? -

Was the Law of Conservation of Energy ever proven for electricity?
studiot replied to Windevoid's topic in Speculations
Well I think COE applies to processes not systems. -
Well I'd like to thank you for bringing something I'd never heard of to my attention. There really are some fascinating relationships in number theory.
-

Was the Law of Conservation of Energy ever proven for electricity?
studiot replied to Windevoid's topic in Speculations
As stated I find the question meaningless. What do you think the COE applies to and what sort of proof are you looking for? -
That is a perfectly reasonable question to wonder about. You need to distinguish between macroscopic and sub atomic effects. Macroscopic effect are due to the combined efforts of a very large number of particles and any network of forces or bonds joining them. Sub atomic effects are due (usually) to the interaction between a few (sub atomic) particles or even the interaction between one particle and its surroundings. The absorbtion and subsequent emission of photons is a sub atomic effect, called the photo-electric effect. Note that this occurs for a specific wavelength or a number of wavelengths, for example the sodium yellow spectrum lines. It does not occur for a broad range of frequencies as happens in reflection of light. Reflection is a macroscopic effect that is best not thought of in terms of quantum mechanics. Note I did not offer any structure to my reflective wall. This is usual for the physics of macroscopic quantites - we do not enquire into the fine structure. Note, however if you made a mirror of polished sodium, it would remove the yellow light and perhaps re emit it randomly, as you describe. Meanwhile it would reflect the rest of the wavelengths you shone onto it.
-
The Mathematical Description of Shape and Form Lord and Wilson pub Ellis Horwwod Geometry of Spatial Forms Gasson pub Ellis Horwood Computational Geometry for Design and Manufacture Faux and Pratt pub Ellis Horwood Elementary Linear Algebra Anton and Rorres pub Wiley
-

How do Physicists Consider Interpolation?
studiot replied to Iwonderaboutthings's topic in Classical Physics
Extrapolation means going beyond what you know to guess at what you don't know. This can lead to your guess being wildly wrong, as in the above. The Wave Function is in no sense an extrapolation. Interpolation means bracketing what you don't know between things you do know in the hope that your guess will be closer. It can also be very inaccurate, but it can yield useful, even good results as well. -
I'm glad to learn that you have solved the problem yourself, this is always the best way. I did observe that this problem has subleties - you need to invoke most of the principles of mechanics to justify the steps in the solution, so I would be interested to see your solution if you would care to post it.
-

How do Physicists Consider Interpolation?
studiot replied to Iwonderaboutthings's topic in Classical Physics
There are too many questions in the original post. As a start I suggest you get hold of the difference between interpolation and extrapolation and why the latter is a more risky method. Both methods are ways of obtaining the value of some function at points you have not measured or do not have tabulated data for and cannot calculate exactly. -
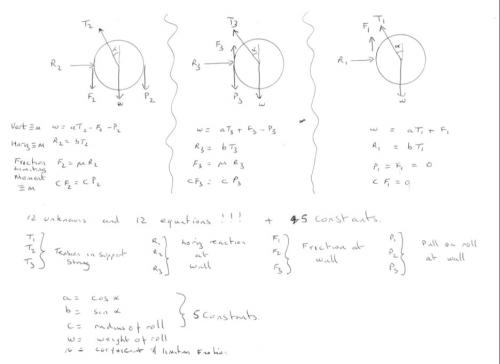
Thank you for replying. You haven't got your mechanics quite right. The above is true but the only because it determines the tension in the string, which in turn determines the horizontal reaction from the wall. Nor does it negate what I said since I did say moment about the centre of the roll, not some other point where there are undoubtedly different moments.The trick in mechanics is to take your moments about points where as many forces as possible pass through and therefore have zero moment. This question is actually quite subtle. There are three situations: 1) With the roll just hanging there and no pull on the paper. 2) With the roll hanging and a pull on the side opposite the wall. 3) With the rolll hanging, and a pull on the side by the wall. Since the tension is not necessarily the same in each case that yields 9 equations in 12 unknowns. There is no deformation so we cannot use compatibility Stress is not considered so we cannot use elasticity However we can consider the situation at limiting friction which yields a further 3 equations, bringing the total up to 12 - a happy situation since we now have enough to solve the question. Here is the full set of 12. Note I have replace sin and cos by constants a and b since the geometry does not change so we can more easily see the set of equations is linear and and manipulate them. What remains is a page of further manipulation, first substitution then setting up and manipulating an inequality to achieve the desired result. I will leave that part to bon if (s)he is still interested.