Woxor

Unfortunately, that's no good since I need to work with the function ([math]\phi^{n}[/math]) analytically -- numerical values aren't too hard to obtain. As for the initial value: it seems as though [math]a_{0}=0[/math] would suit my purposes fine, so there's not necessarily a buried variable. As for the divergence, there's nothing vital about the base -- [math]a_{n+1}=e^{\frac{a_{n}}{e}}[/math] would be just as enlightening. (Interestingly, [math]e^{\frac{1}{e}}[/math] seems to be the highest base value for which this converges.) But yeah, I am certainly worried as to whether it's even possible. I haven't taken any classes specifically on recurrence relations, and I haven't worked much with the Reimann zeta function, so I was just wondering if anyone out there actually happens to know one way or the other.

Regarding CMBR: I was under the impression that it did pass "us" (or what would eventually form the solar system and its inhabitants) at the beginning, and is being pulled back inward by universal gravitation.

Those values are correct, but I'm looking for something more or less like this: [math]a_{n}=a_{0}*f(n)[/math], except with an actual, explicit function. EDIT: Also, I realize that "[math]a_{n}=\phi^{n}(a_{0})[/math] where [math]\phi(x)=e^{x}[/math]" is the solution, but I need something even more explicit than that. In other words, what is [math]\phi^{n}[/math] in terms of [math]n[/math]?

I'm assuming this is the proper forum to post this in, even though I'm asking rather than testing whether anyone knows the answer to this (because I don't). Does anyone know how to solve [math]a_{n+1}=e^{a_{n}}[/math] explicitly for [math]a_{n}[/math] in terms of [math]n[/math] and [math]a_{0}[/math]? I would greatly appreciate knowing both the solution and the method used to obtain it, since my own studies have taken me where a solution would be extremely helpful. I haven't been able to determine whether this is a simple textbook equation or a problem entirely unsolved by the mathematical community.

I should probably know how to do that, but I seem to have no idea. My thermo test next week seems more intimidating now.

I'll certainly grant that it's interesting, though like I said I do think it's possible to derive some experiment to approximate any given number. It does certainly agitate the old curiosity about why e and pi behave like they do and why they have the particular, seemingly-arbitrary values they have.

You could probably figure out a way to estimate any number this way -- I don't think pi or e or even the irrationals are unique in this respect. Also, Buffon's needle requires something like [math]10^{8}[/math] trials to yield a reasonable approximation of pi to 4 decimal places -- not very practical.

Interesting idea. Highly unlikely -- Ockham's Razor and all that.

I don't know if it still matters, but I have been trying to find Antoine coefficients for bromine, but I can't seem to do so. Perhaps it's no good describing bromine with that equation, or something. I have no idea whether I'm talking down to you by explaining, but to be safe rather than sorry: the Antoine equation gives the log of the vapor pressure (i.e. the pressure when liquid and gas are present) of a given substance as a function of the temperature. Specifically, log(P*) = A - B/(T+C), where A, B, and C are constants for the substance. I can't find A, B, and C for bromine anywhere on the web or in my textbooks, but if you can find them, they might help in theory. I also don't know how much this equation will help, given that it only applies when both phases are present, i.e. it doesn't predict the point at which vaporization occurs, since, as you say, the event depends on the pressure as well. In sum, I may be entirely useless. But good luck.

You are right; I retract my statement that the finitude of stars is the main reason. I don't know why the finitude of light speed and history didn't occur to me as the best reason. However, the argument (that there are finitely many stars) is still a good one in countering the original idea (that the sky should be extremely bright), for the simple reason that your first sentence (after "No.") is false. Age and time, no matter how infinite, do not produce radiation. If you want infinite light bombardment, you probably need an infinite source; in other words, a finite number of stars will not produce infinite radiation, no matter how much time and space you give them. Similarly, the other argument (that stars are not evenly distributed across the sky, and that not all light radiated in our direction actually makes it here) is also a good counter. And just to be mind-bogglingly picky: why say "at night"? Wouldn't the light from an infinite number of stars probably overcome the intensity of the sun?

I think this is a point where it is helpful to think in terms of light consisting of particles rather than waves (though it is not necessarily one or the other). Light is radiation emitted by the star' date=' and one can imagine that this radiation comes in tiny packets (called "photons") which, in some respects, behave just like other particles. They are ejected from the star and travel away from it at the speed of light. When the star dies, it stops emitting the photons, but that doesn't change the fact that it already emitted a bunch of them already. So, the light that has already started doesn't "go out," it simply continues on its way. The reason it appears to us on Earth that light "goes out" after the source is shut off is that light travels so fast that we can't tell the difference between a) the light source shutting off, and b) the "existing" light traveling to wherever it ends up. The density of space is something on the order of one molecule per cubic meter. To give you an idea how little that is, the density of air at sea level (which light passes through easily enough) is about 25,000,000,000,000,000,000,000,000 molecules per cubic meter. In order to keep the light from reaching us, it has to change directions or be absorbed. The former can be accomplished by forces like gravity and magnetism, but the most dramatic cause of both is collision with things like atoms. Since there are so few atoms in open space, light is free to pass through almost indefinitely. As for your second question, yes, the light would remain even after the torch is switched off. If the mirrors were ideal (reflecting 100% of the light), the light would probably bounce around forever, although any attempt to "see" the light would probably have to involve absorbing it (like you do with your eyes). As has been said, though, the light would collide with the mirrors so many times (because c is so fast) that even a 99.99% reflecting mirror would soon absorb it all. That's not entirely fair; the second two reasons given are very good. The stars are grouped into galaxies (and the light that comes from stars doesn't always make it around other objects, due both to actual collision and to gravity), making it very difficult to argue that they would light up the entire sky, even if the other problems were circumvented. Also, the fact that there are finitely many stars is something I would consider to be the main reason the entire sky is not glowing.

I'm taking an astronomy course right now, so I don't know precisely yet. But here's my attempt: 1. I don't think there's anything "making" them spin, per se, but they spin simply because they have angular momentum, and they have angular momentum simply because the constituents that formed the galaxy had it going in. I could definitely be wrong about this one, though. 2. Because matter tends to coalesce due to the law of gravitation (for large scales such as this). Also, since we're all rapidly moving away from the center of the universe, we end up with more and more space between galaxies simply because of geometry. (In other words, if you have finitely many galaxies on the surface of an expanding sphere, the intergalactic distance is going to increase.) 3. Almost. Google says the universe is 13.7 +/- 0.2 billion years old, and that the galaxy is 13.6 billion. You can easily reason that the galaxies are not quite as old, since (very) shortly after the big bang, there weren't even atoms, let alone stars, let alone galaxies. But by an astronomic scale, galaxies apparently formed pretty quickly. 4. Not from what I've heard. Supposedly, the universe will end with "heat death," which will be the passage into such an entropic state that everything, including galaxies, stars, planets, molecules, and perhaps even atoms will break apart and all that will be left is dust. I would imagine that the dust could still coalesce, but I don't know if you'd still call it a galaxy. But all that's just something I read somewhere, it could easily be a layman's (i.e. my) misinterpretation.

That might have been a trick proof (i.e. one that's not really a proof, but a clever argument that looks like a proof), or else there is something about "being the same" that I'm not getting. A negative number will never equal a positive number. A negative number and a positive number can have the same absolute value, but beyond that I don't know what to make of the word "same."

They exist as much as any number can be said to "exist," I imagine. I think it's misleading to speak of a number "existing" in reality. I take a positivist stance: negative numbers are helpful in describing things we observe and things we do. So are imaginary numbers, infinitessimals, and infinity. I've never seen an imaginary number or a negative one, but I've never seen a positive number, either.

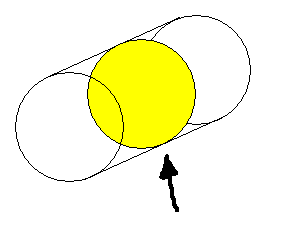
Assuming you're not supposed to take the cross-section "long-ways" (i.e. splitting it down the middle like string cheese), the cross-sectional area is the area of a slice through the object. Since I can't think of a better way to describe it, the yellow circle in this picture is a cross-section. The area of the circle is the cross-sectional area.