Orion1

Affirmative, revision complete. First order metric tensor field for spin-0 and spin-1 particles: (ref. 1, pg. 21, eq. 1.68) [math]T^{'\mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] Second order metric tensor field for spin-2 particles: (ref. 3) [math]M^{'\mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} M^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] The graviton is a spin-two particle, as opposed to the spin-one photon, so that the interaction forms are somewhat more complex, involving symmetric and traceless second order tensors rather than simple Lorentz four-vectors. [math]\;[/math] In relativistic mechanics, the Center Of Mass-Energy boost and orbital 3-space angular momentum of a rotating object are combined into a four-dimensional bivector in terms of the four-position X and the four-momentum P of the object. (ref. 3) [math]\mathbf{M} = \mathbf{X} \wedge \mathbf{P}[/math] [math]\;[/math] With matrix components: [math]M^{\alpha \beta} = X^{\alpha} P^{\beta} - X^{\beta} P^{\alpha}[/math] [math]\;[/math] Which are six independent quantities altogether. Since the components of X and P are frame-dependent, so is M. Three components [math]M^{ij} = x^{i} p^{j} - x^{j} p^{i} = L^{ij}[/math] [math]\;[/math] are those of the familiar classical 3-space orbital angular momentum, and the other three components [math]M^{0i} = x^{0} p^{i} - x^{i} p^{0} = c \; \left(tp^{i} - x^{i}{\frac{E}{c^{2}}} \right) = -cN^{i}[/math] are the relativistic mass moment, multiplied by -c. The tensor is antisymmetric: [math]M^{\alpha \beta} = -M^{\beta \alpha}[/math] [math]\;[/math] The components of the tensor can be systematically displayed as a matrix. [math]\;[/math] The angular momentum [math]L = x \; \wedge \; p[/math] of a particle with relativistic mass m and relativistic momentum p, as measured by an observer in a lab frame, combines with another vector quantity dynamic mass-energy moment [math]N = mx - pt[/math] in the relativistic angular momentum tensor: (ref. 2) [math]M^{\alpha \beta} = {\begin{pmatrix} 0 & -N_{x}^{1}c & -N_{y}^{2}c & -N_{z}^{3}c \\ N_{x}^{1}c & 0 & L^{12} & -L^{13} \\ N_{y}^{2}c & -L^{21} & 0 & L^{23} \\ N_{z}^{3}c & L^{31} & -L^{32} & 0 \end{pmatrix}}[/math] [math]\;[/math] In classical mechanics, the orbital angular momentum of a particle with instantaneous three-dimensional position vector [math]x = (x, y, z)[/math] and momentum vector [math]p = (px, py, pz)[/math], is defined as the axial vector: (ref. 4) [math]\mathbf{L} = \mathbf{x} \times \mathbf{p}[/math] [math]\;[/math] Which has three components, that are systematically given by cyclic permutations of Cartesian directions, change x to y, y to z, z to x, repeat. [math]L_{x} = yp_{z} - zp_{y}[/math] [math]L_{y} = zp_{x} - xp_{z}[/math] [math]L_{z} = xp_{y} - yp_{x}[/math] [math]\;[/math] A related definition is to conceive orbital angular momentum as a plane element. This can be achieved by replacing the cross product by the exterior product in the language of exterior algebra, and angular momentum becomes a contravariant second order antisymmetric tensor: [math]\mathbf{L} = \mathbf{x} \wedge \mathbf{p}[/math] [math]\;[/math] or writing [math]x = (x_{1}, x_{2}, x_{3}) = (x, y, z)[/math] and momentum vector [math]p = (p_{1}, p_{2}, p_{3}) = (p_{x}, p_{y}, p_{z})[/math], the components can be compactly abbreviated in tensor index notation: [math]L^{ij} = x^{i} p^{j} - x^{j} p^{i}[/math] [math]\;[/math] Where the indices i and j take the values 1, 2, 3. On the other hand, the components can be systematically displayed fully in a 3 x 3 antisymmetric matrix: [math]\mathbf{L} = {\begin{pmatrix} L^{11} & L^{12} & L^{13} \\ L^{21} & L^{22} & L^{23} \\ L^{31} & L^{32} & L^{33} \\ \end{pmatrix}} = {\begin{pmatrix} 0 & L_{xy} & L_{xz} \\ L_{yx} & 0 & L_{yz} \\ L_{zx} & L_{zy} & 0 \end{pmatrix}} = \begin{pmatrix} 0 & L_{xy} & -L_{zx} \\ -L_{xy} & 0 & L_{yz} \\ L_{zx} & -L_{yz} & 0 \end{pmatrix}[/math] [math]\;[/math] [math]\mathbf{L} = {\begin{pmatrix} 0 & xp_{y} - yp_{x} & -\left(zp_{x} - xp_{z} \right) \\ -\left(xp_{y} - yp_{x} \right) & 0 & yp_{z} - zp_{y} \\ zp_{x} - xp_{z} & -\left(yp_{z} - zp_{y} \right) & 0 \end{pmatrix}}[/math] [math]\;[/math] This quantity is additive, and for an isolated system, the total angular momentum of a system is conserved. [math]\;[/math] Dynamic mass-energy moment: [math]\mathbf{N} = m \mathbf{x} - \mathbf{p} t = \frac{E}{c^{2}} \mathbf{x} - \mathbf{p} t = \gamma (\mathbf{u}) m_{0} (\mathbf{x} - \mathbf{u} t)[/math] [math]\;[/math] Expressing N in terms of relativistic mass-energy and momentum, rather than rest mass and velocity, avoids extra Lorentz factors. However, relativistic mass is discouraged by some authors since it can be a misleading quantity to apply in certain equations. [math]\;[/math] Defined here so that the relativistic equation in terms of the relativistic mass-energy equivalence, and classical definition, have the same form. The Cartesian components are: [math]N_{x} = mx - p_{x} t = \frac{E}{c^{2}} x - p_{x} t = \gamma \left(u \right) m_{0}\left(x - u_{x} t \right)[/math] [math]N_{y} = my - p_{y} t = \frac{E}{c^{2}} y - p_{y} t = \gamma \left(u \right) m_{0}\left(y - u_{y} t \right)[/math] [math]N_{z} = mz - p_{z} t = \frac{E}{c^{2}} z - p_{z} t = \gamma \left(u \right) m_{0}\left(z - u_{z} t \right)[/math] [math]\;[/math] For a massless spin-2 graviton, [math]E = pc[/math], and the relativistic angular momentum tensor is: [math]M^{\alpha \beta} = {\begin{pmatrix} 0 & -p_{x}\left(\frac{x}{c} - t \right) & -p_{y}\left(\frac{y}{c} - t \right) & -p_{z}\left(\frac{z}{c} - t \right) \\ p_{x}\left(\frac{x}{c} - t \right) & 0 & xp_{y} - yp_{x} & -\left(zp_{x} - xp_{z} \right) \\ p_{y}\left(\frac{y}{c} - t \right) & -\left(xp_{y} - yp_{x} \right) & 0 & yp_{z} - zp_{y} \\ p_{z}\left(\frac{z}{c} - t \right) & zp_{x} - xp_{z} & -\left(yp_{z} - zp_{y} \right) & 0 \end{pmatrix}}[/math] [math]\;[/math] In spherical coordinates [math](ct, r, \theta, \phi)[/math] the Minkowski flat spacetime metric in contravariant form: [math]ds^{2} = -c^{2} dt^{2} + dr^{2} + r^{2} d\theta^{2} + r^{2} \sin^{2} \theta \; d\phi^{2}[/math] [math]\;[/math] In spherical coordinates [math](ct, r, \theta, \phi)[/math] the relativistic angular momentum tensor in contravariant form: [math]M^{\alpha \beta} = {\begin{pmatrix} 0 & -p_{x}\left(\frac{dr}{c} - t \right) & -p_{y}\left(\frac{r d\phi}{c} - t \right) & -p_{z}\left(\frac{r \sin \theta d\phi}{c} - t \right) \\ p_{x}\left(\frac{dr}{c} - t \right) & 0 & dr p_{y} - r d\theta p_{x} & -\left(r \sin \theta d\phi p_{x} - dr p_{z} \right) \\ p_{y}\left(\frac{r d\theta}{c} - t \right) & -\left(dr p_{y} - r d\theta p_{x} \right) & 0 & r d\theta p_{z} - r \sin \theta d\phi p_{y} \\ p_{z}\left(\frac{r \sin \theta d\phi}{c} - t \right) & r \sin \theta d\phi p_{x} - dr p_{z} & -\left(r d\theta p_{z} - r \sin \theta d\phi p_{y} \right) & 0 \end{pmatrix}}[/math] [math]\;[/math] General Relativity stress-energy tensor: [math]M_{\mu \nu} = \pm \left(\begin{matrix} -\rho\left(r \right) c^{2} & 0 & 0 & 0 \\ 0 & p\left(r \right) & 0 & 0 \\ 0 & 0 & p\left(r \right) & 0 \\ 0 & 0 & 0 & p\left(r \right) \end{matrix} \right)[/math] [math]\;[/math] Jacobian matrix transformation matrices: (ref. 5, pg. 15, eq. 41, para. 1, ref 6) [math]\Lambda^{\mu}_{\alpha} = \frac{\partial \xi^{\mu} }{\partial x^{\alpha}} \; \; \; \; \; \; \Lambda^{\nu}_{\beta} = \frac{\partial \xi^{\nu}}{\partial x^{\beta}}[/math] [math]\;[/math] [math]x^{\alpha} = \left(ct, r, \theta, \phi \right) \; \; \; \; \; \; x^{\beta} = \left(ct, r, \theta, \phi \right)[/math] [math]\;[/math] [math]\Lambda^{\mu}_{\alpha} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{c^{2} \partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}} = {\begin{bmatrix} -2 dt & 0 & 0 & 0 \\ 0 & 2 dr & 0 & 0 \\ 0 & 0 & 2r^2 d\theta & 0 \\ 0 & 0 & 0 & 2r^2 \sin^{2} \theta \; d\phi \\ \end{bmatrix}}[/math] [math]\;[/math] [math]\;[/math] [math]\Lambda^{\nu}_{\beta} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{c^{2} \partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}} = {\begin{bmatrix} -2 dt & 0 & 0 & 0 \\ 0 & 2 dr & 0 & 0 \\ 0 & 0 & 2r^2 d\theta & 0 \\ 0 & 0 & 0 & 2r^2 \sin^{2} \theta \; d\phi \\ \end{bmatrix}}[/math] [math]\;[/math] The matrix dot product transformation matrices in covariant form for each of the two four-momentum components as seen from two reference frames, S and S' prime: [math]\Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} = {\begin{bmatrix} 4 dt dt' & 0 & 0 & 0 \\ 0 & 4 dr dr' & 0 & 0 \\ 0 & 0 & 4r^2 r'^{2} d\theta \; d\theta' & 0 \\ 0 & 0 & 0 & 4r^2 r'^{2} \sin^{2} \theta \sin^{2} \theta' \; d\phi \; d\phi' \\ \end{bmatrix}}[/math] [math]\;[/math] Newton's constant: (ref. 7, pg. 9, eq. 37) [math]\kappa^{2} = 32 \pi G[/math] [math]\;[/math] General relativity weak field limit spacetime metric: (ref. 7, pg. 9, eq. 37) [math]g_{\mu \nu} = \eta_{\mu \nu} + \kappa h_{\mu \nu}[/math] [math]\;[/math] General Relativity weak field limit spacetime metric and Planck quantum gravity identity 6: [math]\boxed{\frac{8 \pi G}{c^{4}} M_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} M^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - \kappa h^{\mu \nu} \right)\left(\eta_{\mu \nu} + \kappa h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] Tensor matrix solution key: [math]s\left(\mu, \nu, \alpha, \beta \right)[/math] [math]\;[/math] [math]s\left(1, 1, 1, 2 \right):[/math] [math]\boxed{2 \pi G p\left(r \right) = c^{4}\left(dr' dr^{2} p_{y} - dr dr' r d\theta p_{x} \right)}[/math] [math]\;[/math] [math]s\left(2, 2, 2, 3 \right):[/math] [math]\boxed{2 \pi G p\left(r \right) = c^{4} \left(r^{3} r'^{2} d\theta^{2} d\theta' p_{z} - r^{3} r'^{2} \sin \theta d\theta d\theta' d\phi p_{y} \right)}[/math] [math]\;[/math] With four stress-energy tensor elements and twelve relativistic angular momentum tensor elements, there are forty-eight possible solution keys. [math]\;[/math] Are these energy-momentum tensors compatible with a massless spin-2 graviton? [math]\;[/math] Is this approach mathematically and symbolically correct to this point? [math]\;[/math] Are there any other tensor matrix solution keys that you want to examine? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf Wikipedia - Four-tensor - Second order tensors: (ref. 2) https://en.wikipedia.org/wiki/Four-tensor#Second_order_tensors Wikipedia - Relativistic angular momentum - 4d Angular momentum as a bivector: (ref. 3) https://en.wikipedia.org/wiki/Relativistic_angular_momentum#4d_Angular_momentum_as_a_bivector Wikipedia - Relativistic angular momentum - Orbital 3d angular momentum: (ref. 4) https://en.wikipedia.org/wiki/Relativistic_angular_momentum#Orbital_3d_angular_momentum Introduction to Tensor Calculus for General Relativity - Edmund Bertschinger: (ref. 5) https://web.mit.edu/edbert/GR/gr1.pdf Wikipedia - Jacobian matrix: (ref. 6) https://en.wikipedia.org/wiki/Jacobian_matrix_and_determinant#Example_3:_spherical-Cartesian_transformation Barry R. Holstein - Department of Physics-LGRT - University of Massachusetts: (ref. 7) https://arxiv.org/pdf/gr-qc/0607045.pdf

Affirmative, revision complete. Metric tensor field: (ref. 1, pg. 21, eq. 1.68) [math]T^{'\mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] In geometry, the line element or length element can be informally thought of as a line segment associated with an infinitesimal displacement vector in a metric space. The length of the line element, which may be thought of as a differential arc length, is a function of the metric tensor. [math]\;[/math] General Relativity line element where spacetime is modelled as a curved Pseudo-Riemannian manifold with an appropriate metric tensor: (ref. 3, ref 4, pg. 15, eq. 41, para. 1) [math]ds^2 = g_{\mu \nu} dx^{\mu} dx^{\nu}[/math] [math]\;[/math] The General Relativity line element with a curved Pseudo-Riemannian manifold metric tensor condition imposes constraints on the coefficients [math]\Lambda^{\mu}_{\nu}[/math] (ref. 3, pg. 1, eq. 3) [math]g_{\mu \nu} = g_{\alpha \beta} \Lambda^{\alpha}_{\mu} \Lambda^{\beta}_{\nu}[/math] [math]\;[/math] General Relativity curved Pseudo-Riemannian manifold line element identity: [math]\boxed{ds^2 = g_{\alpha \beta} \Lambda^{\alpha}_{\mu} \Lambda^{\beta}_{\nu} dx^{\mu} dx^{\nu}}[/math] [math]\;[/math] The [math]g_{\alpha \beta}[/math] metric tensor will vary according to the spacetime being modeled. It can have either or both the covariant and contravariant terms accordingly to the Einstein summation convention. In this form it is specifying covariant. [math]g_{\alpha \beta} = \pm \begin{pmatrix} -\xi^0 & 0 & 0 & 0 \\ 0 & \xi^1 & 0 & 0 \\ 0 & 0 & \xi^2 & 0 \\ 0 & 0 & 0 & \xi^3 \end{pmatrix}[/math] [math]\;[/math] In spherical coordinates [math](ct, r, \theta, \phi)[/math] the Minkowski flat spacetime metric takes the form: [math]ds^{2} = -c^{2} dt^{2} + dr^{2} + r^{2} d\theta^{2} + r^{2} \sin^{2} \theta \; d\phi^{2}[/math] [math]\;[/math] The Minkowski flat spacetime metric in covariant form: [math]ds \; ds' = -c^{2} dt dt' + dr dr' + r r' d\theta \; d\theta' + r r' \sin \theta \sin \theta' \; d\phi \; d\phi'[/math] [math]\;[/math] General relativity stress-energy tensor: [math]T_{\mu \nu} = \pm \left(\begin{matrix} -\rho c^{2} & 0 & 0 & 0 \\ 0 & p & 0 & 0 \\ 0 & 0 & p & 0 \\ 0 & 0 & 0 & p \end{matrix} \right)[/math] [math]\;[/math] General Relativity Minkowski flat spacetime metric tensor in covariant form: [math]T^{\alpha \beta} \left(r \right) = \pm \begin{pmatrix} -c^{2} dt dt' & 0 & 0 & 0 \\ 0 & dr dr' & 0 & 0 \\ 0 & 0 & r r' d\theta \; d\theta' & 0 \\ 0 & 0 & 0 & r r' \sin \theta \sin \theta' \; d\phi \; d\phi' \end{pmatrix}[/math] [math]\;[/math] Jacobian matrix transformation matrices: (ref. 4, ref 5, pg. 15, eq. 41, para. 1) [math]\Lambda^{\mu}_{\alpha} = \frac{\partial \xi^{\mu} }{\partial x^{\alpha}} \; \; \; \; \; \; \Lambda^{\nu}_{\beta} = \frac{\partial \xi^{\nu}}{\partial x^{\beta}}[/math] [math]\;[/math] [math]x^{\alpha} = \left(ct, r, \theta, \phi \right) \; \; \; \; \; \; x^{\beta} = \left(ct, r, \theta, \phi \right)[/math] [math]\;[/math] [math]\Lambda^{\mu}_{\alpha} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{c^{2} \partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}} = {\begin{bmatrix} -2 dt & 0 & 0 & 0 \\ 0 & 2 dr & 0 & 0 \\ 0 & 0 & 2r^2 d\theta & 0 \\ 0 & 0 & 0 & 2r^2 \sin^{2} \theta \; d\phi \\ \end{bmatrix}}[/math] [math]\;[/math] [math]\;[/math] [math]\Lambda^{\nu}_{\beta} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{c^{2} \partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}} = {\begin{bmatrix} -2 dt & 0 & 0 & 0 \\ 0 & 2 dr & 0 & 0 \\ 0 & 0 & 2r^2 d\theta & 0 \\ 0 & 0 & 0 & 2r^2 \sin^{2} \theta \; d\phi \\ \end{bmatrix}}[/math] [math]\;[/math] The matrix dot product transformation matrices in covariant form for each of the two four-momentum components as seen from two reference frames, S and S' prime: [math]\Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} = {\begin{bmatrix} 4 dt dt' & 0 & 0 & 0 \\ 0 & 4 dr dr' & 0 & 0 \\ 0 & 0 & 4r^2 r'^{2} d\theta \; d\theta' & 0 \\ 0 & 0 & 0 & 4r^2 r'^{2} \sin^{2} \theta \sin^{2} \theta' \; d\phi \; d\phi' \\ \end{bmatrix}}[/math] [math]\;[/math] General Relativity weak field limit spacetime metric and Planck quantum gravity identity 4: (ref. 6) [math]\boxed{\frac{8 \pi G}{c^{4}} T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - h^{\mu \nu} \right)\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] Solution 1: [math]\mu = \nu = 0[/math] [math]\boxed{2 \pi G \rho \left(r \right) = c^{4} dt^{2} dt'^{2}}[/math] [math]\;[/math] Solution 2: [math]\mu = \nu = 1[/math] [math]\boxed{2 \pi G p \left(r \right) = c^{4} dr^{2} dr'^{2}}[/math] [math]\;[/math] Solution 3: [math]\mu = \nu = 2[/math] [math]\boxed{2 \pi G p \left(r \right) = c^{4} dr^{3} dr'^{3} d\theta^{2} \; d\theta'^{2}}[/math] [math]\;[/math] Solution 4: [math]\mu = \nu = 3[/math] [math]\boxed{2 \pi G p \left(r \right) = c^{4} dr^{3} dr'^{3} \sin^{3} \theta \; \sin^{3} \theta' d\phi^{2} \; d\phi'^{2}}[/math] [math]\;[/math] Is this approach mathematically and symbolically correct to this point? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf Wikipedia - General relativity - Metric tensor - Local coordinates and matrix representations: (ref. 2) https://en.wikipedia.org/wiki/Metric_tensor_(general_relativity)#Local_coordinates_and_matrix_representations Lorentz Transformations - Bernard Durney: (ref. 3) https://arxiv.org/pdf/1103.0156.pdf Introduction to Tensor Calculus for General Relativity - Edmund Bertschinger: (ref. 4) https://web.mit.edu/edbert/GR/gr1.pdf Wikipedia - Jacobian matrix: (ref. 5) https://en.wikipedia.org/wiki/Jacobian_matrix_and_determinant#Example_3:_spherical-Cartesian_transformation Science Forums - Lagrangian equation for a massless Planck graviton - Orion1: (ref. 6) https://www.scienceforums.net/topic/117992-the-lagrangian-equation/?do=findComment&comment=1096894

Affirmative, revision complete. [math]\eta_{\mu \nu}[/math] - perturbed non-dynamical background metric [math]\;[/math] General Relativity Minkowski flat spacetime metric: (ref. 1) [math]\eta_{\mu \nu} = \pm \begin{pmatrix} -1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{pmatrix}[/math] [math]\;[/math] General Relativity Minkowski flat spacetime metric is equivalent to the inverse metric: (ref.1, ref. 2) [math]\boxed{\eta_{\mu \nu} = \eta^{\mu \nu}}[/math] [math]\;[/math] What is the formal mathematical definition for [math]g_{\alpha \beta}[/math]? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Four-gradient As a Jacobian matrix for the SR Minkowski metric tensor: (ref. 1) https://en.wikipedia.org/wiki/Four-gradient#As_a_Jacobian_matrix_for_the_SR_Minkowski_metric_tensor Wikipeda - Lorentz covariance: (ref. 2) https://en.wikipedia.org/wiki/Lorentz_covariance

Affirmative, revision complete. Metric tensor field: (ref. 1, pg. 21, eq. 1.68) [math]T^{'\mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] In geometry, the line element or length element can be informally thought of as a line segment associated with an infinitesimal displacement vector in a metric space. The length of the line element, which may be thought of as a differential arc length, is a function of the metric tensor. [math]\;[/math] General Relativity line element where spacetime is modelled as a curved Pseudo-Riemannian manifold with an appropriate metric tensor: (ref. 3, ref 4, pg. 15, eq. 41, para. 1) [math]ds^2 = g_{\mu \nu} dx^{\mu} dx^{\nu}[/math] [math]\;[/math] The General Relativity line element with a curved Pseudo-Riemannian manifold metric tensor condition imposes constraints on the coefficients [math]\Lambda^{\mu}_{\nu}[/math]: (ref. 3, pg. 1, eq. 3) [math]g_{\mu \nu} = g_{\alpha \beta} \Lambda^{\alpha}_{\mu} \Lambda^{\beta}_{\nu}[/math] [math]\;[/math] General Relativity curved Pseudo-Riemannian manifold line element identity: [math]\boxed{ds^2 = g_{\alpha \beta} \Lambda^{\alpha}_{\mu} \Lambda^{\beta}_{\nu} dx^{\mu} dx^{\nu}}[/math] [math]\;[/math] General Relativity matrix symmetry expression: [math]\mu \cdot \nu = \nu \cdot \mu = \eta[/math] [math]\;[/math] Jacobian matrix transformation matrices: (ref. 4, ref 5, pg. 15, eq. 41, para. 1) [math]\Lambda^{\mu}_{\alpha} = \frac{\partial \xi^{\mu} }{\partial x^{\alpha}} \; \; \; \; \; \; \Lambda^{\nu}_{\beta} = \frac{\partial \xi^{\nu}}{\partial x^{\beta}}[/math] [math]\;[/math] [math]x^{\alpha} = \left(ct, r, \theta, \phi \right) \; \; \; \; \; \; x^{\beta} = \left(ct, r, \theta, \phi \right)[/math] [math]\;[/math] [math]\Lambda^{\mu}_{\alpha} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{\partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}}[/math] [math]\;[/math] [math]\;[/math] [math]\Lambda^{\nu}_{\beta} \left(ct, r, \theta, \phi \right) = {\begin{bmatrix} \dfrac{\partial \xi^0}{\partial t^0} & 0 & 0 & 0 \\ 0 & \dfrac{\partial \xi^1}{\partial r^1} & 0 & 0 \\ 0 & 0 & \dfrac{\partial \xi^2}{\partial \theta^2} & 0 \\ 0 & 0 & 0 & \dfrac{\partial \xi^3}{\partial \phi^3} \\ \end{bmatrix}}[/math] [math]\;[/math] Is this approach mathematically and symbolically correct to this point? [math]\;[/math] What is the formal mathematical definition for [math]g_{\alpha \beta}[/math]? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf Wikipedia - General relativity - Metric tensor - Local coordinates and matrix representations: (ref. 2) https://en.wikipedia.org/wiki/Metric_tensor_(general_relativity)#Local_coordinates_and_matrix_representations Lorentz Transformations - Bernard Durney: (ref. 3) https://arxiv.org/pdf/1103.0156.pdf Introduction to Tensor Calculus for General Relativity - Edmund Bertschinger: (ref. 4) https://web.mit.edu/edbert/GR/gr1.pdf Wikipedia - Jacobian matrix: (ref. 5) https://en.wikipedia.org/wiki/Jacobian_matrix_and_determinant#Example_3:_spherical-Cartesian_transformation

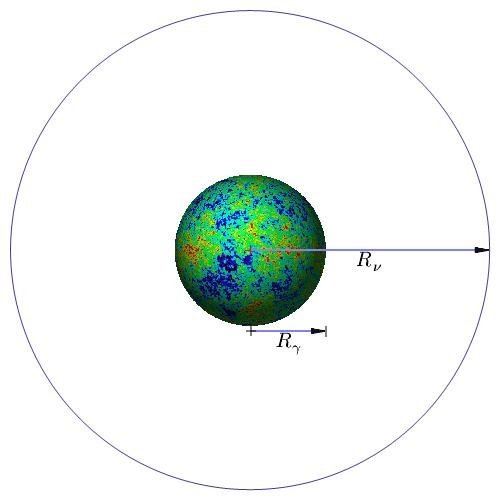
Affirmative, revision complete. WMAP satellite cosmological composition parameters at photon decoupling time: (ref. 1) [math]\Omega_{\gamma,t} = 0.15[/math] [math]\;[/math] Photon species total effective degeneracy number: [math]\boxed{N_{\gamma} = 2}[/math] [math]\;[/math] Solar surface temperature: (ref. 2) [math]T_{\odot} = 5772 \; \text{K}[/math] [math]\;[/math] Total solar radius: (ref. 2) [math]R_{\odot} = 6.963 \cdot 10^{5} \; \text{km}[/math] [math]\;[/math] Earth orbital radius semi-major axis: (ref. 3) [math]r_{\oplus} = 1.496 \cdot 10^{8} \; \text{km}[/math] [math]\;[/math] Earth albedo reflectivity: (ref. 3) [math]\alpha_{\oplus} = 0.367[/math] [math]\;[/math] Earth surface temperature at present time: (ref. 4) [math]T_{\oplus} = T_{\odot} \left(\frac{\left(1 - \alpha_{\oplus} \right)^{\frac{1}{2}} R_{\odot}}{2 r_{\oplus}} \right)^{\frac{1}{2}} = 248.367 \; \text{K}[/math] [math]\boxed{T_{\oplus} = 248.367 \; \text{K}}[/math] [math]\;[/math] Isotropic cosmic photon background radiation temperature at present time: (ref. 5) [math]T_{\gamma} = 2.72548 \; \text{K}[/math] [math]\;[/math] Toy model universe age at isotropic cosmic photon background radiation time: (ref. 6) [math]\boxed{T_{u,\gamma} = \frac{3}{4\left(k_B T_{\gamma,t} \right)^2} \sqrt{\frac{5 \Omega_{\gamma,t} \hbar^3 c^5}{G N_{\gamma} \pi^3}}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] Isotropic cosmic photon background radiation temperature at past time is equivalent to Earth surface temperature at present time: [math]\boxed{T_{\gamma,t} = T_{\oplus}}[/math] [math]\;[/math] Toy model universe age at isotropic cosmic photon background radiation time integration via substitution: [math]T_{u,\gamma} = \frac{3}{4\left(k_B T_{\gamma,t} \right)^2} \sqrt{\frac{5 \Omega_{\gamma,t} \hbar^3 c^5}{G N_{\gamma} \pi^3}} = \frac{3}{2 \left(k_B T_{\odot} \right)^2} \left( \frac{r_{\oplus}}{R_{\odot}} \right) \sqrt{\frac{5 \Omega_{\gamma,t} \hbar^3 c^5}{G N_{\gamma} \left(1 - \alpha_{\oplus} \right) \pi^3}}[/math] [math]\;[/math] Toy model universe age at abiogenesis epoch time: [math]\boxed{T_{u,\gamma} = \frac{3 r_{\oplus}}{2 R_{\odot} \left(k_B T_{\odot} \right)^2} \sqrt{\frac{5 \Omega_{\gamma,t} \hbar^3 c^5}{G N_{\gamma} \left(1 - \alpha_{\oplus} \right) \pi^3}}}[/math] [math]\boxed{T_{u,\gamma} = 1.447 \cdot 10^{15} \; \text{s}} \; \; \; \left(4.585 \cdot 10^{7} \; \text{years} \right)[/math] [math]\;[/math] If every planet around every second generation star and every third generation star were illuminated with a luminous isotropic cosmic photon background radiation source that is more cleaner and isotropic and more stable than the host star radiation source, could this cosmic photon background radiation have initiated a universe abiogenesis epoch? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: WMAP satellite content of the Universe: (ref. 1) http://map.gsfc.nasa.gov/media/080998/index.html Wikipedia - Sun Sol: (ref. 2) https://en.wikipedia.org/wiki/Sun Wikipedia - Earth: (ref. 3) https://en.wikipedia.org/wiki/Earth UVIC - The blackbody temperature of a planet: (ref. 4) http://www.astro.uvic.ca/~venn/A201/maths.7.planet_temperature.pdf Wikipedia - Cosmic microwave background radiation: (ref. 5) https://en.wikipedia.org/wiki/Cosmic_microwave_background Importance_of_precise_measurement Science Forums - Orion1 - Photon decoupling time: (ref. 6) https://www.scienceforums.net/topic/86694-observable-universe-mass/?do=findComment&comment=1037262

Solar surface temperature: (ref. 1) [math]T_{\odot} = 5772 \; \text{K}[/math] [math]\;[/math] Total solar radius: (ref. 1) [math]R_{\odot} = 6.963 \cdot 10^{5} \; \text{km}[/math] [math]\;[/math] Earth orbital radius semi-major axis: (ref. 2) [math]r_{\oplus} = 1.496 \cdot 10^{8} \; \text{km}[/math] [math]\;[/math] Earth albedo reflectivity: (ref. 2) [math]\alpha_{\oplus} = 0.367[/math] [math]\;[/math] Earth surface temperature at present time: (ref. 3) [math]T_{\oplus} = T_{\odot} \left(\frac{\left(1 - \alpha_{\oplus} \right)^{\frac{1}{2}} R_{\odot}}{2 r_{\odot}} \right)^{\frac{1}{2}} = 248.367 \; \text{K}[/math] [math]\boxed{T_{\oplus} = 248.367 \; \text{K}}[/math] [math]\;[/math] Cosmic photon background radiation temperature at present time: (ref. 4) [math]T_{\gamma,0} = 2.72548 \; \text{K}[/math] [math]\;[/math] Cosmology scale factor: [math]\boxed{\frac{a\left(t_0 \right)}{a\left(t \right)} = \frac{T_{\gamma,t}}{T_{\gamma,0}}}[/math] [math]\;[/math] Cosmology scale factor at present time: [math]\boxed{a\left(t_0 \right) = 1}[/math] [math]\;[/math] Cosmic photon background radiation temperature at past time is equivalent to Earth surface temperature at present time: [math]\boxed{T_{\gamma,t} = T_{\oplus}}[/math] [math]\;[/math] Universe abiogenesis epoch scale factor at past time: [math]a\left(t \right) = \frac{T_{\gamma,0}}{T_{\gamma,t}} = 0.011[/math] [math]\boxed{a\left(t \right) = \frac{T_{\gamma,0}}{T_{\gamma,t}}}[/math] [math]\boxed{a\left(t \right) = 0.011}[/math] [math]\;[/math] If every planet around every second generation star and every third generation star were illuminated with a luminous cosmic photon background radiation source that is cleaner and more stable than the host star radiation source, could this cosmic photon background radiation have initiated a universe abiogenesis epoch? [math]\;[/math] According to your universe model calculator, what is the universe age at this scale factor? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Sun Sol: (ref. 1) https://en.wikipedia.org/wiki/Sun Wikipedia - Earth: (ref. 2) https://en.wikipedia.org/wiki/Earth UVIC - The blackbody temperature of a planet: (ref. 3) http://www.astro.uvic.ca/~venn/A201/maths.7.planet_temperature.pdf Wikipedia - Cosmic microwave background radiation: (ref. 4) https://en.wikipedia.org/wiki/Cosmic_microwave_background#Importance_of_precise_measurement

Planck satellite baryonic cosmological composition parameter: (ref. 1, pg. 11, ref. 2, pg. 3) [math]\Omega_{b} = 0.0495[/math] [math]\;[/math] Black holes cosmological composition parameter: {ref. 2, pg. 3) [math]\Omega_{bh} = 0.00007[/math] [math]\;[/math] Solar mass: (ref. 3) [math]M_{\odot} = 1.9885 \cdot 10^{30} \; \text{kg}[/math] [math]\;[/math] Milky Way galaxy mass: (ref. 4, pg. 1) [math]M_{mw} = 1.260 \cdot 10^{12} \cdot M_{\odot} = 2.506 \cdot 10^{42} \; \text{kg}[/math] [math]\boxed{M_{mw} = 2.506 \cdot 10^{42} \; \text{kg}}[/math] [math]\;[/math] PSR J2215+5135 pulsar Tolman-Oppenheimer-Volkoff observational lower mass limit: (ref. 5) [math]\boxed{M_{bh} \geq 2.27 \cdot M_{\odot}}[/math] [math]\boxed{M_{bh} \geq 4.514 \cdot 10^{30} \; \text{kg}}[/math] [math]\;[/math] Stellar class O upper mass limit: (ref. 6) [math]\boxed{M_{bh} \geq 16 \cdot M_{\odot}}[/math] [math]\boxed{M_{bh} \geq 3.182 \cdot 10^{31} \; \text{kg}}[/math] [math]\;[/math] Toy model black holes per galaxy average number: [math]\frac{N_{bh}}{N_g} = \frac{\Omega_{bh} M_{mw}}{\Omega_b M_{bh}} = \left(1.114 \cdot 10^{8} \rightarrow 7.851 \cdot 10^{8} \right) \; \frac{\text{black holes}}{\text{galaxy}}[/math] [math]\boxed{\frac{N_{bh}}{N_g} = \frac{\Omega_{bh} M_{mw}}{\Omega_b M_{bh}}}[/math] [math]\boxed{\frac{N_{bh}}{N_g} = \left(1.114 \cdot 10^{8} \rightarrow 7.851 \cdot 10^{8} \right) \; \frac{\text{black holes}}{\text{galaxy}}}[/math] [math]\;[/math] Synthetic catalog black holes per galaxy average number: (ref. 7, pg. 1) [math]\frac{N_{bh}}{N_g} = 1.693 \cdot 10^{8} \; \frac{\text{black holes}}{\text{galaxy}}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf The Cosmic Energy Inventory: (ref. 2) http://arxiv.org/pdf/astro-ph/0406095v2.pdf Wikipedia - Sun Sol: (ref. 3) https://en.wikipedia.org/wiki/Sun Mass models of the Milky Way: (ref. 4) http://arxiv.org/pdf/1102.4340v1 Wikipedia - Tolman-Oppenheimer-Volkoff limit: (ref. 5) https://en.wikipedia.org/wiki/Tolman-Oppenheimer-Volkoff_limit Wikipedia - Stellar classification - Harvard spectral classification: (ref. 6) https://en.wikipedia.org/wiki/Stellar_classification#Harvard_spectral_classification Synthetic catalog of black holes in the Milky Way: (ref. 7) https://arxiv.org/pdf/1908.08775.pdf

Affirmative, I seem to have reached a reference citation impasse regarding the tensor field functions integration of [math]\Lambda^{\mu}_{\alpha}[/math] and [math]\Lambda^{\nu}_{\beta}[/math]. All of the references that have been cited do not demonstrate a functions integration past this initial point for a tensor field. Is this approach at least mathematically and symbolically correct to this point? Any citations or recommendations? Any discussions and/or peer reviews about this specific topic thread?

Affirmative. Your nearest college or university book store should have Calculus I and Physics and Astrophysics books available for purchase. Or you could locate the same or similar books at your local city, college or university library available for checkout. If these options are not available due to remote locality, then online purchases may also be available.

Toy model calculation versus observation comparison summary: [math]\begin{array}{l*{3}{c}r} \text{symbol} & \text{quantity} & \text{percent} \\ N_{g} & 2.330 \cdot 10^{12} \; \text{galaxies} & 116.5 \\ N_{g} & 2.000 \cdot 10^{12} \; \text{galaxies} & \\ N_{s} & 2.453 \cdot 10^{23} \; \text{stars} & 81.767 \\ N_{s} & 3.000 \cdot 10^{23} \; \text{stars} \\ N_p & 3.536 \cdot 10^{23} \; \text{planets} & 110.5 \\ N_p & 3.200 \cdot 10^{23} \; \text{planets} \\ \frac{N_s}{N_g} & 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}} & 70.2 \\ \frac{N_s}{N_g} & 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}} \\ \frac{N_p}{N_g} & 1.518 \cdot 10^{11} \; \frac{\text{planets}}{\text{galaxy}} & 94.875 \\ \frac{N_p}{N_g} & 1.600 \cdot 10^{11} \; \frac{\text{planets}}{\text{galaxy}} \\ \frac{N_p}{N_s} & 1.443 \; \frac{\text{planets}}{\text{star}} & 135.24 \\ \frac{N_p}{N_s} & 1.067 \; \frac{\text{planets}}{\text{star}} \\ \end{array}[/math] [math]\;[/math] Toy model particle properties chart: [math]\begin{array}{l*{9}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} & \text{decoupling time} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda & >0 \; \text{s} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 18.658 \; \text{eV} & \phi & 0.0255 \; \text{s} \\ f & \text{sterile neutrino} & +,- & 1/2 & 2 & 3 & 3 & 8.167 \; \text{eV} & \nu_{s} & 0.0255 \; \text{s} \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & 0.038 \; \text{eV} & \nu & 0.148 \; \text{s} \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma & 1.009 \cdot 10^{13} \; \text{s} & \left(3.197 \cdot 10^{5} \; \text{years} \right) \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} & >0 \; \text{s} \\ \end{array}[/math] [math][/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Scienceforums - Orion1 - toy model revision: https://www.scienceforums.net/topic/86694-observable-universe-mass/?do=findComment&comment=1089828 https://www.scienceforums.net/topic/86694-observable-universe-mass/?do=findComment&comment=1091408

Affirmative, in this toy model, sterile neutrinos have equivalent dark matter candidacy as scalar particles. [math]\;[/math] Planck satellite cosmological parameters at present time: (ref. 1, pg. 11) [math]\Omega_{dm} = 0.268[/math] [math]\;[/math] sterile neutrino composition is equivalent to dark matter composition at present time. [math]\boxed{\Omega_{s \nu} = \Omega_{dm}}[/math] [math]\;[/math] Fermi-Dirac total dark matter sterile neutrino distribution constant: [math]\boxed{C_{s \nu} = \frac{4 G N_{s \nu} \pi^3 \left(k_B T_{s \nu} \right)^4}{3 \Omega_{s \nu} H_0^2 \hbar^3 c^5}}[/math] [math]\;[/math] [math]\boxed{C_{s \nu} = 1.109 \cdot 10^{-3}}[/math] [math]\;[/math] Fermi-Dirac sterile neutrino density: [math]\boxed{\rho_{s \nu} = \frac{N_{s \nu} \pi^2 \left(k_B T_{s \nu} \right)^4}{2 C_{s \nu} \hbar^3 c^5}}[/math] [math]\;[/math] Fermi-Dirac sterile neutrino number density: (ref. 3, eq. 8) [math]n_{s \nu} = \left(\frac{3 \zeta \left(3 \right) N_{s \nu} \left(k_{B} T_{s \nu} \right)^3}{4 \pi^2 \left(\hbar c \right)^3} \right)[/math] [math]\;[/math] Fermi-Dirac dark matter sterile neutrino mass integration via substitution: [math]m_{s \nu} = \frac{\rho_{s \nu}}{n_{s \nu}} = \left(\frac{N_{s \nu} \pi^2 \left(k_B T_{s \nu} \right)^4}{2 C_{s \nu} \hbar^3 c^5} \right)\left(\frac{4 \pi^2 \left(\hbar c \right)^3}{3 \zeta \left(3 \right) N_{s \nu} \left(k_{B} T_{s \nu} \right)^3} \right) = \frac{2 \pi^{4} k_B T_{s \nu}}{3 C_{s \nu} c^{2} \zeta \left(3 \right)} = 1.456 \cdot 10^{-35} \; \text{kg}[/math] [math]\;[/math] Fermi-Dirac dark matter sterile neutrino mass: [math]\boxed{m_{s \nu} = \frac{2 \pi^{4} k_B T_{s \nu}}{3 C_{s \nu} c^{2} \zeta \left(3 \right)}}[/math] [math]\;[/math] [math]\boxed{m_{s \nu} = 1.456 \cdot 10^{-35} \; \text{kg}}[/math] [math]\boxed{m_{s \nu} = 8.167 \; \frac{\text{eV}}{c^2}}[/math] [math]\;[/math] WMAP satellite cosmological parameters at photon decoupling time: (ref. 2) [math]\Omega_{dm,t} = 0.63[/math] [math]\;[/math] Sterile neutrino composition is equivalent to dark matter composition at photon decoupling time. [math]\boxed{\Omega_{s \nu,t} = \Omega_{dm,t}}[/math] [math]\;[/math] Fermi-Dirac dark matter sterile neutrino decoupling time: [math]\boxed{T_{u,s \nu} = \frac{}{2\left(k_B T_{s \nu,t} \right)^2} \sqrt{\frac{3 \Omega_{s \nu,t} C_{s \nu} \hbar^3 c^5}{G N_{s \nu} \pi^3}}} \; \; \; m_{s \nu} \neq 0[/math] [math]\;[/math] [math]\boxed{T_{u,s \nu} = 0.0255 \; \text{s}} \; \; \; m_{s \nu} \neq 0[/math] [math]\;[/math] Toy model dark matter particle candidates chart: [math]\begin{array}{l*{8}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} & \text{decoupling time} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 18.658 \; \text{eV} & \phi & 0.0255 \; \text{s} \\ f & \text{sterile neutrino} & +,- & 1/2 & 2 & 3 & 3 & 8.167 \; \text{eV} & \nu_{s} & 0.0255 \; \text{s} \\ \end{array}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf WMAP satellite cosmological parameters at photon decoupling time: (ref. 2) https://map.gsfc.nasa.gov/media/080998/index.html Introduction to Cosmology: Lecture 6 - Thermal history of the Universe: (ref. 3) http://gravitation.web.ua.pt/sites/default/files/migrated2016/Lecture_6.pdf Wikipedia - Scalar boson: (ref. 4) https://en.wikipedia.org/wiki/Scalar_boson Wikipedia - Sterile neutrinos: (ref. 5) https://en.wikipedia.org/wiki/Sterile_neutrino Wikipedia - Dark matter: (ref. 6) https://en.wikipedia.org/wiki/Dark_matter

Toy model particle chart: [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & \neq 0 & \phi \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & \neq 0 & \nu \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} \\ \end{array}[/math] [math]\;[/math] Supersymmetry particle and sparticle chart: [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ sf & \text{sneutrino} & 0 & 0 & 1 & 3 & 3 & \neq 0 & \tilde{\nu} \\ b & \text{Higgs} & 0 & 0 & 1 & 1 & 1 & 125.18 \; \text{GeV} & H^{0} \\ sf & \text{higgsino} & +,- & 1/2 & 2 & 1 & 2 & 1.1 \; \text{TeV} & \ddot{H} \\ f & \text{sterile neutrino} & +,- & 1/2 & 2 & 3 & 3 & <1 \; \text{eV} - 10^{15} \; \text{GeV} & \nu \\ sf & \text{neutralino} & +,- & 1/2 & 2 & 4 & 4 & 100 \; \text{GeV} - 1 \; \text{TeV} & \ddot{N}^{0} \\ sf & \text{gravitino} & +,- & 3/2 & 2 & 1 & 2 & 1 \; \text{TeV} & \ddot{G} \\ \end{array}[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter density: [math]\boxed{\rho_{\phi} = \frac{N_{\phi} \pi^2 \left(k_B T_{\phi} \right)^4}{2 C_{\phi} \hbar^3 c^5}}[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter number density: (ref. 1, eq. 7) [math]n_{\phi} = \left(\frac{\zeta \left(3 \right) N_{\phi} \left(k_{B} T_{\phi} \right)^3}{\pi^2 \left(\hbar c \right)^3} \right)[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter mass: [math]m_{\phi} = \frac{\rho_{\phi}}{n_{\phi}} = \left(\frac{N_{\phi} \pi^2 \left(k_B T_{\phi} \right)^4}{2 C_{\phi} \hbar^3 c^5} \right)\left(\frac{\pi^2 \left(\hbar c \right)^3}{\zeta \left(3 \right) N_{\phi} \left(k_{B} T_{\phi} \right)^3} \right) = \frac{\pi^{4} k_B T_{\phi}}{2 C_{\phi} c^{2} \zeta \left(3 \right)} = 3.326 \cdot 10^{-35} \; \text{kg}[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter mass: [math]\boxed{m_{\phi} = \frac{\pi^{4} k_B T_{\phi}}{2 C_{\phi} c^{2} \zeta \left(3 \right)}}[/math] [math]\;[/math] [math]\boxed{m_{\phi} = 3.326 \cdot 10^{-35} \; \text{kg}}[/math] [math]\boxed{m_{\phi} = 18.658 \; \frac{\text{eV}}{c^2}}[/math] [math]\;[/math] Fermi-Dirac neutrino density: [math]\boxed{\rho_{\nu} = \frac{N_{\nu} \pi^2 \left(k_B T_{\nu} \right)^4}{2 C_{\nu} \hbar^3 c^5}}[/math] [math]\;[/math] Fermi-Dirac neutrino number density: (ref. 1, eq. 8) [math]n_{\nu} = \left(\frac{3 \zeta \left(3 \right) N_{\nu} \left(k_{B} T_{\nu} \right)^3}{4 \pi^2 \left(\hbar c \right)^3} \right)[/math] [math]\;[/math] Fermi-Dirac neutrino mass: [math]m_{\nu} = \frac{\rho_{\nu}}{n_{\nu}} = \left(\frac{N_{\nu} \pi^2 \left(k_B T_{\nu} \right)^4}{2 C_{\nu} \hbar^3 c^5} \right)\left(\frac{4 \pi^2 \left(\hbar c \right)^3}{3 \zeta \left(3 \right) N_{\nu} \left(k_{B} T_{\nu} \right)^3} \right) = \frac{2 \pi^{4} k_B T_{\nu}}{3 C_{\nu} c^{2} \zeta \left(3 \right)} = 6.839 \cdot 10^{-38} \; \text{kg}[/math] [math]\;[/math] Fermi-Dirac neutrino mass: [math]\boxed{m_{\nu} = \frac{2 \pi^{4} k_B T_{\nu}}{3 C_{\nu} c^{2} \zeta \left(3 \right)}}[/math] [math]\;[/math] [math]\boxed{m_{\nu} = 6.839 \cdot 10^{-38} \; \text{kg}}[/math] [math]\boxed{m_{\nu} = 0.038 \; \frac{\text{eV}}{c^2}}[/math] [math]\;[/math] Disqualifying dark matter particle candidates for this toy model based upon the predicted mass range, results in the remaining candidates chart. [math]\;[/math] Toy model dark matter particle remaining candidates chart: [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 18.658 \; \text{eV} & \phi \\ f & \text{sterile neutrino} & +,- & 1/2 & 2 & 3 & 3 & <1 \; \text{eV} - 10^{15} \; \text{GeV} & \nu \\ sf & \text{sneutrino} & 0 & 0 & 1 & 3 & 3 & \neq 0 & \tilde{\nu} \\ \end{array}[/math] [math]\;[/math] Would dark matter particle candidates that quantum mechanically decouple before/after neutrinos be a candidate qualifier/disqualifier? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Introduction to Cosmology: Lecture 6 - Thermal history of the Universe: (ref. 1) http://gravitation.web.ua.pt/sites/default/files/migrated2016/Lecture_6.pdf Wikipedia - Scalar boson: (ref. 2) https://en.wikipedia.org/wiki/Scalar_boson Wikipedia - Sterile neutrinos: (ref. 3) https://en.wikipedia.org/wiki/Sterile_neutrino Wikipedia - Dark matter: (ref. 4) https://en.wikipedia.org/wiki/Dark_matter

Toy model particle chart: [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & \neq 0 & \phi \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & \neq 0 & \nu \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} \\ \end{array}[/math] [math]\;[/math] Supersymmetry particle and sparticle chart: [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{quinton} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda^{0} \\ b & \text{higgson} & 0 & 0 & 1 & 1 & 1 & \neq 0 & h^{0} \\ sf & \text{sneutrino} & 0 & 0 & 1 & 3 & 3 & \neq 0 & \tilde{\nu} \\ b & \text{Higgs} & 0 & 0 & 1 & 1 & 1 & \neq 0 & H^{0} \\ sf & \text{higgsino} & +,- & 1/2 & 2 & 1 & 2 & \neq 0 & \ddot{H} \\ sf & \text{gravitino} & +,- & 3/2 & 2 & 1 & 2 & \neq 0 & \ddot{G} \\ \end{array}[/math] [math]\;[/math] A quintessence boson scalar particle is named a 'quinton' for speculative discussion, because no quintessence scalar boson particle is named in quintessence research. [math]\;[/math] A Higgs boson supersymmetry superpartner scalar sparticle boson is named a 'higgson', for speculative discussion. [math]\;[/math] Higgson sparticles generation via nuclear reaction is speculated to be: [math]H^{0} + H^{0} \rightarrow h^{0} + h^{0}[/math] [math]h^{0} + h^{0} \rightarrow H^{0} + H^{0}[/math] [math]\;[/math] Do these sparticle nuclear reactions violate any already known conservation laws? [math]\;[/math] Note that only a quinton and a higgson particle is all that is required to explain all observations in this toy model. [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? Reference: Wikipedia - Quintessence (physics): https://en.wikipedia.org/wiki/Quintessence_(physics) Wikipedia - Lightest Supersymmetric Particle: https://en.wikipedia.org/wiki/Lightest_Supersymmetric_Particle

Affirmative. The quantum-mechanical model boundary conditions for the scalar particle decoupling time versus the [math]\Lambda[/math]CDM model scalar particle decoupling time and a non-zero neutrino mass particle decoupling time and a non-zero sterile neutrino mass particle decoupling time appears to favor a scalar particle for dark matter. [math]\;[/math] Scalar particle decoupling time: [math]T_{u,\phi} = \frac{1}{H_{\phi,t}} = \frac{}{2 \left(k_B T_{\phi,t} \right)^2} \sqrt{\frac{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}{G N_{\phi} \pi^3}} = 0.0255 \; \text{s}[/math] [math]\boxed{T_{u,\phi} = \frac{}{2 \left(k_B T_{\phi,t} \right)^2} \sqrt{\frac{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}{G N_{\phi} \pi^3}}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{T_{u,\phi} = 0.0255 \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] [math]\Lambda[/math]CDM universe model semi-emperical temperature-time scale factor: (ref. 2, pg. 18, eq. 2, ref. 3) [math]\left(\frac{T_{\phi}}{T_{\phi,t}} \right)^{2} = \frac{T_{u,\phi}}{T_{u}} = T_{u,\phi} H_0[/math] [math]T_{u,\phi} = \frac{}{H_0} \left(\frac{T_{\phi}}{T_{\phi,t}} \right)^{2} = 0.0166 \; \text{s}[/math] [math]\Lambda[/math]CDM universe model semi-emperical scalar particle decoupling time: [math]\boxed{T_{u,\phi} = \frac{}{H_0} \left(\frac{T_{\phi}}{T_{\phi,t}} \right)^{2}}[/math] [math]\boxed{T_{u,\phi} = 0.0166 \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] Neutrino decoupling time: [math]T_{u,\nu} = \frac{1}{H_{\nu,t}} = \frac{}{2 \left(k_B T_{\nu,t} \right)^2} \sqrt{\frac{3 C_{\nu} \Omega_{\nu,t} \hbar^3 c^5}{G N_{\nu} \pi^3}} = 0.148 \; \text{s}[/math] [math]\boxed{T_{u,\nu} = \frac{}{2 \left(k_B T_{\nu,t} \right)^2} \sqrt{\frac{3 C_{\nu} \Omega_{\nu,t} \hbar^3 c^5}{G N_{\nu} \pi^3}}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{T_{u,\nu} = 0.148 \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Sterile neutrino decoupling time: [math]\boxed{T_{u,\nu} = \frac{}{2 \left(k_B T_{\nu,t} \right)^2} \sqrt{\frac{3 C_{\nu} \Omega_{\nu,t} \hbar^3 c^5}{G N_{\nu} \pi^3}}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{T_{u,\nu} = 0.372 \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Particle decoupling time summary: [math]\boxed{T_{u,\phi} = 0.0255 \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{T_{u,\phi} = 0.0166 \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{T_{u,\nu} = 0.148 \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{T_{u,\nu} = 0.372 \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Is it possible for the Higgs boson to have a related supersymmetric scalar particle boson with non-zero mass? (ref. 4) Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: WMAP satellite content of the Universe: (ref. 1) http://map.gsfc.nasa.gov/media/080998/index.html Cosmology: nucleosynthesis and inflation: (ref. 2) http://www.uio.no/studier/emner/matnat/astro/AST1100/h07/undervisningsmateriale/lecture25.pdf Wikipedia - Lambda-CDM_model parameters: (ref. 3) https://en.wikipedia.org/wiki/Lambda-CDM_model Wikipedia - Lightest Supersymmetric Particle: (ref. 4) https://en.wikipedia.org/wiki/Lightest_Supersymmetric_Particle

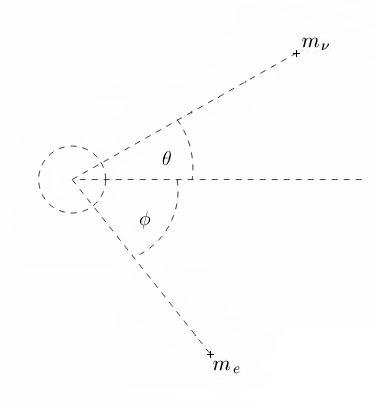
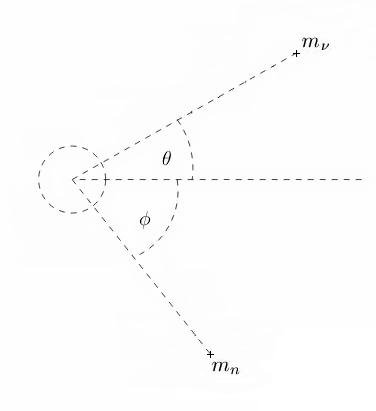
Affirmative, revision complete. Derivation of neutrino mass from neutrino scattering: [math]\;[/math] [math]\theta[/math] - scattered neutrino angle [math]\phi[/math] - electron recoil angle [math]E_{\nu i}[/math] - initial neutrino total energy [math]E_{\nu f}[/math] - final neutrino total energy [math]E_{e}[/math] - electron total energy [math]E_{\nu}[/math] - neutrino total energy Scattered particles rebounding with relativistic momentum have total energy: [math]E_{e} = \gamma_{e} m_{e} c^{2} \; \; \; \; \; \; E_{\nu} = \gamma_{\nu} m_{\nu} c^{2}[/math] Where [math]m_{e}[/math] and [math]m_{\nu}[/math] are the particle rest masses. [math]\gamma_{e}[/math] and [math]\gamma_{\nu}[/math] are the Lorentz factors. (ref. 1) [math]\;[/math] Observational measurements of both particle total energy and velocity, it is possible to calculate the particle rest masses [math]m_{e}[/math] and [math]m_{\nu}[/math]: [math]\boxed{m_{e} = \frac{E_{e}}{\gamma_{e} c^{2}}} \; \; \; \; \; \; \boxed{m_{\nu} = \frac{E_{\nu}}{\gamma_{\nu} c^{2}}} \tag{0}[/math] [math]\;[/math] [math]E_{e} \sin \phi = E_{\nu f} \sin \theta \tag{1}[/math] [math]\;[/math] [math]E_{e} \cos \phi + E_{\nu f} \cos \theta = E_{\nu i} \tag{2}[/math] [math]\;[/math] Isolate [math]E_{e} \cos \phi[/math] from equation (2): [math]E_{e} \cos \phi = E_{\nu i} - E_{\nu f} \cos \theta \tag{3}[/math] [math]\;[/math] Divide equation (1) by equation (3) for an expression for [math]\tan \phi[/math]. [math]\;[/math] [math]\tan \phi = \frac{E_{\nu f} \sin \theta}{E_{\nu i} - E_{\nu f} \cos \theta} = \frac{\sin \theta}{\frac{E_{\nu i}}{E_{\nu f}} - \cos \theta} \tag{4}[/math] [math]\;[/math] Acquire a substitution for [math]\frac{E_{\nu i}}{E_{\nu f}}[/math] to eliminate [math]E_{\nu f}[/math]. Use the Compton equation, which can be rearranged to yield [math]\frac{\lambda_{\nu f}}{\lambda_{\nu i}} = \frac{E_{\nu i}}{E_{\nu f}}[/math] in terms of [math]\lambda_{\nu i}[/math] alone. [math]\;[/math] [math]\frac{\lambda_{\nu f}}{\lambda_{\nu i}} = \frac{E_{\nu i}}{E_{\nu f}} \tag{5}[/math] [math]\;[/math] [math]\frac{\lambda_{\nu f}}{\lambda_{\nu i}} = \frac{E_{\nu i}}{E_{\nu f}} = 1 + \frac{E_{\nu i}}{E_{e}} \left(1 - \cos \theta \right) = 1 + \frac{\gamma_{\nu} m_{\nu} c^2}{\gamma_{e} m_{e} c^2} \left(1 - \cos \theta \right) = 1 + \frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \left(1 - \cos \theta \right) \tag{6}[/math] [math]\;[/math] Substituting equation (6) into equation (4) and eliminate [math]E_{\nu i}[/math] and [math]E_{\nu f}[/math] in favor of [math]m_{\nu}[/math] alone. [math]\tan \phi = \frac{\sin \theta}{\frac{E_{\nu i}}{E_{\nu f}} - \cos \theta} = \frac{\sin \theta}{1 + \frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \left(1 - \cos \theta \right) - \cos \theta} = \frac{\sin \theta}{\left(1 + \frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \right)\left(1 - \cos \theta \right)} \tag{7}[/math] [math]\;[/math] Utilizing a trigonometric identity produces the desired result, specifically: [math]\frac{1 - \cos \theta}{\sin \theta} = \tan \left(\frac{\theta}{2} \right) \tag{8}[/math] [math]\;[/math] Substituting this trigonometric identity into equation (7) results in: [math]\left(1 + \frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \right) \tan \phi = \cot \frac{\theta}{2} \tag{9}[/math] [math]\;[/math] Solve for neutrino rest mass [math]m_{\nu}[/math]: [math]\tan \phi + \frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \tan \phi = \cot \frac{\theta}{2} \tag{10}[/math] [math]\;[/math] [math]\frac{\gamma_{\nu} m_{\nu}}{\gamma_{e} m_{e}} \tan \phi = \left(\cot \frac{\theta}{2} - \tan \phi \right) \tag{11}[/math] [math]\;[/math] Electron-neutrino scattering neutrino rest mass: [math]\boxed{m_{\nu} = \frac{\gamma_{e} m_{e} \cot \phi}{\gamma_{\nu}} \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{12}[/math] [math]\;[/math] Nuclear-neutrino scattering neutrino rest mass: [math]\boxed{m_{\nu} = \frac{\gamma_{n} m_{n} \cot \phi}{\gamma_{\nu}} \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{13}[/math] [math]m_{n}[/math] - nuclear rest mass [math]\;[/math] Electron interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{\left(\gamma_{e} m_{e} + \gamma_{\nu} m_{\nu} \right) \tan \phi}{\gamma_{e} m_{e}} \right)} \tag{14}[/math] [math]\;[/math] Neutrino interaction electron recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{\gamma_{e} m_{e} \cot \frac{\theta}{2}}{\gamma_{e} m_{e} + \gamma_{\nu} m_{\nu}} \right)} \tag{15}[/math] [math]\;[/math] Nuclear interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{\left(\gamma_{n} m_{n} + \gamma_{\nu} m_{\nu} \right) \tan \phi}{\gamma_{n} m_{n}} \right)} \tag{16}[/math] [math]\;[/math] Neutrino interaction nuclear recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{\gamma_{n} m_{n} \cot \frac{\theta}{2}}{\gamma_{n} m_{n} + \gamma_{\nu} m_{\nu}} \right)} \tag{17}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Lorentz factor: (ref. 1) https://en.wikipedia.org/wiki/Lorentz_factor Wikipedia - Compton scattering - Derivation of the scattering formula: https://en.wikipedia.org/wiki/Compton_scattering#Derivation_of_the_scattering_formula Physics 253 - Compton Scattering - Patrick LeClair http://pleclair.ua.edu//PH253/Notes/compton.pdf Orion1 - Neutrino mass from Fermi-Dirac statistics...: https://www.scienceforums.net/topic/90189-neutrino-mass-from-fermi-dirac-statistics/ Science News - Neutrinos seen scattering off an atom’s nucleus for the first time: https://www.sciencenews.org/article/neutrinos-seen-scattering-atoms-nucleus-first-time

De Broglie relativistic momentum: (ref. 1) [math]p = \frac{\hbar}{\overline{\lambda}} = \gamma m_0 v[/math] [math]\;[/math] Relativistic energy-momentum relation and relativistic mass particle total energy identity: [math]\boxed{E_{t} = \sqrt{\left(m_{0} c^{2} \right)^{2} + \left(pc \right)^{2}} = \gamma m_0 c^2}[/math] [math]\;[/math] [math]\boxed{E_{t} = \sqrt{\left(m_{0} c^{2} \right)^{2} + \left(\frac{\hbar c}{\overline{\lambda}} \right)^{2}} = \gamma m_0 c^2}[/math] [math]\;[/math] Is this equation an identity for a relativistic mass particle? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Matter wave: (ref. 1) https://en.wikipedia.org/wiki/Matter_wave

Relativistic energy-momentum relation total energy: (ref. 1) [math]E_{t}^{2} = \left(m_{0} c^{2} \right)^{2} + \left(pc \right)^{2} [/math] [math]E_{t} = \sqrt{\left(m_{0} c^{2} \right)^{2} + \left(pc \right)^{2}}[/math] [math]\;[/math] Relativistic mass particle total energy: (ref. 2) [math]E_{t} = \gamma m_0 c^{2}[/math] [math]\;[/math] Relativistic energy-momentum relation and relativistic mass particle total energy identity: [math]\boxed{E_{t} = \sqrt{\left(m_{0}c^{2} \right)^{2} + \left(pc \right)^{2}} = \gamma m_0 c^2}[/math] [math]\;[/math] Is this equation an identity for a relativistic mass particle? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Energy-momentum relation: (ref. 1) https://en.wikipedia.org/wiki/Energy–momentum_relation#Special_relativity Wikipedia - Energy-momentum relation - heuristic approach for massive particles: (ref. 2) https://en.wikipedia.org/wiki/Energy–momentum_relation#Heuristic_approach_for_massive_particle

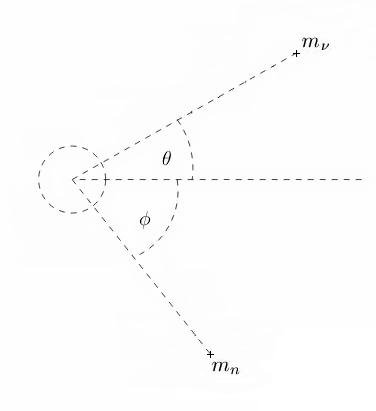
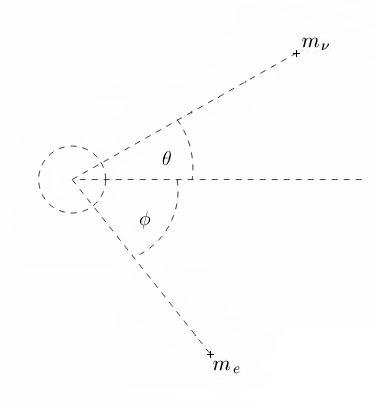
Derivation of neutrino mass from neutrino scattering: [math]\;[/math] [math]\theta[/math] - scattered neutrino angle [math]\phi[/math] - electron recoil angle [math]p_{i}[/math] - initial neutrino momentum [math]p_{f}[/math] - final neutrino momentum [math]p_{e}[/math] - electron momentum [math]\;[/math] Scattered particles rebounding with relativistic momentum have total energy: [math]E_{e} = \gamma m_{0e} c^{2} = m_{e} c^{2} \; \; \; \; \; \; E_{\nu} = \gamma m_{0\nu} c^{2} = m_{\nu} c^{2}[/math] Where [math]m_{e}[/math] and [math]m_{\nu}[/math] are the relativistic particle masses. [math]\;[/math] Observational measurements of both particle total energy and velocity, it is possible to calculate the rest particle masses [math]m_{0e}[/math] and [math]m_{0\nu}[/math]: [math]\boxed{m_{0e} = \frac{E_{e}}{\gamma c^{2}}} \; \; \; \; \; \; \boxed{m_{0\nu} = \frac{E_{\nu}}{\gamma c^{2}}} \tag{0}[/math] [math]\;[/math] [math]p_{e} \sin \phi = p_{f} \sin \theta \tag{1}[/math] [math]\;[/math] [math]p_{e} \cos \phi + p_{f} \cos \theta = p_{i} \tag{2}[/math] [math]\;[/math] Isolate [math]p_{e} \cos \phi[/math] from equation [math](2)[/math]: [math]p_{e} \cos \phi = p_{i} - p_{f} \cos \theta \tag{3}[/math] [math]\;[/math] Divide equation [math](1)[/math] by equation [math](3)[/math] for an expression for [math](3)[/math] for an expression for [math]\tan \phi[/math]: [math]\;[/math] [math]\tan \phi = \frac{p_{f} \sin \theta}{p_{i} - p_{f} \cos \theta} = \frac{\sin \theta}{\frac{p_{i}}{p_{f}} - \cos \theta} \tag{4}[/math] [math]\;[/math] Acquire a substitution for [math]\frac{p_{i}}{p_{f}}[/math] to eliminate [math]p_{f}[/math]. Use the Compton equation, which can be rearranged to yield [math]\frac{\lambda_{f}}{\lambda_{i}} = \frac{p_{i}}{p_{f}}[/math] in terms of [math]\lambda_{i}[/math] alone. [math]\;[/math] [math]\frac{\lambda_{f}}{\lambda_{i}} = \frac{p_{i}}{p_{f}} \tag{5}[/math] [math]\;[/math] [math]\frac{\lambda_{f}}{\lambda_{i}} = \frac{p_{i}}{p_{f}} = 1 + \frac{E_{\nu}}{E_{e}} \left(1 - \cos \theta \right) = 1 + \frac{m_{\nu} c^2}{m_{e} c^2} \left(1 - \cos \theta \right) = 1 + \frac{m_{\nu}}{m_{e}} \left(1 - \cos \theta \right) \tag{6}[/math] [math]\;[/math] Substituting equation [math](6)[/math] into equation [math](4)[/math], and eliminate [math]p_{i}[/math] and [math]p_{f}[/math] in favor of [math]m_{\nu}[/math] alone. [math]\;[/math] [math]\tan \phi = \frac{\sin \theta}{\frac{p_{i}}{p_{f}} - \cos \theta} = \frac{\sin \theta}{1 + \frac{m_{\nu}}{m_{e}} \left(1 - \cos \theta \right) - \cos \theta} = \frac{\sin \theta}{\left(1 + \frac{m_{\nu}}{m_{e}} \right)\left(1 - \cos \theta \right)} \tag{7}[/math] [math]\;[/math] Utilizing a trigonometric identity produces the desired result, specifically: [math]\frac{1 - \cos \theta}{\sin \theta} = \tan \left(\frac{\theta}{2} \right) \tag{8}[/math] [math]\;[/math] Substituting this trigonometric identity into equation [math](7)[/math] results in: [math]\left(1 + \frac{m_{\nu}}{m_{e}} \right) \tan \phi = \cot \frac{\theta}{2} \tag{9}[/math] [math]\;[/math] Solve for neutrino mass [math]m_{\nu}[/math]: [math]\tan \phi + \frac{m_{\nu}}{m_{e}} \tan \phi = \cot \frac{\theta}{2} \tag{10}[/math] [math]\;[/math] [math]\frac{m_{\nu}}{m_{e}} \tan \phi = \left(\cot \frac{\theta}{2} - \tan \phi \right) \tag{11}[/math] [math]\;[/math] Electron-neutrino scattering neutrino mass: [math]\boxed{m_{\nu} = m_{e} \cot \phi \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{12}[/math] [math]\;[/math] Nuclear-neutrino scattering neutrino mass: [math]\boxed{m_{\nu} = m_{n} \cot \phi \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{13}[/math] [math]\;[/math] [math]m_{n}[/math] - nuclear mass [math]\;[/math] Electron interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{\left(m_{e} + m_{\nu} \right) \tan \phi}{m_{e}} \right)} \tag{14}[/math] [math]\;[/math] Neutrino interaction electron recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{m_{e} \cot \frac{\theta}{2}}{m_{e} + m_{\nu}} \right)} \tag{15}[/math] [math]\;[/math] Nuclear interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{\left(m_{n} + m_{\nu} \right) \tan \phi}{m_{n}} \right)} \tag{16}[/math] [math]\;[/math] Neutrino interaction nuclear recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{m_{n} \cot \frac{\theta}{2}}{m_{n} + m_{\nu}} \right)} \tag{17}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Compton scattering - Derivation of the scattering formula: https://en.wikipedia.org/wiki/Compton_scattering#Derivation_of_the_scattering_formula Physics 253 - Compton Scattering - Patrick LeClair http://pleclair.ua.edu//PH253/Notes/compton.pdf Orion1 - Neutrino mass from Fermi-Dirac statistics...: https://www.scienceforums.net/topic/90189-neutrino-mass-from-fermi-dirac-statistics/ Science News - Neutrinos seen scattering off an atom’s nucleus for the first time: https://www.sciencenews.org/article/neutrinos-seen-scattering-atoms-nucleus-first-time

Please cite which Wikipedia reference? Also, I am not certain which 'discussion' with 'list of references' that is being inferred, please clarify. (ref. 7) Server guideline rule Section 2 (7) prohibits me from directing discussion to another server. My apology if my stringent interpretation of this rule is overtly strict or interpreted as a deflection or deferment, please clarify. (ref. 7) Do you agree with this equation for deriving a relativistic lagrangian? Relativistic Lagrangian integration via substitution: [math]\mathcal{L} = \sum_{1}^{n} E_{k}\left(n \right) - \sum_{1}^{n} E_{p}\left(n \right) = \sum_{1}^{n} \mathcal{L}\left(n \right) = 0[/math] Relativistic Lagrangian: [math]\boxed{\mathcal{L} = \sum_{1}^{n} \mathcal{L}\left(n \right) = 0}[/math] Affirmative, revision complete. [math]\;[/math] Einstein's field equations: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = \frac{8 \pi G}{c^{4}} T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations in natural units: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = 8 \pi T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations Ricci tensor in natural units: [math]\boxed{ R_{\mu \nu} = 8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}[/math] [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{ \mathbb{R} }_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] General relativity Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] A massless gravitational wave is still massless on a Planck scale, and the result is still a metric tensor field. [math]\;[/math] Metric tensor field: (ref. 1, pg. 21, eq. 1.68) [math]T^{' \mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] The general relativity Ricci tensor is a metric tensor field: [math]\boxed{R_{\mu \nu} = T^{' \mu \nu} \left(x' \right)}[/math] [math]\;[/math] Lagrangian equation for a massless Planck graviton: [math]\boxed{\mathcal{L} = \underbrace{ \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right) }_{QG} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] General relativity and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric: (ref. 2) [math]g_{\mu \nu} = \eta_{\mu \nu} + h_{\mu \nu}[/math] [math]\;[/math] [math]\eta_{\mu \nu}[/math] - perturbed nondynamical background metric [math]h_{\mu \nu}[/math] - true metric deviation of [math]g_{\mu \nu}[/math] from flat spacetime [math]\;[/math] [math]h_{\mu \nu}[/math] must be negligible compared to [math]\eta_{\mu \nu}[/math]: [math]|h_{\mu \nu}| \ll 1[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 1: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 2: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) - \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right)}[/math] [math]\;[/math] General relativity Ricci scalar curvature: (ref. 3, ref. 4) [math]R = g^{\mu \nu } R_{\mu \nu}[/math] [math]\;[/math] General relativity Ricci scalar curvature and Planck quantum gravity scalar curvature: [math]R = g^{\mu \nu } R_{\mu \nu} = g^{\mu \nu } \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)[/math] [math]\boxed{R = g^{\mu \nu } \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 2 integration via substitution: [math]8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) - \frac{1}{2} g^{\mu \nu} \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} g^{\mu \nu}\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 3: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} g^{\mu \nu}\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime inverse metric: (ref. 2) [math]g^{\mu \nu} = \eta^{\mu \nu} - h^{\mu \nu}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 4: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - h^{\mu \nu} \right)\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] [math]\Lambda^{\mu}_{\alpha} = \frac{\partial \xi^{\mu}}{\partial x^{\alpha}} \; \; \; \; \; \; \Lambda^{\nu}_{\beta} = \frac{\partial \xi^{\nu}}{\partial x^{\beta}}[/math] [math]\;[/math] [math]x^{\alpha} = \left(ct, r, \theta, \phi \right) \; \; \; \; \; \; x^{\beta} = \left(ct, r, \theta, \phi \right)[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 5: (ref. 5) [math]\boxed{8 \pi T_{\mu \nu} = \left(\frac{\partial \xi^{\mu}}{\partial x^{\alpha}} \right)\left(\frac{\partial \xi^{\nu}}{\partial x^{\beta}} \right) T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - h^{\mu \nu} \right)\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] General relativity stress-energy tensor: [math]T_{\mu \nu} = \pm \left(\begin{matrix} -\rho & 0 & 0 & 0 \\ 0 & p & 0 & 0 \\ 0 & 0 & p & 0 \\ 0 & 0 & 0 & p \end{matrix} \right)[/math] [math]\;[/math] In spherical coordinates [math](ct, r, \theta, \phi)[/math] the Minkowski flat spacetime metric takes the form: [math]ds^{2} = -c^{2} dt^{2} + dr^{2} + r^{2} d\theta^{2} + r^{2} \sin^{2} \theta \; d\phi^{2}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric tensor: [math]T^{\alpha \beta} \left(x \right) = \pm \begin{pmatrix} -c^{2} dt^{2} & 0 & 0 & 0 \\ 0 & dr^{2} & 0 & 0 \\ 0 & 0 & r^{2} d\theta^{2} & 0 \\ 0 & 0 & 0 & r^{2} \sin^{2} \theta \; d\phi^{2} \end{pmatrix}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric: [math]\eta_{\mu \nu} = \pm \begin{pmatrix} -1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{pmatrix}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric is equivalent to the inverse metric: (ref. 6) [math]\boxed{\eta_{\mu \nu} = \eta^{\mu \nu}}[/math] [math]\;[/math] General relativity Minkowski flat spacetime perturbed nondynamical background metric deviation is equivalent to the inverse metric deviation: [math]\boxed{h_{\mu \nu} = h^{\mu \nu}}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf Wikipeda - General relativity - linearized gravity: (ref. 2) https://en.wikipedia.org/wiki/Linearized_gravity Wikipeda - General relativity: (ref. 3) https://en.wikipedia.org/wiki/General_relativity#Einstein's_equations Wikipeda - General relativity: (ref. 4) https://en.wikipedia.org/wiki/Metric_tensor_(general_relativity)#Einstein's_equations Wikipeda - General relativity - Metric tensor - Local coordinates and matrix representations: (ref. 5) https://en.wikipedia.org/wiki/Metric_tensor_(general_relativity)#Local_coordinates_and_matrix_representations Wikipeda - Lorentz covariance: (ref. 6) https://en.wikipedia.org/wiki/Lorentz_covariance Science Forums - Guidelines: (ref. 7) https://www.scienceforums.net/guidelines/

Affirmative, is this an identity of the lagrangian Dirac equation with EM interaction? [math]\mathcal{L} = \underbrace{\overline{\psi} \left(i \gamma^{\mu} D_{\mu} - m \right) \psi}_{Dirac} - \underbrace{e \overline{\psi} \gamma^{\mu} Q \psi A_{\mu}}_{EM \text{ } interaction}[/math] [math]\;[/math] [math]\mathcal{L} = \overline{\psi} \left(i \gamma^{\mu} D_{\mu} - m \right) \psi - e \overline{\psi} \gamma^{\mu} Q \psi A_{\mu}[/math] [math]\;[/math] [math]\mathcal{L} = \overline{\psi} i \gamma^{\mu} D_{\mu} \psi - \overline{\psi} m \psi - e \overline{\psi} \gamma^{\mu} Q \psi A_{\mu}[/math] [math]\;[/math] [math]\mathcal{L} = \overline{\psi} i \gamma^{\mu} D_{\mu} \psi - e \overline{\psi} \gamma^{\mu} Q \psi A_{\mu} - \overline{\psi} m \psi[/math] [math]\;[/math] [math]\mathcal{L} = \overline{\psi} \gamma^{\mu} \left(i D_{\mu} - e Q A_{\mu} \right)\psi - \overline{\psi} m \psi[/math] [math]\;[/math] [math]\boxed{\mathcal{L} = \overline{\psi} \left[\gamma^{\mu}\left(i D_{\mu} - e Q A_{\mu} \right) - m \right] \psi}[/math]

The scientific author of the original lagrangian equation appears to be modeling a massless and chargeless quantum field interaction. [math]\;[/math] General relativity Lagrangian equation with mass and charge and a GUT quaternion: (ref. 1, ref. 2, pg. 8, eq. 2.8) [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{\overline{\psi} \left(i \gamma^{\mu} D_{\mu} - m \right) \psi}_{Dirac} - \underbrace{e \overline{\psi} \gamma^{\mu} Q \psi A_{\mu}}_{EM \text{ } interaction} + \underbrace{|D_{\mu} h|^2 - V\left(|h| \right)}_{Higgs} + \underbrace{\overline{\psi^{\alpha}} \gamma_{\mu} \left(A_{\mu}^{\alpha \beta} \psi^{\beta} + \psi^{\alpha} B_{\mu} \right)}_{GUT \text{ } quaternion} = 0}[/math] [math]\;[/math] Reference: Wikipedia - Grand Unified Theory - Symplectic groups and quaternion representations: (ref. 1) https://en.wikipedia.org/wiki/Grand_Unified_Theory#Symplectic_groups_and_quaternion_representations Search For The Standard Model Higgs Boson In Leptons Plus Jets Final States: (ref. 2) https://www-d0.fnal.gov/results/publications_talks/thesis/nguyen/thesis.pdf

There does not appear to be a scientific consensus for the lagrangian equation for GUT Pati-Salam models. The integration strategy appears to involve modeling both gauge symmetry and particle hierarchy into the Yukawa lagrangian. [math]\;[/math] Yukawa lagrangian: [math]\mathcal{L} = \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] However, the GUT quaternion lagrangian equation appears to exhibit both gauge symmety and particle heirarchy and matrix parameters which could be compatible with the SO(10) regime under Pati-Salam. [math]\;[/math] GUT quaternion lagrangian equation: (ref. 1) [math]\mathcal{L} = \overline{\psi^{a}} \gamma_{\mu} \left(A_{\mu}^{ab} \psi^{b} + \psi^{a} B_{\mu} \right)[/math] [math]\;[/math] Because the GUT energy scale is well below the Planck energy scale, the general relativity Lagrangian equation may still be utilized without the introduction of a graviton. [math]\;[/math] GUT energy scale: [math]\Lambda_{\text{GUT}} \approx 10^{16} \; \text{GeV}[/math] [math]\;[/math] Planck energy scale: [math]E_{P} = 1.221 \cdot 10^{19} \; \mathrm {GeV}[/math] [math]\;[/math] General relativity Lagrangian equation with a GUT quaternion: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{\overline{\psi^{a}} \gamma_{\mu} \left(A_{\mu}^{ab} \psi^{b} + \psi^{a} B_{\mu} \right)}_{\text{GUT quaternion}} = 0}[/math] Reference: Wikipedia - Grand Unified Theory - Symplectic groups and quaternion representations: (ref. 1) https://en.wikipedia.org/wiki/Grand_Unified_Theory#Symplectic_groups_and_quaternion_representations

Affirmative, revision complete. [math]\;[/math] Einstein's field equations: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = \frac{8 \pi G}{c^{4}} T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations in natural units: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = 8 \pi T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations Ricci tensor in natural units: [math]\boxed{ R_{\mu \nu} = 8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}[/math] [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{ \mathbb{R} }_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] General relativity Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] A massless gravitational wave is still massless on a Planck scale, and the result is still a metric tensor field. [math]\;[/math] Metric tensor field: (ref. 1, pg. 21, eq. 1.68) [math]T^{' \mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] The general relativity Ricci tensor is a metric tensor field: [math]\boxed{R_{\mu \nu} = T^{' \mu \nu} \left(x' \right)}[/math] [math]\;[/math] Lagrangian equation for a massless Planck graviton: [math]\boxed{\mathcal{L} = \underbrace{ \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right) }_{QG} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] General relativity and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric: (ref. 2) [math]g_{\mu \nu} = \eta_{\mu \nu} + h_{\mu \nu}[/math] [math]\;[/math] [math]\eta_{\mu \nu}[/math] - perturbed non-dynamical background metric [math]h_{\mu \nu}[/math] - true metric deviation of [math]g_{\mu \nu}[/math] from flat spacetime [math]\;[/math] [math]h_{\mu \nu}[/math] must be negligible compared to [math]\eta_{\mu \nu}[/math]: [math]|h_{\mu \nu}| \ll 1[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 1: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 2: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) - \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right)}[/math] [math]\;[/math] General relativity curvature scalar: (ref. 3) [math]R = g^{\mu \nu } R_{\mu \nu}[/math] [math]\;[/math] General relativity and Planck quantum gravity curvature scalar: [math]R = g^{\mu \nu } R_{\mu \nu} = g^{\mu \nu } \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)[/math] [math]\boxed{R = g^{\mu \nu } \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 2 integration via substitution: [math]8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) - \frac{1}{2} g^{\mu \nu} \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} g^{\mu \nu}\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 3: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} g^{\mu \nu}\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime inverse metric: (ref. 2) [math]g^{\mu \nu} = \eta^{\mu \nu} - h^{\mu \nu}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 4: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - h^{\mu \nu} \right)\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] [math]\Lambda^{\mu}_{\alpha} = \frac{\partial \xi^{\mu}}{\partial x^{\alpha}} \; \; \; \; \; \; \Lambda^{\nu}_{\beta} = \frac{\partial \xi^{\nu}}{\partial x^{\beta}}[/math] [math]\;[/math] [math]x^{\alpha} = \left(ct, r, \theta, \phi \right) \; \; \; \; \; \; x^{\beta} = \left(ct, r, \theta, \phi \right)[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 5: [math]\boxed{8 \pi T_{\mu \nu} = \left(\frac{\partial \xi^{\mu}}{\partial x^{\alpha}} \right)\left(\frac{\partial \xi^{\nu}}{\partial x^{\beta}} \right) T^{\alpha \beta} \left(x \right) \left(1 - \frac{1}{2} \left(\eta^{\mu \nu} - h^{\mu \nu} \right)\left(\eta_{\mu \nu} + h_{\mu \nu} \right) \right)}[/math] [math]\;[/math] General relativity stress-energy tensor: [math]T_{\mu \nu} = \pm \left(\begin{matrix} -\rho & 0 & 0 & 0 \\ 0 & p & 0 & 0 \\ 0 & 0 & p & 0 \\ 0 & 0 & 0 & p \end{matrix} \right)[/math] [math]\;[/math] In spherical coordinates [math](ct, r, \theta, \phi)[/math] the Minkowski flat spacetime metric takes the form: [math]ds^{2} = -c^{2} dt^{2} + dr^{2} + r^{2} d\theta^{2} + r^{2} \sin^{2} \theta \; d\phi^{2}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric tensor: [math]T^{\alpha \beta} \left(x \right) = \pm \begin{pmatrix} -c^{2} dt^{2} & 0 & 0 & 0 \\ 0 & dr^{2} & 0 & 0 \\ 0 & 0 & r^{2} d\theta^{2} & 0 \\ 0 & 0 & 0 & r^{2} d\phi^{2} \end{pmatrix}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric: [math]\eta_{\mu \nu} = \pm \begin{pmatrix} -1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 0 & 0 & 0 & 1 \end{pmatrix}[/math] [math]\;[/math] General relativity Minkowski flat spacetime metric is equivalent to the inverse metric: [math]\boxed{\eta_{\mu \nu} = \eta^{\mu \nu}} \left(ref. 4 \right)[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf Wikipeda - General relativity - linearized gravity: (ref. 2) https://en.wikipedia.org/wiki/Linearized_gravity Wikipeda - General relativity: (ref. 3) https://en.wikipedia.org/wiki/General_relativity Wikipeda - Lorentz covariance: (ref. 4) https://en.wikipedia.org/wiki/Lorentz_covariance

Affirmative, revision complete. [math]\;[/math] Einstein's field equations: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = \frac{8 \pi G}{c^{4}} T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations in natural units: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = 8 \pi T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations Ricci tensor in natural units: [math]\boxed{ R_{\mu \nu} = 8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}[/math] [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{ \mathbb{R} }_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] General relativity Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] A massless gravitational wave is still massless on a Planck scale, and the result is still a tensor field. [math]\;[/math] Tensor field: (ref. 1,pg. 21, eq. 1.68) [math]T^{' \mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] The general relativity Ricci tensor is a tensor field: [math]\boxed{R_{\mu \nu} = T^{' \mu \nu} \left(x' \right)}[/math] [math]\;[/math] Lagrangian equation for a massless Planck graviton: [math]\boxed{\mathcal{L} = \underbrace{ \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right) }_{QG} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] General relativity and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric: [math]g_{\mu \nu} = \eta_{\mu \nu} + h_{\mu \nu}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 1: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity weak field limit spacetime metric and Planck quantum gravity identity 2: [math]\boxed{8 \pi T_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right) - \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right)}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf