Orion1

Affirmative, that is correct. [math]\;[/math] Einstein's field equations: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = \frac{8 \pi G}{c^{4}} T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations in natural units: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{1}{2} R g_{\mu \nu} = 8 \pi T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations Ricci tensor in natural units: [math]\boxed{ R_{\mu \nu} = 8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}[/math] [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{ \mathbb{R} }_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] General relativity Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] A massless gravitational wave is still massless on a Planck scale, and the result is still a tensor field. [math]\;[/math] Tensor field: (ref. 1, pg. 21, eq. 1.68) [math]T^{' \mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] The general relativity Ricci tensor is a tensor field: [math]\boxed{R_{\mu \nu} = T^{' \mu \nu} \left(x' \right)}[/math] [math]\;[/math] Lagrangian equation for a massless Planck graviton: [math]\boxed{\mathcal{L} = \underbrace{ \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right) }_{QG} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] General relativity and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R g_{\mu \nu} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] General relativity spacetime metric: [math]g_{\mu \nu} = \eta_{\mu \nu} + h_{\mu \nu}[/math] [math]\;[/math] General relativity spacetime metric and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{1}{2} R \left(\eta_{\mu \nu} + h_{\mu \nu} \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta} \left(x \right)}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf

[math]\;[/math] Affirmative, according to Wikipedia, The action S is given by: [math]S = -mc \int ds = \int L dt[/math] [math]\;[/math] Where L is the relativistic Lagrangian for a free particle: [math]L = -mc^{2} \sqrt{1 - \frac {v^{2}}{c^{2}}}[/math] [math]\;[/math] And my solution for the proper time relativistic Lagrangian for a free particle: [math]\boxed{\mathcal{L} = - \frac{m_{0} c^{2}}{\gamma\left(\dot{\mathbf{r}} \right)} = -m_{0} c^2 \sqrt{1 - \frac{\dot{\mathbf{r}}^2 \left(t \right)}{c^2}}}[/math] [math]\;[/math] [math]\;[/math] A free particle that encounters a gravity field potential will always form a closed path in x,y,z, and time dilation t, and invoke Keplers laws: [math]L = - \frac{m_0 c^2}{\gamma\left( \dot{\mathbf{r}} \right)} - V\left(\mathbf{r}, \dot{\mathbf{r}}, t \right)[/math] [math]\;[/math] [math]\mathbf{v} = \dot{\mathbf{r}} = \frac{d\mathbf{r}}{dt} = \left(\frac{dx}{dt} , \frac{dy}{dt} , \frac{dz}{dt} \right)[/math] [math]\;[/math] Except in the case for a hyperbolic trajectory with escape velocity, where the path integral is inflection curved at the point source for the gravitational field potential. [math]\;[/math] Because, It is implied that the Ricci tensor is still the classical theory of general relativity in this form. Affirmative, I think that you have answered your own question. [math]\;[/math] Einstein's field equations: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{R g_{\mu \nu}}{2} = \frac{8 \pi G}{c^{4}} T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations in natural units: [math]G_{\mu \nu} = R_{\mu \nu} - \frac{R g_{\mu \nu}}{2} = 8 \pi T_{\mu \nu}[/math] [math]\;[/math] Einstein's field equations Ricci tensor in natural units: [math]\boxed{ R_{\mu \nu} = 8 \pi T_{\mu \nu} + \frac{R g_{\mu \nu}}{2} }[/math] [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{ \mathbb{R} }_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] General relativity Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{8 \pi T_{\mu \nu} + \frac{R g_{\mu \nu}}{2}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] Conventional gravitational waves that are quantized below the Planck radius with a total Planck energy would be indistinguishable from what scientists refer to as gravitons. Absent a total Planck energy available to generate them, scientists will never observe them to add them to the standard model. [math]\;[/math] A massless gravitational wave is still massless on a Planck scale, and the result is still a tensor field. [math]\;[/math] Tensor field: (ref. 1,pg. 21, eq. 1.68) [math]T^{' \mu \nu} \left(x' \right) = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)[/math] [math]\;[/math] Lagrangian equation for a massless Planck graviton: [math]\boxed{\mathcal{L} = \underbrace{ \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right) }_{QG} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0}[/math] [math]\;[/math] General relativity and Planck quantum gravity identity: [math]\boxed{8 \pi T_{\mu \nu} + \frac{R g_{\mu \nu}}{2} = \Lambda^{\mu}_{\alpha} \Lambda^{\nu}_{\beta} T^{\alpha \beta}\left(x \right)}[/math] [math]\;[/math] Reference: Lorentz Group and Lorentz Invariance: (ref. 1) https://gdenittis.files.wordpress.com/2016/04/ayudantiavi.pdf

Relativistic Lagrangian Lorentz factor: (ref. 1, ref. 2, ref. 6) [math]dt = \gamma\left(\dot{\mathbf{r}} \right) d\tau[/math] [math]\;[/math] [math]\gamma\left(\dot{\mathbf{r}} \right) = \frac{dt}{d\tau} = \frac{1}{\sqrt{1 - \frac{\dot{\mathbf{r}}^2}{c^2}}}[/math] [math]\boxed{\gamma\left(\dot{\mathbf{r}} \right) = \frac{1}{\sqrt{1 - \frac{\dot{\mathbf{r}}^2}{c^2}}}}[/math] [math]\;[/math] [math]\dot{\mathbf{r}} = \frac{d\mathbf{r}}{dt}[/math] [math]\;[/math] Relativistic Lagrangian neutral particle total energy integration via substitution: (ref. 2) [math]E_{t} = m_0 c^2 \frac{dt}{d \tau} = \gamma\left(\dot{\mathbf{r}} \right) m_0 c^2 = \frac{m_0 c^2}{\sqrt {1 - \frac{\dot{\mathbf{r}}^2 \left(t \right)}{c^2}}} = m_0 c^2 + {1 \over 2} m_0 \dot{\mathbf{r}}^2 \left(t \right) + {3 \over 8} m_0 \frac{\dot{\mathbf{r}}^4 \left(t \right)}{c^2} + \cdots[/math] [math]\;[/math] Relativistic Lagrangian neutral particle total energy: (ref. 2) [math]\boxed{E_{t} = \frac{m_{0} c^2}{\sqrt{1 - \frac{\dot{\mathbf{r}}^2 \left(t \right)}{c^2}}}}[/math] [math]\;[/math] Classical neutral particle kinetic energy: [math]E_{k} = \frac{m_{0} v^{2}}{2} = \frac{m_{0}}{2} \frac{ds^{2}}{dt^{2}}[/math] [math]\boxed{E_{k} = \frac{m_{0}}{2} \frac{ds^{2}}{dt^{2}}}[/math] [math]\;[/math] Classical Lagrangian neutral particle kinetic energy: (ref. 4) [math]E_{k} = \frac{m_{0} g_{bc}}{2} \frac{\mathrm{d}\xi^{b}}{\mathrm{d}t} \frac{\mathrm{d}\xi^{c}}{\mathrm{d}t}[/math] [math]\;[/math] Relativistic Lagrangian neutral particle kinetic energy: [math]E_{k} = m_{0} c^{2} \left(\gamma\left(\dot{\mathbf{r}} \right) - 1 \right)[/math] [math]\;[/math] Newtons second law for neutral particle integration via substitution: (ref. 3) [math]\mathbf{F} = \frac{d \mathbf{p}}{dt} = \frac{d(m_{0} \mathbf{v})}{\mathrm{d}t} = m_{0} \frac{d\mathbf{v}}{\mathrm{d}t} = m_{0} \frac{ds}{dt^2}[/math] [math]\;[/math] Newtons second law for neutral particle: [math]\boxed{\mathbf{F} = m_{0} \frac{ds}{dt^2}}[/math] [math]\;[/math] Relativistic Newtons second law for neutral particle: [math]\boxed{\mathbf{F} = \gamma m_{0} \frac{ds}{dt^2}}[/math] [math]\;[/math] General relativity geodesic equation: (ref. 4) [math]\frac{d^{2}x^{\mu}}{dt^{2}} + \Gamma^{\mu}{}_{\alpha \beta} \frac{dx^{\alpha}}{dt} \frac{dx^{\beta}}{dt} = 0[/math] [math]\;[/math] Newtons second law in Lagrangian form for neutral particle: (ref. 5) [math]F^{a} = m_{0} \left(\frac{d^{2} \xi^{a}}{dt^{2}}+ \Gamma^{a}{}_{bc} \frac{d\xi^{b}}{dt} \frac{d\xi^{c}}{dt} \right)[/math] [math]\;[/math] Relativistic Newtons second law in Lagrangian form for neutral particle: [math]\boxed{F^{a} = \gamma\left(\dot{\mathbf{r}} \right) m_{0} \left( \frac{d^{2} \xi^{a}}{dt^{2}} + \Gamma^{a}{}_{bc} \frac{d\xi^{b}}{dt} \frac{d\xi^{c}}{dt} \right)}[/math] [math]\;[/math] Relativistic Lagrangian for a neutral particle: [math]\mathcal{L} = E_{k} - E_{p}[/math] [math]\;[/math] [math]\boxed{\mathcal{L} = m_{0} c^{2} \left(\gamma\left(\dot{\mathbf{r}} \right) - 1 \right) - E_{p}}[/math] [math]\;[/math] Relativistic Lagrangian integration via substitution: [math]\mathcal{L} = \sum_{1}^{n} E_{k}\left(n \right) - \sum_{1}^{n} E_{p}\left(n \right) = \sum_{1}^{n} \mathcal{L}\left(n \right) = 0[/math] [math]\;[/math] Relativistic Lagrangian: [math]\boxed{\mathcal{L} = \sum_{1}^{n} \mathcal{L}\left(n \right) = 0}[/math] [math]\;[/math] The Lagrangian equation integration via substitution: [math]\mathcal{L} = \sum_{1}^{n} \mathcal{L}\left(n \right) = \underbrace{\mathbb{R}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0 \; \; \; \; \; \; n = 5[/math] The Lagrangian equation: [math]\boxed{\mathcal{L} = \underbrace{\mathbb{R}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0} \; \; \; \; \; \; n = 5[/math] [math]\;[/math] [math]\;[/math] I think that this revision has compensated for the 4 momentum and 4 velocity under general relativity with respect to proper time [math]\tau[/math], through the Lorentz factor. The 4 momentum and 4 velocity is intrinsic to general relativity as required by four-dimensional space-time. [math]\;[/math] I note two general relativity geodesic equation forms, a spacial and a temporal form. [math]\;[/math] General relativity spacial geodesic equation: (ref. 4) [math]\frac{d^{2}x^{\mu}}{ds^{2}} + \Gamma^{\mu}{}_{\alpha \beta} \frac{dx^{\alpha}}{ds} \frac{dx^{\beta}}{ds} = 0[/math] [math]\;[/math] General relativity temporal geodesic equation: (ref. 4) [math]\frac{d^{2}x^{\mu}}{dt^{2}} + \Gamma^{\mu}{}_{\alpha \beta} \frac{dx^{\alpha}}{dt} \frac{dx^{\beta}}{dt} = 0[/math] [math]\;[/math] Do you agree with this mathematical symbolic formalism derivation revision for the formal Lagrangian equation? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - The Lorentz factor: (ref. 1) https://en.wikipedia.org/wiki/Lorentz_factor Wikipedia - Relativistic Lagrangian mechanics: (ref. 2) https://en.wikipedia.org/wiki/Relativistic_Lagrangian_mechanics#Coordinate_formulation Wikipedia - Newtons second law: (ref. 3) https://en.wikipedia.org/wiki/Newton's_laws_of_motion#Newton's_second_law Wikipedia - Geodesics in general relativity: (ref. 4) https://en.wikipedia.org/wiki/Geodesics_in_general_relativity Wikipedia - Newtons second law Lagrangian form: (ref. 5) https://en.wikipedia.org/wiki/Lagrangian_mechanics#From_Newtonian_to_Lagrangian_mechanics Wikipedia - Four-velocity: (ref. 6) https://en.wikipedia.org/wiki/Four-velocity

[math]\;[/math] Please consider Newtons second law and the relativistic Newtons second law for a moment. [math]\;[/math] [math]\gamma[/math] - Lorentz factor [math]\;[/math] Newtons second law: (ref. 1) [math]\mathbf{F} = \frac{d \mathbf{p}}{dt} = \frac{d\left(m \mathbf{v} \right)}{\mathrm{d}t} = m {\frac{d\mathbf{v}}{\mathrm{d}t}} = m \frac{ds}{dt^2}[/math] [math]\boxed{\mathbf{F} = m \frac{ds}{dt^2}}[/math] [math]\;[/math] Relativistic Newtons second law: [math]\boxed{\mathbf{F} = \gamma m \frac{ds}{dt^2}}[/math] [math]\;[/math] Newtons second law in Lagrangian form: (ref. 2) [math]F^{a} = m \left( \frac{d^{2} \xi^{a}}{dt^{2}}+ \Gamma^{a}{}_{bc} \frac{d\xi^{b}}{dt} \frac{d\xi^{c}}{dt} \right)[/math] [math]\;[/math] Relativistic Newtons second law in Lagrangian form: [math]\boxed{F^{a} = \gamma m \left( \frac{d^{2} \xi^{a}}{dt^{2}}+ \Gamma^{a}{}_{bc} \frac{d\xi^{b}}{dt} \frac{d\xi^{c}}{dt} \right)}[/math] [math]\;[/math] Thus including all classical nonrelativistic Lagrangian mechanics into relativistic Lagrangian mechanics? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Newtons second law: (ref. 1) https://en.wikipedia.org/wiki/Newton's_laws_of_motion#Newton's_second_law Wikipedia - Newtons second law in Lagrangian form: (ref. 2) https://en.wikipedia.org/wiki/Lagrangian_mechanics#From_Newtonian_to_Lagrangian_mechanics

I request to initiate a thread on what I only know as the Lagrangian equation. The original published scientific paper derivation proof is unknown to me. [math]\;[/math] Lagrangian equation: [math]\mathcal{L} = \underbrace{\mathbb{R}}_{GR} - \overbrace{\underbrace{\frac{1}{4} F_{\mu \nu} F^{\mu \nu}}_{Yang-Mills}}^{Maxwell} + \underbrace{i \overline{\psi} \gamma^\mu D_\mu \psi}_{Dirac} + \underbrace{|D_\mu h|^2-V\left(|h| \right)}_{Higgs} + \underbrace{h \overline{\psi} \psi}_{Yukawa} = 0[/math] [math]\;[/math] This Lagrangian equation appears to represent a massless field tensor fundamental field interaction Lagrangian combination zero summation action in natural units. [math]\;[/math] The classical General Relativity Lagrangian tensor appears to describe a Ricci tensor on a smooth spacially flat Ricci maniold [math]\mathbb{R}[/math]. (ref. 1, ref. 2) [math]\;[/math] The massless field tensor Yang-Mills Maxwell Lagrangian term represents at the core of the unification of the electromagnetic force and weak forces [math](U(1) \times SU(2))[/math] and quantum chromodynamics, the theory of the strong force [math](SU(3))[/math] and predicts all the massless spin one Maxwells equations. (ref. 3, ref. 4) [math]\;[/math] The massless field tensor Dirac Lagrangian term is a relativistic wave equation that describes all spin one-half particle interactions. (ref. 5) [math]\;[/math] The massless field tensor Higgs Lagrangian term describes all spin zero Higgs field interactions. (ref. 6) [math]\;[/math] The massless field tensor Yukawa coupling interaction term describes the interaction between a massless spin zero scalar field [math]\phi[/math] and a massless spin one-half Dirac field [math]\psi[/math] (ref. 7) [math]\;[/math] Do you know where the original published scientific paper derivation proof is located? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Ricci curvature: (ref. 1) https://en.wikipedia.org/wiki/Ricci_curvature Wikipedia - Riemannian manifold: (ref. 2) https://en.wikipedia.org/wiki/Riemannian_manifold Wikipedia - Maxwells equations: (ref. 3) https://en.wikipedia.org/wiki/Maxwell's_equations#Formulation_in_SI_units_convention Stackexchange - derivation of maxwells equations from field tensor lagrangian: (ref. 4) https://physics.stackexchange.com/questions/3005/derivation-of-maxwells-equations-from-field-tensor-lagrangian Wikipedia - Dirac Lagrangian: (ref. 5) https://en.wikipedia.org/wiki/Dirac_equation#Dirac_Lagrangian Wikipedia - Higgs field: (ref. 6) https://simple.wikipedia.org/wiki/Higgs_field Wikipedia - Yukawa Lagrangian: (ref. 7) https://en.wikipedia.org/wiki/Yukawa_interaction#The_action

Physics: The branch of science concerned with the nature and properties of matter and energy. The subject matter of physics, distinguished from that of chemistry and biology, includes mechanics, heat, light and other radiation, sound, electricity, magnetism, and the structure of atoms. The physical properties and phenomena of something. Physical: Relating to things perceived through the senses as opposed to the mind; tangible or concrete. "everything physical in the universe" We are defining the Original Posters semantic definitions?

Solar star system average planetary mass: (ref. 1) [math]\boxed{M_{ap} = \frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n \right)} \; \; \; \; \; \; n_p = 8[/math] [math]\;[/math] Observable Universe total planetary number based upon solar star system: [math]\boxed{N_p = \frac{\Omega_p \pi^3 k_B^4}{12 M_{ap} c^2 \left(\hbar H_0 \right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}} \right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}} \right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}} \right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}} \right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}} \right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\phi,t}}{T_{\phi}} \right]^3 \right)}[/math] [math]\;[/math] Observable Universe total planetary number based upon solar star system: [math]\boxed{N_p = 3.536 \cdot 10^{23} \; \text{planets}}[/math] [math]\;[/math] Wikipedia observable universe total planetary number: [math]\boxed{N_p = \left(2.000 \cdot 10^{23} \rightarrow 3.200 \cdot 10^{23} \right) \; \text{planets}}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Wikipedia - Planetary mass: (ref. 1) https://en.wikipedia.org/wiki/Planetary_mass#Values_from_the_DE405_ephemeris

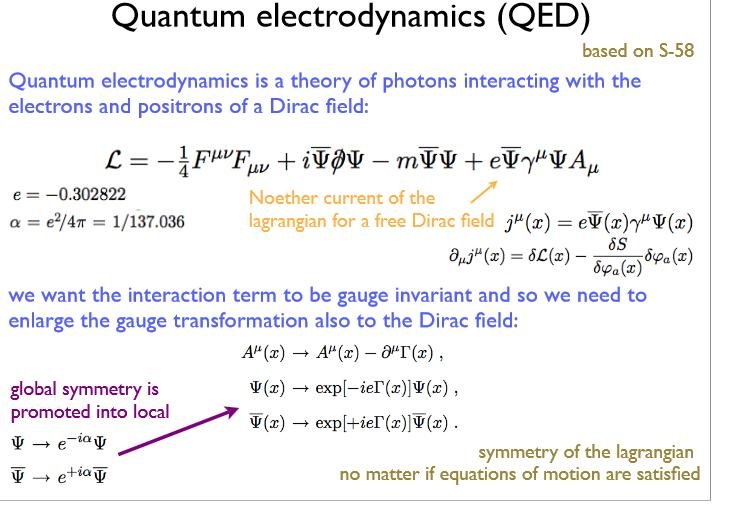
Planck satellite cosmological parameters: (ref. 1, pg. 11) [math]\Omega_{dm} = 0.268[/math] [math]\Omega_{b} = 0.0495[/math] [math]\Omega_{\Lambda} = 0.6825[/math] [math]\Omega_{\nu} = 1.259 \cdot 10^{-3}[/math] [math]\Omega_{\gamma} = 5.012 \cdot 10^{-5}[/math] [math]\;[/math] Symbolic identity key: [math]n_{s}[/math] - spin states total number [math]N_{s}[/math] - species total number [math]N_{n}[/math] - total effective degeneracy number [math]\text{if } n_{s} \geq N_{s} \text{ then } N_{n} = n_{s}[/math] [math]\text{if } n_{s} \leq N_{s} \text{ then } N_{n} = N_{s}[/math] [math]\;[/math] [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & \neq 0 & \phi \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & \neq 0 & \nu \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} \\ \end{array}[/math] [math]\;[/math] Total stellar class number: (ref. 2) [math]n_c = 7[/math] key: 1 O, 2 B, 3 A, 4 F, 5 G, 6 K, 7 M [math]\Omega_f[/math] - main sequence stars stellar class fraction [math]N_s[/math] - total observable stellar number [math]M_s[/math] - Main-sequence mass [math]\;[/math] Observable Universe average stellar mass: [math]M_{as} = \frac{1}{N_s} \sum_{n = 1}^{n_c} \Omega_f\left(n\right) N_s M_s\left(n\right) = \sum_{n = 1}^{n_c} \Omega_f\left(n\right) M_s\left(n\right) = 0.219 \cdot M_{\odot} \rightarrow 0.595 \cdot M_{\odot}[/math] [math]\boxed{M_{as} = \sum_{n = 1}^{n_c} \Omega_f\left(n\right) M_s\left(n\right)} \; \; \; n_c = 7[/math] [math]\boxed{M_{as} = \left(0.219 \rightarrow 0.595\right) \cdot M_{\odot}}[/math] Observable Universe average stellar mass upper bound limit: [math]\boxed{M_{as} = 1.184 \cdot 10^{30} \; \text{kg}}[/math] Observable Universe average stellar mass: (ref. 3, pg. 20) [math]M_{as} = 0.6 \cdot M_{\odot} = 1.193 \cdot 10^{30} \; \text{kg}[/math] [math]M_{as} = 1.193 \cdot 10^{30} \; \text{kg}[/math] [math]\;[/math] Observable Universe stellar baryon composition: (ref. 4, pg. 3) [math]\Omega_s = \left(\Omega_{ms} + \Omega_{wd} + \Omega_{ns}\right) = 2.460 \cdot 10^{-3}[/math] [math]\Omega_s = 2.460 \cdot 10^{-3}[/math] [math]---[/math] Milky Way galaxy mass: (ref. 5, pg. 1) [math]M_{mw} = 1.260 \cdot 10^{12} \cdot M_{\odot} = 2.506 \cdot 10^{42} \; \text{kg}[/math] [math]\boxed{M_{mw} = 2.506 \cdot 10^{42} \; \text{kg}}[/math] [math]---[/math] Redshift parameter at photon decoupling time: [math]z = 1090.43[/math] [math]\;[/math] Cosmic photon background radiation temperature at present time: [math]T_{\gamma} = 2.72548 \; \text{K}[/math] [math]\;[/math] Cosmic photon background radiation temperature at photon decoupling time: [math]T_{\gamma,t} = T_{\gamma} \left(1 + z\right) = 2974.67 \; \text{K}[/math] [math]\boxed{T_{\gamma,t} = 2974.67 \; \text{K}}[/math] [math]\;[/math] Cosmic neutrino background radiation temperature at present time: (ref. 6, pg. 44, eq. 220) [math]T_{\nu} = \left(\frac{4}{11}\right)^{\frac{1}{3}} T_{\gamma} = 1.945 \; \text{K}[/math] [math]\boxed{T_{\nu} = 1.945 \; \text{K}}[/math] [math]\;[/math] Cosmic neutrino background radiation temperature at neutrino decoupling time: (ref. 7) [math]T_{\nu,t} = 1 \cdot 10^{10} \; \text{K}[/math] [math]\;[/math] Observable Universe dark matter scalar particle temperature is equivalent to cosmic neutrino background radiation temperature: [math]\boxed{T_{\phi} = T_{\nu}}[/math] [math]\;[/math] Observable Universe dark matter scalar particle decoupling temperature is equivalent to cosmic neutrino background radiation decoupling temperature: [math]\boxed{T_{\phi,t} = T_{\nu,t}}[/math] [math]\;[/math] Cosmic scalar particle dark energy background radiation temperature: [math]\boxed{T_{\Lambda} = \frac{}{k_{B}} \left(\frac{45 \Omega_{\Lambda} H_0^2 \hbar^3 c^5}{4 G N_{\Lambda} \pi^3}\right)^{1/4}}[/math] [math]\boxed{T_{\Lambda} = 35.013 \; \text{K}}[/math] [math]\;[/math] Observable Universe Cosmological Constant: [math]\Lambda_s = \frac{3 \Omega_{\Lambda} H_0^2}{c^2} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] [math]\;[/math] Observable Universe scalar particle dark energy Cosmological Constant integration via substitution: [math]\Lambda_s = \frac{3 H_0^2}{c^2} \left(\frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{45 H_0^2 \hbar^3 c^5}\right) = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] [math]\;[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7}}[/math] [math]\;[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = 1.180 \cdot 10^{-52} \; \text{m}^{-2}}[/math] [math]\;[/math] Dark matter density: [math]\rho_{dm} = \frac{3 \Omega_{dm} H_0^2}{8 \pi G}[/math] Baryonic density: [math]\rho_{b} = \frac{3 \Omega_{b} H_0^2}{8 \pi G}[/math] Dark energy density: [math]\rho_{\Lambda} = \frac{3 \Omega_{\Lambda} H_0^2}{8 \pi G}[/math] [math]\;[/math] Bose-Einstein total dark matter scalar particle distribution constant: [math]\boxed{C_{\phi} = \frac{4 G N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{3 \Omega_{\phi} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\phi} = 3.640 \cdot 10^{-4}}[/math] [math]\;[/math] Fermi-Dirac total neutrino distribution constant: [math]\boxed{C_{\nu} = \frac{4 G N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{3 \Omega_{\nu} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\nu} = 0.236}[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter density: [math]\boxed{\rho_{\phi} = \frac{N_{\phi} \pi^2 \left(k_B T_{\phi}\right)^4}{2 C_{\phi} \hbar^3 c^5}}[/math] [math]\;[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter density: [math]\boxed{\rho_{b} = \frac{N_{\gamma} \pi^2 \left(k_B T_{\gamma}\right)^4}{30 \hbar^3 c^5} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) + \frac{N_{\nu} \pi^2 \left(k_B T_{\nu}\right)^4}{2 C_{\nu} \hbar^3 c^5} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right)}[/math] [math]\;[/math] Bose-Einstein scalar particle dark energy density: [math]\boxed{\rho_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{30 \hbar^3 c^5}}[/math] [math]\;[/math] Observable Universe total scalar particle dark matter and photon and neutrino co-moving volumes: [math]V_{\phi} = \frac{4 \pi R_{\phi}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]\right)^3[/math] [math]V_{\gamma} = \frac{4 \pi R_{\gamma}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]\right)^3[/math] [math]V_{\nu} = \frac{4 \pi R_{\nu}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]\right)^3[/math] Observable Universe scalar particle dark matter and scalar particle dark energy and neutrino total co-moving volumes are equivalent: [math]\boxed{V_{\phi} = V_{\Lambda} = V_{\nu}}[/math] [math]\;[/math] Bose-Einstein scalar particle dark matter total mass: [math]\boxed{\rho_{\phi} V_{\phi} = \frac{N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{12 C_{\phi} c^2 \left(\hbar H_0\right)^3} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3}[/math] [math]\;[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter relative composition total mass: [math]\boxed{\rho_{b} V_{b} = \frac{N_{\gamma} \pi^3 \left(k_B T_{\gamma}\right)^4}{180 c^2 \left(\hbar H_0\right)^3} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{12 C_{\nu} c^2 \left(\hbar H_0\right)^3} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3}[/math] [math]\;[/math] Bose-Einstein scalar particle dark energy total mass: [math]\boxed{\rho_{\Lambda} V_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{180 c^2 \left(\hbar H_0\right)^3} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3}[/math] [math]\;[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{dm} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] [math]\;[/math] Observable Universe total critical mass: [math]M_{c} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{dm} V_{dm} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\boxed{M_c = \frac{c^3}{16 G H_0} \left(\left(\Omega_{dm} + \Omega_{\Lambda}\right) \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \Omega_b \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3\right)}[/math] [math]\boxed{M_c = 1.179 \cdot 10^{56} \; \text{kg}}[/math] [math]\;[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{\phi} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] [math]\;[/math] Observable Universe scalar particle dark matter and scalar particle dark energy composition total mass: [math]M_{u} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{\phi} V_{\phi} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\;[/math] [math]\boxed{M_u = \frac{\pi^3 k_B^4}{12 c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3\right)}[/math] [math]\boxed{M_u = 1.179 \cdot 10^{56} \; \text{kg}}[/math] [math]\;[/math] Observable Universe total energy: [math]E_u = M_u c^2[/math] [math]\boxed{E_u = \frac{\pi^3 k_B^4}{12 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3\right)}[/math] [math]\boxed{E_u = 1.060 \cdot 10^{73} \; \text{j}}[/math] [math]\;[/math] Observable Universe total stellar number: [math]\boxed{N_s = \frac{\Omega_s \pi^3 k_B^4}{12 M_{as} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3\right)}[/math] [math]\;[/math] Observable Universe total stellar number: [math]\boxed{N_s = 2.453 \cdot 10^{23} \; \text{stars}}[/math] [math]\;[/math] Wikipedia observable universe total stellar number: (ref. 8) [math]N_s = 3.000 \cdot 10^{23} \; \text{stars}[/math] [math]\;[/math] Observable Universe total galaxy number: [math]\boxed{N_g = \frac{\Omega_b \pi^3 k_B^4}{12 M_{mw} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3\right)}[/math] [math]\;[/math] Observable Universe total galaxy number: [math]\boxed{N_g = 2.330 \cdot 10^{12} \; \text{galaxies}}[/math] [math]\;[/math] Wikipedia observable universe total galaxy number: (ref. 9) [math]N_g = 2.000 \cdot 10^{12} \; \text{galaxies}[/math] [math]\;[/math] Observable Universe stars per galaxy average number: [math]\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] [math]\boxed{\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}}}[/math] [math]\boxed{\frac{N_s}{N_g} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}}[/math] [math]\;[/math] Wikipedia stars per galaxy average number: (ref. 8, ref. 9) [math]\frac{N_s}{N_g} = 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] [math]\;[/math] Wikipedia Milky Way galaxy total stellar number: (ref. 10) [math]\frac{N_s}{N_g} = 2.500 \cdot 10^{11} \pm 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] [math]\;[/math] Observable Universe planetary composition: (ref. 4, pg. 3) [math]\Omega_p = 1 \cdot 10^{-6}[/math] [math]\;[/math] Wikipedia Milky Way galaxy total planetary number: (ref. 10, ref. 11) [math]\frac{N_p}{N_g} = \left(1.000 \cdot 10^{11} \rightarrow 1.600 \cdot 10^{11}\right) \; \frac{\text{planets}}{\text{galaxy}}[/math] [math]\;[/math] Observable Universe average planetary mass: [math]\boxed{M_{ap} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{N_g}{N_p}\right)}[/math] [math]\boxed{M_{ap} = \left(52.974 \rightarrow 84.758\right) \cdot M_{\oplus}}[/math] [math]\;[/math] Solar star system average planetary mass: (ref. 12) [math]\boxed{M_{ap} = \frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{M_{ap} = 55.855 \cdot M_{\oplus}}[/math] [math]\;[/math] Milky Way galaxy total planetary number based upon solar star system: [math]\boxed{\frac{N_p}{N_g} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)\right)^{-1}} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{\frac{N_p}{N_g} = 1.518 \cdot 10^{11} \; \frac{\text{planets}}{\text{galaxy}}}[/math] [math]\;[/math] Wikipedia Milky Way galaxy total planetary number: (ref. 10, ref. 11) [math]\frac{N_p}{N_g} = \left(1.000 \cdot 10^{11} \rightarrow 1.600 \cdot 10^{11}\right) \; \frac{\text{planets}}{\text{galaxy}}[/math] [math]\;[/math] Milky Way galaxy total planetary number based upon solar star system: [math]\boxed{\frac{N_p}{N_g} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)\right)^{-1}} \; \; \; \; \; \; n_p = 8[/math] [math]\;[/math] Observable Universe stars per galaxy average number: [math]\boxed{\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}}}[/math] [math]\;[/math] Observable Universe average stellar mass: [math]\boxed{M_{as} = \sum_{n = 1}^{n_c} \Omega_f\left(n\right) M_s\left(n\right)} \; \; \; n_c = 7[/math] [math]\;[/math] Observable Universe planets per star average number based upon solar star system integration via substitution: [math]\frac{N_p}{N_s} = \left(\frac{N_p}{N_g}\right)\left(\frac{N_g}{N_s}\right) = \left[\frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)\right)^{-1}\right]\left(\frac{\Omega_b M_{as}}{\Omega_s M_{mw}}\right)[/math] [math]\frac{N_p}{N_s} = \left[\frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)\right)^{-1}\right]\left(\frac{\Omega_b}{\Omega_s M_{mw}}\right)\left(\sum_{n = 1}^{n_c} \Omega_f\left(n\right) M_s\left(n\right)\right) = \frac{\Omega_p}{\Omega_s} \left(\sum_{n = 1}^{n_c} \Omega_f\left(n\right) M_s\left(n\right)\right)\left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_p\left(n\right)\right)^{-1}[/math] [math]\;[/math] Observable Universe planets per star average number based upon solar star system: [math]\boxed{\frac{N_p}{N_s} = \frac{\Omega_p}{\Omega_s} \left(\sum_{n = 1}^{n_c} \Omega_{f}\left(n\right) M_{s}\left(n\right)\right)\left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_{p}\left(n\right)\right)^{-1}} \; \; \; \; \; \; n_c = 7, n_p = 8[/math] [math]\;[/math] [math]\boxed{\frac{N_p}{N_s} = 1.443 \; \frac{\text{planets}}{\text{star}}}[/math] [math]\;[/math] Wikipedia planets per star average number: [math]\boxed{\frac{N_p}{N_s} = \left(0.667 \rightarrow 1.067\right) \; \frac{\text{planets}}{\text{star}}}[/math] [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Is there anything else that you want to see quantified based upon this model for observational comparison? [math]\;[/math] Reference: Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf Wikipedia - Stellar classification - Harvard spectral classification: (ref. 2) https://en.wikipedia.org/wiki/Stellar_classification Harvard_spectral_classification (insert number symbol) On The Mass Distribution Of Stars...: (ref. 3) http://www.doiserbia.nb.rs/img/doi/1450-698X/2006/1450-698X0672017N.pdf The Cosmic Energy Inventory: (ref. 4) http://arxiv.org/pdf/astro-ph/0406095v2.pdf Mass models of the Milky Way: (ref. 5) http://arxiv.org/pdf/1102.4340v1 PHYS: 652 Cosmic Inventory I: Radiation: (ref. 6) http://www.nicadd.niu.edu/~bterzic/PHYS652/Lecture_09.pdf Wikipedia - neutrino decoupling: (ref. 7) https://en.wikipedia.org/wiki/Neutrino_decoupling Wikipedia - Observable universe total stellar number: (ref. 8) https://en.wikipedia.org/wiki/Star#Distribution Wikipedia - Galaxy: (ref. 9) https://en.wikipedia.org/wiki/Galaxy Wikipedia - Milky Way Galaxy: (ref. 10) https://en.wikipedia.org/wiki/Milky_Way Space.com - 160 Billion Alien Planets May Exist in Our Milky Way Galaxy: (ref. 11) https://www.space.com/14200-160-billion-alien-planets-milky-galaxy.html Wikipedia - Planetary mass: (ref. 12) https://en.wikipedia.org/wiki/Planetary_mass#Values_from_the_DE405_ephemeris (source code errors corrected on 01-18-2019)

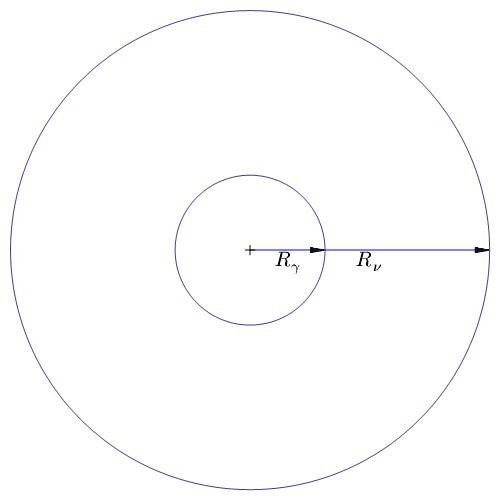
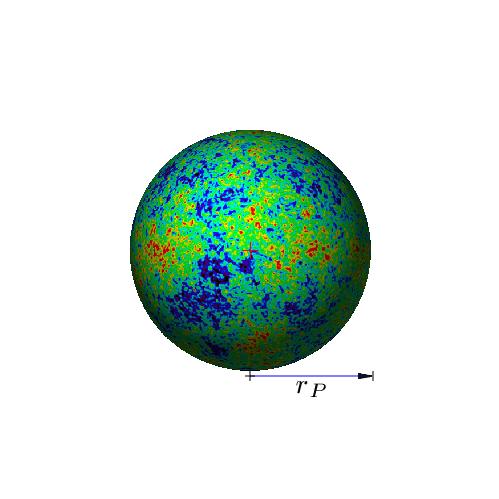
Planck satellite cosmological parameters: (ref. 1) [math]\Omega_{dm} = 0.268[/math] [math]\Omega_{b} = 0.0495[/math] [math]\Omega_{\Lambda} = 0.6825[/math] [math]\Omega_{\nu} = 1.259 \cdot 10^{-3}[/math] [math]\Omega_{\gamma} = 5.012 \cdot 10^{-5}[/math] [math]\;[/math] Dark matter scalar particle composition is equivalent to dark matter composition: [math]\boxed{\Omega_{\phi} = \Omega_{dm}}[/math] [math]\;[/math] Planck mass: (ref. 2) [math]m_{P} = \sqrt{\frac{\hbar c}{G}}[/math] [math]\;[/math] Planck temperature (ref. 3) [math]T_{P} = \frac{E_{P}}{k_{B}} = \frac{m_{P} c^{2}}{k_{B}} = \left(\sqrt{\frac{\hbar c}{G}}\right) \frac{c^2}{k_{B}} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}} = 1.417 \cdot 10^{32} \; \text{K}[/math] [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\boxed{T_{P} = 1.417 \cdot 10^{32} \; \text{K}}[/math] [math]\;[/math] Planck radius: (ref. 4) [math]r_P = \sqrt{\frac{\hbar G}{c^{3}}}[/math] [math]\;[/math] Planck volume: (ref. 5) [math]V_{P} = \frac{4 \pi R_{P}^{3}}{3} = \frac{4 \pi}{3} \left(\sqrt{\frac{\hbar G}{c^{3}}}\right)^{3} = \frac{4 \pi}{3} \sqrt{\frac{\left(\hbar G\right)^{3}}{c^{9}}} = 1.768 \cdot 10^{-104} \; \text{m}^3[/math] [math]\;[/math] [math]\boxed{V_{P} = \frac{4 \pi}{3} \sqrt{\frac{\left(\hbar G\right)^{3}}{c^{9}}}}[/math] [math]\boxed{V_{P} = 1.768 \cdot 10^{-104} \; \text{m}^3}[/math] [math]\;[/math] Observable Universe total energy at present time: [math]\boxed{E_u = \frac{\pi^3 k_B^4}{12 \left(\hbar H_{0}\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_u = 1.060 \cdot 10^{73} \; \text{j}}[/math] [math]\;[/math] All particle peak radiation temperatures are equivalent to Planck temperature at Planck time: [math]\boxed{T_{\phi,t_P} = T_{\gamma,t_P} = T_{\nu,t_P} = T_P}[/math] Dark energy scalar particle peak radiation temperature is non-zero at Planck time: [math]\boxed{T_{\Lambda,t_P} \neq 0}[/math] [math]\;[/math] Present time radial metric decoupling temperature redshift parameter global quantizations remain the same: [math]\boxed{\ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right] \; \; \; \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right] \; \; \; \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]}[/math] [math]\;[/math] Observable Universe total energy at Planck time integration via substitution: [math]E_{u}\left(T_P\right) = \frac{\pi^3 k_B^4}{12 \left(\hbar H_{0}\right)^3} \left(\frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}\right)^{4} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\;[/math] Observable Universe total energy at Planck time: [math]\boxed{E_{u}\left(T_P\right) = \frac{\pi^3 c^{10}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_{u}\left(T_P\right) = 8.439 \cdot 10^{199} \; \text{j}}[/math] [math]\;[/math] Observable Universe total mass at Planck time: [math]M_{u}\left(T_P\right) = \frac{E_{u}\left(T_P\right)}{c^{2}} = \frac{\pi^3 c^{8}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\boxed{M_{u}\left(T_P\right) = \frac{\pi^3 c^{8}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{M_{u}\left(T_P\right) = 9.390 \cdot 10^{182} \; \text{kg}}[/math] [math]\;[/math] Observable Universe total energy density at Planck time integration via substitution: [math]\epsilon_{u}\left(T_{P}\right) = \frac{E_{u}\left(T_{P}\right)}{V_{P}} = \frac{\pi^3 c^{10}}{12 \hbar G^{2} H_{0}^3} \left(\frac{3}{4 \pi} \sqrt{\frac{c^{9}}{\left(\hbar G\right)^{3}}}\right) \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\;[/math] Observable Universe total energy density at Planck time: [math]\boxed{\epsilon_{u}\left(T_{P}\right) = \frac{\pi^{2}}{16 H_{0}^{3}} \sqrt{\frac{c^{29}}{\hbar^{5} G^{7}}} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\;[/math] [math]\boxed{\epsilon_{u}\left(T_{P}\right) = 4.772 \cdot 10^{303} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] Planck energy density integration via substitution: [math]\epsilon_{P} = \frac{E_{P}}{V_{P}} = \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)\left(\frac{3}{4 \pi} \sqrt{\frac{c^{9}}{\left(\hbar G\right)^{3}}}\right) = \frac{3 c^{7}}{4 \pi \hbar G^{2}} = 1.106 \cdot 10^{113} \; \frac{\text{j}}{\text{m}^{3}}[/math] [math]\;[/math] Planck energy density: (ref. 5) [math]\boxed{\epsilon_{P} = \frac{3 c^{7}}{4 \pi \hbar G^{2}}}[/math] [math]\boxed{\epsilon_{P} = 1.106 \cdot 10^{113} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] Planck vacuum energy density at Planck time: (ref. 6) [math]\epsilon_{vac} = \frac{\epsilon_{P}}{2} = \frac{3 c^{7}}{8 \pi \hbar G^{2}} = 5.531 \cdot 10^{112} \; \frac{\text{j}}{\text{m}^{3}}[/math] [math]\boxed{\epsilon_{vac} = \frac{3 c^{7}}{8 \pi \hbar G^{2}}}[/math] [math]\boxed{\epsilon_{vac} = 5.531 \cdot 10^{112} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] Planck time: (ref. 7) [math]t_{P} = \sqrt{\frac{\hbar G}{c^{5}}}[/math] [math]\boxed{t_{P} = 5.391 \cdot 10^{-44} \; \text{s}}[/math] [math]\;[/math] Particle interaction rate is equivalent to Hubble Parameter at Planck interaction time: [math]\boxed{\Gamma_{t} = n \langle \sigma v \rangle = H_{t}}[/math] [math]\Gamma_{P} = H_{P} = \frac{}{t_{P}} = \sqrt{\frac{c^{5}}{\hbar G}} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}[/math] Planck particle interaction rate at Planck interaction time: [math]\boxed{\Gamma_{P} = \sqrt{\frac{c^{5}}{\hbar G}}}[/math] [math]\;[/math] [math]\boxed{\Gamma_{P} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}}[/math] [math]\;[/math] Dark matter scalar particle interaction rate at scalar particle Planck time: [math]\boxed{\Gamma_{\phi,t_P} = 2 \left(k_B T_{\phi,t_P}\right)^2 \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] Planck temperature: (ref. 3) [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] Dark matter scalar particle interaction rate at scalar particle Planck time integration via substitution: [math]\Gamma_{\phi,t_P} = 2 \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}} = \frac{2 \hbar c^{5}}{G} \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}} = 2 \sqrt{\frac{N_{\phi} \pi^3 c^{5}}{3 C_{\phi} \Omega_{\phi,t_P} \hbar G}}[/math] Dark matter scalar particle interaction rate at scalar particle Planck time: [math]\boxed{\Gamma_{\phi,t_P} = 2 \sqrt{\frac{N_{\phi} \pi^3 c^{5}}{3 C_{\phi} \Omega_{\phi,t_P} \hbar G}}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{\Gamma_{\phi,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] Photon interaction rate at photon Planck time: [math]\boxed{\Gamma_{\gamma,t_P} = \frac{4 \left(k_B T_{\gamma,t_P}\right)^2}{3} \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] Planck temperature: (ref. 3) [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] Photon interaction rate at photon Planck time integration via substitution: [math]\Gamma_{\gamma,t_P} = \frac{4}{3} \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}} = \frac{4}{3} \left(\frac{\hbar c^{5}}{G}\right) \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}} = \frac{4}{3} \sqrt{\frac{N_{\gamma} \pi^3 c^{5}}{5 \Omega_{\gamma,t_P} \hbar G}}[/math] [math]\;[/math] Photon interaction rate at photon Planck time: [math]\boxed{\Gamma_{\gamma,t_P} = \frac{4}{3} \sqrt{\frac{N_{\gamma} \pi^3 c^{5}}{5 \Omega_{\gamma,t_P} \hbar G}}} \; \; \; m_{\gamma} = 0[/math] [math]\boxed{\Gamma_{\gamma,t_P} = 1.230 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time: [math]\boxed{\Gamma_{\nu,t_P} = 2 \left(k_B T_{\nu,t_P}\right)^2 \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Planck temperature: (ref. 3) [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time integration via substitution: [math]\Gamma_{\nu,t_P} = 2 \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}} = 2 \left(\frac{\hbar c^{5}}{G}\right) \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}} = 2 \sqrt{\frac{N_{\nu} \pi^3 c^5}{3 C_{\nu} \Omega_{\nu,t_P} \hbar G}}[/math] Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time: [math]\boxed{\Gamma_{\nu,t_P} = 2 \sqrt{\frac{N_{\nu} \pi^3 c^5}{3 C_{\nu} \Omega_{\nu,t_P} \hbar G}}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{\Gamma_{\nu,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Particle interaction rate summary: Planck particle interaction rate at Planck interaction time: [math]\boxed{\Gamma_{P} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}}[/math] Dark matter scalar particle interaction rate at scalar particle Planck time: [math]\boxed{\Gamma_{\phi,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\phi} \neq 0[/math] Photon interaction rate at photon Planck time: [math]\boxed{\Gamma_{\gamma,t_P} = 1.230 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\gamma} = 0[/math] Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time: [math]\boxed{\Gamma_{\nu,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Planck particle strong decoupling time: (ref. 7) [math]t_{P} = \sqrt{\frac{\hbar G}{c^{5}}}[/math] [math]\boxed{t_{P} = 5.391 \cdot 10^{-44} \; \text{s}}[/math] [math]\;[/math] Dark matter scalar particle strong decoupling time: [math]\boxed{t_{\phi,t} = \frac{}{2} \sqrt{\frac{3 C_{\phi} \Omega_{\phi,t_P} \hbar G}{N_{\phi} \pi^3 c^{5}}}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{t_{\phi,t} = 2.282 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] Photon particle strong decoupling time: [math]\boxed{t_{\gamma,t} = \frac{3}{4} \sqrt{\frac{5 \Omega_{\gamma,t_P} \hbar G}{N_{\gamma} \pi^3 c^{5}}}} \; \; \; m_{\gamma} = 0[/math] [math]\boxed{t_{\gamma,t} = 8.128 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] Cosmic neutrino background radiation particle strong decoupling time: [math]\boxed{t_{\nu,t} = \frac{}{2} \sqrt{ \frac{3 C_{\nu} \Omega_{\nu,t_P} \hbar G}{N_{\nu} \pi^3 c^5}}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{t_{\nu,t} = 2.282 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Particle strong decoupling time summary: Planck particle strong decoupling time: [math]\boxed{t_{P} = 5.391 \cdot 10^{-44} \; \text{s}}[/math] Dark matter scalar particle strong decoupling time: [math]\boxed{t_{\phi,t} = 2.282 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] Photon particle strong decoupling time: [math]\boxed{t_{\gamma,t} = 8.128 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\gamma} = 0[/math] Cosmic neutrino background radiation particle strong decoupling time: [math]\boxed{t_{\nu,t} = 2.282 \cdot 10^{-47} \; \text{s}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] Is the Planck energy density the maximum energy density limit in the universe? [math]\;[/math] Any discussions and/or peer reviews about this specific topic thread? [math]\;[/math] Reference: Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf Wikipedia - Planck mass: (ref. 2) https://en.wikipedia.org/wiki/Planck_mass Wikipedia - Planck temperature: (ref. 3) https://en.wikipedia.org/wiki/Planck_temperature Wikipedia - Planck radius: (ref. 4) https://en.wikipedia.org/wiki/Planck_length Wikipedia - Planck volume: (ref. 5) https://en.wikipedia.org/wiki/Planck_units#Derived_units Wikipedia - Vacuum_energy: (ref. 6) https://en.wikipedia.org/wiki/Vacuum_energy Wikipedia - Planck time: (ref. 7) https://en.wikipedia.org/wiki/Planck_time (source code errors corrected on 12-30-2018)

test

[math]\text{Planck satellite cosmological parameters:} \; \left(\text{ref. 1}\right)[/math] [math]\Omega_{dm} = 0.268[/math] [math]\Omega_{b} = 0.0495[/math] [math]\Omega_{\Lambda} = 0.6825[/math] [math]\Omega_{\nu} = 1.259 \cdot 10^{-3}[/math] [math]\Omega_{\gamma} = 5.012 \cdot 10^{-5}[/math] [math]\;[/math] [math]\text{Dark matter scalar particle composition is equivalent to dark matter composition:}[/math] [math]\boxed{\Omega_{\phi} = \Omega_{dm}}[/math] [math]\;[/math] [math]\text{Planck mass:} \; \left(\text{ref. 2}\right)[/math] [math]m_{P} = \sqrt{\frac{\hbar c}{G}}[/math] [math]\;[/math] [math]\text{Planck temperature} \; \left(\text{ref. 3}\right)[/math] [math]T_{P} = \frac{E_{P}}{k_{B}} = \frac{m_{P} c^{2}}{k_{B}} = \left(\sqrt{\frac{\hbar c}{G}}\right) \frac{c^2}{k_{B}} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}} = 1.417 \cdot 10^{32} \; \text{K}[/math] [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\boxed{T_{P} = 1.417 \cdot 10^{32} \; \text{K}}[/math] [math]\;[/math] [math]\text{Planck radius:} \; \left(\text{ref. 4}\right)[/math] [math]r_P = \sqrt{\frac{\hbar G}{c^{3}}}[/math] [math]\;[/math] [math]\text{Planck volume:} \; \left(\text{ref. 5}\right)[/math] [math]V_{P} = \frac{4 \pi R_{P}^{3}}{3} = \frac{4 \pi}{3} \left(\sqrt{\frac{\hbar G}{c^{3}}}\right)^{3} = \frac{4 \pi}{3} \sqrt{\frac{\left(\hbar G\right)^{3}}{c^{9}}} = 1.768 \cdot 10^{-104} \; \text{m}^3[/math] [math]\;[/math] [math]\boxed{V_{P} = \frac{4 \pi}{3} \sqrt{\frac{\left(\hbar G\right)^{3}}{c^{9}}}}[/math] [math]\boxed{V_{P} = 1.768 \cdot 10^{-104} \; \text{m}^3}[/math] [math]\;[/math] [math]\text{Observable Universe total energy at present time:}[/math] [math]\boxed{E_u = \frac{\pi^3 k_B^4}{12 \left(\hbar H_{0}\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_u = 1.060 \cdot 10^{73} \; \text{j}}[/math] [math]\;[/math] [math]\text{All particle peak radiation temperatures are equivalent to Planck temperature at Planck time:}[/math] [math]\boxed{T_{\phi,t_P} = T_{\gamma,t_P} = T_{\nu,t_P} = T_P}[/math] [math]\text{Dark energy scalar particle peak radiation temperature is non-zero at Planck time:}[/math] [math]\boxed{T_{\Lambda,t_P} \neq 0}[/math] [math]\;[/math] [math]\text{Present time radial metric decoupling temperature redshift parameter global quantizations remain the same:}[/math] [math]\boxed{\ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right] \; \; \; \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right] \; \; \; \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]}[/math] [math]\;[/math] [math]\text{Observable Universe total energy at Planck time integration via substitution:}[/math] [math]E_{u}\left(T_P\right) = \frac{\pi^3 k_B^4}{12 \left(\hbar H_{0}\right)^3} \left(\frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}\right)^{4} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\;[/math] [math]\text{Observable Universe total energy at Planck time:}[/math] [math]\boxed{E_{u}\left(T_P\right) = \frac{\pi^3 c^{10}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_{u}\left(T_P\right) = 8.439 \cdot 10^{199} \; \text{j}}[/math] [math]\;[/math] [math]\text{Observable Universe total mass at Planck time:}[/math] [math]M_{u}\left(T_P\right) = \frac{E_{u}\left(T_P\right)}{c^{2}} = \frac{\pi^3 c^{8}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\boxed{M_{u}\left(T_P\right) = \frac{\pi^3 c^{8}}{12 \hbar G^{2} H_{0}^3} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{M_{u}\left(T_P\right) = 9.390 \cdot 10^{182} \; \text{kg}}[/math] [math]\;[/math] [math]\text{Observable Universe total energy density at Planck time integration via substitution:}[/math] [math]\epsilon_{u}\left(T_{P}\right) = \frac{E_{u}\left(T_{P}\right)}{V_{P}} = \frac{\pi^3 c^{10}}{12 \hbar G^{2} H_{0}^3} \left(\frac{3}{4 \pi} \sqrt{\frac{c^{9}}{\left(\hbar G\right)^{3}}}\right) \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)[/math] [math]\;[/math] [math]\text{Observable Universe total energy density at Planck time:}[/math] [math]\boxed{\epsilon_{u}\left(T_{P}\right) = \frac{\pi^{2}}{16 H_{0}^{3}} \sqrt{\frac{c^{29}}{\hbar^{5} G^{7}}} \left(\frac{N_{\phi}}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma}}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu}}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\;[/math] [math]\boxed{\epsilon_{u}\left(T_{P}\right) = 4.772 \cdot 10^{303} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] [math]\text{Planck energy density integration via substitution:}[/math] [math]\epsilon_{P} = \frac{E_{P}}{V_{P}} = \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)\left(\frac{3}{4 \pi} \sqrt{\frac{c^{9}}{\left(\hbar G\right)^{3}}}\right) = \frac{3 c^{7}}{4 \pi \hbar G^{2}} = 1.106 \cdot 10^{113} \; \frac{\text{j}}{\text{m}^{3}}[/math] [math]\;[/math] [math]\text{Planck energy density:} \; \left(\text{ref. 5}\right)[/math] [math]\boxed{\epsilon_{P} = \frac{3 c^{7}}{4 \pi \hbar G^{2}}}[/math] [math]\boxed{\epsilon_{P} = 1.106 \cdot 10^{113} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] [math]\text{Vacuum energy density at Planck time:} \; \left(\text{ref. 6}\right)[/math] [math]\epsilon_{vac} = \frac{\epsilon_{P}}{2} = \frac{3 c^{7}}{8 \pi \hbar G^{2}} = 5.531 \cdot 10^{112} \; \frac{\text{j}}{\text{m}^{3}}[/math] [math]\boxed{\epsilon_{vac} = \frac{3 c^{7}}{8 \pi \hbar G^{2}}}[/math] [math]\boxed{\epsilon_{vac} = 5.531 \cdot 10^{112} \; \frac{\text{j}}{\text{m}^{3}}}[/math] [math]\;[/math] [math]\text{Planck time:} \; \left(\text{ref. 7}\right)[/math] [math]t_{P} = \sqrt{\frac{\hbar G}{c^{5}}}[/math] [math]\;[/math] [math]\text{Particle interaction rate is equivalent to Hubble Parameter at Planck interaction time:}[/math] [math]\boxed{\Gamma_{t} = n \langle \sigma v \rangle = H_{t}}[/math] [math]\Gamma_{P} = H_{P} = \frac{}{t_{P}} = \sqrt{\frac{c^{5}}{\hbar G}} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}[/math] [math]\text{Planck particle interaction rate at Planck interaction time:}[/math] [math]\boxed{\Gamma_{P} = \sqrt{\frac{c^{5}}{\hbar G}}}[/math] [math]\;[/math] [math]\boxed{\Gamma_{P} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}}[/math] [math]\;[/math] [math]\text{Dark matter scalar particle interaction rate at scalar particle Planck time:}[/math] [math]\boxed{\Gamma_{\phi,t_P} = 2 \left(k_B T_{\phi,t_P}\right)^2 \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] [math]\text{Planck temperature:} \; \left(\text{ref. 3}\right)[/math] [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] [math]\text{Dark matter scalar particle interaction rate at scalar particle Planck time integration via substitution:}[/math] [math]\Gamma_{\phi,t_P} = 2 \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}} = \frac{2 \hbar c^{5}}{G} \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t_P} \hbar^3 c^5}} = 2 \sqrt{\frac{N_{\phi} \pi^3 c^{5}}{3 C_{\phi} \Omega_{\phi,t_P} \hbar G}}[/math] [math]\text{Dark matter scalar particle interaction rate at scalar particle Planck time:}[/math] [math]\boxed{\Gamma_{\phi,t_P} = 2 \sqrt{\frac{N_{\phi} \pi^3 c^{5}}{3 C_{\phi} \Omega_{\phi,t_P} \hbar G}}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{\Gamma_{\phi,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\phi} \neq 0[/math] [math]\;[/math] [math]\text{Photon interaction rate at photon Planck time:}[/math] [math]\boxed{\Gamma_{\gamma,t_P} = \frac{4 \left(k_B T_{\gamma,t_P}\right)^2}{3} \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] [math]\text{Planck temperature:} \; \left(\text{ref. 3}\right)[/math] [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] [math]\text{Photon interaction rate at photon Planck time integration via substitution:}[/math] [math]\Gamma_{\gamma,t_P} = \frac{4}{3} \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}} = \frac{4}{3} \left(\frac{\hbar c^{5}}{G}\right) \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t_P} \hbar^3 c^5}} = \frac{4}{3} \sqrt{\frac{N_{\gamma} \pi^3 c^{5}}{5 \Omega_{\gamma,t_P} \hbar G}}[/math] [math]\;[/math] [math]\text{Photon interaction rate at photon Planck time:}[/math] [math]\boxed{\Gamma_{\gamma,t_P} = \frac{4}{3} \sqrt{\frac{N_{\gamma} \pi^3 c^{5}}{5 \Omega_{\gamma,t_P} \hbar G}}} \; \; \; m_{\gamma} = 0[/math] [math]\boxed{\Gamma_{\gamma,t_P} = 1.230 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\gamma} = 0[/math] [math]\;[/math] [math]\text{Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time:}[/math] [math]\boxed{\Gamma_{\nu,t_P} = 2 \left(k_B T_{\nu,t_P}\right)^2 \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] [math]\text{Planck temperature:} \; \left(\text{ref. 3}\right)[/math] [math]\boxed{T_{P} = \frac{}{k_{B}} \sqrt{\frac{\hbar c^{5}}{G}}}[/math] [math]\;[/math] [math]\text{Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time integration via substitution:}[/math] [math]\Gamma_{\nu,t_P} = 2 \left(\sqrt{\frac{\hbar c^{5}}{G}}\right)^2 \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}} = 2 \left(\frac{\hbar c^{5}}{G}\right) \sqrt{\frac{G N_{\nu} \pi^3}{3 C_{\nu} \Omega_{\nu,t_P} \hbar^3 c^5}} = 2 \sqrt{\frac{N_{\nu} \pi^3 c^5}{3 C_{\nu} \Omega_{\nu,t_P} \hbar G}}[/math] [math]\text{Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time:}[/math] [math]\boxed{\Gamma_{\nu,t_P} = 2 \sqrt{\frac{N_{\nu} \pi^3 c^5}{3 C_{\nu} \Omega_{\nu,t_P} \hbar G}}} \; \; \; m_{\nu} \neq 0[/math] [math]\boxed{\Gamma_{\nu,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] [math]\text{Particle interaction rate summary:}[/math] [math]\text{Planck particle interaction rate at Planck interaction time:}[/math] [math]\boxed{\Gamma_{P} = 1.855 \cdot 10^{43} \; \frac{\text{particles}}{\text{s}}}[/math] [math]\text{Dark matter scalar particle interaction rate at scalar particle Planck time:}[/math] [math]\boxed{\Gamma_{\phi,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\phi} \neq 0[/math] [math]\text{Photon interaction rate at photon Planck time:}[/math] [math]\boxed{\Gamma_{\gamma,t_P} = 1.230 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\gamma} = 0[/math] [math]\text{Cosmic neutrino background radiation neutrino interaction rate at neutrino Planck time:}[/math] [math]\boxed{\Gamma_{\nu,t_P} = 1.207 \cdot 10^{46} \; \frac{\text{particles}}{\text{s}}} \; \; \; m_{\nu} \neq 0[/math] [math]\;[/math] [math]\text{Is the Planck energy density the maximum energy density limit in the universe?}[/math] [math]\;[/math] [math]\text{Any discussions and/or peer reviews about this specific topic thread?}[/math] [math]\;[/math] [math]\text{Reference:}[/math] Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf Wikipedia - Planck mass: (ref. 2) https://en.wikipedia.org/wiki/Planck_mass Wikipedia - Planck temperature: (ref. 3) https://en.wikipedia.org/wiki/Planck_temperature Wikipedia - Planck radius: (ref. 4) https://en.wikipedia.org/wiki/Planck_length Wikipedia - Planck volume: (ref. 5) https://en.wikipedia.org/wiki/Planck_units#Derived_units Wikipedia - Vacuum_energy: (ref. 6) https://en.wikipedia.org/wiki/Vacuum_energy Wikipedia - Planck time: (ref. 7)

\[ \text{test} \]

\[ F(x) = \int_{0}^{\infty} f(x) dx \] \[ F(x) = \int_{0}^{\infty} f(x) dx \]

This is true for the Compton equation, where a mass-less particle is scattering from a mass particle. However, in equation [math](6)[/math], the energy terms for [math]E[/math] represent the total energy of a mass particle, which includes its rest mass plus kinetic energy. I should have been more clear about that equation description and will include the total energy description in the next revision, hence peer review. If I understand this inelastic scattering correctly, when a lighter particle scatters from a heavier particle, as total kinetic energy is increased, then more kinetic energy is absorbed by the recoiling heavier particle and less kinetic energy is carried away by the lighter particle. So, the limit of equations [math](12)[/math] and [math](13)[/math] as kinetic energy approaches infinity, should be the rest mass of the neutrino. [math]\lim_{E_k \to \infty} m_{\nu} = m_{\nu,0}[/math]

test

This is some centered text

Derivation of neutrino mass from neutrino scattering: [math]\theta[/math] - scattered neutrino angle [math]\phi[/math] - electron recoil angle [math]p_{i}[/math] - initial neutrino momentum [math]p_{f}[/math] - final neutrino momentum [math]p_{e}[/math] - electron momentum [math]p_{e} \sin \phi = p_{f} \sin \theta \tag{1}[/math] [math]p_{e} \cos \phi + p_{f} \cos \theta = p_{i} \tag{2}[/math] Isolate [math]p_{e} \cos \phi[/math] from equation [math](2)[/math]: [math]p_{e} \cos \phi = p_{i} - p_{f} \cos \theta \tag{3}[/math] Divide equation [math](1)[/math] by equation [math](3)[/math] for an expression for [math]\tan \phi[/math]: [math]\tan \phi = \frac{p_{f} \sin \theta}{p_{i} - p_{f} \cos \theta} = \frac{\sin \theta}{\frac{p_{i}}{p_{f}} - \cos \theta} \tag{4}[/math] Acquire a substitution for [math]\frac{p_{i}}{p_{f}}[/math] to eliminate [math]p_{f}[/math]. Use the Compton equation, which can be rearranged to yield [math]\frac{\lambda_{f}}{\lambda_{i}} = \frac{p_{i}}{p_{f}}[/math] in terms of [math]\lambda_{i}[/math] alone, noting that [math]p = \frac{E}{c}[/math]. [math]\lambda_{f} - \lambda_{i} = \frac{h}{m_{e} c} (1 - \cos \theta) \tag{5}[/math] [math]\frac{\lambda_{f}}{\lambda_{i}} = \frac{p_{i}}{p_{f}} = 1 + \frac{E_{\nu}}{E_{e}} (1 - \cos \theta) = 1 + \frac{m_{\nu} c^2}{m_{e} c^2} (1 - \cos \theta) = 1 + \frac{m_{\nu}}{m_{e}} (1 - \cos \theta) \tag{6}[/math] Substituting equation [math](6)[/math] into equation [math](4)[/math], and eliminate [math]p_{i}[/math] and [math]p_{f}[/math] in favor of [math]m_{\nu}[/math] alone. [math]\tan \phi = \frac{\sin \theta}{\frac{p_{i}}{p_{f}} - \cos \theta} = \frac{\sin \theta}{1 + \frac{m_{\nu}}{m_{e}} (1 - \cos \theta) - \cos \theta} = \frac{\sin \theta}{\left(1 + \frac{m_{\nu}}{m_{e}} \right)(1 - \cos \theta)} \tag{7}[/math] Utilizing a trigonometric identity produces the desired result, specifically: [math]\frac{1 - \cos \theta}{\sin \theta} = \tan \left(\frac{\theta}{2} \right) \tag{8}[/math] Substituting this trigonometric identity into equation [math](7)[/math] results in: [math]\left(1 + \frac{m_{\nu}}{m_{e}} \right) \tan \phi = \cot \frac{\theta}{2} \tag{9}[/math] Solve for neutrino mass [math]m_{\nu}[/math]: [math]\tan \phi + \frac{m_{\nu}}{m_{e}} \tan \phi = \cot \frac{\theta}{2} \tag{10}[/math] [math]\frac{m_{\nu}}{m_{e}} \tan \phi = \left(\cot \frac{\theta}{2} - \tan \phi \right) \tag{11}[/math] Electron-neutrino scattering neutrino mass: [math]\boxed{m_{\nu} = m_{e} \cot \phi \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{12}[/math] Nuclear-neutrino scattering neutrino mass: [math]\boxed{m_{\nu} = m_{n} \cot \phi \left(\cot \frac{\theta}{2} - \tan \phi \right)} \tag{13}[/math] [math]m_{n}[/math] - nuclear mass Electron interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{(m_{e} + m_{\nu}) \tan \phi}{m_{e}} \right)} \tag{14}[/math] Neutrino interaction electron recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{m_{e} \cot \frac{\theta}{2}}{m_{e} + m_{\nu}} \right)} \tag{15}[/math] Nuclear interaction neutrino scattering angle [math]\theta[/math]: [math]\boxed{\theta = 2 \operatorname{arccot} \left(\frac{(m_{n} + m_{\nu}) \tan \phi}{m_{n}} \right)} \tag{16}[/math] Neutrino interaction nuclear recoil angle [math]\phi[/math]: [math]\boxed{\phi = \arctan \left(\frac{m_{n} \cot \frac{\theta}{2}}{m_{n} + m_{\nu}} \right)} \tag{17}[/math] Any discussions and/or peer reviews about this specific topic thread? Reference: Wikipedia - Compton scattering - Derivation of the scattering formula: https://en.wikipedia.org/wiki/Compton_scattering#Derivation_of_the_scattering_formula Physics 253 - Compton Scattering - Patrick LeClair: http://pleclair.ua.edu//PH253/Notes/compton.pdf Orion1 - Neutrino mass from Fermi-Dirac statistics...: https://www.scienceforums.net/topic/90189-neutrino-mass-from-fermi-dirac-statistics/ Science News - Neutrinos seen scattering off an atom’s nucleus for the first time: https://www.sciencenews.org/article/neutrinos-seen-scattering-atoms-nucleus-first-time

[math]F(x) = \int_{0}^{\infty} f(x) dx[/math] [math]\boxed{F(x) = \int_{0}^{\infty} f(x) dx} \tag{1}[/math] [math]\text{Equation} (1) \tag{}[/math].

Planck satellite cosmological parameters: (ref. 1, pg. 11) [math]\Omega_{dm} = 0.268[/math] [math]\Omega_{b} = 0.0495[/math] [math]\Omega_{\Lambda} = 0.6825[/math] Symbolic identity key: [math]n_{s}[/math] - spin states total number [math]N_{s}[/math] - species total number [math]N_{n}[/math] - total effective degeneracy number [math]\text{if} \; n_{s} \geq N_{s} \; \text{then} \; N_{n} = n_{s}[/math] [math]\text{if} \; n_{s} \leq N_{s} \; \text{then} \; N_{n} = N_{s}[/math] [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & \neq 0 & \phi \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & \neq 0 & \nu \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} \\ \end{array}[/math] Stellar class number parameters: (ref. 2) [math]n_c = 1 \rightarrow 7[/math] key: 1 O, 2 B, 3 A, 4 F, 5 G, 6 K, 7 M [math]\Omega_n[/math] - main sequence stars stellar class fraction [math]N_s[/math] - total observable stellar number [math]M_n[/math] - Main-sequence mass Observable Universe average stellar mass: [math]M_{as} = \frac{1}{N_s} \sum_{n_c = 1}^{7} \left(\Omega_n N_s M_n\right) = \sum_{n_c = 1}^{7} \Omega_n M_n = 0.595 \cdot M_{\odot} \rightarrow 0.769 \cdot M_{\odot}[/math] [math]\boxed{M_{as} = \sum_{n_c = 1}^{7} \Omega_n M_n}[/math] [math]\boxed{M_{as} = \left(0.595 \rightarrow 0.769 \right) \cdot M_{\odot}}[/math] Observable Universe average stellar mass lower bound limit: [math]\boxed{M_{as} = 1.183 \cdot 10^{30} \; \text{kg}}[/math] Observable Universe average stellar mass: (ref. 3, pg. 20) [math]M_{as} = 0.6 \cdot M_{\odot} = 1.193 \cdot 10^{30} \; \text{kg}[/math] [math]M_{as} = 1.193 \cdot 10^{30} \; \text{kg}[/math] Observable Universe stellar baryon composition: (ref. 4, pg. 3) [math]\Omega_s = \left(\Omega_{ms} + \Omega_{wd} + \Omega_{ns}\right) = 2.460 \cdot 10^{-3}[/math] [math]\Omega_s = 2.460 \cdot 10^{-3}[/math] --- Milky Way galaxy mass: (ref. 5, pg. 1) [math]M_{mw} = 1.260 \cdot 10^{12} \cdot M_{\odot} = 2.506 \cdot 10^{42} \; \text{kg}[/math] [math]\boxed{M_{mw} = 2.506 \cdot 10^{42} \; \text{kg}}[/math] --- Redshift parameter at photon decoupling time: [math]z = 1090.43[/math] Cosmic photon background radiation temperature at present time: [math]T_{\gamma} = 2.72548 \; \text{K}[/math] Cosmic photon background radiation temperature at photon decoupling time: [math]T_{\gamma,t} = T_{\gamma} \left(1 + z\right) = 2974.67 \; \text{K}[/math] [math]\boxed{T_{\gamma,t} = 2974.67 \; \text{K}}[/math] Cosmic neutrino background radiation temperature at present time: (ref. 6, pg. 44, eq. 220) [math]T_{\nu} = \left(\frac{4}{11}\right)^{\frac{1}{3}} T_{\gamma} = 1.945 \; \text{K}[/math] [math]\boxed{T_{\nu} = 1.945 \; \text{K}}[/math] Cosmic neutrino background radiation temperature at neutrino decoupling time: (ref. 7) [math]T_{\nu,t} = 1 \cdot 10^{10} \; \text{K}[/math] Observable Universe dark matter scalar particle temperature is equivalent to cosmic neutrino background radiation temperature: [math]\boxed{T_{\phi} = T_{\nu}}[/math] Cosmic scalar particle dark energy background radiation temperature: [math]\boxed{T_{\Lambda} = \frac{}{k_{B}} \left(\frac{45 \Omega_{\Lambda} H_0^2 \hbar^3 c^5}{4 G N_{\Lambda} \pi^3}\right)^{1/4}}[/math] [math]\boxed{T_{\Lambda} = 35.013 \; \text{K}}[/math] Observable Universe Cosmological Constant: [math]\Lambda_s = \frac{3 \Omega_{\Lambda} H_0^2}{c^2} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] Observable Universe scalar particle dark energy Cosmological Constant integration via substitution: [math]\Lambda_s = \frac{3 H_0^2}{c^2} \left(\frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{45 H_0^2 \hbar^3 c^5}\right) = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7}}[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = 1.180 \cdot 10^{-52} \; \text{m}^{-2}}[/math] Dark matter density: [math]\rho_{dm} = \frac{3 \Omega_{dm} H_0^2}{8 \pi G}[/math] Baryonic density: [math]\rho_{b} = \frac{3 \Omega_{b} H_0^2}{8 \pi G}[/math] Dark energy density: [math]\rho_{\Lambda} = \frac{3 \Omega_{\Lambda} H_0^2}{8 \pi G}[/math] Dark matter scalar particle composition is equivalent to dark matter composition: [math]\boxed{\Omega_{\phi} = \Omega_{dm}}[/math] Bose-Einstein total dark matter scalar particle distribution constant: [math]\boxed{C_{\phi} = \frac{4 G N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{3 \Omega_{\phi} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\phi} = 3.640 \cdot 10^{-4}}[/math] Fermi-Dirac total neutrino distribution constant: [math]\boxed{C_{\nu} = \frac{4 G N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{3 \Omega_{\nu} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\nu} = 0.236}[/math] Bose-Einstein scalar particle dark matter density: [math]\boxed{\rho_{\phi} = \frac{N_{\phi} \pi^2 \left(k_B T_{\phi}\right)^4}{2 C_{\phi} \hbar^3 c^5}}[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter density: [math]\boxed{\rho_{b} = \frac{N_{\gamma} \pi^2 \left(k_B T_{\gamma}\right)^4}{30 \hbar^3 c^5} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) + \frac{N_{\nu} \pi^2 \left(k_B T_{\nu}\right)^4}{2 C_{\nu} \hbar^3 c^5} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right)}[/math] Bose-Einstein scalar particle dark energy density: [math]\boxed{\rho_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{30 \hbar^3 c^5}}[/math] Observable Universe total photon and neutrino co-moving volumes: [math]V_{\gamma} = \frac{4 \pi R_{\gamma}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]\right)^3[/math] [math]V_{\nu} = \frac{4 \pi R_{\nu}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]\right)^3[/math] Observable Universe scalar particle dark matter and scalar particle dark energy and neutrino total co-moving volumes are equivalent: [math]\boxed{V_{\phi} = V_{\Lambda} = V_{\nu}}[/math] Bose-Einstein scalar particle dark matter total mass: [math]\boxed{\rho_{\phi} V_{\phi} = \frac{N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{12 C_{\phi} c^2 \left(\hbar H_0\right)^3} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3}[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter relative composition total mass: [math]\boxed{\rho_{b} V_{b} = \frac{N_{\gamma} \pi^3 \left(k_B T_{\gamma}\right)^4}{180 \left(\hbar H_0\right)^3 c^2} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{12 C_{\nu} \left(\hbar H_0\right)^3 c^2} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3}[/math] Bose-Einstein scalar particle dark energy total mass: [math]\boxed{\rho_{\Lambda} V_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{180 \left(\hbar H_0\right)^3 c^2} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3}[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{dm} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] Observable Universe total critical mass: [math]M_{c} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{dm} V_{dm} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\boxed{M_c = \frac{c^3}{16 G H_0} \left(\left(\Omega_{dm} + \Omega_{\Lambda}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \Omega_b \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3\right)}[/math] [math]\boxed{M_c = 1.179 \cdot 10^{56} \; \text{kg}}[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{\phi} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] Observable Universe scalar particle dark matter and scalar particle dark energy composition total mass: [math]M_{u} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{\phi} V_{\phi} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\boxed{M_u = \frac{\pi^3 k_B^4}{12 c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{M_u = 1.179 \cdot 10^{56} \; \text{kg}}[/math] Observable Universe total energy: [math]E_u = M_u c^2[/math] [math]\boxed{E_u = \frac{\pi^3 k_B^4}{12 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_u = 1.060 \cdot 10^{73} \; \text{j}}[/math] Observable Universe total observable stellar number: [math]\boxed{N_s = \frac{\Omega_s \pi^3 k_B^4}{12 M_{as} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] Observable Universe total stellar number: [math]\boxed{N_s = 2.453 \cdot 10^{23} \; \text{stars}}[/math] Wikipedia observable universe total stellar number: (ref. 8) [math]N_s = 3.000 \cdot 10^{23} \; \text{stars}[/math] Observable Universe total galaxy number: [math]\boxed{N_g = \frac{\Omega_b \pi^3 k_B^4}{12 M_{mw} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] Observable Universe total galaxy number: [math]\boxed{N_g = 2.330 \cdot 10^{12} \; \text{galaxies}}[/math] Wikipedia observable universe total galaxy number: (ref. 9) [math]N_g = 2.000 \cdot 10^{12} \; \text{galaxies}[/math] Observable Universe stars per galaxy average number: [math]\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] [math]\boxed{\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}}}[/math] [math]\boxed{\frac{N_s}{N_g} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}}[/math] Wikipedia stars per galaxy average number: (ref. 8, ref. 9) [math]\frac{N_s}{N_g} = 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] Wikipedia Milky Way galaxy total stellar number: (ref. 10) [math]\frac{N_s}{N_g} = 2.500 \cdot 10^{11} \pm 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] Observable Universe planetary composition: (ref. 4, pg. 3) [math]\Omega_p = 1 \cdot 10^{-6}[/math] Wikipedia Milky Way galaxy total planetary number: (ref. 10, ref. 11) [math]\frac{N_p}{N_g} = \left(1.000 \cdot 10^{11} \rightarrow 1.600 \cdot 10^{11}\right) \; \frac{\text{planets}}{\text{galaxy}}[/math] Observable Universe average planetary mass: [math]\boxed{M_{ap} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{N_g}{N_p}\right)}[/math] [math]\boxed{M_{ap} = \left(52.974 \rightarrow 84.758\right) \cdot M_{\oplus}}[/math] Solar star system average planetary mass: (ref. 12) [math]\boxed{M_{ap} = \frac{}{n_p} \sum_{n = 1}^{n_p} M_n} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{M_{ap} = 55.855 \cdot M_{\oplus}}[/math] Milky Way galaxy total planetary number based upon solar star system: [math]\boxed{\frac{N_p}{N_g} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_n\right)^{-1}} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{\frac{N_p}{N_g} = 1.518 \cdot 10^{11} \; \frac{\text{planets}}{\text{galaxy}}}[/math] Any discussions and/or peer reviews about this specific topic thread? Is there anything else that you want to see quantified based upon this model for observational comparison? Reference: Planck 2013 results. XVI. Cosmological parameters: (ref. 1) http://planck.caltech.edu/pub/2013results/Planck_2013_results_16.pdf Wikipedia - Stellar classification - Harvard spectral classification: (ref. 2) https://en.wikipedia.org/wiki/Stellar_classification#Harvard_spectral_classification On The Mass Distribution Of Stars...: (ref. 3) http://www.doiserbia.nb.rs/img/doi/1450-698X/2006/1450-698X0672017N.pdf The Cosmic Energy Inventory: (ref. 4) http://arxiv.org/pdf/astro-ph/0406095v2.pdf Mass models of the Milky Way: (ref. 5) http://arxiv.org/pdf/1102.4340v1 PHYS: 652 Cosmic Inventory I: Radiation: (ref. 6) http://www.nicadd.niu.edu/~bterzic/PHYS652/Lecture_09.pdf Wikipedia - neutrino decoupling: (ref. 7) https://en.wikipedia.org/wiki/Neutrino_decoupling Wikipedia - Observable universe total observable stellar number: (ref. 8) https://en.wikipedia.org/wiki/Star#Distribution Wikipedia - Galaxy: (ref. 9) https://en.wikipedia.org/wiki/Galaxy Wikipedia - Milky Way Galaxy: (ref. 10) https://en.wikipedia.org/wiki/Milky_Way Space.com - 160 Billion Alien Planets May Exist in Our Milky Way Galaxy: (ref. 11) https://www.space.com/14200-160-billion-alien-planets-milky-galaxy.html Wikipedia - Planetary mass: (ref. 12) https://en.wikipedia.org/wiki/Planetary_mass#Values_from_the_DE405_ephemeris (source code errors corrected 05-19-2018)

[math]F(x) = \int_{0}^{\infty} f(x) dx[/math] [math]\boxed{F(x) = \int_{0}^{\infty} f(x) dx}[/math] My pizza is arriving squashed also, no tip for the delivery driver! The boxed code appears to override whatever is causing it, but I do not want to box every equation, only my solutions.

Photon species total effective degeneracy number: [math]\boxed{N_{\gamma} = 2}[/math] Photon radiation energy radiant emmittance Bose-Einstein distribution integration via substitution: [math]j^{*} = \sigma_{\gamma} T_{\gamma}^4 = \int_{0}^{2 \pi} \int_{0}^{\frac{\pi}{2}} \cos \phi \sin \phi \; d \phi \; d \theta \; \frac{N_{\gamma} E_{\gamma}^4}{c^2 (2 \pi \hbar)^3} \int_{0}^\infty \frac{E_t (\omega)^3}{e^{\frac{E_t (\omega)}{E_{\gamma} (T_{\gamma})}} - 1} d \omega = \frac{\pi N_{\gamma} (k_B T_{\gamma})^4}{c^2 (2 \pi \hbar)^3} \left(\frac{\pi^4}{15}\right) = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{120 c^2 \hbar^3}[/math] Radiant emmittance Stefan-Boltzmann constant: [math]\boxed{\sigma_{\gamma} = \frac{N_{\gamma} \pi^2 k_B^4}{120 c^2 \hbar^3}}[/math] Radiant emmittance Stefan-Boltzmann law: [math]\boxed{j^{*} = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{120 c^2 \hbar^3}}[/math] Photon radiation energy density Bose-Einstein distribution integration via substitution: [math]\epsilon_{\gamma} = \alpha_{\gamma} T_{\gamma}^4 = \int_{0}^{2 \pi} \int_{0}^{\pi} \sin \theta \; d \theta \; d \phi \; \frac{N_{\gamma} E_{\gamma}^4}{(2 \pi \hbar c)^3} \int_{0}^\infty \frac{E_t (\omega)^3}{e^{\frac{E_t (\omega)}{E_{\gamma} (T_{\gamma})}} - 1} d \omega = \frac{4 \pi N_{\gamma} (k_B T_{\gamma})^4}{(2 \pi \hbar c)^3} \left(\frac{\pi^4}{15}\right) = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{30 (\hbar c)^3}[/math] Photon radiation constant: [math]\boxed{\alpha_{\gamma} = \frac{N_{\gamma} \pi^2 k_B^4}{30 (\hbar c)^3}}[/math] Photon radiation energy density: [math]\boxed{\epsilon_{\gamma} = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{30 (\hbar c)^3}}[/math] Planck's law: (ref. 1) [math]\boxed{I_{\gamma}(\nu,T_{\gamma}) = \frac{N_{\gamma} h \nu^3}{c^2 \left(e^{\frac{E_t}{E_{\gamma}}} - 1\right)}}[/math] Radiant emmittance integration via substitution: (ref. 2) [math]j^* = \int d\Omega \int_0^\infty I_{\gamma}(\nu,T_{\gamma}) \; d\nu[/math] [math]\int d\Omega = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \sin \phi \; d \phi \; d\theta[/math] [math]j^* = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \; \sin \phi \; d \phi \; d\theta \int_0^\infty I_{\gamma}(\nu,T_{\gamma}) \; d\nu[/math] [math]j^* = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \; \sin \phi \; d \phi \; d\theta \; \frac{N_{\gamma} h}{c^2} \int_0^\infty \frac{\nu^3}{e^{\frac{E_t}{E_{\gamma}}} - 1} \; d\nu[/math] Differential calculus theorem: [math]\boxed{\frac{\int_a^b f(u)^n \; du}{\int_a^b f(v)^n \; dv} = \left( \frac{du}{dv} \right)^{n+1}}[/math] [math]\int_0^\infty \frac{\nu^3}{e^{\frac{E_t}{E_{\gamma}}} - 1} \; d\nu = \left( \frac{d\nu}{du} \right)^4 \int_0^\infty \frac{u^3}{e^u - 1} \; du[/math] [math]j^* = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \; \sin \phi \; d \phi \; d\theta \; \frac{N_{\gamma} h}{c^2} \left( \frac{d\nu}{du} \right)^4 \int_0^\infty \frac{u^3}{e^u - 1} \; du[/math] [math]\frac{d\nu}{du} = \frac{E_{\gamma}}{h}[/math] [math]j^* = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \; \sin \phi \; d \phi \; d\theta \; \frac{N_{\gamma} h}{c^2} \left( \frac{E_{\gamma}}{h} \right)^4 \int_{0}^\infty \frac{E_t (\nu)^3}{e^{\frac{E_t (\nu)}{E_{\gamma} (T_{\gamma})}} - 1} d \nu[/math] [math]j^* = \int_0^{2 \pi} \int_0^{\frac{\pi}{2}} \cos \phi \; \sin \phi \; d \phi \; d\theta \; \frac{N_{\gamma} E_{\gamma}^4}{c^2 h^3} \int_{0}^\infty \frac{E_t (\nu)^3}{e^{\frac{E_t(\nu)}{E_{\gamma} (T_{\gamma})}} - 1} d \nu[/math] [math]j^{*} = \int_{0}^{2 \pi} \int_{0}^{\frac{\pi}{2}} \cos \phi \sin \phi \; d \phi \; d \theta \; \frac{N_{\gamma} E_{\gamma}^4}{c^2 (2 \pi \hbar)^3} \int_{0}^\infty \frac{E_t (\omega)^3}{e^{\frac{E_t (\omega)}{E_{\gamma} (T_{\gamma})}} - 1} d \omega = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{120 c^2 \hbar^3}[/math] Radiant emmittance Stefan-Boltzmann law: (ref. 3) [math]\boxed{j^{*} = \frac{N_{\gamma} \pi^2 (k_B T_{\gamma})^4}{120 c^2 \hbar^3}}[/math] --- The Stefan-Boltzmann law was first theorized in 1879, Planck's law was first theorized in 1914, I attempted to trace the derivation of the Stefan-Boltzmann law and Planck's law to determine the mathematical and theoretical origin of the number "2" in the numerator of Planck's law, however there was no formal derivation formulas published in their original papers. According to Planck, the specific intensity[math]\; K \;[/math]of a monochromatic plane polarized ray of frequency[math]\; \nu \;[/math]is: (ref. 4, pg. 168, eq. 274, 276) [math]K_{\nu}(\nu,T) = \frac{h \nu^3}{c^2} \frac{1}{e^{\frac{h \nu}{k_B T}} - 1}[/math] [math]E_{\lambda}(\lambda,T) = \frac{h c^2}{\lambda^5} \frac{1}{e^{\frac{h c}{\lambda k_B T}} - 1}[/math] Wikipedia Planck's laws: (ref. 1, ref. 5, pg. 22, eq. 1.51, 1.52) [math]B_{\nu}(\nu,T) = \frac{2 h \nu^3}{c^2} \frac{1}{e^{\frac{h \nu}{k_B T}} - 1}[/math] [math]B_{\lambda}(\lambda,T) = \frac{2 h c^2}{\lambda^5} \frac{1}{e^{\frac{h c}{\lambda k_B T}} - 1}[/math] Note that the number "2" in the numerator was not published in Planck's original paper. These equations in Planck's original paper describe a scalar particle. According to the derivation above, the number "2" in the numerator corresponds to the photon species total effective degeneracy number[math]\; N_{\gamma}[/math]. According to Rybicki and Lightman, photons have two independent polarizations (two states per wave vector)[math]\; k[/math], corresponding to the density of states (the number of states per solid angle per volume per frequency): (ref. 5, pg. 20, eq 1.47) [math]\rho_{s} = \frac{2 \nu^2}{c^3}[/math] Is this the theoretical definition for the total effective degeneracy number[math]\; N_{\gamma} \;[/math]for photon radiation? [math]\boxed{\rho_{s} = \frac{N_{\gamma} \nu^2}{c^3}}[/math] Any discussions and/or peer reviews about this specific topic thread? Reference: Wikipedia - Planck's law: (ref. 1) https://en.wikipedia.org/wiki/Planck's_law Wikipedia - Stefan–Boltzmann law - derivation from Planck's law: (ref. 2) https://en.wikipedia.org/wiki/Stefan–Boltzmann_law#Derivation_from_Planck.27s_law Wikipedia - Stefan-Boltzmann law: (ref. 3) https://en.wikipedia.org/wiki/Stefan–Boltzmann_law Planck, Maxwell (1914) - The Theory of Heat Radiation: (ref. 4) https://archive.org/stream/theoryofheatradi00planrich?ref=ol#page/168/mode/2up Rybicki and Lightman (1979): (ref. 5) http://www.bartol.udel.edu/~owocki/phys633/RadProc-RybLightman.pdf

Symbolic identity key: [math]n_{s}[/math] - spin states total number [math]N_{s}[/math] - species total number [math]N_{n}[/math] - total effective degeneracy number [math]\text{if} \; n_{s} \geq N_{s} \; \text{then} \; N_{n} = n_{s}[/math] [math]\text{if} \; n_{s} \leq N_{s} \; \text{then} \; N_{n} = N_{s}[/math] [math]\begin{array}{l*{7}{c}r} & \text{identity} & \text{state} & \text{spin} & n_{s} & N_{s} & N_{n} & \text{mass} & \text{type} \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & 0 & \Lambda \\ b & \text{scalar} & 0 & 0 & 1 & 1 & 1 & \neq 0 & \phi \\ f & \text{neutrino} & +,- & 1/2 & 2 & 3 & 3 & \neq 0 & \nu \\ b & \text{photon} & +,- & 1 & 2 & 1 & 2 & 0 & \gamma \\ b & \text{graviton} & +,- & 2 & 2 & 1 & 2 & 0 & \text{G} \\ \end{array}[/math] Stellar class number parameters: (ref. 1) [math]n_c = 1 \rightarrow 7[/math] key: 1 O, 2 B, 3 A, 4 F, 5 G, 6 K, 7 M [math]\Omega_n[/math] - main sequence stars stellar class fraction [math]N_s[/math] - total observable stellar number [math]M_n[/math] - Main-sequence mass Observable Universe average stellar mass: [math]M_{as} = \frac{1}{N_s} \sum_{n_c = 1}^{7} \left(\Omega_n N_s M_n\right) = \sum_{n_c = 1}^{7} \Omega_n M_n = 0.595 \cdot M_{\odot} \rightarrow 0.769 \cdot M_{\odot}[/math] [math]\boxed{M_{as} = \sum_{n_c = 1}^{7} \Omega_n M_n}[/math] [math]\boxed{M_{as} = \left(0.595 \rightarrow 0.769 \right) \cdot M_{\odot}}[/math] Observable Universe average stellar mass lower bound limit: [math]\boxed{M_{as} = 1.183 \cdot 10^{30} \; \text{kg}}[/math] Observable Universe average stellar mass: (ref. 2, pg. 20) [math]M_{as} = 0.6 \cdot M_{\odot} = 1.193 \cdot 10^{30} \; \text{kg}[/math] Observable Universe stellar baryon density: (ref. 3, pg. 3) [math]\Omega_s = \left(\Omega_{ms} + \Omega_{wd} + \Omega_{ns}\right) = 2.460 \cdot 10^{-3}[/math] [math]\Omega_s = 2.460 \cdot 10^{-3}[/math] --- Milky Way galaxy mass: (ref. 4, pg. 1) [math]M_{mw} = 1.260 \cdot 10^{12} \cdot M_{\odot} = 2.506 \cdot 10^{42} \; \text{kg}[/math] [math]\boxed{M_{mw} = 2.506 \cdot 10^{42} \; \text{kg}}[/math] --- Redshift parameter at photon decoupling time: [math]z = 1090.43[/math] Cosmic photon background radiation temperature at present time: [math]T_{\gamma} = 2.72548 \; \text{K}[/math] Cosmic photon background radiation temperature at photon decoupling time: [math]T_{\gamma,t} = T_{\gamma} \left(1 + z\right) = 2974.67 \; \text{K}[/math] [math]\boxed{T_{\gamma,t} = 2974.67 \; \text{K}}[/math] Cosmic neutrino background radiation temperature at present time: (ref. 5, pg. 44, eq. 220) [math]T_{\nu} = \left(\frac{4}{11}\right)^{\frac{1}{3}} T_{\gamma} = 1.945 \; \text{K}[/math] [math]\boxed{T_{\nu} = 1.945 \; \text{K}}[/math] Cosmic neutrino background radiation temperature at neutrino decoupling time: (ref. 6) [math]T_{\nu,t} = 1 \cdot 10^{10} \; \text{K}[/math] Observable Universe dark matter scalar particle temperature is equivalent to cosmic neutrino background radiation temperature: [math]\boxed{T_{\phi} = T_{\nu}}[/math] Cosmic scalar particle dark energy background radiation temperature: [math]\boxed{T_{\Lambda} = \frac{}{k_{B}} \left(\frac{45 \Omega_{\Lambda} H_0^2 \hbar^3 c^5}{4 G N_{\Lambda} \pi^3}\right)^{1/4}}[/math] [math]\boxed{T_{\Lambda} = 35.013 \; \text{K}}[/math] Observable Universe Cosmological Constant: [math]\Lambda_s = \frac{3 \Omega_{\Lambda} H_0^2}{c^2} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] Observable Universe scalar particle dark energy Cosmological Constant integration via substitution: [math]\Lambda_s = \frac{3 H_0^2}{c^2} \left(\frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{45 H_0^2 \hbar^3 c^5}\right) = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7} = 1.180 \cdot 10^{-52} \; \text{m}^{-2}[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = \frac{4 G N_{\Lambda} \pi^3 \left(k_B T_{\Lambda}\right)^4}{15 \hbar^3 c^7}}[/math] Observable Universe scalar particle dark energy Cosmological Constant: [math]\boxed{\Lambda_s = 1.180 \cdot 10^{-52} \; \text{m}^{-2}}[/math] Dark matter density: [math]\rho_{dm} = \frac{3 \Omega_{dm} H_0^2}{8 \pi G}[/math] Baryonic density: [math]\rho_{b} = \frac{3 \Omega_{b} H_0^2}{8 \pi G}[/math] Dark energy density: [math]\rho_{\Lambda} = \frac{3 \Omega_{\Lambda} H_0^2}{8 \pi G}[/math] Bose-Einstein total dark matter scalar particle distribution constant: [math]\boxed{C_{\phi} = \frac{4 G N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{3 \Omega_{\phi} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\phi} = 3.640 \cdot 10^{-4}}[/math] Fermi-Dirac total neutrino distribution constant: [math]\boxed{C_{\nu} = \frac{4 G N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{3 \Omega_{\nu} H_0^2 \hbar^3 c^5}}[/math] [math]\boxed{C_{\nu} = 0.236}[/math] Bose-Einstein scalar particle dark matter density: [math]\boxed{\rho_{\phi} = \frac{N_{\phi} \pi^2 \left(k_B T_{\phi}\right)^4}{2 C_{\phi} \hbar^3 c^5}}[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter density: [math]\boxed{\rho_{b} = \frac{N_{\gamma} \pi^2 \left(k_B T_{\gamma}\right)^4}{30 \hbar^3 c^5} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) + \frac{N_{\nu} \pi^2 \left(k_B T_{\nu}\right)^4}{2 C_{\nu} \hbar^3 c^5} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right)}[/math] Bose-Einstein scalar particle dark energy density: [math]\boxed{\rho_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{30 \hbar^3 c^5}}[/math] Observable Universe total photon and neutrino co-moving volumes: [math]V_{\gamma} = \frac{4 \pi R_{\gamma}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]\right)^3[/math] [math]V_{\nu} = \frac{4 \pi R_{\nu}^3}{3} = \frac{4 \pi}{3} \left(\left(\frac{c}{2 H_{0}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]\right)^3[/math] Observable Universe scalar particle dark matter and scalar particle dark energy and neutrino total co-moving volumes are equivalent: [math]\boxed{V_{\phi} = V_{\Lambda} = V_{\nu}}[/math] Bose-Einstein scalar particle dark matter total mass: [math]\boxed{\rho_{\phi} V_{\phi} = \frac{N_{\phi} \pi^3 \left(k_B T_{\phi}\right)^4}{12 C_{\phi} c^2 \left(\hbar H_0\right)^3} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3}[/math] Bose-Einstein and Fermi-Dirac baryonic photon and neutrino matter total mass: [math]\boxed{\rho_{b} V_{b} = \frac{N_{\gamma} \pi^3 \left(k_B T_{\gamma}\right)^4}{180 \left(\hbar H_0\right)^3 c^2} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} \pi^3 \left(k_B T_{\nu}\right)^4}{12 C_{\nu} \hbar^3 c^2} \left(\frac{\Omega_{b}}{\Omega_{b} - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3}[/math] Bose-Einstein scalar particle dark energy total mass: [math]\boxed{\rho_{\Lambda} V_{\Lambda} = \frac{N_{\Lambda} \pi^2 \left(k_B T_{\Lambda}\right)^4}{180 \left(\hbar H_0\right)^3 c^2} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3}[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{dm} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] Observable Universe total critical mass: [math]M_{c} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{dm} V_{dm} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\boxed{M_c = \frac{c^3}{16 G H_0} \left(\left(\Omega_{dm} + \Omega_{\Lambda}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \Omega_b \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3\right)}[/math] [math]\boxed{M_c = 1.179 \cdot 10^{56} \; \text{kg}}[/math] Observable Universe compositional Equation of State and compositional number: [math]\Omega_{\phi} + \Omega_{b} + \Omega_{\Lambda} = 1 \; \; \; \; \; \; n_{c} = 3[/math] Observable Universe scalar particle dark matter and scalar particle dark energy total mass: [math]M_{u} = \sum_{n = 1}^{n_{c}} \rho_n V_n = \rho_{\phi} V_{\phi} + \rho_{b} V_{b} + \rho_{\Lambda} V_{\Lambda}[/math] [math]\boxed{M_u = \frac{\pi^3 k_B^4}{12 c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{M_u = 1.179 \cdot 10^{56} \; \text{kg}}[/math] Observable Universe total energy: [math]E_u = M_u c^2[/math] [math]\boxed{E_u = \frac{\pi^3 k_B^4}{12 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] [math]\boxed{E_u = 1.060 \cdot 10^{73} \; \text{j}}[/math] Observable Universe total observable stellar number: [math]\boxed{N_s = \frac{\Omega_s \pi^3 k_B^4}{12 M_{as} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] Observable Universe total stellar number: [math]\boxed{N_s = 2.453 \cdot 10^{23} \; \text{stars}}[/math] Wikipedia observable universe total stellar number: (ref. 7) [math]N_s = 3 \cdot 10^{23} \; \text{stars}[/math] Observable Universe total galaxy number: [math]\boxed{N_g = \frac{\Omega_b \pi^3 k_B^4}{12 M_{mw} c^2 \left(\hbar H_0\right)^3} \left(\frac{N_{\phi} T_{\phi}^4}{C_{\phi}} \ln \left[\frac{T_{\phi,t}}{T_{\phi}}\right]^3 + \frac{N_{\gamma} T_{\gamma}^4}{15} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\gamma}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]^3 + \frac{N_{\nu} T_{\nu}^4}{C_{\nu}} \left(\frac{\Omega_b}{\Omega_b - \Omega_{\nu}}\right) \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3 + \frac{N_{\Lambda} T_{\Lambda}^4}{15} \ln \left[\frac{T_{\nu,t}}{T_{\nu}}\right]^3\right)}[/math] Observable Universe total galaxy number: [math]\boxed{N_g = 2.330 \cdot 10^{12} \; \text{galaxies}}[/math] Wikipedia observable universe total galaxy number: (ref. 8) [math]N_g = 2.000 \cdot 10^{12} \; \text{galaxies}[/math] Observable Universe stars per galaxy average number: [math]\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] [math]\boxed{\frac{N_s}{N_g} = \frac{\Omega_s M_{mw}}{\Omega_b M_{as}}}[/math] [math]\boxed{\frac{N_s}{N_g} = 1.053 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}}[/math] Wikipedia Milky Way galaxy total stellar number: (ref. 9) [math]\frac{N_s}{N_g} = 2.500 \cdot 10^{11} \pm 1.500 \cdot 10^{11} \; \frac{\text{stars}}{\text{galaxy}}[/math] Observable Universe planetary composition: (ref. 3, pg. 3) [math]\Omega_p = 1 \cdot 10^{-6}[/math] Wikipedia Milky Way galaxy total planetary number: (ref. 9, ref. 10) [math]\frac{N_p}{N_g} = \left(1.000 \cdot 10^{11} \rightarrow 1.600 \cdot 10^{11}\right) \; \frac{\text{planets}}{\text{galaxy}}[/math] Observable Universe average planetary mass: [math]\boxed{M_{ap} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{N_g}{N_p}\right)}[/math] [math]\boxed{M_{ap} = \left(52.974 \rightarrow 84.758\right) \cdot M_{\oplus}}[/math] Solar star system average planetary mass: (ref. 11) [math]\boxed{M_{ap} = \frac{}{n_p} \sum_{n = 1}^{n_p} M_n} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{M_{ap} = 55.855 \cdot M_{\oplus}}[/math] Milky Way galaxy total planetary number based upon solar star system: [math]\boxed{\frac{N_p}{N_g} = \frac{\Omega_p M_{mw}}{\Omega_b} \left(\frac{}{n_p} \sum_{n = 1}^{n_p} M_n\right)^{-1}} \; \; \; \; \; \; n_p = 8[/math] [math]\boxed{\frac{N_p}{N_g} = 1.518 \cdot 10^{11} \; \frac{\text{planets}}{\text{galaxy}}}[/math] Any discussions and/or peer reviews about this specific topic thread? Is there anything else that you want to see quantified based upon this model for observational comparison? Reference: Wikipedia - Stellar classification - Harvard spectral classification: (ref. 1) https://en.wikipedia.org/wiki/Stellar_classification#Harvard_spectral_classification On The Mass Distribution Of Stars...: (ref. 2) http://www.doiserbia.nb.rs/img/doi/1450-698X/2006/1450-698X0672017N.pdf The Cosmic Energy Inventory: (ref. 3) http://arxiv.org/pdf/astro-ph/0406095v2.pdf Mass models of the Milky Way: (ref. 4) http://arxiv.org/pdf/1102.4340v1 PHYS: 652 Cosmic Inventory I: Radiation: (ref. 5) http://www.nicadd.niu.edu/~bterzic/PHYS652/Lecture_09.pdf Wikipedia - neutrino decoupling: (ref. 6) https://en.wikipedia.org/wiki/Neutrino_decoupling Wikipedia - Observable universe total observable stellar number: (ref. 7) https://en.wikipedia.org/wiki/Star#Distribution Wikipedia - Galaxy: (ref. 8) https://en.wikipedia.org/wiki/Galaxy Wikipedia - Milky Way Galaxy: (ref. 9) https://en.wikipedia.org/wiki/Milky_Way Space.com - 160 Billion Alien Planets May Exist in Our Milky Way Galaxy: (ref. 10) https://www.space.com/14200-160-billion-alien-planets-milky-galaxy.html Wikipedia - Planetary mass: (ref. 11) https://en.wikipedia.org/wiki/Planetary_mass#Values_from_the_DE405_ephemeris

WMAP satellite cosmological parameters at photon decoupling time: (ref. 1) [math]\Omega_{dm,t} = 0.63[/math] Observable Universe dark matter scalar particle composition is equivalent to dark matter composition at photon decoupling time: [math]\boxed{\Omega_{\phi,t} = \Omega_{dm,t}}[/math] Scalar particle temperature is equivalent to cosmic neutrino background radiation temperature: [math]\boxed{T_{\phi} = T_{\nu}}[/math] Scalar particle decoupling temperature is equivalent to cosmic neutrino background radiation temperature at neutrino decoupling time: [math]\boxed{T_{\phi,t} = T_{\nu,t}}[/math] Observable Universe total scalar particle co-moving radius at present time: [math]\boxed{R_{\phi} = \left( \frac{c}{2 H_{0}} \right) \ln \left[ \frac{T_{\phi,t}}{T_{\phi}} \right]}[/math] [math]\boxed{R_{\phi} = 1.472 \cdot 10^{27} \; \text{m}} \; \; \; (155.635 \cdot 10^{9} \; \text{ly})[/math] Observable Universe total scalar particle co-moving radius at past time integration via substitution: [math]R_{\phi,t} = R_{\phi} \left( \frac{T_{\phi}}{T_{\phi,t}} \right) = \left( \frac{c}{2 H_{0}} \right) \ln \left[ \frac{T_{\phi,t}}{T_{\phi}} \right] \left( \frac{T_{\phi}}{T_{\phi,t}} \right) = 2.864 \cdot 10^{17} \; \text{m} \; \; \; (30.277 \; \text{ly})[/math] Observable Universe total scalar particle co-moving radius at past time: [math]\boxed{R_{\phi,t} = \left( \frac{c}{2 H_{0}} \right) \ln \left[ \frac{T_{\phi,t}}{T_{\phi}} \right] \left( \frac{T_{\phi}}{T_{\phi,t}} \right)}[/math] [math]\boxed{R_{\phi,t} = 2.864 \cdot 10^{17} \; \text{m}} \; \; \; (30.277 \; \text{ly})[/math] Bose-Einstein scalar particle decoupling time: [math]T_{u,\phi} = \frac{1}{H_{\phi,t}} = \frac{}{2 (k_B T_{\phi,t})^2} \sqrt{\frac{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}{G N_{\phi} \pi^3}} = 0.0255 \; \text{s}[/math] [math]\boxed{T_{u,\phi} = \frac{}{2 (k_B T_{\phi,t})^2} \sqrt{\frac{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}{G N_{\phi} \pi^3}}} \; \; \; m_{\phi} \neq 0[/math] [math]\boxed{T_{u,\phi} = 0.0255 \; \text{s}} \; \; \; m_{\phi} \neq 0[/math] [math]\Lambda[/math]CDM universe model semiemperical temperature-time scale factor: (ref. 2, pg. 18, eq. 2), (ref. 3) [math]\left( \frac{T_{\phi}}{T_{\phi,t}} \right)^{2} = \frac{T_{u,\phi}}{T_{u}} = T_{u,\phi} H_0[/math] [math]T_{u,\phi} = \frac{}{H_0} \left( \frac{T_{\phi}}{T_{\phi,t}} \right)^{2} = 0.0166 \; \text{s}[/math] [math]\Lambda[/math]CDM universe model semiemperical scalar particle decoupling time: [math]\boxed{T_{u,\phi} = \frac{}{H_0} \left( \frac{T_{\phi}}{T_{\phi,t}} \right)^{2}}[/math] [math]\boxed{T_{u,\phi} = 0.0166 \; \text{s}}[/math] Observable Universe expansion rate at scalar particle decoupling time integration via substitution: [math]\frac{dr}{dt} = \frac{R_{\phi,t}}{T_{u,\phi}} = \left[ \left( \frac{c}{2 H_{0}} \right) \ln \left[ \frac{T_{\phi,t}}{T_{\phi}} \right] \left( \frac{T_{\phi}}{T_{\phi,t}} \right) \right] 2 (k_B T_{\phi,t})^2 \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}} = 1.124 \cdot 10^{19} \; \frac{\text{m}}{\text{s}} \; \; \; (1187.8 \; \frac{\text{ly}}{\text{s}})[/math] Observable Universe expansion rate at scalar particle decoupling time: [math]\boxed{\frac{dr}{dt} = \sqrt{\frac{G N_{\phi} \pi^3}{3 C_{\phi} \Omega_{\phi,t} \hbar^3 c^5}} \left( \frac{c}{H_{0}} \right) \ln \left[ \frac{T_{\phi,t}}{T_{\phi}} \right] \left( \frac{T_{\phi}}{T_{\phi,t}} \right) (k_B T_{\phi,t})^2}[/math] [math]\boxed{\frac{dr}{dt} = 1.124 \cdot 10^{19} \; \frac{\text{m}}{\text{s}}} \; \; \; (1187.8 \; \frac{\text{ly}}{\text{s}})[/math] Is it possible for dark matter cosmic scalar particle background radiation to have inflated to a distance of 156 billion light-years? Did the universe have a co-moving radius of 30 light-years at scalar particle decoupling time? Did the universe inflate and expand at a rate of 1188 light-years per second at scalar particle decoupling time? Is dark matter composed of scalar particles? Any discussions and/or peer reviews about this specific topic thread? Reference: WMAP satellite content of the Universe: (ref. 1) http://map.gsfc.nasa.gov/media/080998/index.html Cosmology: nucleosynthesis and inflation: (ref. 2) http://www.uio.no/studier/emner/matnat/astro/AST1100/h07/undervisningsmateriale/lecture25.pdf Wikipedia - Lambda-CDM model: (ref. 3) https://en.wikipedia.org/wiki/Lambda-CDM_model Wikipedia - Dark matter: https://en.wikipedia.org/wiki/Dark_matter Wikipedia - Inflation cosmology: https://en.wikipedia.org/wiki/Inflation_(cosmology) Wikipedia - Metric space expansion cosmology: https://en.wikipedia.org/wiki/Metric_expansion_of_space

WMAP satellite cosmological parameters at photon decoupling time: (ref. 1) [math]\Omega_{\gamma,t} = 0.15[/math] Observable Universe cosmology scale factor: [math]\boxed{\frac{R_u \left(t_0 \right)}{R_u \left(t \right)} = \frac{a \left(t_0 \right)}{a \left(t \right)} = \frac{T_t}{T_0} = 1 + z}[/math] Observable Universe total photon co-moving radius at present time: [math]\boxed{R_{\gamma} = \left( \frac{c}{2 H_{0}} \right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]}[/math] [math]\boxed{R_{\gamma} = 4.606 \cdot 10^{26} \; \text{m}} \; \; \; \left( 48.689 \cdot 10^{9} \; \text{ly} \right)[/math] Observable Universe total photon co-moving radius at past time integration via substitution: [math]R_{\gamma,t} = R_{\gamma} \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right) = \left( \frac{c}{2 H_{0}} \right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right] \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right) = 4.220 \cdot 10^{23} \; \text{m} \; \; \; \left( 44.610 \; \cdot 10^{6} \; \text{ly} \right)[/math] Observable Universe total photon co-moving radius at past time: [math]\boxed{R_{\gamma,t} = \left( \frac{c}{2 H_{0}} \right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right] \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right)}[/math] [math]\boxed{R_{\gamma,t} = 4.220 \cdot 10^{23} \; \text{m}} \; \; \; \left( 44.610 \; \cdot 10^{6} \; \text{ly} \right)[/math] Photon decoupling time: [math]\boxed{T_{u,\gamma} = \frac{3}{ \left(2 k_B T_{\gamma,t} \right)^2} \sqrt{\frac{5 \Omega_{\gamma,t} \hbar^3 c^5}{G N_{\gamma} \pi^3}}} \; \; \; m_{\gamma} = 0[/math] [math]\boxed{T_{u,\gamma} = 1.009 \cdot 10^{13} \; \text{s}} \; \; \; \left( 3.197 \cdot 10^{5} \; \text{years} \right) \; \; \; m_{\gamma} = 0[/math] [math]\Lambda[/math]CDM universe model semiemperical temperature-time scale factor: (ref. 2, pg. 19, eq. 1, ref. 3) [math]\left( \frac{T_{\gamma}}{T_{\gamma,t}} \right)^{\frac{3}{2}} = \frac{T_{u,\gamma}}{T_{u}} = T_{u,\gamma} H_0[/math] [math]T_{u,\gamma} = \frac{}{H_0} \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right)^{\frac{3}{2}} = 1.218 \cdot 10^{13} \; \text{s} \; \; \; \left( 3.861 \cdot 10^{5} \; \text{years} \right)[/math] [math]\Lambda[/math]CDM universe model semiemperical photon decoupling time: [math]\boxed{T_{u,\gamma} = \frac{}{H_0} \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right)^{\frac{3}{2}}}[/math] [math]\boxed{T_{u,\gamma} = 1.218 \cdot 10^{13} \; \text{s}} \; \; \; \left(3.861 \cdot 10^{5} \; \text{years}\right)[/math] Observable Universe expansion rate at photon decoupling time integration via substitution: [math]\frac{dr}{dt} = \frac{R_{\gamma,t}}{T_{u,\gamma}} = \left[ \left(\frac{c}{2 H_{0}} \right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right] \left( \frac{T_{\gamma}}{T_{\gamma,t}} \right)\right] \frac{ \left(2 k_B T_{\gamma,t} \right)^2}{3} \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t} \hbar^3 c^5}} = 4.184 \cdot 10^{10} \; \frac{\text{m}}{\text{s}} \; \; \; \left( 139.557 \cdot c \; \; \; 4.422 \cdot 10^{-6} \; \frac{\text{ly}}{\text{s}} \right)[/math] Observable Universe expansion rate at photon decoupling time: [math]\boxed{\frac{dr}{dt} = \frac{2}{3} \sqrt{\frac{G N_{\gamma} \pi^3}{5 \Omega_{\gamma,t} \hbar^3 c^5}} \left(\frac{c}{H_{0}}\right) \ln \left[\frac{T_{\gamma,t}}{T_{\gamma}}\right]\left(\frac{T_{\gamma}}{T_{\gamma,t}}\right)\left(k_B T_{\gamma,t}\right)^2}[/math] [math]\boxed{\frac{dr}{dt} = 4.184 \cdot 10^{10} \; \frac{\text{m}}{\text{s}}} \; \; \; \left( 139.557 \cdot c \; \; \; 4.422 \cdot 10^{-6} \; \frac{\text{ly}}{\text{s}} \right)[/math] Does the universe have a photon co-moving radius of 49 billion light-years at present time? Did the universe have a co-moving radius of 45 million light-years at photon decoupling time? Did the universe inflate and expand at a rate of 140 c or 4 micro light-years per second at photon decoupling time? Any discussions and/or peer reviews about this specific topic thread? Reference: WMAP satellite content of the Universe: (ref. 1) http://map.gsfc.nasa.gov/media/080998/index.html Cosmology: nucleosynthesis and inflation: (ref. 2) http://www.uio.no/studier/emner/matnat/astro/AST1100/h07/undervisningsmateriale/lecture25.pdf Wikipedia - Lambda-CDM_model parameters: (ref. 3) https://en.wikipedia.org/wiki/Lambda-CDM_model#Parameters Wikipedia - Inflation cosmology: https://en.wikipedia.org/wiki/Inflation_(cosmology) Wikipedia - Metric space expansion cosmology: https://en.wikipedia.org/wiki/Metric_expansion_of_space